Integrals from trigonometric functions.
Solution examples

In this lesson, we will consider the integrals of trigonometric functions, that is, the filling of the integrals will be sines, cosines, tangents and cotangents in various combinations. All examples will be analyzed in detail, accessible and understandable even for a teapot.

To successfully study integrals of trigonometric functions, you must be well versed in the simplest integrals, as well as master some integration techniques. You can get acquainted with these materials at the lectures. Indefinite integral. Solution examples and .

And now we need: Table of integrals, Derivative table and Reference book of trigonometric formulas. All teaching aids can be found on the page Mathematical formulas and tables. I recommend printing everything. I especially focus on trigonometric formulas, they should be in front of your eyes– without it, the efficiency of work will noticeably decrease.

But first, about which integrals in this article No. Here there are no integrals of the form , - cosine, sine multiplied by some polynomial (less often, something with a tangent or cotangent). Such integrals are integrated by parts, and to learn the method, visit the lesson Integration by parts. Examples of solutions. Also, there are no integrals with "arches" - arc tangent, arc sine, etc., they are also most often integrated by parts.

When finding integrals of trigonometric functions, a number of methods are used:

(4) Use the tabular formula , the only difference is that instead of "x" we have a complex expression.

Example 2

Example 3

Find the indefinite integral.

A classic of the genre for those who are drowning in the standings. As you probably noticed, there is no integral of tangent and cotangent in the table of integrals, but, nevertheless, such integrals can be found.

(1) We use the trigonometric formula

(2) We bring the function under the sign of the differential.

(3) Use the tabular integral .

Example 4

Find the indefinite integral.

This is an example for self-solving, the full solution and answer are at the end of the lesson.

Example 5

Find the indefinite integral.

Our levels will gradually increase =).
Solution first:

(1) We use the formula

(2) We use the basic trigonometric identity , from which it follows that .

(3) Divide the numerator by the denominator term by term.

(4) We use the property of linearity of the indefinite integral.

(5) We integrate using the table.

Example 6

Find the indefinite integral.

This is an example for self-solving, the full solution and answer are at the end of the lesson.

There are also integrals of tangents and cotangents, which are in more high degrees. The integral of the tangent in the cube is considered in the lesson How to calculate the area of ​​a plane figure? Integrals of the tangent (cotangent) in the fourth and fifth powers can be obtained on the page Complex integrals.

Reducing the degree of the integrand

This technique works when the integrands are stuffed with sines and cosines in even degrees. Trigonometric formulas are used to reduce the degree , and , and the last formula is more often used in the opposite direction: .

Example 7

Find the indefinite integral.

Decision:

In principle, there is nothing new here, except that we have applied the formula (lowering the degree of the integrand). Please note that I have shortened the solution. As experience is gained, the integral of can be found orally, this saves time and is quite acceptable when finishing assignments. In this case, it is advisable not to write the rule , first we verbally take the integral of 1, then - of .

Example 8

Find the indefinite integral.

This is an example for self-solving, the full solution and answer are at the end of the lesson.

The promised increase in degree:

Example 9

Find the indefinite integral.

Solution first, comments later:

(1) Prepare the integrand to apply the formula .

(2) We actually apply the formula.

(3) We square the denominator and take the constant out of the integral sign. It could be done a little differently, but, in my opinion, it's more convenient.

(4) We use the formula

(5) In the third term, we again lower the degree, but using the formula .

(6) We give like terms (here I divided term by term and did the addition).

(7) We actually take the integral, the linearity rule and the method of bringing the function under the sign of the differential is performed orally.

(8) We comb the answer.

! In the indefinite integral, the answer can often be written in several ways.

In the example just considered, the final answer could be written differently - open the brackets and even do this before integrating the expression, that is, the following ending of the example is quite acceptable:

It is possible that this option is even more convenient, I just explained it the way I used to decide myself). Here is another typical example for an independent solution:

Example 10

Find the indefinite integral.

This example is solved in two ways, and you can get two completely different answers.(more precisely, they will look completely different, but from a mathematical point of view they will be equivalent). Most likely, you will not see the most rational way and will suffer with opening brackets, using other trigonometric formulas. The most effective solution is given at the end of the lesson.

Summing up the paragraph, we conclude that any integral of the form , where and - even number, is solved by lowering the degree of the integrand.
In practice, I met integrals with 8 and 10 degrees, I had to solve their terrible hemorrhoids by lowering the degree several times, resulting in long, long answers.

Variable replacement method

As mentioned in the article Variable change method in indefinite integral, the main prerequisite for using the replacement method is the fact that the integrand contains some function and its derivative :
(functions are not necessarily in the product)

Example 11

Find the indefinite integral.

We look at the table of derivatives and notice the formulas, , that is, in our integrand there is a function and its derivative. However, we see that when differentiating, cosine and sine mutually transform into each other, and the question arises: how to make a change of variable and what to designate for - sine or cosine ?! The question can be solved by the method of scientific poke: if we do the replacement incorrectly, then nothing good will come of it.

General guideline: in similar cases, you need to denote the function that is in the denominator.

We interrupt the solution and carry out a replacement

In the denominator, everything is fine with us, everything depends only on , now it remains to find out what it will turn into.
To do this, we find the differential:

Or, in short:
From the resulting equality, according to the rule of proportion, we express the expression we need:

So:

Now the entire integrand depends only on and we can continue the solution

Ready. I remind you that the purpose of the replacement is to simplify the integrand, in this case it all comes down to integration power function according to the table.

It was not by chance that I painted this example in such detail, this was done in order to repeat and consolidate the lesson materials. Variable change method in indefinite integral.

And now two examples for an independent solution:

Example 12

Find the indefinite integral.

Example 13

Find the indefinite integral.

Complete solutions and answers at the end of the lesson.

Example 14

Find the indefinite integral.

Here again, in the integrand, there is a sine with a cosine (a function with a derivative), but already in the product, and a dilemma arises - what should be denoted for, sine or cosine?

You can try to make a replacement using the scientific poke method, and if nothing works, then designate it as another function, but there is:

General guideline: for you need to designate the function that, figuratively speaking, is in an "uncomfortable position".

We see that in this example, the student cosine "suffers" from the degree, and the sine sits freely like that, on its own.

So let's make a substitution:

If anyone still has difficulties with the variable change algorithm and finding the differential, then you should return to the lesson Variable change method in indefinite integral.

Example 15

Find the indefinite integral.

We analyze the integrand, what should be denoted by ?
Let's take a look at our guidelines:
1) The function is most likely in the denominator;
2) The function is in an "uncomfortable position".

By the way, these guidelines are valid not only for trigonometric functions.

Under both criteria (especially under the second one), the sine fits, so a replacement suggests itself. In principle, the replacement can already be carried out, but first it would be nice to figure out what to do with? First, we “pin off” one cosine:

We reserve for our "future" differential

And we express through the sine using the basic trigonometric identity:

Now here's the replacement:

General rule: If in the integrand one of the trigonometric functions (sine or cosine) is in odd degree, then you need to “bite off” one function from the odd degree, and designate another function behind. We are talking only about integrals, where there are cosines and sines.

In the example considered, we had a cosine in an odd degree, so we pinched off one cosine from the degree, and denoted the sine.

Example 16

Find the indefinite integral.

The levels are going up =).
This is a do-it-yourself example. Complete Solution and the answer at the end of the lesson.

Universal trigonometric substitution

Universal trigonometric substitution is a common case of the change of variable method. You can try to apply it when you "do not know what to do." But in fact, there are some guidelines for its application. Typical integrals where the universal trigonometric substitution needs to be applied are the following integrals: , , , etc.

Example 17

Find the indefinite integral.

The universal trigonometric substitution in this case is implemented in the following way. Let's replace: . I do not use the letter , but the letter , this is not some kind of rule, just again, I'm so used to deciding.

Here it is more convenient to find the differential, for this, from the equality, I express:
I hang on both parts of the arc tangent:

Arctangent and tangent cancel each other out:

Thus:

In practice, you can not paint in such detail, but simply use the finished result:

! The expression is valid only if under the sines and cosines we just have “xes”, for the integral (which we will talk about later) everything will be a little different!

When replacing sines and cosines, we turn into the following fractions:
, , these equalities are based on well-known trigonometric formulas: ,

So the cleanup could look like this:

Let's carry out a universal trigonometric substitution:

Examples of solutions of integrals by parts are considered in detail, the integrand of which is the product of a polynomial and an exponential (e to the power of x) or a sine (sin x) or a cosine (cos x).

Content

See also: Method of integration by parts
Table of indefinite integrals
Methods for calculating indefinite integrals
Basic elementary functions and their properties

Integration by parts formula

When solving the examples in this section, the formula for integration by parts is used:
;
.

Examples of integrals containing the product of a polynomial and sin x, cos x, or e x

Here are examples of such integrals:
, , .

To integrate such integrals, the polynomial is denoted by u and the remainder by v dx . Next, the integration-by-parts formula is applied.

Below is given detailed solution these examples.

Examples of solving integrals

Example with exponent, e to the power of x

Define integral:
.

We introduce the exponent under the differential sign:
e - x dx = - e - x d(-x) = - d(e - x).

We integrate by parts.

here
.
The remaining integral is also integrable by parts.
.
.
.
Finally we have:
.

An example of defining an integral with a sine

Calculate integral:
.

We introduce the sine under the sign of the differential:

We integrate by parts.

here u = x 2 , v = cos(2x+3), du = ( x2 )′ dx

The remaining integral is also integrable by parts. To do this, we introduce the cosine under the sign of the differential.


here u = x, v = sin(2x+3), du = dx

Finally we have:

An example of the product of a polynomial and cosine

Calculate integral:
.

We introduce the cosine under the sign of the differential:

We integrate by parts.

here u = x 2+3x+5, v = sin2x, du = ( x 2 + 3 x + 5 )′ dx

Table of antiderivatives ("integrals"). Table of integrals. Tabular indefinite integrals. (Simple integrals and integrals with a parameter). Formulas for integration by parts. Newton-Leibniz formula.

Table of antiderivatives ("integrals"). Tabular indefinite integrals. (Simple integrals and integrals with a parameter).
Power function integral.	Power function integral.
An integral that reduces to an integral of a power function if x is driven under the sign of the differential.
	The exponential integral, where a is a constant number.
Complex integral exponential function.	The integral of the exponential function.
An integral equal to the natural logarithm.	Integral: "Long logarithm".
	Integral: "Long logarithm".
Integral: "High logarithm".	The integral, where x in the numerator is brought under the sign of the differential (the constant under the sign can be both added and subtracted), as a result, is similar to the integral equal to the natural logarithm.
Integral: "High logarithm".
Cosine integral.	Sine integral.
An integral equal to the tangent.	An integral equal to the cotangent.
Integral equal to both arcsine and arcsine
An integral equal to both the inverse sine and the inverse cosine.	An integral equal to both the arc tangent and the arc cotangent.
The integral is equal to the cosecant.	Integral equal to secant.
An integral equal to the arcsecant.	An integral equal to the arc cosecant.
An integral equal to the arcsecant.	An integral equal to the arcsecant.
An integral equal to the hyperbolic sine.	An integral equal to the hyperbolic cosine.
An integral equal to the hyperbolic sine, where sinhx is the hyperbolic sine in English.	An integral equal to the hyperbolic cosine, where sinhx is the hyperbolic sine in the English version.
An integral equal to the hyperbolic tangent.	An integral equal to the hyperbolic cotangent.
An integral equal to the hyperbolic secant.	An integral equal to the hyperbolic cosecant.

Formulas for integration by parts. Integration rules.

Formulas for integration by parts. Newton-Leibniz formula. Integration rules.
Integration of a product (function) by a constant:
Integration of the sum of functions:
indefinite integrals:
Integration by parts formula definite integrals:
Newton-Leibniz formula definite integrals:	Where F(a),F(b) are the values ​​of the antiderivatives at the points b and a, respectively.

Derivative table. Table derivatives. Derivative of the product. Derivative of private. Derivative of a complex function.

If x is an independent variable, then:

Derivative table. Table derivatives. "table derivative" - ​​yes, unfortunately, that's how they are searched on the Internet
	Power function derivative
	Derivative of the exponent
Derivative of a compound exponential function	Derivative of exponential function
Derivative of a logarithmic function	Derivative of the natural logarithm
	Derivative of the natural logarithm of a function
Sine derivative	cosine derivative
Cosecant derivative	Secant derivative
Derivative of arcsine	Arc cosine derivative
Derivative of arcsine	Arc cosine derivative
Tangent derivative	Cotangent derivative
Arc tangent derivative	Derivative of inverse tangent
Arc tangent derivative	Derivative of inverse tangent
Arcsecant derivative	Derivative of arc cosecant
Arcsecant derivative	Derivative of arc cosecant
Derivative of the hyperbolic sine Derivative of the hyperbolic sine in the English version	Hyperbolic cosine derivative The derivative of the hyperbolic cosine in the English version
Derivative of the hyperbolic tangent	Derivative of the hyperbolic cotangent
Derivative of hyperbolic secant	Derivative of the hyperbolic cosecant

Differentiation rules. Derivative of the product. Derivative of private. Derivative of a complex function.
Derivative of a product (function) by a constant:
Derivative of the sum (functions):
Derivative of the product (of functions):
The derivative of the quotient (of functions):
Derivative of a complex function:

Properties of logarithms. Basic formulas of logarithms. Decimal (lg) and natural logarithms (ln).

Basic logarithmic identity
Let us show how any function of the form a b can be made exponential. Since a function of the form e x is called exponential, then
Any function of the form a b can be represented as a power of ten

Natural logarithm ln (logarithm base e = 2.718281828459045…) ln(e)=1; log(1)=0

Taylor series. Expansion of a function in a Taylor series.

It turns out that most practically occurring mathematical functions can be represented with any accuracy in the vicinity of a certain point in the form of power series containing the powers of the variable in ascending order. For example, in the vicinity of the point x=1:

When using rows called taylor rows, mixed functions containing, say, algebraic, trigonometric, and exponential functions can be expressed as purely algebraic functions. With the help of series, differentiation and integration can often be quickly carried out.

The Taylor series in the vicinity of the point a has the following forms:

1) , where f(x) is a function that has derivatives of all orders at x=a. R n - the remainder term in the Taylor series is determined by the expression

2)

k-th coefficient (at x k) of the series is determined by the formula

3) A special case of the Taylor series is the Maclaurin series (=McLaren) (the decomposition takes place around the point a=0)

for a=0

the members of the series are determined by the formula

Conditions for the application of Taylor series.

1. In order for the function f(x) to be expanded in a Taylor series on the interval (-R;R), it is necessary and sufficient that the remainder term in the Taylor formula (Maclaurin (=McLaren)) for this function tends to zero at k →∞ on the specified interval (-R;R).

2. It is necessary that there are derivatives for this function at the point in the vicinity of which we are going to build a Taylor series.

Properties of Taylor series.

If f is an analytic function, then its Taylor series at any point a of the domain of f converges to f in some neighborhood of a.

There are infinitely differentiable functions whose Taylor series converges but differs from the function in any neighborhood of a. For example:

Taylor series are used for approximation (an approximation is a scientific method that consists in replacing some objects with others, in one sense or another close to the original, but simpler) functions by polynomials. In particular, linearization ((from linearis - linear), one of the methods of approximate representation of closed nonlinear systems, in which the study of a nonlinear system is replaced by an analysis of a linear system, in a sense equivalent to the original one.) of equations occurs by expanding into a Taylor series and cutting off all the terms above first order.

Thus, almost any function can be represented as a polynomial with a given accuracy.

Examples of some common expansions of power functions in Maclaurin series (=McLaren,Taylor in the vicinity of point 0) and Taylor in the vicinity of point 1. The first terms of expansions of the main functions in Taylor and MacLaren series.

Examples of some common expansions of power functions in Maclaurin series (= MacLaren, Taylor in the vicinity of the point 0)

Examples of some common Taylor series expansions around point 1

There will also be tasks for an independent solution, to which you can see the answers.

The integrand can be converted from a product of trigonometric functions to a sum

Consider integrals in which the integrand is the product of sines and cosines of the first degree of x multiplied by different factors, that is, integrals of the form

Taking advantage of the well-known trigonometric formulas

(2)
(3)
(4)
it is possible to transform each of the products in integrals of the form (31) into algebraic sum and integrate by the formulas

(5)

(6)

Example 1 To find

Decision. According to formula (2) at

Example 2 To find integral of trigonometric function

Decision. According to formula (3) at

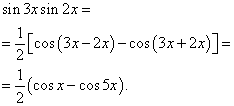
Example 3 To find integral of trigonometric function

Decision. According to formula (4) at we obtain the following transformation of the integrand:

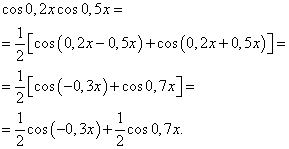
Applying formula (6), we obtain

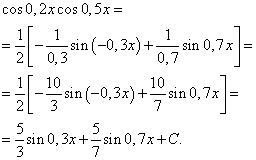
Integral of the product of powers of sine and cosine of the same argument

Let us now consider the integrals of functions that are the product of the powers of the sine and cosine of the same argument, i.e.

(7)

In particular cases, one of the indicators ( m or n) may be zero.

When integrating such functions, it is used that the even power of the cosine can be expressed in terms of the sine, and the differential of the sine is equal to cos x dx(or an even power of the sine can be expressed in terms of cosine, and the cosine differential is - sin x dx ) .

Two cases should be distinguished: 1) at least one of the indicators m and n odd; 2) both indicators are even.

Let the first case take place, namely the exponent n = 2k+ 1 - odd. Then, considering that

The integrand is presented in such a way that one part of it is a function of only the sine, and the other is the differential of the sine. Now with the change of variable t= sin x the solution is reduced to integrating the polynomial with respect to t. If only the degree m is odd, then do the same, separating the factor sin x, expressing the rest of the integrand in terms of cos x and assuming t= cos x. This approach can also be used when integration of partial powers of sine and cosine , when at least one of the indicators is odd . The whole point is that the quotient of the powers of sine and cosine is special case their works : when the trigonometric function is in the denominator of the integrand, its degree is negative. But there are also cases of partial trigonometric functions, when their degrees are only even. About them - the next paragraph.

If both indicators m and n are even, then using trigonometric formulas

lower the exponents of the sine and cosine, after which an integral of the same type as above will be obtained. Therefore, the integration should be continued in the same way. If one of the even indicators is negative, that is, the quotient is considered. even powers sine and cosine, then this scheme is not suitable . Then a change of variable is used, depending on how the integrand can be transformed. Such a case will be considered in the next section.

Example 4 To find integral of trigonometric function

Decision. The exponent of the cosine is odd. Therefore, imagine

t= sin x(then dt= cos x dx ). Then we get

Returning to the old variable, we finally find

Example 5 To find integral of trigonometric function

.

Decision. The exponent of the cosine, as in the previous example, is odd, but more. Imagine

and make the change of variable t= sin x(then dt= cos x dx ). Then we get

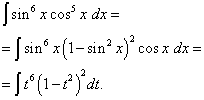
Let's open the brackets

and get

Returning to the old variable, we obtain the solution

Example 6 To find integral of trigonometric function

Decision. The exponents of sine and cosine are even. Therefore, we transform the integrand as follows:

Then we get

In the second integral, we make a change of variable, setting t= sin2 x. Then (1/2)dt= cos2 x dx . Hence,

Finally we get

Using the Variable Replace Method

Variable replacement method when integrating trigonometric functions, it can be used in cases where only a sine or only a cosine is present in the integrand, the product of sine and cosine, in which either sine or cosine is in the first degree, tangent or cotangent, as well as the quotient of even powers of sine and cosine of one and the same argument. In this case, it is possible to perform permutations not only sin x = t and sin x = t, but also tg x = t and ctg x = t .

Example 8 To find integral of trigonometric function

.

Decision. Let's change the variable: , then . The resulting integrand is easily integrated over the table of integrals:

.

Example 9 To find integral of trigonometric function

Decision. Let's convert the tangent to the ratio of sine and cosine:

Let's change the variable: , then . The resulting integrand is table integral with minus sign:

.

Returning to the original variable, we finally get:

.

Example 10 To find integral of trigonometric function

Decision. Let's change the variable: , then .

We transform the integrand to apply the trigonometric identity :

We make a change of variable, not forgetting to put a minus sign in front of the integral (see above, what is equal to dt). Next, we decompose the integrand into factors and integrate according to the table:

Returning to the original variable, we finally get:

.

Find the integral of the trigonometric function yourself, and then see the solution

Universal trigonometric substitution

Universal trigonometric substitution can be used in cases where the integrand does not fall under the cases discussed in the previous paragraphs. Basically when the sine or cosine (or both) is in the denominator of a fraction. It is proved that the sine and cosine can be replaced by another expression containing the tangent of half the original angle as follows:

But note that the universal trigonometric substitution often entails rather complex algebraic transformations, so it is best used when no other method works. Let's look at examples when, together with the universal trigonometric substitution, subtraction under the sign of the differential and the method of indefinite coefficients are used.

Example 12. To find integral of trigonometric function

.

Decision. Decision. Let's use universal trigonometric substitution. Then
.

We multiply the fractions in the numerator and denominator by , and take out the deuce and put it in front of the integral sign. Then