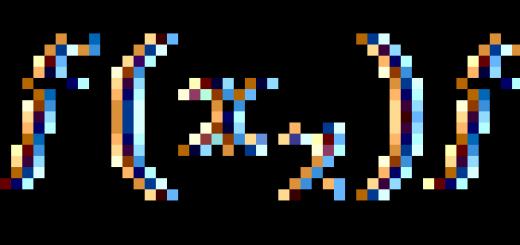The simplest trigonometric equations are usually solved by formulas. Let me remind you that the following trigonometric equations are called the simplest:
sinx = a
cosx = a
tgx = a
ctgx = a
x is the angle to be found,
a is any number.
And here are the formulas with which you can immediately write down the solutions of these simplest equations.
For sinus:
For cosine:
x = ± arccos a + 2π n, n ∈ Z
For tangent:
x = arctg a + π n, n ∈ Z
For cotangent:
x = arcctg a + π n, n ∈ Z
Actually, this is theoretical part solutions of the simplest trigonometric equations. And, the whole!) Nothing at all. However, the number of errors on this topic just rolls over. Especially, with a slight deviation of the example from the template. Why?
Yes, because a lot of people write down these letters, without understanding their meaning at all! With apprehension he writes down, no matter how something happens ...) This needs to be sorted out. Trigonometry for people, or people for trigonometry, after all!?)
Let's figure it out?
One angle will be equal to arccos a, second: -arccos a.
And that's how it will always work. For any a.
If you don't believe me, hover your mouse over the picture, or touch the picture on your tablet.) I changed the number a to some negative. Anyway, we got one corner arccos a, second: -arccos a.
Therefore, the answer can always be written as two series of roots:
x 1 = arccos a + 2π n, n ∈ Z
x 2 = - arccos a + 2π n, n ∈ Z
We combine these two series into one:
x= ± arccos a + 2π n, n ∈ Z
And all things. We have obtained a general formula for solving the simplest trigonometric equation with cosine.
If you understand that this is not some kind of super-scientific wisdom, but just an abbreviated record of two series of answers, you and tasks "C" will be on the shoulder. With inequalities, with the selection of roots from a given interval ... There, the answer with plus / minus does not roll. And if you treat the answer businesslike, and break it into two separate answers, everything is decided.) Actually, for this we understand. What, how and where.
In the simplest trigonometric equation
sinx = a
also get two series of roots. Always. And these two series can also be recorded one line. Only this line will be smarter:
x = (-1) n arcsin a + π n, n ∈ Z
But the essence remains the same. Mathematicians simply constructed a formula to make one instead of two records of series of roots. And that's it!
Let's check the mathematicians? And that's not enough...)
In the previous lesson, the solution (without any formulas) of the trigonometric equation with a sine was analyzed in detail:
The answer turned out to be two series of roots:
x 1 = π /6 + 2π n, n ∈ Z
x 2 = 5π /6 + 2π n, n ∈ Z
If we solve the same equation using the formula, we get the answer:
x = (-1) n arcsin 0.5 + π n, n ∈ Z
Actually, this is a half-finished answer.) The student must know that arcsin 0.5 = π /6. The full answer would be:
x = (-1) n π /6+ πn, n ∈ Z
Here an interesting question arises. Reply via x 1; x 2 (this is the correct answer!) and through the lonely X (and this is the correct answer!) - the same thing, or not? Let's find out now.)
Substitute in response with x 1 values n =0; one; 2; etc., we consider, we get a series of roots:
x 1 \u003d π / 6; 13π/6; 25π/6 etc.
With the same substitution in response to x 2 , we get:
x 2 \u003d 5π / 6; 17π/6; 29π/6 etc.
And now we substitute the values n (0; 1; 2; 3; 4...) into the general formula for the lonely X . That is, we raise minus one to the zero power, then to the first, second, and so on. And, of course, we substitute 0 into the second term; one; 2 3; 4 etc. And we think. We get a series:
x = π/6; 5π/6; 13π/6; 17π/6; 25π/6 etc.
That's all you can see.) The general formula gives us exactly the same results which are the two answers separately. All at once, in order. Mathematicians did not deceive.)
Formulas for solving trigonometric equations with tangent and cotangent can also be checked. But let's not.) They are so unpretentious.
I painted all this substitution and verification on purpose. Here it is important to understand one simple thing: there are formulas for solving elementary trigonometric equations, just a summary of the answers. For this brevity, I had to insert plus/minus into the cosine solution and (-1) n into the sine solution.
These inserts do not interfere in any way in tasks where you just need to write down the answer to an elementary equation. But if you need to solve an inequality, or then you need to do something with the answer: select roots on an interval, check for ODZ, etc., these inserts can easily unsettle a person.
And what to do? Yes, either paint the answer in two series, or solve the equation / inequality in a trigonometric circle. Then these inserts disappear and life becomes easier.)
You can sum up.
To solve the simplest trigonometric equations, there are ready-made answer formulas. Four pieces. They are good for instantly writing the solution to an equation. For example, you need to solve the equations:
sinx = 0.3
Easily: x = (-1) n arcsin 0.3 + π n, n ∈ Z
cosx = 0.2
No problem: x = ± arccos 0.2 + 2π n, n ∈ Z
tgx = 1.2
Easily: x = arctg 1,2 + πn, n ∈ Z
ctgx = 3.7
One left: x= arcctg3,7 + πn, n ∈ Z
cos x = 1.8
If you, shining with knowledge, instantly write the answer:
x= ± arccos 1.8 + 2π n, n ∈ Z
then you already shine, this ... that ... from a puddle.) The correct answer is: there are no solutions. Don't understand why? Read what an arccosine is. In addition, if on the right side of the original equation there are tabular values \u200b\u200bof sine, cosine, tangent, cotangent, - 1; 0; √3; 1/2; √3/2 etc. - the answer through the arches will be unfinished. Arches must be converted to radians.
And if you already come across an inequality, like
then the answer is:
x πn, n ∈ Z
there is a rare nonsense, yes ...) Here it is necessary to trigonometric circle decide. What we will do in the corresponding topic.
For those who heroically read up to these lines. I just can't help but appreciate your titanic efforts. you a bonus.)
Bonus:
When writing formulas in an anxious combat situation, even hardened nerds often get confused where pn, And where 2πn. Here's a simple trick for you. In all formulas pn. Except for the only formula with arc cosine. It stands there 2πn. Two pien. Keyword - two. In the same single formula are two sign at the beginning. Plus and minus. Here and there - two.
So if you wrote two sign in front of the arc cosine, it is easier to remember what will happen at the end two pien. And vice versa happens. Skip the man sign ± , get to the end, write correctly two pien, yes, and catch it. Ahead of something two sign! The person will return to the beginning, but he will correct the mistake! Like this.)
If you like this site...
By the way, I have a couple more interesting sites for you.)
You can practice solving examples and find out your level. Testing with instant verification. Learning - with interest!)
you can get acquainted with functions and derivatives.
The simplest trigonometric equations are the equations
Cos(x)=a, sin(x)=a, tg(x)=a, ctg(x)=a
Equation cos(x) = a

Explanation and rationale
- The roots of the equation cosx = a. When | a | > 1 the equation has no roots because | cosx |< 1 для любого x (прямая y = а при а >1 or at a< -1 не пересекает график функцииy = cosx).
Let | a |< 1. Тогда прямая у = а пересекает график функции
y = cos x. On the interval, the function y = cos x decreases from 1 to -1. But a decreasing function takes each of its values only at one point in its domain, so cos equation x \u003d a has only one root on this interval, which, by definition of the arc cosine, is: x 1 \u003d arccos a (and for this root cos x \u003d a).
Cosine - even function, so on the interval [-n; 0] the equation cos x = and also has only one root - the number opposite to x 1, that is
x 2 = -arccos a.
Thus, on the interval [-n; n] (length 2n) the equation cos x = a for | a |< 1 имеет только корни x = ±arccos а.
The function y = cos x is periodic with a period of 2n, so all other roots differ from those found by 2np (n € Z). We get the following formula for the roots of the equation cos x = a when
x = ± arccos a + 2n, n £ Z.
- Particular cases of solving the equation cosx = a.
It is useful to remember the special notation for the roots of the equation cos x = a when
a \u003d 0, a \u003d -1, a \u003d 1, which can be easily obtained using the unit circle as a guide.
Since the cosine is equal to the abscissa of the corresponding point unit circle, we obtain that cos x = 0 if and only if the corresponding point of the unit circle is point A or point B.
Similarly, cos x = 1 if and only if the corresponding point of the unit circle is the point C, therefore,
x = 2πp, k € Z.
Also cos x \u003d -1 if and only if the corresponding point of the unit circle is the point D, thus x \u003d n + 2n,



Equation sin(x) = a


Explanation and rationale
- Roots sinx equations= a. When | a | > 1 the equation has no roots because | sinx |< 1 для любого x (прямая y = а на рисунке при а >1 or at a< -1 не пересекает график функции y = sinx).
The video course "Get an A" includes all the topics you need to successful delivery USE in mathematics for 60-65 points. Completely all tasks 1-13 of the Profile USE in mathematics. Also suitable for passing the Basic USE in mathematics. If you want to pass the exam with 90-100 points, you need to solve part 1 in 30 minutes and without mistakes!
Preparation course for the exam for grades 10-11, as well as for teachers. Everything you need to solve part 1 of the exam in mathematics (the first 12 problems) and problem 13 (trigonometry). And this is more than 70 points on the Unified State Examination, and neither a hundred-point student nor a humanist can do without them.
All the necessary theory. Quick Ways solutions, traps and secrets of the exam. All relevant tasks of part 1 from the Bank of FIPI tasks have been analyzed. The course fully complies with the requirements of the USE-2018.
The course contains 5 big topics, 2.5 hours each. Each topic is given from scratch, simply and clearly.
Hundreds of exam tasks. Text problems and probability theory. Simple and easy to remember problem solving algorithms. Geometry. Theory, reference material, analysis of all types of USE tasks. Stereometry. Cunning tricks for solving, useful cheat sheets, development of spatial imagination. Trigonometry from scratch - to task 13. Understanding instead of cramming. Visual explanation of complex concepts. Algebra. Roots, powers and logarithms, function and derivative. Base for solving complex problems of the 2nd part of the exam.
Your privacy is important to us. For this reason, we have developed a Privacy Policy that describes how we use and store your information. Please read our privacy policy and let us know if you have any questions.
Collection and use of personal information
Personal information refers to data that can be used to identify a specific person or contact him.
You may be asked to provide your personal information at any time when you contact us.
The following are some examples of the types of personal information we may collect and how we may use such information.
What personal information we collect:
- When you submit an application on the site, we may collect various information, including your name, phone number, address Email etc.
How we use your personal information:
- Collected by us personal information allows us to contact you and inform you about unique offers, promotions and other events and upcoming events.
- From time to time, we may use your personal information to send you important notices and communications.
- We may also use personal information for internal purposes, such as conducting audits, data analysis and various research in order to improve the services we provide and provide you with recommendations regarding our services.
- If you enter a prize draw, contest or similar incentive, we may use the information you provide to administer such programs.
Disclosure to third parties
We do not disclose information received from you to third parties.
Exceptions:
- In the event that it is necessary - in accordance with the law, judicial order, in legal proceedings, and / or based on public requests or requests from state bodies in the territory of the Russian Federation - disclose your personal information. We may also disclose information about you if we determine that such disclosure is necessary or appropriate for security, law enforcement, or other public interest reasons.
- In the event of a reorganization, merger or sale, we may transfer the personal information we collect to the relevant third party successor.
Protection of personal information
We take precautions - including administrative, technical and physical - to protect your personal information from loss, theft, and misuse, as well as from unauthorized access, disclosure, alteration and destruction.
Maintaining your privacy at the company level
To ensure that your personal information is secure, we communicate privacy and security practices to our employees and strictly enforce privacy practices.
The main methods for solving trigonometric equations are: reducing equations to the simplest ones (using trigonometric formulas), introduction of new variables, factorization. Let's consider their application with examples. Pay attention to the registration of the solution of trigonometric equations.
Necessary condition successful solution trigonometric equations is the knowledge of trigonometric formulas (topic 13 of work 6).
Examples.
1. Equations Reducing to the Simplest.
1) Solve the equation
Decision:
Answer:
2) Find the roots of the equation
(sinx + cosx) 2 = 1 – sinxcosx belonging to the segment .
Decision:
Answer:
2. Equations Reducing to Quadratic Equations.
1) Solve the equation 2 sin 2 x - cosx -1 = 0.
Decision: Using the formula sin 2 x \u003d 1 - cos 2 x, we get
Answer:
2) Solve the equation cos 2x = 1 + 4 cosx.
Decision: Using the formula cos 2x = 2 cos 2 x - 1, we get
Answer:
3) Decide tgx equation– 2ctgx + 1 = 0
Decision:
Answer:
3. Homogeneous equations
1) Solve the equation 2sinx - 3cosx = 0
Solution: Let cosx = 0, then 2sinx = 0 and sinx = 0 - a contradiction with the fact that sin 2 x + cos 2 x = 1. So cosx ≠ 0 and you can divide the equation by cosx. Get
Answer:
2) Solve the equation 1 + 7 cos 2 x = 3 sin 2x
Decision:
Using the formulas 1 = sin 2 x + cos 2 x and sin 2x = 2 sinxcosx, we get
sin2x + cos2x + 7cos2x = 6sinxcosx
sin2x - 6sinxcosx+ 8cos2x = 0
Let cosx = 0, then sin 2 x = 0 and sinx = 0 - a contradiction with the fact that sin 2 x + cos 2 x = 1.
So cosx ≠ 0 and we can divide the equation by cos 2 x .
Get
tg 2x – 6 tgx + 8 = 0
Denote tgx = y
y 2 – 6 y + 8 = 0
y 1 = 4; y2=2
a) tanx = 4, x= arctg4 + 2 k, k
b) tgx = 2, x= arctg2 + 2 k, k .
Answer: arctg4 + 2 k, arctan2 + 2 k, k
4. Equations of the form a sinx + b cosx = with, with≠ 0.
1) Solve the equation.
Decision:
Answer:
5. Equations Solved by Factorization.
1) Solve the equation sin2x - sinx = 0.
The root of the equation f (X) = φ ( X) can only serve as the number 0. Let's check this:
cos 0 = 0 + 1 - the equality is true.
The number 0 is the only root of this equation.
Answer: 0.