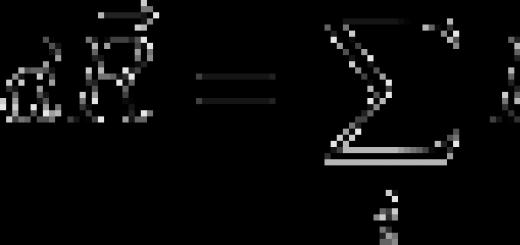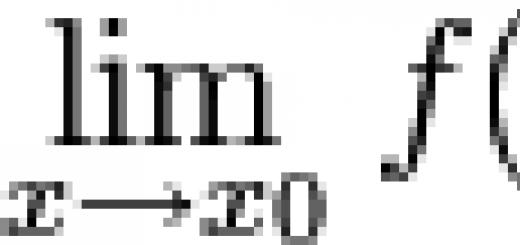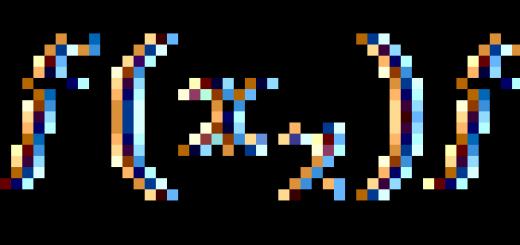Calculus of infinitesimals and large

Infinitesimal calculus- calculations performed with infinitesimal values, in which the derived result is considered as an infinite sum of infinitesimal ones. The calculus of infinitesimals is a general concept for differential and integral calculus, which form the basis of modern higher mathematics. The concept of an infinitesimal quantity is closely related to the concept of a limit.

Infinitesimal

Subsequence a n called infinitesimal, if . For example, a sequence of numbers is infinitely small.

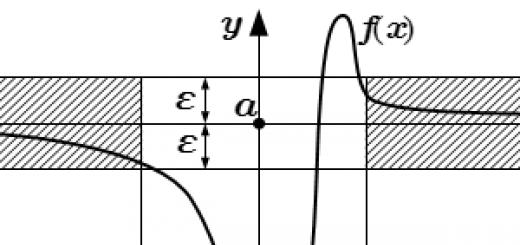
The function is called infinitesimal in a neighborhood of a point x 0 if .

The function is called infinitesimal at infinity, if or .

Also infinitely small is a function that is the difference between a function and its limit, that is, if , then f(x) − a = α( x) , .

infinitely large

Subsequence a n called infinitely large, if .

The function is called infinitely large in a neighborhood of a point x 0 if .

The function is called infinitely large at infinity, if or .

In all cases, infinity to the right of equality is assumed to have a certain sign (either "plus" or "minus"). That is, for example, the function x sin x is not infinitely large for .

Properties of infinitesimals and infinitesimals

Comparison of infinitesimals

How to compare infinitesimal quantities?
The ratio of infinitesimal quantities forms the so-called uncertainty.

Definitions

Suppose we have infinitely small for the same value α( x) and β( x) (or, which is not important for the definition, infinitesimal sequences).

To calculate such limits, it is convenient to use L'Hospital's rule.

Comparison examples

Using ABOUT-symbols of the results obtained can be written in the following form x 5 = o(x 3). In this case, the entries 2x 2 + 6x = O(x) And x = O(2x 2 + 6x).

Equivalent quantities

Definition

If , then infinitesimal quantities α and β are called equivalent ().
Obviously, equivalent quantities are a special case of infinitesimal quantities of the same order of smallness.

For , the following equivalence relations are valid: , , .

Theorem

The limit of the quotient (ratio) of two infinitesimal quantities will not change if one of them (or both) is replaced by an equivalent value.

This theorem is of practical importance in finding limits (see example).

Usage example

Replacing sin 2x equivalent value 2 x, we get

Historical outline

The concept of "infinitely small" was discussed in ancient times in connection with the concept of indivisible atoms, but did not enter classical mathematics. Again, it was revived with the advent in the 16th century of the "method of indivisibles" - the division of the figure under study into infinitesimal sections.

The algebraization of the infinitesimal calculus took place in the 17th century. They began to be defined as numerical values ​​that are less than any finite (non-zero) value and yet not equal to zero. The art of analysis consisted in drawing up a relation containing infinitesimals (differentials), and then in integrating it.

Old school mathematicians subjected the concept infinitesimal harsh criticism. Michel Rolle wrote that the new calculus is " set of brilliant mistakes»; Voltaire pointed out venomously that this calculus is the art of calculating and accurately measuring things whose existence cannot be proven. Even Huygens admitted that he did not understand the meaning of higher-order differentials.

The disputes in the Paris Academy of Sciences on the issues of justification of analysis became so scandalous that the Academy once forbade its members to speak on this topic at all (this mainly concerned Rolle and Varignon). In 1706, Rolle publicly withdrew his objections, but discussions continued.

In 1734, the famous English philosopher, Bishop George Berkeley, published a sensational pamphlet, known under the abbreviated title " Analyst". Its full name is: Analyst or discourse addressed to the unbelieving mathematician, investigating whether the subject, principles, and conclusions of modern analysis are more clearly perceived or more clearly deduced than the religious sacraments and articles of faith».

The Analyst contained a witty and in many respects fair criticism of the infinitesimal calculus. Berkeley considered the method of analysis to be inconsistent with logic and wrote that, " however useful it may be, it can only be regarded as a kind of conjecture; dexterity, art, or rather subterfuge, but not as a method of scientific proof". Quoting Newton's phrase about the increment of current quantities "at the very beginning of their birth or disappearance", Berkeley ironically: " they are neither finite, nor infinitesimal, nor even nothing. Could we not call them phantoms of deceased magnitudes?... And how can one speak at all about the relationship between things that have no magnitude?.. He who can digest the second or third flux [derivative], the second or third it seems to me to find fault with something in theology».

It is impossible, writes Berkeley, to imagine instantaneous speed, that is, speed at a given moment and at a given point, because the concept of motion includes concepts of (finite non-zero) space and time.

How does the analysis get the right results? Berkeley came to the conclusion that this is due to the presence of several errors in the analytical conclusions of mutual compensation, and illustrated this with the example of a parabola. Interestingly, some major mathematicians (for example, Lagrange) agreed with him.

There was a paradoxical situation when rigor and fruitfulness in mathematics interfered with each other. Despite the use of illegal actions with ill-defined concepts, the number of direct errors was surprisingly small - intuition helped out. And yet, throughout the 18th century, mathematical analysis developed rapidly, having essentially no justification. Its effectiveness was amazing and spoke for itself, but the meaning of the differential was still unclear. The infinitesimal increment of a function and its linear part were especially often confused.

Throughout the 18th century, tremendous efforts were made to correct the situation, and the best mathematicians of the century participated in them, but only Cauchy was able to convincingly build the foundation of analysis at the beginning of the 19th century. He strictly defined the basic concepts - limit, convergence, continuity, differential, etc., after which the actual infinitesimals disappeared from science. Some of the remaining subtleties explained later

Infinitely small functions

The function %%f(x)%% is called infinitesimal(b.m.) for %%x \to a \in \overline(\mathbb(R))%%, if the limit of the function is equal to zero when the argument tends to this.

The concept of b.m. function is inextricably linked with an indication of a change in its argument. We can talk about b.m. functions for %%a \to a + 0%% and for %%a \to a - 0%%. Usually b.m. functions are denoted by the first letters of the Greek alphabet %%\alpha, \beta, \gamma, \ldots%%

Examples

1. The function %%f(x) = x%% is b.m. at %%x \to 0%%, because its limit at %%a = 0%% is zero. According to the theorem on the connection between the two-sided limit and the one-sided limit, this function is b.m. both with %%x \to +0%% and with %%x \to -0%%.
2. Function %%f(x) = 1/(x^2)%% - b.m. with %%x \to \infty%% (as well as with %%x \to +\infty%% and with %%x \to -\infty%%).

A non-zero constant number, no matter how small in absolute value, is not a b.m. function. For constant numbers, the only exception is zero, since the function %%f(x) \equiv 0%% has a zero limit.

Theorem

The function %%f(x)%% has an end limit at the point %%a \in \overline(\mathbb(R))%% of the extended numeric line equal to the number %%b%% if and only if this function is equal to the sum of this number %%b%% and b.m. functions %%\alpha(x)%% with %%x \to a%%, or $$ \exists~\lim\limits_(x \to a)(f(x)) = b \in \mathbb(R ) \Leftrightarrow \left(f(x) = b + \alpha(x)\right) \land \left(\lim\limits_(x \to a)(\alpha(x) = 0)\right). $$

Properties of infinitesimal functions

According to the rules for passing to the limit, for %%c_k = 1~ \forall k = \overline(1, m), m \in \mathbb(N)%%, the following statements follow:

1. The sum of the final number b.m. functions for %%x \to a%% is f.m. with %%x \to a%%.
2. The product of any number of b.m. functions for %%x \to a%% is f.m. with %%x \to a%%.

The product of b.m. functions at %%x \to a%% and a function bounded in some punctured neighborhood %%\stackrel(\circ)(\text(U))(a)%% of the point a, is b.m. with %%x \to a%% function.

It is clear that the product of a constant function and b.m. at %%x \to a%% there is b.m. function at %%x \to a%%.

Equivalent infinitesimal functions

Infinitely small functions %%\alpha(x), \beta(x)%% for %%x \to a%% are called equivalent and are written %%\alpha(x) \sim \beta(x)%% if

$$ \lim\limits_(x \to a)(\frac(\alpha(x))(\beta(x))) = \lim\limits_(x \to a)(\frac(\beta(x) )(\alpha(x))) = 1. $$

Theorem on the replacement of b.m. functions equivalent

Let %%\alpha(x), \alpha_1(x), \beta(x), \beta_1(x)%% be b.m. functions at %%x \to a%%, and %%\alpha(x) \sim \alpha_1(x); \beta(x) \sim \beta_1(x)%%, then $$ \lim\limits_(x \to a)(\frac(\alpha(x))(\beta(x))) = \lim\ limits_(x \to a)(\frac(\alpha_1(x))(\beta_1(x))). $$

Equivalent b.m. functions.

Let %%\alpha(x)%% be b.m. function at %%x \to a%%, then

1. %%\sin(\alpha(x)) \sim \alpha(x)%%
2. %%\displaystyle 1 - \cos(\alpha(x)) \sim \frac(\alpha^2(x))(2)%%
3. %%\tan \alpha(x) \sim \alpha(x)%%
4. %%\arcsin\alpha(x) \sim \alpha(x)%%
5. %%\arctan\alpha(x) \sim \alpha(x)%%
6. %%\ln(1 + \alpha(x)) \sim \alpha(x)%%
7. %%\displaystyle\sqrt[n](1 + \alpha(x)) - 1 \sim \frac(\alpha(x))(n)%%
8. %%\displaystyle a^(\alpha(x)) - 1 \sim \alpha(x) \ln(a)%%

Example

$$ \begin(array)(ll) \lim\limits_(x \to 0)( \frac(\ln\cos x)(\sqrt(1 + x^2) - 1)) & = \lim\limits_ (x \to 0)(\frac(\ln(1 + (\cos x - 1)))(\frac(x^2)(4))) = \\ & = \lim\limits_(x \to 0)(\frac(4(\cos x - 1))(x^2)) = \\ & = \lim\limits_(x \to 0)(-\frac(4 x^2)(2 x^ 2)) = -2 \end(array) $$

Infinitely large functions

The function %%f(x)%% is called infinitely large(b.b.) for %%x \to a \in \overline(\mathbb(R))%%, if the function has an infinite limit as the argument tends to do so.

Like b.m. functions the concept of b.b. function is inextricably linked with an indication of a change in its argument. We can talk about b.b. functions at %%x \to a + 0%% and %%x \to a - 0%%. The term “infinitely large” does not mean the absolute value of the function, but the nature of its change in the vicinity of the considered point. No constant number, however large in absolute value, is infinitely large.

Examples

1. Function %%f(x) = 1/x%% - b.b. at %%x \to 0%%.
2. Function %%f(x) = x%% - b.b. at %%x \to \infty%%.

If the conditions of the definitions $$ \begin(array)(l) \lim\limits_(x \to a)(f(x)) = +\infty, \\ \lim\limits_(x \to a)(f( x)) = -\infty, \end(array) $$

then they talk about positive or negative b.b. at %%a%% function.

Example

The function %%1/(x^2)%% is a positive b.b. at %%x \to 0%%.

The connection between b.b. and b.m. functions

If %%f(x)%% is b.b. if %%x \to a%% is a function, then %%1/f(x)%% is b.m.

with %%x \to a%%. If %%\alpha(x)%% is b.m. for %%x \to a%% is a non-zero function in some punctured neighborhood of the point %%a%%, then %%1/\alpha(x)%% is b.b. with %%x \to a%%.

Properties of infinitely large functions

Let us present several properties of b.b. functions. These properties follow directly from the definition of b.b. functions and properties of functions that have finite limits, as well as from the connection theorem between b.b. and b.m. functions.

1. The product of a finite number b.b. functions for %%x \to a%% are b.b. function at %%x \to a%%. Indeed, if %%f_k(x), k = \overline(1, n)%% is b.b. functions at %%x \to a%%, then in some punctured neighborhood of the point %%a%% %%f_k(x) \ne 0%%, and by the connection theorem b.b. and b.m. functions %%1/f_k(x)%% - b.m. function at %%x \to a%%. It turns out %%\displaystyle\prod^(n)_(k = 1) 1/f_k(x)%% is a b.m function for %%x \to a%%, and %%\displaystyle\prod^(n )_(k = 1)f_k(x)%% — b.b. function at %%x \to a%%.
2. The product of b.b. functions at %%x \to a%% and a function whose absolute value is greater than a positive constant in some punctured neighborhood of the point %%a%% is a b.b. function at %%x \to a%%. In particular, the product of b.b. functions at %%x \to a%% and a function that has a finite non-zero limit at the point %%a%% will be b.b. function at %%x \to a%%.

The sum of a function bounded in some punctured neighborhood of the point %%a%% and b.b. functions at %%x \to a%% are b.b. function at %%x \to a%%.

For example, the functions %%x - \sin x%% and %%x + \cos x%% are b.b. at %%x \to \infty%%.

3. The sum of two b.b. functions at %%x \to a%% there is uncertainty. Depending on the sign of the terms, the nature of the change in such a sum can be very different.

   Example

   Let the functions %%f(x)= x, g(x) = 2x, h(x) = -x, v(x) = x + \sin x%% - b.b. functions at %%x \to \infty%%. Then:

   • %%f(x) + g(x) = 3x%% - b.b. function at %%x \to \infty%%;
   • %%f(x) + h(x) = 0%% - b.m. function at %%x \to \infty%%;
   • %%h(x) + v(x) = \sin x%% has no limit at %%x \to \infty%%.

From Wikipedia, the free encyclopedia

Infinitesimal- a numerical function or sequence that tends to zero.

infinitely large- a numerical function or sequence that tends to infinity a certain sign.

Calculus of infinitesimals and large

Infinitesimal calculus- calculations performed with infinitesimal values, in which the derived result is considered as infinite sum infinitely small. Infinitesimal calculus is a general concept for differential And integral calculus, which form the basis of modern higher mathematics. The concept of an infinitesimal quantity is closely related to the concept limit.

Infinitesimal

Subsequence $a\_n$ called infinitesimal, if $\backslash lim\backslash limits\_(n\backslash to\backslash infty)a\_n=0$. For example, the sequence of numbers $a\_n=\backslash dfrac(1)(n)$- infinitely small.

The function is called infinitesimal in a neighborhood of a point $x\_0$, if $\backslash lim\backslash limits\_(x\backslash to\; x\_0)f(x)=0$.

The function is called infinitesimal at infinity, if $\backslash lim\backslash limits\_(x\backslash to+\backslash infty)f(x)=0$ or $\backslash lim\backslash limits\_(x\backslash to-\backslash infty)f(x)=0$.

Also infinitely small is a function that is the difference between a function and its limit, that is, if $\backslash lim\backslash limits\_(x\backslash to+\backslash infty)f(x)=a$, then $f(x)-a=\backslash alpha(x)$, $\backslash lim\backslash limits\_(x\backslash to+\backslash infty)(f(x)-a)=0$.

We emphasize that an infinitesimal quantity should be understood as variable(function), which is only in the process of changing[when striving $x$ to $a$(from $\backslash lim\backslash limits\_(x\backslash to\; a)f(x)=0$)] is made less than an arbitrary number ( $\backslash varepsilon$). Therefore, for example, a statement like "one millionth is an infinitesimal quantity" is incorrect: o including[absolute value] it does not make sense to say that it is infinitesimal.

infinitely large

In all the formulas below, infinity to the right of equality implies a certain sign (either "plus" or "minus"). That is, for example, the function $x\backslash sin\; x$, unbounded on both sides, is not infinitely large for $x\backslash to+\backslash infty$.

Subsequence $a\_n$ called infinitely large, if $\backslash lim\backslash limits\_(n\backslash to\backslash infty)a\_n=\backslash infty$.

The function is called infinitely large in a neighborhood of a point $x\_0$, if $\backslash lim\backslash limits\_(x\backslash to\; x\_0)f(x)=\backslash infty$.

The function is called infinitely large at infinity, if $\backslash lim\backslash limits\_(x\backslash to+\backslash infty)f(x)=\backslash infty$ or $\backslash lim\backslash limits\_(x\backslash to-\backslash infty)f(x)=\backslash infty$.

As in the case of infinitesimals, it should be noted that no single value of an infinitely large quantity can be called "infinitely large" - an infinitely large quantity is function, which is only in the process of changing can be greater than an arbitrary number.

Properties of infinitesimals

• The algebraic sum of a finite number of infinitely small functions is an infinitely small function.
• The product of infinitesimals is infinitesimal.
• The product of an infinitesimal sequence by a bounded one is infinitesimal. As a consequence, the product of an infinitesimal by a constant is an infinitesimal.
• If $a\_n$ is an infinitesimal sign-preserving sequence, then $b\_n=\backslash dfrac(1)(a\_n)$ is an infinitely large sequence.

Comparison of infinitesimals

Definitions

Suppose we have infinitesimals for the same $x\backslash to\; a$ quantities $\backslash alpha(x)$ And $\backslash beta(x)$(or, which is not important for the definition, infinitesimal sequences).

• If $\backslash lim\backslash limits\_(x\backslash to\; a)\backslash dfrac(\backslash beta)(\backslash alpha)=0$, then $\backslash beta$- infinitely small higher order of smallness, how $\backslash alpha$. designate $\backslash beta=o(\backslash alpha)$ or $\backslash beta\backslash prec\backslash alpha$.
• If $\backslash lim\backslash limits\_(x\backslash to\; a)\backslash dfrac(\backslash beta)(\backslash alpha)=\backslash infty$, then $\backslash beta$- infinitely small the lowest order of smallness, how $\backslash alpha$. Respectively $\backslash alpha=o(\backslash beta)$ or $\backslash alpha\backslash prec\backslash beta$.
• If $\backslash lim\backslash limits\_(x\backslash to\; a)\backslash dfrac(\backslash beta)(\backslash alpha)=c$(the limit is finite and not equal to 0), then $\backslash alpha$ And $\backslash beta$ are infinitesimal quantities one order of magnitude. This is denoted as $\backslash alpha\backslash asymp\backslash beta$ or as simultaneous execution of relations $\backslash beta=O(\backslash alpha)$ And $\backslash alpha=O(\backslash beta)$. It should be noted that in some sources one can come across a designation when the sameness of orders is written in the form of only one “big o” ratio, which is a free use of this symbol.
• If $\backslash lim\backslash limits\_(x\backslash to\; a)\backslash dfrac(\backslash beta)(\backslash alpha^m)=c$(the limit is finite and not equal to 0), then the infinitesimal quantity $\backslash beta$ It has $m$-th order of smallness relatively infinitesimal $\backslash alpha$.

To calculate such limits, it is convenient to use L'Hopital's rule.

Comparison examples

• At $(x\backslash to\; 0)$ magnitude $x^5$ has the highest order of smallness with respect to $x^3$, because $\backslash lim\backslash limits\_(x\backslash to\; 0)\backslash dfrac(x^5)(x^3)=0$. On the other hand, $x^3$ has the lowest order of smallness with respect to $x^5$, because $\backslash lim\backslash limits\_(x\backslash to\; 0)\backslash dfrac(x^3)(x^5)=\backslash infty$.

Using ABOUT-symbols the results obtained can be written in the following form $x^5=o(x^3)$.

• $\backslash lim\backslash limits\_(x\backslash to\; 0)\backslash dfrac(2x^2+6x)(x)=\backslash lim\backslash limits\_(x\backslash to\; 0)\backslash dfrac(2x+6)(1)=\backslash lim\backslash limits\_(x\; \backslash to\; 0)(2x+6)=6,$ that is, when $x\backslash to\; 0$ functions $f(x)=2x^2+6x$ And $g(x)=x$ are infinitesimal quantities of the same order.

In this case, the entries $2x^2+6x\; =\; O(x)$ And $x\; =\; O(2x^2+6x).$

• At $(x\backslash to\; 0)$ infinitesimal $2x^3$ has the third order of smallness with respect to $x$, insofar as $\backslash lim\backslash limits\_(x\backslash to\; 0)\backslash dfrac(2x^3)(x^3)=2$, infinitesimal $0(,)7x^2$- second order, infinitesimal $\backslash sqrt(x)$- order 0.5.

Equivalent quantities

Definition

If $\backslash lim\backslash limits\_(x\backslash to\; a)\backslash dfrac(\backslash beta)(\backslash alpha)=1$, then infinitesimal or infinitely large quantities $\backslash alpha$ And $\backslash beta$ called equivalent(denoted as $\backslash alpha\backslash thicksim\backslash beta$).

Obviously, equivalent quantities are a special case of infinitely small (infinitely large) quantities of the same order of smallness.

At $$ the following equivalence relations hold true (as consequences of the so-called wonderful limits):

• $\backslash sin\backslash alpha(x)\backslash thicksim\backslash alpha(x);$
• $\backslash mathrm(tg)\backslash ,\backslash alpha(x)\backslash thicksim\backslash alpha(x);$
• $\backslash arcsin(\backslash alpha(x))\backslash thicksim\backslash alpha(x);$
• $\backslash mathrm(arctg)\backslash ,\backslash alpha(x)\backslash thicksim\backslash alpha(x);$
• $\backslash log\_a(1+\backslash alpha(x))\backslash thicksim\backslash alpha(x)\backslash cdot\backslash frac(1)(\backslash ln(a))$, where $a>0$;
• $\backslash ln(1+\backslash alpha(x))\backslash thicksim\backslash alpha(x);$
• $a^(\backslash alpha(x))-1\backslash thicksim\backslash alpha(x)\backslash cdot\backslash ln(a)$, where $a>0$;
• $e^(\backslash alpha(x))-1\backslash thicksim\backslash alpha(x);$
• $1-\backslash cos(\backslash alpha(x))\backslash thicksim\backslash frac(\backslash alpha^2(x))(2);$
• $(1+\backslash alpha(x))^\backslash mu-1\backslash thicksim\backslash mu\backslash cdot\backslash alpha(x),\backslash quad\backslash mu\backslash in\backslash R$, so use the expression:

$\backslash sqrt[n](1+\backslash alpha(x))\backslash approx\backslash frac(\backslash alpha(x))(n)+1$, where $\backslash alpha(x)\backslash xrightarrow()0$.

Theorem

The limit of the quotient (ratio) of two infinitesimal or infinitely large quantities will not change if one of them (or both) is replaced by an equivalent value.

This theorem is of practical importance in finding limits (see example).

Examples of using

• To find $\backslash lim\backslash limits\_(x\backslash to\; 0)\backslash dfrac(\backslash sin\; 2x)(x).$

Replacing $\backslash sin\; 2x$ equivalent value $2x$, we get $\backslash lim\backslash limits\_(x\backslash to\; 0)\backslash dfrac(\backslash sin\; 2x)(x)=\backslash lim\backslash limits\_(x\backslash to\; 0)\backslash dfrac(2x)(x)=2.$

• To find $\backslash lim\backslash limits\_(x\backslash to\backslash frac(\backslash pi)(2))\backslash dfrac(\backslash sin(4\backslash cos\; x))(\backslash cos\; x).$

Because $\backslash sin(4\backslash cos\; x)\backslash thicksim(4\backslash cos\; x)$ at $x\backslash to\backslash dfrac(\backslash pi)(2)$ we get $\backslash lim\backslash limits\_(x\backslash to\; \backslash frac(\backslash pi)(2))\backslash dfrac(\backslash sin(4\backslash cos\; x))(\backslash cos\; x)=\backslash lim\backslash limits\_(x\backslash to\backslash frac(\backslash pi)\; (2))\backslash dfrac(4\backslash cos\; x)(\backslash cos\; x)=4.$

• Calculate $\backslash sqrt(1(,)2)$.

Using the formula : $\backslash sqrt(1(,)2)\backslash approx\; 1+\backslash frac(0(,)2)(2)=1(,)1$, while using calculator(more accurate calculations), we got: $\backslash sqrt(1(,)2)\backslash approx\; 1(,)095$, thus the error was 0.005 (less than 1%), that is, the method is useful, due to its simplicity, with a rough estimate arithmetic roots close to unity.

History

Old school mathematicians subjected the concept infinitesimal harsh criticism. Michelle Roll wrote that the new calculus is " set of brilliant mistakes»; Voltaire pointed out venomously that this calculus is the art of calculating and accurately measuring things whose existence cannot be proven. Even Huygens admitted that he did not understand the meaning higher order differentials.

As an irony of fate, one can consider the appearance in the middle XX century non-standard analysis, who proved that the original point of view - the actual infinitesimals - is also consistent and could be the basis of the analysis. With the advent of non-standard analysis, it became clear why mathematicians of the 18th century, performing actions that were illegal from the point of view of the classical theory, nevertheless received correct results.

see also

Write a review on the article "Infinitesimal and infinitely large"

Notes

Literature

• // Encyclopedic Dictionary of Brockhaus and Efron: in 86 tons (82 tons and 4 additional). - St. Petersburg. , 1890-1907.

An excerpt characterizing the Infinitesimal and the infinitely large

“Well, my friend, I’m afraid that you and the monk are wasting your gunpowder,” Prince Andrei said mockingly, but affectionately.
- Ah! mon ami. [BUT! My friend.] I just pray to God and hope that He hears me. Andre,” she said timidly after a moment of silence, “I have a big request for you.
- What, my friend?
No, promise me you won't refuse. It will not cost you any work, and there will be nothing unworthy of you in it. Only you can comfort me. Promise, Andryusha, - she said, putting her hand into the purse and holding something in it, but not yet showing, as if what she was holding was the subject of the request and as if before receiving the promise in fulfillment of the request she could not remove it from the purse It is something.
She looked timidly, imploringly at her brother.
“If it would cost me a lot of work ...” Prince Andrei answered, as if guessing what was the matter.
- Whatever you want, think! I know you are the same as mon pere. Think whatever you want, but do it for me. Do it please! My father's father, our grandfather, wore it in all wars ... - She still did not get what she was holding from her purse. "So you promise me?"
"Of course, what's the matter?"
- Andre, I will bless you with the image, and you promise me that you will never take it off. Promise?
“If he doesn’t drag his neck down to two pounds ... To please you ...” said Prince Andrei, but at the same moment, noticing the distressed expression that his sister’s face assumed at this joke, he repented. “Very glad, really very glad, my friend,” he added.
“Against your will, He will save and have mercy on you and turn you to Himself, because in Him alone is truth and peace,” she said in a voice trembling with excitement, with a solemn gesture holding in both hands in front of her brother an oval ancient icon of the Savior with a black face in silver chasuble on a silver chain of fine workmanship.
She crossed herself, kissed the icon and handed it to Andrey.
– Please, Andre, for me…
Beams of kind and timid light shone from her large eyes. These eyes illuminated the whole sickly, thin face and made it beautiful. The brother wanted to take the scapular, but she stopped him. Andrei understood, crossed himself and kissed the icon. His face was at the same time gentle (he was touched) and mocking.
- Merci, mon ami. [Thank you my friend.]
She kissed him on the forehead and sat back down on the sofa. They were silent.
- So I told you, Andre, be kind and generous, as you have always been. Don't judge Lise harshly, she began. - She is so sweet, so kind, and her position is very difficult now.
- It seems that I didn’t tell you anything, Masha, so that I reproach my wife for anything or be dissatisfied with her. Why are you telling me all this?
Princess Mary blushed in spots and became silent, as if she felt guilty.
“I didn’t say anything to you, but you were already told. And it makes me sad.
Red spots appeared even more strongly on the forehead, neck and cheeks of Princess Marya. She wanted to say something and could not utter it. The brother guessed right: the little princess cried after dinner, said that she foresaw an unfortunate birth, was afraid of them, and complained about her fate, her father-in-law and her husband. After crying, she fell asleep. Prince Andrei felt sorry for his sister.
- Know one thing, Masha, I cannot reproach, have not reproached and will never reproach my wife, and I myself cannot reproach myself with anything in relation to her; and it will always be so, in whatever circumstances I may be. But if you want to know the truth... you want to know if I'm happy? No. Is she happy? No. Why is this? Do not know…
Saying this, he stood up, went over to his sister, and, bending down, kissed her on the forehead. His beautiful eyes shone with an intelligent and kind, unaccustomed brilliance, but he looked not at his sister, but into the darkness of the open door, through her head.
- Let's go to her, we must say goodbye. Or go alone, wake her up, and I'll come right now. Parsley! he shouted to the valet, “come here, clean it up.” It's in the seat, it's on the right side.
Princess Marya got up and went to the door. She stopped.
Andre, si vous avez. la foi, vous vous seriez adresse a Dieu, pour qu "il vous donne l" amour, que vous ne sentez pas et votre priere aurait ete exaucee. [If you had faith, you would turn to God with a prayer, so that He would give you love that you do not feel, and your prayer would be heard.]
- Yes, is it! - said Prince Andrew. - Go, Masha, I'll come right away.
On the way to his sister's room, in the gallery that connected one house with another, Prince Andrei met a sweetly smiling m lle Bourienne, for the third time that day with an enthusiastic and naive smile he came across in secluded passages.
- Ah! je vous croyais chez vous, [Ah, I thought you were in your room,] she said, blushing for some reason and lowering her eyes.
Prince Andrei looked sternly at her. Anger suddenly appeared on the face of Prince Andrei. He said nothing to her, but looked at her forehead and hair, without looking into her eyes, so contemptuously that the Frenchwoman blushed and left without saying anything.
When he approached his sister's room, the princess was already awake, and her cheerful voice, hurrying one word after another, was heard from the open door. She spoke as if, after a long period of abstinence, she wanted to make up for lost time.
- Non, mais figurez vous, la vieille comtesse Zouboff avec de fausses boucles et la bouche pleine de fausses dents, comme si elle voulait defier les annees ... [No, imagine, old Countess Zubova, with fake curls, with fake teeth, like as if mocking the years…] Xa, xa, xa, Marieie!
Exactly the same phrase about Countess Zubova and the same laugh had already been heard five times in front of strangers by Prince Andrei from his wife.
He quietly entered the room. The princess, plump, ruddy, with work in her hands, sat on an armchair and talked incessantly, sorting through Petersburg memories and even phrases. Prince Andrei came up, stroked her head and asked if she had rested from the journey. She answered and continued the same conversation.
The stroller stood in six at the entrance. It was a dark autumn night outside. The coachman did not see the drawbar of the carriage. People with lanterns bustled about on the porch. The huge house burned with lights through its large windows. In the hall crowded the courtyards, who wanted to say goodbye to the young prince; all the household were standing in the hall: Mikhail Ivanovich, m lle Bourienne, Princess Mary and the princess.
Prince Andrei was called to his father's office, who wanted to say goodbye to him face to face. Everyone was waiting for them to come out.
When Prince Andrei entered the office, the old prince, wearing old man's glasses and in his white coat, in which he received no one except his son, was sitting at the table and writing. He looked back.
– Are you going? And he began to write again.
- I came to say goodbye.
- Kiss here, - he showed his cheek, - thank you, thank you!
- What do you thank me for?
- Because you don’t overstay, you don’t hold on to a woman’s skirt. Service first. Thank you, thank you! And he continued to write, so that the spray flew from the crackling pen. - If you need to say something, say it. These two things I can do together,” he added.
“About my wife… I’m so ashamed that I’m leaving her in your arms…”
- What are you lying? Say what you need.
- When your wife has time to give birth, send to Moscow for an obstetrician ... So that he is here.
The old prince stopped and, as if not understanding, stared with stern eyes at his son.
“I know that no one can help if nature does not help,” said Prince Andrei, apparently embarrassed. “I agree that out of a million cases, one is unfortunate, but this is her fantasy and mine. They told her, she saw it in a dream, and she is afraid.
“Hm ... hm ...” the old prince said to himself, continuing to finish writing. - I will.
He crossed out the signature, suddenly turned quickly to his son and laughed.
- It's bad, isn't it?
- What's wrong, father?
- Wife! said the old prince shortly and significantly.
“I don’t understand,” said Prince Andrei.
“Yes, there’s nothing to do, my friend,” the prince said, “they are all like that, you won’t get married.” Do not be afraid; I won't tell anyone; and you yourself know.
He grabbed his hand with his bony little hand, shook it, looked straight into his son's face with his quick eyes, which seemed to see right through the man, and again laughed his cold laugh.
The son sighed, confessing with this sigh that his father understood him. The old man, continuing to fold and print letters, with his usual speed, grabbed and threw sealing wax, seal and paper.
- What to do? Beautiful! I'll do everything. You be calm,” he said curtly while typing.
Andrey was silent: it was both pleasant and unpleasant for him that his father understood him. The old man got up and handed the letter to his son.
“Listen,” he said, “do not worry about your wife: what can be done will be done.” Now listen: give the letter to Mikhail Ilarionovich. I am writing that he will use you in good places and not keep you as an adjutant for a long time: a bad post! Tell him that I remember him and love him. Yes, write how he will accept you. If it's good, serve. Nikolai Andreich Bolkonsky's son, out of mercy, will not serve anyone. Well, now come here.
He spoke in such a rapid way that he did not finish half of the words, but the son was used to understanding him. He led his son to the bureau, threw back the lid, pulled out a drawer, and took out a notebook covered in his large, long, concise handwriting.
“I must die before you.” Know that here are my notes, to transfer them to the sovereign after my death. Now here - here is a pawn ticket and a letter: this is a prize to the one who writes the history of the Suvorov wars. Submit to the academy. Here are my remarks, after me read for yourself, you will find something useful.
Andrei did not tell his father that he would probably live for a long time. He knew he didn't need to say it.
“I will do everything, father,” he said.
- Well, now goodbye! He let his son kiss his hand and hugged him. “Remember one thing, Prince Andrei: if they kill you, the old man will hurt me ...” He suddenly fell silent and suddenly continued in a loud voice: “and if I find out that you did not behave like the son of Nikolai Bolkonsky, I will be ... ashamed! he screeched.
“You could not tell me that, father,” said the son, smiling.
The old man was silent.
“I also wanted to ask you,” continued Prince Andrei, “if they kill me and if I have a son, do not let him go away from you, as I told you yesterday, so that he grows up with you ... please.
- Don't give it to your wife? the old man said and laughed.
They stood silently facing each other. The old man's quick eyes were fixed directly on his son's eyes. Something quivered in the lower part of the old prince's face.
- Goodbye ... go! he suddenly said. - Get up! he shouted in an angry and loud voice, opening the office door.
– What is, what? - asked the princess and princess, seeing Prince Andrei and for a moment the figure of an old man in a white coat, without a wig and in old man's glasses, leaning out screaming in an angry voice.
Prince Andrei sighed and did not answer.
“Well,” he said, turning to his wife.
And this “well” sounded like a cold mockery, as if he was saying: “now you do your tricks.”
Andre, deja! [Andrey, already!] - said the little princess, turning pale and looking at her husband with fear.
He hugged her. She screamed and fell unconscious on his shoulder.
He gently drew back the shoulder on which she was lying, looked into her face, and carefully seated her in a chair.
- Adieu, Marieie, [Farewell, Masha,] - he said quietly to his sister, kissed her hand in hand and quickly left the room.
The princess was lying in an armchair, m lle Bourienne was rubbing her temples. Princess Mary, supporting her daughter-in-law, with tearful beautiful eyes, was still looking at the door through which Prince Andrei went out, and baptized him. From the study were heard, like shots, the often repeated angry sounds of the old man blowing his nose. As soon as Prince Andrei left, the door of the office quickly opened and a stern figure of an old man in a white coat looked out.
- Left? Well, good! he said, looking angrily at the insensible little princess, shook his head reproachfully and slammed the door.

In October 1805, Russian troops occupied the villages and cities of the Archduchy of Austria, and more new regiments came from Russia and, weighing down the inhabitants with billeting, were located near the Braunau fortress. In Braunau was the main apartment of the commander-in-chief Kutuzov.
On October 11, 1805, one of the infantry regiments that had just arrived at Braunau, waiting for the review of the commander-in-chief, stood half a mile from the city. Despite the non-Russian terrain and situation (orchards, stone fences, tiled roofs, mountains visible in the distance), the non-Russian people, who looked at the soldiers with curiosity, the regiment had exactly the same appearance as any Russian regiment preparing for a show somewhere in the middle of Russia.

Definitions and properties of infinitely small and infinitely large functions at a point. Proofs of properties and theorems. Relationship between infinitesimal and infinitely large functions.

Content

See also: Infinitely small sequences - definition and properties
Properties of infinitely large sequences

Definition of infinitesimal and infinitely large functions

Let x 0 is a finite or at infinity point: ∞ , -∞ or +∞ .

Definition of an infinitesimal function
Function α (x) called infinitesimal as x tends to x 0 0 , and it is equal to zero:
.

Definition of an infinite function
function f (x) called infinitely large as x tends to x 0 , if the function has a limit as x → x 0 , and it is equal to infinity:
.

Properties of infinitesimal functions

Property of sum, difference and product of infinitesimal functions

Sum, difference and product a finite number of infinitely small functions as x → x 0 is an infinitesimal function as x → x 0 .

This property is a direct consequence of the arithmetic properties of the limits of a function.

Theorem on the product of a bounded function by an infinitesimal

The product of a function bounded on some punctured neighborhood of the point x 0 , to an infinitesimal, as x → x 0 , is an infinitesimal function as x → x 0 .

Property on representing a function as a sum of a constant and an infinitesimal function

In order for the function f (x) has a finite limit , it is necessary and sufficient that
,
where is an infinitesimal function as x → x 0 .

Properties of infinitely large functions

Theorem on the sum of a bounded function and an infinitely large one

The sum or difference of a bounded function, on some punctured neighborhood of the point x 0 , and an infinitely large function, as x → x 0 , is an infinite function as x → x 0 .

The quotient theorem for a bounded function by an infinitely large one

If the function f (x) is infinite as x → x 0 , and the function g (x)- bounded on some punctured neighborhood of the point x 0 , then
.

Theorem on the quotient of division of a function bounded below by an infinitesimal one

If the function , on some punctured neighborhood of the point , is bounded from below by a positive number in absolute value:
,
and the function is infinitesimal as x → x 0 :
,
and there is a punctured neighborhood of the point on which , then
.

Property of inequalities of infinitely large functions

If the function is infinitely large for :
,
and functions and , on some punctured neighborhood of the point satisfy the inequality:
,
then the function is also infinitely large for :
.

This property has two special cases.

Let, on some punctured neighborhood of the point , the functions and satisfy the inequality:
.
Then if , then and .
If , then and .

Relationship between infinitely large and infinitely small functions

The connection between infinitely large and infinitely small functions follows from the two previous properties.

If a function is infinitely large at , then the function is infinitely small at .

If the function is infinitely small for , and , then the function is infinitely large for .

The relationship between an infinitesimal and an infinitely large function can be expressed symbolically:
, .

If an infinitesimal function has a definite sign at , that is, it is positive (or negative) on some punctured neighborhood of the point , then it can be written as follows:
.
Similarly, if an infinitely large function has a certain sign at , then they write:
, or .

Then the symbolic connection between infinitely small and infinitely large functions can be supplemented by the following relations:
, ,
, .

Additional formulas relating infinity symbols can be found on the page
"Points at infinity and their properties".

Proof of properties and theorems

Proof of the theorem on the product of a bounded function by an infinitesimal

To prove this theorem, we will use . We also use the property of infinitesimal sequences, according to which

Let the function be infinitesimal at , and the function be bounded in some punctured neighborhood of the point :
at .

Since there is a limit, there is a punctured neighborhood of the point on which the function is defined. Let there be an intersection of neighborhoods and . Then the functions and are defined on it.

.
,
a sequence is infinitesimal:
.

We use the fact that the product of a bounded sequence by an infinitesimal one is an infinitesimal sequence:
.
.

The theorem has been proven.

Proof of a property on the representation of a function as a sum of a constant and an infinitesimal function

Need. Let the function have a finite limit at a point
.
Consider a function:
.
Using the property of the limit of the difference of functions , we have:
.
That is, there is an infinitesimal function for .

Adequacy. Let and . Let's apply the limit property of the sum of functions:
.

The property has been proven.

Proof of the theorem on the sum of a bounded function and an infinitely large one

To prove the theorem, we will use the Heine definition of the limit of a function

at .

Since there is a limit , then there is a punctured neighborhood of the point on which the function is defined. Let there be an intersection of neighborhoods and . Then the functions and are defined on it.

Let there be an arbitrary sequence converging to , whose elements belong to the neighborhood :
.
Then sequences and are defined. And the sequence is limited:
,
a sequence is infinite:
.

Since the sum or difference of a bounded sequence and an infinitely large
.
Then, according to the Heine definition of the limit of a sequence,
.

The theorem has been proven.

Proof of the quotient theorem for a bounded function by an infinitely large one

For the proof, we will use Heine's definition of the limit of a function. We also use the property of infinitely large sequences, according to which is an infinitely small sequence.

Let the function be infinitely large at , and the function be bounded in some punctured neighborhood of the point :
at .

Since the function is infinitely large, there is a punctured neighborhood of the point on which it is defined and does not vanish:
at .
Let there be an intersection of neighborhoods and . Then the functions and are defined on it.

Let there be an arbitrary sequence converging to , whose elements belong to the neighborhood :
.
Then sequences and are defined. And the sequence is limited:
,
a sequence is infinite with non-zero terms:
, .

Since the quotient of dividing a bounded sequence by an infinitely large one is an infinitesimal sequence, then
.
Then, according to the Heine definition of the limit of a sequence,
.

The theorem has been proven.

Proof of the theorem on the quotient of division of a function bounded below by an infinitesimal one

To prove this property, we will use Heine's definition of the limit of a function. We also use the property of infinitely large sequences, according to which is an infinitely large sequence.

Let the function be infinitesimal at , and the function be bounded in absolute value from below by a positive number, on some punctured neighborhood of the point :
at .

By assumption, there is a punctured neighborhood of the point on which the function is defined and does not vanish:
at .
Let there be an intersection of neighborhoods and . Then the functions and are defined on it. And and.

Let there be an arbitrary sequence converging to , whose elements belong to the neighborhood :
.
Then sequences and are defined. Moreover, the sequence is bounded from below:
,
and the sequence is infinitesimal with non-zero terms:
, .

Since the quotient of dividing a sequence bounded below by an infinitesimal one is an infinitely large sequence, then
.
And let there be a punctured neighborhood of the point on which
at .

Take an arbitrary sequence converging to . Then, starting from some number N , the elements of the sequence will belong to this neighborhood:
at .
Then
at .

According to Heine's definition of the limit of a function,
.
Then, by the property of inequalities of infinitely large sequences,
.
Since the sequence is arbitrary, converging to , then, by the definition of the limit of a function according to Heine,
.

The property has been proven.

References:
L.D. Kudryavtsev. Course of mathematical analysis. Volume 1. Moscow, 2003.

See also: