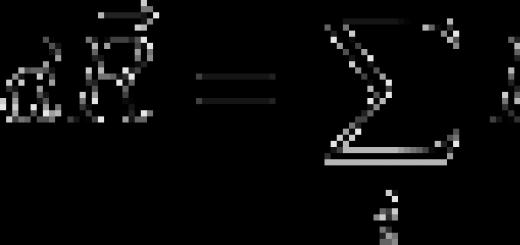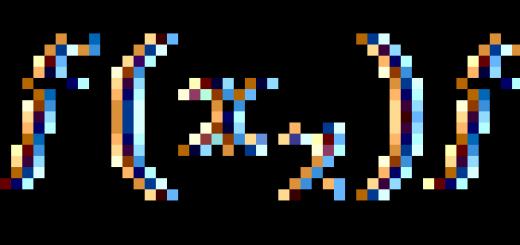Theorem on the change in the motion of a material point. Theorems on the change in the momentum of a point and a system. Theorem on the change of the kinetic moment

Similarly, as for one material point, we derive a theorem on the change in the momentum for the system in various forms.

We transform the equation (theorem on the movement of the center of mass of a mechanical system)

in the following way:

;

The resulting equation expresses the theorem on the change in the momentum of a mechanical system in differential form: the time derivative of the momentum of a mechanical system is equal to the main vector of external forces acting on the system .

In projections onto Cartesian coordinate axes:

; ; .

Taking the integrals of both parts of the last equations in time, we obtain a theorem on the change in the momentum of a mechanical system in integral form: the change in the momentum of a mechanical system is equal to the momentum of the main vector of external forces acting on the system .

Or in projections onto the Cartesian coordinate axes:

; ; .

Consequences from the theorem (laws of conservation of momentum)

The law of conservation of momentum is obtained as special cases of the theorem on the change in momentum for a system depending on the features of the system of external forces. Internal forces can be anything, as they do not affect changes in momentum.

Two cases are possible:

1. If the vector sum of all external forces applied to the system is equal to zero, then the momentum of the system is constant in magnitude and direction

2. If the projection of the main vector of external forces on any coordinate axis and/or and/or is equal to zero, then the projection of the amount of motion on the same axes is a constant value, i.e. and/or and/or respectively.

Similar records can be made for a material point and for a material point.

The task. From a gun whose mass M, a projectile of mass flies out in a horizontal direction m with speed v. Find speed V guns after firing.

Solution. All external forces acting on the gun-projectile mechanical system are vertical. Hence, based on the corollary of the theorem on the change in the momentum of the system, we have: .

The amount of movement of the mechanical system before the shot:

The amount of movement of the mechanical system after the shot:

Equating the right parts of the expressions, we get that

The “-” sign in the resulting formula indicates that after the shot, the gun will roll back in the direction opposite to the axis Ox.

EXAMPLE 2. A jet of liquid with a density flows out at a speed V from a pipe with a cross-sectional area F and hits a vertical wall at an angle. Determine the fluid pressure on the wall.

SOLUTION. We apply the theorem on the change in momentum in integral form to the volume of liquid with mass m hitting a wall over a period of time t.

MESHCHERSKY EQUATION

(basic equation of the dynamics of a body of variable mass)

In modern technology, cases arise when the mass of a point and a system does not remain constant in the process of movement, but changes. So, for example, during the flight of space rockets, due to the ejection of combustion products and individual unnecessary parts of rockets, the change in mass reaches 90-95% of the total initial value. But not only space technology can be an example of the dynamics of the movement of a variable mass. In the textile industry, there is a significant change in the mass of various spindles, spools, rolls at modern machine and machine speeds.

Consider the main features associated with a change in mass, using the example of the translational motion of a body of variable mass. The basic law of dynamics cannot be directly applied to a body of variable mass. Therefore, we obtain differential equations of motion of a point of variable mass, applying the theorem on the change in the momentum of the system.

Let a point of mass m+dm moves at speed. Then there is a detachment from the point of some particle with a mass dm moving at speed.

The amount of motion of the body before the detachment of the particle:

The amount of motion of a system consisting of a body and a detached particle after its detachment:

Then the change in momentum is:

Based on the theorem on the change in the momentum of the system:

Let us denote the value - the relative velocity of the particle:

Denote

the value R called reactive force. The jet force is the thrust of the engine, due to the release of gas from the nozzle.

Finally we get

This formula expresses the basic equation of the dynamics of a body of variable mass (Meshchersky's formula). It follows from the last formula that the differential equations of motion of a point of variable mass have the same form as for a point of constant mass, except for the additional reactive force applied to the point due to the change in mass.

The basic equation of the dynamics of a body of variable mass indicates that the acceleration of this body is formed not only due to external forces, but also due to the reactive force.

Reactive force is a force akin to that felt by a shooting person - when firing a pistol, it is felt by the hand; when shooting from a rifle, it is perceived by the shoulder.

Tsiolkovsky's first formula (for a single-stage rocket)

Let a point of variable mass or a rocket move in a straight line under the action of only one reactive force. Since for many modern jet engines , where is the maximum reactive force allowed by the engine design (engine thrust); - the force of gravity acting on the engine, located on the earth's surface. Those. the foregoing allows the component in the Meshchersky equation to be neglected and for further analysis to accept this equation in the form: ,

Denote:

Fuel reserve (for liquid-propellant jet engines - the dry mass of the rocket (its remaining mass after all the fuel burns out);

The mass of particles separated from the rocket; considered as a variable varying from to .

Let us write the equation of rectilinear motion of a point of variable mass in the following form:

Since the formula for determining the variable mass of a rocket

Therefore, the equations of motion of a point Taking the integrals of both parts, we get

where - characteristic speed- this is the speed that the rocket acquires under the action of thrust after the eruption of all particles from the rocket (with liquid-propellant jet engines - after burning out all the fuel).

Taken out of the integral sign (which can be done on the basis of the mean value theorem known from higher mathematics) is the average velocity of particles ejected from the rocket.

Consisting of n material points. Let us single out some point from this system Mj with mass mj. It is known that external and internal forces act on this point.

Apply to a point Mj resultant of all internal forces F j i and the resultant of all external forces F j e(Figure 2.2). For selected material point Mj(as for a free point) we write the theorem on the change in momentum in differential form (2.3):

We write similar equations for all points of the mechanical system (j=1,2,3,…,n).

Figure 2.2

Let's put everything together n equations:

∑d(m j ×V j)/dt = ∑F j e + ∑F j i, (2.9)

d∑(m j ×V j)/dt = ∑ F j e + ∑ F j i. (2.10)

Here ∑mj ×Vj =Q is the momentum of the mechanical system;
∑ F j e = R e is the main vector of all external forces acting on the mechanical system;
∑ F j i = R i =0- the main vector of the internal forces of the system (according to the property of internal forces, it is equal to zero).

Finally, for the mechanical system, we obtain

dQ/dt = Re. (2.11)

Expression (2.11) is a theorem on the change in the momentum of a mechanical system in differential form (in vector expression): the time derivative of the momentum vector of a mechanical system is equal to the main vector of all external forces acting on the system.

Projecting the vector equality (2.11) onto the Cartesian coordinate axes, we obtain expressions for the theorem on the change in the momentum of a mechanical system in a coordinate (scalar) expression:

dQ x /dt = R x e;

dQ y /dt = R y e;

dQ z /dt = R z e, (2.12)

those. the time derivative of the projection of the momentum of a mechanical system onto any axis is equal to the projection onto this axis of the main vector of all external forces acting on this mechanical system.

Multiplying both sides of equality (2.12) by dt, we obtain the theorem in another differential form:

dQ = R e ×dt = δS e, (2.13)

those. the differential of momentum of a mechanical system is equal to the elementary impulse of the main vector (the sum of elementary impulses) of all external forces acting on the system.

Integrating equality (2.13) within the time range from 0 to t, we obtain a theorem on the change in the momentum of a mechanical system in a finite (integral) form (in vector expression):

Q - Q 0 \u003d S e,

those. the change in the amount of motion of a mechanical system over a finite period of time is equal to the total impulse of the main vector (the sum of the total impulses) of all external forces acting on the system over the same period of time.

Projecting the vector equality (2.14) onto the Cartesian coordinate axes, we obtain expressions for the theorem in projections (in a scalar expression):

those. the change in the projection of the momentum of the mechanical system on any axis over a finite period of time is equal to the projection on the same axis of the total impulse of the main vector (the sum of the total impulses) of all external forces acting on the mechanical system for the same period of time.

From the considered theorem (2.11) - (2.15) follow the following corollaries:

If R e = ∑ F j e = 0, then Q = const– we have the law of conservation of the momentum vector of the mechanical system: if the main vector Re of all external forces acting on a mechanical system is equal to zero, then the momentum vector of this system remains constant in magnitude and direction and equal to its initial value Q0, i.e. Q = Q0.
If R x e = ∑X j e =0 (R e ≠ 0), then Q x = const- we have the law of conservation of the projection onto the axis of the momentum of the mechanical system: if the projection of the main vector of all forces acting on the mechanical system on any axis is zero, then the projection onto the same axis of the momentum vector of this system will be a constant value and equal to the projection onto this axis initial momentum vector, i.e. Qx = Q0x.

The differential form of the theorem on the change in momentum of a material system has important and interesting applications in continuum mechanics. From (2.11) one can obtain Euler's theorem.

The amount of movement of a material point is called a vector quantity mv, equal to the product of the mass of the point and the vector of its velocity. Vector mV attached to a moving point.

Quantity of system movement is called a vector quantity Q, equal to the geometric sum (principal vector) of the momentum of all points of the system:

Vector Q is a free vector. In the SI system of units, the momentum modulus is measured in kg m/s or N s.

As a rule, the velocities of all points of the system are different (see, for example, the distribution of velocities of the points of a rolling wheel shown in Fig. 6.21), and therefore the direct summation of the vectors on the right side of equality (17.2) is difficult. Let us find a formula with the help of which the quantity Q much easier to calculate. It follows from equality (16.4) that

Taking the time derivative of both parts, we get Hence, taking into account equality (17.2), we find that

i.e., the amount of motion of the system is equal to the product of the mass of the entire system and the speed of its center of mass.

Note that the vector Q, like the main vector of forces in statics, is some generalized vector characteristic of the motion of the entire mechanical system. In the general case of motion of a system, its momentum is Q can be considered as a characteristic of the translational part of the motion of the system together with its center of mass. If during the movement of the system (body) the center of mass is stationary, then the momentum of the system will be equal to zero. Such, for example, is the momentum of a body rotating around a fixed axis passing through its center of mass.

Example. Determine the amount of motion of the mechanical system (Fig. 17.1, but), consisting of cargo BUT weight t A - 2 kg, homogeneous block IN weighing 1 kg and wheels D weight mD-4 kg. Cargo BUT moving at a speed V A - 2 m/s, wheel D rolls without slipping, the thread is inextensible and weightless. Solution. The amount of movement of the body system

Body BUT moving forward and Q A \u003d m A V A(numerically Q A= 4 kg m/s, vector direction Q A coincides with the direction VA). Block IN performs rotational motion around a fixed axis passing through its center of mass; Consequently, QB- 0. Wheel D makes a plane-parallel

motion; its instantaneous center of velocities is at the point TO, so the speed of its center of mass (points E) is equal to V E = V A /2= 1 m/s. Number of wheel movement Q D - m D V E - 4 kg m/s; vector Q D directed horizontally to the left.

Depicting vectors Q A And Q D in fig. 17.1, b, find the momentum Q systems according to formula (a). Taking into account the directions and numerical values of the quantities, we obtain Q ~^Q A +Q E=4l/2~kg m/s, vector direction Q shown in fig. 17.1, b.

Given that a-dV/dt, equation (13.4) of the basic law of dynamics can be represented as

Equation (17.4) expresses the theorem on the change in the momentum of a point in differential form: at each moment of time, the time derivative of the momentum of a point is equal to the force acting on the point. (In essence, this is another formulation of the basic law of dynamics, close to the one given by Newton.) If several forces act on a point, then on the right side of equality (17.4) there will be a resultant of the forces applied to the material point.

If both sides of the equation are multiplied by dt, then we get

The vector value on the right side of this equality characterizes the action exerted on the body by force in an elementary period of time dt this value is denoted dS and call elementary impulse of force, i.e.

Pulse S strength F over a finite time interval /, - / 0 is defined as the limit of the integral sum of the corresponding elementary impulses, i.e.

In a particular case, if the force F constant in modulus and in direction, then S = F(t| -/0) and S- F(t l -/ 0). In the general case, the modulus of the force impulse can be calculated from its projections onto the coordinate axes:

Now, integrating both sides of equality (17.5) with T= const, we get

Equation (17.9) expresses the theorem on changing the momentum of a point in finite (integral) form: the change in the momentum of a point over a certain period of time is equal to the momentum of the force acting on the point (or the momentum of the resultant of all forces applied to it) for the same period of time.

When solving problems, the equations of this theorem are used in projections onto the coordinate axes

Now consider a mechanical system consisting of P material points. Then, for each point, we can apply the momentum change theorem in the form (17.4), taking into account the external and internal forces applied to the points:

Summing up these equalities and taking into account that the sum of derivatives is equal to the derivative of the sum, we obtain

Since by the property of internal forces H.F.k=0 and by definition of momentum ^fn k V/ c = Q, then we finally find

Equation (17.11) expresses the theorem on the change in the momentum of the system in differential form: at each moment of time, the time derivative of the momentum of the system is equal to the geometric sum of all external forces acting on the system.

Projecting equality (17.11) onto the coordinate axes, we obtain

Multiplying both sides of (17.11) by dt and integrating, we get

where 0, Q0 - the amount of motion of the system at times, respectively, and / 0 .

Equation (17.13) expresses the theorem on the change in the momentum of the system in integral form: the change in the momentum of the system over any time is equal to the sum of the impulses of all external forces acting on the system over the same time.

In projections onto the coordinate axes, we get

From the theorem on the change in the momentum of the system, the following important consequences can be obtained, which express law of conservation of momentum of the system.

1. If the geometric sum of all external forces acting on the system is equal to zero (LF k=0), then from equation (17.11) it follows that in this case Q= const, i.e. the momentum vector of the system will be constant in magnitude and direction.
2. If the external forces acting on the system are such that the sum of their projections on any axis is zero (for example, I e kx = 0), then from equations (17.12) it follows that in this case Q x = const, i.e. the projection of the momentum of the system on this axis remains unchanged.

Note that the internal forces of the system do not participate in the equation of the theorem on the change in the momentum of the system. These forces, although they affect the momentum of individual points of the system, cannot change the momentum of the system as a whole. Given this circumstance, when solving problems, it is expedient to choose the system under consideration so that the unknown forces (all or part of them) are internal.

The law of conservation of momentum is convenient to apply in cases where the change in the speed of one part of the system is necessary to determine the speed of another part of it.

Problem 17.1. TO trolley weighing t x- 12 kg moving on a smooth horizontal plane, at a point BUT a weightless rod is attached with the help of a cylindrical hinge AD length /= 0.6 m with load D weight t 2 - 6 kg at the end (Fig. 17.2). At time / 0 = 0, when the speed of the trolley And () - 0.5 m/s, rod AD starts to rotate around the axis BUT, perpendicular to the plane of the drawing, according to the law φ \u003d (tg / 6) (3 ^ 2 - 1) rad (/- in seconds). Define: u=f.

§ 17.3. Theorem on the motion of the center of mass

The theorem on the change in the momentum of a mechanical system can be expressed in another form, which is called the theorem on the motion of the center of mass.

Substituting into equation (17.11) the equality Q=MV C , we get

If mass M system is constant, we get

where and with - acceleration of the center of mass of the system.

Equation (17.15) expresses the theorem on the motion of the center of mass of the system: the product of the mass of the system and the acceleration of its center of mass is equal to the geometric sum of all external forces acting on the system.

Projecting equality (17.15) onto the coordinate axes, we obtain

where x c , y c , z c - coordinates of the center of mass of the system.

These equations are differential equations of motion of the center of mass in projections onto the axes of the Cartesian coordinate system.

Let's discuss the results. Let us preliminarily recall that the center of mass of the system is a geometric point, sometimes located outside the geometric boundaries of the body. The forces acting on the mechanical system (external and internal) are applied to all material points of the system. Equations (17.15) make it possible to determine the motion of the center of mass of the system without determining the motion of its individual points. Comparing the equations (17.15) of the theorem on the motion of the center of mass and the equation (13.5) of Newton's second law for a material point, we come to the conclusion: the center of mass of a mechanical system moves as a material point, the mass of which is equal to the mass of the entire system, and as if all external forces acting on the system are applied to this point. Thus, the solutions that we obtain by considering a given body as a material point determine the law of motion of the center of mass of this body.

In particular, if the body moves forward, then the kinematic characteristics of all points of the body and its center of mass are the same. That's why a progressively moving body can always be considered as a material point with a mass equal to the mass of the entire body.

As can be seen from (17.15), the internal forces acting on the points of the system do not affect the motion of the center of mass of the system. Internal forces can influence the movement of the center of mass in those cases when external forces change under their influence. Examples of this will be given below.

From the theorem on the motion of the center of mass, the following important consequences can be obtained, which express the law of conservation of the motion of the center of mass of the system.

1. If the geometric sum of all external forces acting on the system is zero (LF k=0), then it follows from equation (17.15),

what about a c = 0 or V c = const, i.e. the center of mass of this system

moves with a constant speed in magnitude and direction (otherwise, uniformly and rectilinearly). In a special case, if at the beginning the center of mass was at rest ( Vc=0), then it will remain at rest; where

track predicts that its position in space will not change, i.e. rc = const.

2. If the external forces acting on the system are such that the sum of their projections on some axis (for example, the axis X) zero (?F e kx= 0), then from equation (17.16) it follows that in this case x s=0 or V Cx \u003d x c \u003d const, i.e., the projection of the velocity of the center of mass of the system onto this axis is a constant value. In a special case, if at the initial moment Vex= 0, then at any subsequent time this value will be preserved, and hence it follows that the coordinate x s the center of mass of the system will not change, i.e. x s - const.

Consider examples illustrating the law of motion of the center of mass.

Examples. 1. As noted, the movement of the center of mass depends only on external forces; internal forces cannot change the position of the center of mass. But the internal forces of the system can cause external influences. So, the movement of a person on a horizontal surface occurs under the action of friction forces between the soles of his shoes and the road surface. With the strength of his muscles (internal forces), a person pushes off the road surface with his feet, which causes a friction force (external for a person) at the points of contact with the road, directed in the direction of his movement.

2. The car moves in the same way. The internal pressure forces in its engine make the wheels rotate, but since the latter have traction, the friction forces that arise “push” the car forward (as a result, the wheels do not rotate, but move in a plane-parallel way). If the road is absolutely smooth, then the center of mass of the car will be stationary (at zero initial speed) and the wheels, in the absence of friction, will slip, i.e., rotate.
3. Movement with the help of a propeller, propeller, oars occurs due to the rejection of a certain mass of air (or water). If we consider the discarded mass and the moving body as one system, then the forces of interaction between them, as internal, cannot change the total momentum of this system. However, each of the parts of this system will move, for example, the boat forward, and the water that the oars throw back.
4. In airless space, when the rocket is moving, the “discarded mass” should be “taken with you”: the jet engine informs the rocket about the movement by throwing back the combustion products of the fuel that the rocket is filled with.
5. When descending on a parachute, you can control the movement of the center of mass of the man-parachute system. If by muscular effort a person pulls the parachute lines in such a way that the shape of its canopy or the angle of attack of the air flow changes, then this will cause a change in the external influence of the air flow, and thereby affect the movement of the entire system.

Problem 17.2. IN task 17.1 (see Figure 17.2) determine: 1) law of motion of the trolley X (= /)(/), if it is known that at the initial moment of time t 0 = About the system was at rest and the coordinate x 10 = 0; 2) the law of change with time of the total value of the normal reaction N(N = N" + N") horizontal plane, i.e. N=f 2 (t).

Solution. Here, as in problem 17.1, we consider a system consisting of a trolley and a load D, in an arbitrary position under the action of external forces applied to it (see Fig. 17.2). Coordinate axes Ohu draw so that the x-axis is horizontal and the x-axis at passed through the point A 0 , i.e. the location of the point BUT at the time t-t 0 - 0.

1. Determination of the law of motion of the cart. To determine x, = /, (0, we use the theorem on the motion of the center of mass of the system. Let us compose a differential equation of its motion in projection onto the x axis:

Since all external forces are vertical, then T, F e kx = 0, and therefore

Integrating this equation, we find that Mx c \u003d B, i.e., the projection of the velocity of the center of mass of the system on the x-axis is a constant value. Since at the initial moment of time

Integrating the equation Mx s= 0, we get

i.e. coordinate x s the center of mass of the system is constant.

Let's write the expression Mx s for an arbitrary position of the system (see Fig. 17.2), taking into account that x A - x { , x D - x 2 And x 2 - x ( - I sin f. In accordance with formula (16.5), which determines the coordinate of the center of mass of the system, in this case Mx s - t(x( + t 2 x 2".

for an arbitrary point in time

for time point / () = 0, X (= 0 and

In accordance with equality (b), the coordinate x s the center of mass of the entire system remains unchanged, i.e. x c (t). Therefore, by equating expressions (c) and (d), we obtain the dependence of the x coordinate on time.

Answer: X - 0.2 m, where t- in seconds.

2. Reaction definition N. For determining N=f 2 (t) we compose the differential equation of motion of the center of mass of the system in projection onto the vertical axis at(see fig. 17.2):

Hence, denoting N=N+N", we get

According to the formula that determines the ordinate u s the center of mass of the system, Mu s = t (y x + t 2 y 2, where y, = at C1,at 2= yD = Atbut ~ 1 cos Ф» we get

Differentiating this equality twice with respect to time (taking into account that at C1 And at A the quantities are constant and, consequently, their derivatives are equal to zero), we find

Substituting this expression into equation (e), we determine the required dependence N from t.

Answer: N- 176,4 + 1,13,

where φ \u003d (i / 6) (3 / -1), t- in seconds N- in newtons.

Problem 17.3. Electric motor mass t x attached to the horizontal surface of the foundation with bolts (Fig. 17.3). On the motor shaft at right angles to the axis of rotation, a weightless rod of length l is fixed at one end, and a point weight is mounted on the other end of the rod BUT weight t 2 . The shaft rotates uniformly at an angular velocity o. Find the horizontal pressure of the motor on the bolts. Solution. Consider a mechanical system consisting of a motor and a point weight BUT, in an arbitrary position. Let us depict the external forces acting on the system: gravity R x, R 2, foundation reaction in the form of a vertical force N and horizontal force R. Draw the x-axis horizontally.

To determine the horizontal pressure of the motor on the bolts (and it will be numerically equal to the reaction R and directed opposite to the vector R ), we compose the equation of the theorem on the change in the momentum of the system in projection onto the horizontal axis x:

For the system under consideration in its arbitrary position, given that the amount of motion of the motor housing is zero, we obtain Q x = - t 2 U A col. Taking into account that V A = a s/, φ = ω/ (uniform rotation of the motor), we get Q x - - m 2 co/cos co/. differentiating Q x in time and substituting into equality (a), we find R- m 2 co 2 /sin co/.

Note that it is precisely such forces that are forcing (see § 14.3), when they act, forced vibrations of structures occur.

Exercises for independent work

1. What is called the momentum of a point and a mechanical system?
2. How does the momentum of a point moving uniformly around a circle change?
3. What characterizes the impulse of force?
4. Do the internal forces of the system affect its momentum? On the movement of its center of mass?
5. How do couples of forces applied to it affect the motion of the center of mass of the system?
6. Under what conditions is the center of mass of the system at rest? moving uniformly and in a straight line?

7. In a stationary boat, in the absence of water flow, an adult sits at the stern, and a child sits at the bow of the boat. In which direction will the boat move if they switch places?

In which case will the displacement module of the boat be large: 1) if the child goes to the adult in the stern; 2) if an adult goes to the child on the bow of the boat? What will be the displacements of the center of mass of the “boat and two people” system during these movements?

Let the material point move under the action of force F. It is required to determine the motion of this point with respect to the moving system Oxyz(see the complex motion of a material point), which moves in a known way with respect to a fixed system O 1 x 1 y 1 z 1 .

The basic equation of dynamics in a stationary system

We write the absolute acceleration of a point according to the Coriolis theorem

where a abs– absolute acceleration;

a rel– relative acceleration;

a lane– portable acceleration;

a core is the Coriolis acceleration.

Let us rewrite (25) taking into account (26)

Let us introduce the notation
- portable force of inertia,
is the Coriolis force of inertia. Then equation (27) takes the form

The basic equation of dynamics for studying relative motion (28) is written in the same way as for absolute motion, only the translational and Coriolis forces of inertia must be added to the forces acting on the point.

General theorems of material point dynamics

When solving many problems, you can use pre-made blanks obtained on the basis of Newton's second law. Such problem solving methods are combined in this section.

Theorem on the change in momentum of a material point

Let us introduce the following dynamic characteristics:

1. Quantity of movement of a material point is a vector quantity equal to the product of the mass of a point and the vector of its velocity

. (29)

2. Impulse of force

Elemental Force Impulse- a vector quantity equal to the product of the force vector by an elementary time interval

(30).

Then full impulse

. (31)

At F=const we get S=ft.

The total impulse over a finite period of time can be calculated only in two cases, when the force acting on the point is constant or depends on time. In other cases, it is necessary to express the force as a function of time.

The equality of the dimensions of momentum (29) and momentum (30) makes it possible to establish a quantitative relationship between them.

Consider the motion of a material point M under the action of an arbitrary force F along an arbitrary path.

ABOUT UD:
. (32)

We separate variables in (32) and integrate

. (33)

As a result, taking into account (31), we obtain

. (34)

Equation (34) expresses the following theorem.

Theorem: The change in the momentum of a material point over a certain period of time is equal to the impulse of the force acting on the point over the same time interval.

When solving problems, equation (34) must be projected on the coordinate axes

This theorem is convenient to use when the given and unknown quantities include the mass of a point, its initial and final velocity, forces, and time of motion.

Theorem on the change in the angular momentum of a material point

M
moment of momentum of a material point relative to the center is equal to the product of the momentum modulus of the point and the arm, i.e. shortest distance (perpendicular) from the center to a line coinciding with the velocity vector

, (36)

. (37)

The relationship between the moment of force (cause) and the moment of momentum (effect) is established by the following theorem.

Let point M of given mass m moving under the influence of force F.

,
,

, (38)

. (39)

Let us calculate the derivative of (39)

. (40)

Combining (40) and (38), we finally obtain

. (41)

Equation (41) expresses the following theorem.

Theorem: The time derivative of the angular momentum vector of a material point relative to some center is equal to the moment of the force acting on the point relative to the same center.

When solving problems, equation (41) must be projected on the coordinate axes

In equations (42), the moments of momentum and force are calculated relative to the coordinate axes.

From (41) it follows law of conservation of angular momentum (Kepler's law).

If the moment of force acting on a material point relative to any center is equal to zero, then the angular momentum of the point relative to this center retains its magnitude and direction.

If
, then
.

The theorem and the conservation law are used in curvilinear motion problems, especially under the action of central forces.

For a material point, the basic law of dynamics can be represented as

Multiplying both parts of this relation on the left vectorially by the radius vector (Fig. 3.9), we obtain

(3.32)

On the right side of this formula, we have the moment of force relative to the point O. Let's transform the left side by applying the formula for the derivative of the vector product

But as a cross product of parallel vectors. After that we get

(3.33)

The first time derivative of the moment of momentum of a point relative to any center is equal to the moment of force relative to the same center.

An example of calculating the angular momentum of a system. Calculate the angular momentum relative to point O of a system consisting of a cylindrical shaft with a mass M = 20 kg and a radius R = 0.5 m and a descending load with a mass m = 60 kg (Figure 3.12). The shaft rotates around the Oz axis with an angular velocity ω = 10 s -1 .

Figure 3.12

; ;

For given input data, the angular momentum of the system

Theorem on the change in the kinetic moment of the system. We apply the resultant external and internal forces to each point of the system. For each point of the system, you can apply the theorem on the change in the angular momentum, for example, in the form (3.33)

Summing over all points of the system and taking into account that the sum of derivatives is equal to the derivative of the sum, we obtain

By definition of the kinetic moment of the system and the property of external and internal forces

Therefore, the resulting ratio can be represented as

The first time derivative of the kinetic moment of the system with respect to any point is equal to the main moment of the external forces acting on the system with respect to the same point.

3.3.5. Force work

1) The elementary work of the force is equal to the scalar product of the force and the differential radius of the vector of the point of application of the force (Fig. 3.13)

Figure 3.13

Expression (3.36) can also be written in the following equivalent forms

where is the projection of the force on the direction of the velocity of the point of application of the force.

2) The work of the force on the final displacement

Integrating the elementary work of the force, we obtain the following expressions for the work of the force on the final displacement from point A to point B

3) Work of a constant force

If the force is constant, then from (3.38) it follows

The work of a constant force does not depend on the shape of the trajectory, but depends only on the displacement vector of the point of application of the force.

4) Weight force work

For the weight force (Fig. 3.14) and from (3.39) we obtain

Figure 3.14

If the movement is from point B to point A, then

In general

The sign "+" corresponds to the movement of the point of application of force "down", the sign "-" - up.

4) The work of the force of elasticity

Let the axis of the spring be directed along the x axis (Fig. 3.15), and the end of the spring moves from point 1 to point 2, then from (3.38) we obtain