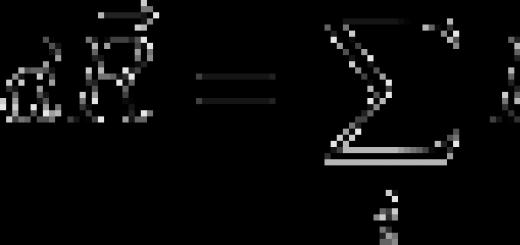ELECTROMAGNETIC OSCILLATIONS. FREE AND FORCED ELECTRIC OSCILLATIONS IN THE OSCILLATION CIRCUIT.
- Electromagnetic vibrations- interconnected fluctuations of electric and magnetic fields.
Electromagnetic oscillations appear in various electrical circuits. In this case, the magnitude of the charge, voltage, current strength, intensity fluctuate electric field, induction magnetic field and other electrodynamic quantities.
Free electromagnetic oscillationsarise in the electromagnetic system after removing it from the state of equilibrium, for example, by imparting a charge to the capacitor or by changing the current in the circuit section.
These are damped vibrations, since the energy communicated to the system is spent on heating and other processes.
Forced electromagnetic oscillations- undamped oscillations in the circuit caused by an external periodically changing sinusoidal EMF.
Electromagnetic oscillations are described by the same laws as mechanical ones, although the physical nature of these oscillations is completely different.
Electrical vibrations - special case electromagnetic, when only oscillations of electrical quantities are considered. In this case, they talk about alternating current, voltage, power, etc.
- OSCILLATORY CIRCUIT
An oscillatory circuit is an electrical circuit consisting of a series-connected capacitor with a capacitance C, an inductor with an inductance Land a resistor with resistance R. Ideal circuit - if the resistance can be neglected, that is, only the capacitor C and the ideal coil L.
The state of stable equilibrium of the oscillatory circuit is characterized by the minimum energy of the electric field (the capacitor is not charged) and the magnetic field (there is no current through the coil).
- CHARACTERISTICS OF ELECTROMAGNETIC OSCILLATIONS
Analogy of mechanical and electromagnetic oscillations
Characteristics: | Mechanical vibrations | Electromagnetic vibrations |
Quantities expressing the properties of the system itself (system parameters): | m- mass (kg) k- spring rate (N/m) | L- inductance (H) 1/C- reciprocal of capacitance (1/F) |
Quantities characterizing the state of the system: | Kinetic energy (J) Potential energy (J) x - displacement (m) | Electrical energy(J) Magnetic energy (J) q - capacitor charge (C) |
Quantities expressing the change in the state of the system: | v = x"(t) displacement speed (m/s) | i = q"(t) current strength - rate of change of charge (A) |
Other Features: T=1/ν T=2π/ω ω=2πν | T- oscillation period time of one complete oscillation (s) |
|
ν- frequency - number of vibrations per unit of time (Hz) |
||
ω - cyclic frequency number of vibrations per 2π seconds (Hz) |
||
φ=ωt - oscillation phase - shows what part of the amplitude value it takes in this moment fluctuating value, i.e.the phase determines the state of the oscillating system at any time t. |
||
where q" is the second derivative of charge with respect to time.
Value is the cyclic frequency. The same equations describe fluctuations in current, voltage, and other electrical and magnetic quantities.
One of the solutions to equation (1) is the harmonic function
This is the integral equation harmonic vibrations.
Oscillation period in the circuit (Thomson formula):
The value φ = ώt + φ 0 , standing under the sign of sine or cosine, is the phase of the oscillation.
The current in the circuit is equal to the derivative of the charge with respect to time, it can be expressed
The voltage on the capacitor plates varies according to the law:
Where I max \u003d ωq poppy is the amplitude of the current (A),
Umax=qmax /C - voltage amplitude (V)
Exercise: for each state of the oscillatory circuit, write down the values of the charge on the capacitor, current in the coil, electric field strength, magnetic field induction, electric and magnetic energy.
The main value of the presentation material is the visibility of the phased accentuated dynamics of the formation of concepts related to the laws of mechanical and especially electromagnetic oscillations in oscillatory systems.
Download:
Slides captions:
Analogy between mechanical and electromagnetic oscillations. For 11th grade students Belgorod region Gubkin MBOU "Secondary School No. 3" Skarzhinsky Ya.Kh. ©
Oscillatory circuit
Oscillating circuit Oscillating circuit in the absence of active R
Electrical oscillatory system Mechanical oscillatory system
Electrical oscillatory system with the potential energy of a charged capacitor Mechanical oscillatory system with the potential energy of a deformed spring
Analogy between mechanical and electromagnetic oscillations. SPRING CAPACITOR LOAD COIL A Mechanical quantities Electrical quantities Coordinate x Charge q Velocity v x Current i Mass m Inductance L Potential energy kx 2 /2 Electric field energy q 2 /2 Spring constant k Reciprocal of capacitance 1/C Kinetic energy mv 2 / 2 Magnetic field energy Li 2 /2
Analogy between mechanical and electromagnetic oscillations. 1 Find the energy of the magnetic field of the coil in the oscillatory circuit, if its inductance is 5 mH, and the maximum current strength is 0.6 mA. 2 What was the maximum charge on the capacitor plates in the same oscillatory circuit, if its capacitance was 0.1 pF? Solving qualitative and quantitative problems on a new topic.
Homework: §
On the topic: methodological developments, presentations and notes
The main goals and objectives of the lesson: To test knowledge, skills and abilities on the topic covered, taking into account individual features each student. Encourage strong students to expand their activities ...
summary of the lesson "Mechanical and electromagnetic oscillations"
This development can be used when studying the topic in grade 11: "Electromagnetic oscillations." The material is designed to study a new topic....
Although mechanical and electromagnetic vibrations have different nature, many analogies can be drawn between them. For example, consider electromagnetic oscillations in an oscillatory circuit and the oscillation of a load on a spring.
Swinging load on a spring
With mechanical oscillations of a body on a spring, the coordinate of the body will periodically change. In this case, we will change the projection of the body velocity on the Ox axis. In electromagnetic oscillations over time periodic law the charge q of the capacitor will change, and the current strength in the oscillatory circuit circuit.
The values will have the same pattern of change. This is because there is an analogy between the conditions under which oscillations occur. When we remove the load on the spring from the equilibrium position, an elastic force F control arises in the spring, which tends to return the load back to the equilibrium position. The coefficient of proportionality of this force will be the stiffness of the spring k.
When the capacitor is discharged, a current appears in the oscillating circuit circuit. The discharge is due to the fact that there is a voltage u on the capacitor plates. This voltage will be proportional to the charge q of any of the plates. The proportionality factor will be the value 1/C, Where C is the capacitance of the capacitor.
When a load moves on a spring, when we release it, the speed of the body increases gradually, due to inertia. And after the termination of the force, the speed of the body does not immediately become equal to zero, it also gradually decreases.
Oscillatory circuit
The same is true in the oscillatory circuit. Electricity in the coil under the action of voltage does not increase immediately, but gradually, due to the phenomenon of self-induction. And when the voltage ceases to act, the current strength does not immediately become equal to zero.
That is, in the oscillatory circuit, the inductance of the coil L will be similar to the mass of the body m, when the load oscillates on the spring. Consequently, the kinetic energy of the body (m * V ^ 2) / 2, will be similar to the energy of the magnetic field of the current (L * i ^ 2) / 2.
When we remove the load from the equilibrium position, we inform the mind of some potential energy (k * (Xm) ^ 2) / 2, where Xm is the displacement from the equilibrium position.
In the oscillatory circuit, the role of potential energy is performed by the charge energy of the capacitor q ^ 2 / (2 * C). We can conclude that the stiffness of the spring in mechanical vibrations will be similar to the value 1/C, where C is the capacitance of the capacitor in electromagnetic vibrations. And the coordinate of the body will be similar to the charge of the capacitor.
Let us consider in more detail the processes of oscillations, in the following figure.
picture
(a) We inform the body of potential energy. By analogy, we charge the capacitor.
(b) We release the ball, the potential energy begins to decrease, and the speed of the ball increases. By analogy, the charge on the capacitor plate begins to decrease, and a current appears in the circuit.
(c) Equilibrium position. There is no potential energy, the speed of the body is maximum. The capacitor is discharged, the current in the circuit is maximum.
(e) The body deviated in the extreme position, its velocity became equal to zero, and the potential energy reached its maximum. The capacitor charged again, the current in the circuit began to equal zero.
Development of a methodology for studying the topic "Electromagnetic oscillations"
Oscillatory circuit. Energy transformations during electromagnetic oscillations.
These questions, which are among the most important in this topic, are dealt with in the third lesson.
First, the concept of an oscillatory circuit is introduced, an appropriate entry is made in a notebook.
Further, in order to find out the cause of the occurrence of electromagnetic oscillations, a fragment is shown, which shows the process of charging the capacitor. The attention of students is drawn to the signs of the charges of the capacitor plates.
After that, the energies of the magnetic and electric fields are considered, the students are told about how these energies and the total energy in the circuit change, the mechanism for the occurrence of electromagnetic oscillations is explained using the model, and the basic equations are recorded.
It is very important to draw students' attention to the fact that such a representation of the current in the circuit (the flow of charged particles) is conditional, since the speed of electrons in the conductor is very low. This method of representation was chosen to facilitate understanding of the essence of electromagnetic oscillations.
Further, the attention of students is focused on the fact that they observe the processes of converting the energy of the electric field into magnetic energy and vice versa, and since the oscillatory circuit is ideal (there is no resistance), the total energy electromagnetic field remains unchanged. After that, the concept of electromagnetic oscillations is given and it is stipulated that these oscillations are free. Then the results are summed up and homework is given.
Analogy between mechanical and electromagnetic oscillations.
This question is considered in the fourth lesson of the study of the topic. First, for repetition and consolidation, you can once again demonstrate the dynamic model of an ideal oscillatory circuit. To explain the essence and prove the analogy between electromagnetic oscillations and oscillations of a spring pendulum, the dynamic oscillatory model “Analogy between mechanical and electromagnetic oscillations” and PowerPoint presentations are used.
A spring pendulum (oscillations of a load on a spring) is considered as a mechanical oscillatory system. Revealing the relationship between mechanical and electrical quantities at oscillatory processes carried out according to the traditional method.
As it was already done in the last lesson, it is necessary to remind the students once again about the conditionality of the movement of electrons along the conductor, after which their attention is drawn to the upper right corner of the screen, where the “communicating vessels” oscillatory system is located. It is stipulated that each particle oscillates around the equilibrium position, therefore, fluid oscillations in communicating vessels can also serve as an analogy for electromagnetic oscillations.

If there is time left at the end of the lesson, then you can dwell on the demonstration model in more detail, analyze all the main points using the newly studied material.
The equation of free harmonic oscillations in the circuit.
At the beginning of the lesson, dynamic models of an oscillatory circuit and analogies of mechanical and electromagnetic oscillations are demonstrated, the concepts of electromagnetic oscillations, an oscillatory circuit, the correspondence of mechanical and electromagnetic quantities in oscillatory processes are repeated.
The new material must begin with the fact that if the oscillatory circuit is ideal, then its total energy remains constant over time
those. its time derivative is constant, and hence the time derivatives of the energies of the magnetic and electric fields are also constant. Then, after a series of mathematical transformations, they come to the conclusion that the equation of electromagnetic oscillations is similar to the equation of oscillations of a spring pendulum.
Referring to the dynamic model, students are reminded that the charge in the capacitor changes periodically, after which the task is to find out how the charge, the current in the circuit and the voltage across the capacitor depend on time.
These dependencies are found by the traditional method. After the equation of capacitor charge fluctuations is found, students are shown a picture that shows graphs of the dependence of the capacitor charge and the displacement of the load on time, which are cosine waves.
In the course of elucidating the equation for oscillations of the charge of a capacitor, the concepts of the period of oscillations, cyclic and natural frequencies of oscillations are introduced. Then the Thomson formula is derived.
Next, the equations for fluctuations in the current strength in the circuit and the voltage on the capacitor are obtained, after which a picture is shown with graphs of the dependence of three electrical quantities on time. Students' attention is drawn to the phase shift between current fluctuations and charges by its absence between voltage and charge fluctuations.
After all three equations are derived, the concept of damped oscillations is introduced and a picture is shown showing these oscillations.
In the next lesson, summed up summary with the repetition of the basic concepts, the problems of finding the period, cyclic and natural frequencies of oscillations are solved, the dependences q(t), U(t), I(t), as well as various qualitative and graphic problems are investigated.
4. Methodical development three lessons
The lessons below are designed as lectures, since this form, in my opinion, is the most productive and leaves enough time in this case to work with dynamic demos. ion models. If desired, this form can be easily transformed into any other form of the lesson.
Lesson topic: Oscillatory circuit. Energy transformations in an oscillatory circuit.
Explanation of new material.
The purpose of the lesson: explanation of the concept of an oscillatory circuit and the essence of electromagnetic oscillations using the dynamic model “Ideal oscillatory circuit”.
Oscillations can occur in a system called an oscillatory circuit, consisting of a capacitor with a capacitance C and an inductance coil L. An oscillatory circuit is called ideal if there is no energy loss in it for heating the connecting wires and coil wires, i.e., the resistance R is neglected.

Let's make a drawing of a schematic image of an oscillatory circuit in notebooks.
In order for electrical oscillations to occur in this circuit, it is necessary to inform it of a certain amount of energy, i.e. charge the capacitor. When the capacitor is charged, the electric field will be concentrated between its plates.
(Let's follow the process of charging the capacitor and stop the process when the charging is completed).
So, the capacitor is charged, its energy is equal to
therefore, therefore,
Since after charging the capacitor will have a maximum charge (pay attention to the capacitor plates, they have charges opposite in sign), then at q \u003d q max, the energy of the electric field of the capacitor will be maximum and equal to
At the initial moment of time, all the energy is concentrated between the plates of the capacitor, the current in the circuit is zero. (Let's now close the capacitor to the coil on our model). When the capacitor closes to the coil, it begins to discharge and a current will appear in the circuit, which, in turn, will create a magnetic field in the coil. The lines of force of this magnetic field are directed according to the gimlet rule.

When the capacitor is discharged, the current does not immediately reach its maximum value, but gradually. This is because the alternating magnetic field generates a second electric field in the coil. Due to the phenomenon of self-induction, an induction current arises there, which, according to the Lenz rule, is directed in the direction opposite to the increase in the discharge current.
When the discharge current reaches its maximum value, the energy of the magnetic field is maximum and is equal to:
and the energy of the capacitor at this moment is zero. Thus, through t=T/4 the energy of the electric field has completely passed into the energy of the magnetic field.
(Let's observe the process of discharging a capacitor on a dynamic model. I draw your attention to the fact that this way of representing the processes of charging and discharging a capacitor in the form of a flow of running particles is conditional and is chosen for ease of perception. You know very well that the speed of electrons is very small ( order of several centimeters per second). So, you see how, with a decrease in the charge on the capacitor, the current strength in the circuit changes, how the energies of the magnetic and electric fields change, what relationship exists between these changes. Since the circuit is ideal, there is no energy loss , so the total energy of the circuit remains constant).
With the start of recharging the capacitor, the discharge current will decrease to zero not immediately, but gradually. This is again due to the occurrence of counter-e. d.s. and inductive current of opposite direction. This current counteracts the decrease in the discharge current, as it previously counteracted its increase. Now it will support the main current. The energy of the magnetic field will decrease, the energy of the electric field will increase, the capacitor will be recharged.
Thus, the total energy of the oscillatory circuit at any time is equal to the sum of the energies of the magnetic and electric fields
The oscillations at which the energy of the electric field of the capacitor is periodically converted into the energy of the magnetic field of the coil are called ELECTROMAGNETIC oscillations. Since these oscillations occur due to the initial energy supply and without external influences, they are FREE.
Lesson topic: Analogy between mechanical and electromagnetic oscillations.
Explanation of new material.
The purpose of the lesson: to explain the essence and prove the analogy between electromagnetic oscillations and oscillations of a spring pendulum using the dynamic oscillation model “Analogy between mechanical and electromagnetic oscillations” and PowerPoint presentations.
Material to repeat:
the concept of an oscillatory circuit;
the concept of an ideal oscillatory circuit;
conditions for the occurrence of fluctuations in c / c;
concepts of magnetic and electric fields;
fluctuations as a process of periodic energy change;
the energy of the circuit at an arbitrary point in time;
the concept of (free) electromagnetic oscillations.
(For repetition and consolidation, students are once again shown a dynamic model of an ideal oscillatory circuit).
In this lesson, we will look at the analogy between mechanical and electromagnetic oscillations. We will consider a spring pendulum as a mechanical oscillatory system.

(On the screen you see a dynamic model that demonstrates the analogy between mechanical and electromagnetic oscillations. It will help us understand oscillatory processes, both in a mechanical system and in an electromagnetic one).
So, in a spring pendulum, an elastically deformed spring imparts velocity to the load attached to it. A deformed spring has the potential energy of an elastically deformed body
a moving object has kinetic energy
The transformation of the potential energy of a spring into the kinetic energy of an oscillating body is a mechanical analogy of the transformation of the energy of the electric field of a capacitor into the energy of the magnetic field of a coil. In this case, the analog of the mechanical potential energy of the spring is the energy of the electric field of the capacitor, and the analog of the mechanical kinetic energy of the load is the energy of the magnetic field, which is associated with the movement of charges. Charging the capacitor from the battery corresponds to the message to the spring of potential energy (for example, displacement by hand).
Let's compare the formulas and derive general patterns for electromagnetic and mechanical vibrations.
From a comparison of the formulas, it follows that the analog of the inductance L is the mass m, and the analog of the displacement x is the charge q, the analog of the coefficient k is the reciprocal of the electrical capacity, i.e. 1/C.
The moment when the capacitor is discharged, and the current strength reaches its maximum, corresponds to the body passing the equilibrium position with maximum speed(pay attention to the screens: there you can observe this correspondence).

As already mentioned in the last lesson, the movement of electrons along a conductor is conditional, because for them the main type of movement is oscillating motion around the equilibrium position. Therefore, sometimes electromagnetic oscillations are compared with oscillations of water in communicating vessels (look at the screen, you can see that such an oscillatory system is located in the upper right corner), where each particle oscillates around the equilibrium position.
So, we found out that the analogy of inductance is mass, and the analogy of displacement is charge. But you know very well that a change in charge per unit of time is nothing more than a current strength, and a change in coordinates per unit of time is a speed, that is, q "= I, and x" = v. Thus, we have found another correspondence between mechanical and electrical quantities.
Let's make a table that will help us systematize the relationships between mechanical and electrical quantities in oscillatory processes.
Correspondence table between mechanical and electrical quantities in oscillatory processes.

Lesson topic: The equation of free harmonic oscillations in the circuit.
Explanation of new material.
The purpose of the lesson: the derivation of the basic equation of electromagnetic oscillations, the laws of change in charge and current strength, obtaining the Thomson formula and the expression for the natural frequency of the oscillation of the circuit using PowerPoint presentations.
Material to repeat:
the concept of electromagnetic oscillations;
the concept of the energy of an oscillatory circuit;
correspondence of electrical quantities to mechanical quantities during oscillatory processes.
(For repetition and consolidation, it is necessary to once again demonstrate the model of the analogy of mechanical and electromagnetic oscillations).
In the past lessons, we found out that electromagnetic oscillations, firstly, are free, and secondly, they represent a periodic change in the energies of the magnetic and electric fields. But in addition to energy, during electromagnetic oscillations, the charge also changes, and hence the current strength in the circuit and the voltage. In this lesson, we must find out the laws by which the charge changes, which means the current strength and voltage.
So, we found out that the total energy of the oscillatory circuit at any time is equal to the sum of the energies of the magnetic and electric fields: . We believe that the energy does not change with time, that is, the contour is ideal. This means that the time derivative of the total energy is equal to zero, therefore, the sum of the time derivatives of the energies of the magnetic and electric fields is equal to zero:
I.e.
The minus sign in this expression means that when the energy of the magnetic field increases, the energy of the electric field decreases and vice versa. BUT physical meaning of this expression is such that the rate of change in the energy of the magnetic field is equal in absolute value and opposite in direction to the rate of change in the electric field.
Calculating the derivatives, we get

But, therefore, and - we got an equation describing free electromagnetic oscillations in the circuit. If we now replace q with x, x""=a x with q"", k with 1/C, m with L, we get the equation
describing the vibrations of a load on a spring. Thus, the equation of electromagnetic oscillations has the same mathematical form, as the equation of oscillation of a spring pendulum.
As you saw in the demo model, the charge on the capacitor changes periodically. It is necessary to find the dependence of the charge on time.
From the ninth grade, you are familiar with the periodic functions sine and cosine. These functions have the following property: the second derivative of the sine and cosine is proportional to the functions themselves, taken with the opposite sign. Apart from these two, no other functions have this property. Now back to electric charge. It can be boldly stated that electric charge, and hence the current strength, with free oscillations change over time according to the cosine or sine law, i.e. make harmonic vibrations. The spring pendulum also perform harmonic oscillations (acceleration is proportional to the displacement, taken with a minus sign).
So, in order to find the explicit dependence of the charge, current and voltage on time, it is necessary to solve the equation
taking into account the harmonic nature of the change in these quantities.
If we take an expression like q = q m cos t as a solution, then, when substituting this solution into the original equation, we get q""=-q m cos t=-q.
Therefore, as a solution, it is necessary to take an expression of the form
q=q m cossh o t,
where q m is the amplitude of charge oscillations (modulus the greatest value fluctuating value),
w o = - cyclic or circular frequency. Its physical meaning is
the number of oscillations in one period, i.e., for 2p s.
The period of electromagnetic oscillations is the period of time during which the current in the oscillatory circuit and the voltage on the capacitor plates make one complete oscillation. For harmonic oscillations Т=2р with ( smallest period cosine).
The oscillation frequency - the number of oscillations per unit time - is determined as follows: n = .
The frequency of free oscillations is called the natural frequency of the oscillatory system.
Since w o \u003d 2p n \u003d 2p / T, then T \u003d.
We defined the cyclic frequency as w o = , which means that for the period we can write
Т= = - Thomson's formula for the period of electromagnetic oscillations.
Then the expression for the natural oscillation frequency takes the form

It remains for us to obtain the equations for the oscillations of the current strength in the circuit and the voltage across the capacitor.
Since, then at q = q m cos u o t we get U=U m cos o t. This means that the voltage also changes according to the harmonic law. Let us now find the law according to which the current strength in the circuit changes.
By definition, but q=q m cosшt, so
where p/2 is the phase shift between current and charge (voltage). So, we found out that the current strength during electromagnetic oscillations also changes according to the harmonic law.
We considered an ideal oscillatory circuit in which there is no energy loss and free oscillations can continue indefinitely due to the energy once received from external source. In a real circuit, part of the energy goes to heating the connecting wires and heating the coil. Therefore, free oscillations in the oscillatory circuit are damped.
Themes USE codifier Keywords: free electromagnetic oscillations, oscillatory circuit, forced electromagnetic oscillations, resonance, harmonic electromagnetic oscillations.
Electromagnetic vibrations are periodic changes in charge, current and voltage that occur in electrical circuit. The simplest system for observing electromagnetic oscillations is an oscillatory circuit.
Oscillatory circuit
Oscillatory circuit It is a closed circuit formed by a capacitor and a coil connected in series.
We charge the capacitor, connect a coil to it and close the circuit. will start happening free electromagnetic oscillations- periodic changes in the charge on the capacitor and the current in the coil. We recall that these vibrations are called free because they occur without any external influence - only due to the energy stored in the circuit.
We denote the period of oscillations in the circuit, as always, through . The resistance of the coil will be considered equal to zero.
Let us consider in detail all the important stages of the oscillation process. For greater clarity, we will draw an analogy with the oscillations of a horizontal spring pendulum.
Starting moment: . The charge of the capacitor is equal, there is no current through the coil (Fig. 1). The capacitor will now start to discharge.

Rice. one.
Despite the fact that the resistance of the coil is zero, the current will not increase instantly. As soon as the current begins to increase, an EMF of self-induction will appear in the coil, which prevents the current from increasing.
Analogy. The pendulum is pulled to the right by a value and is released at the initial moment. The initial speed of the pendulum is zero.
First quarter of the period: . The capacitor is discharging, its current charge is . The current through the coil increases (Fig. 2).

Rice. 2.
The increase in current occurs gradually: the eddy electric field of the coil prevents the increase in current and is directed against the current.
Analogy. The pendulum moves to the left towards the equilibrium position; the speed of the pendulum gradually increases. The deformation of the spring (it is also the coordinate of the pendulum) decreases.
End of the first quarter: . The capacitor is completely discharged. The current strength has reached its maximum value (Fig. 3). The capacitor will now start charging.

Rice. 3.
The voltage on the coil is zero, but the current will not disappear instantly. As soon as the current begins to decrease, an EMF of self-induction will appear in the coil, preventing the current from decreasing.
Analogy. The pendulum passes the equilibrium position. Its speed reaches its maximum value. The spring deflection is zero.
Second quarter: . The capacitor is recharged - a charge of the opposite sign appears on its plates compared to what it was at the beginning ( fig. 4).

Rice. 4.
The current strength decreases gradually: the eddy electric field of the coil, supporting the decreasing current, is co-directed with the current.
Analogy. The pendulum continues to move to the left - from the equilibrium position to the right extreme point. Its speed gradually decreases, the deformation of the spring increases.
End of second quarter. The capacitor is completely recharged, its charge is again equal (but the polarity is different). The current strength is zero (Fig. 5). Now the reverse charge of the capacitor will begin.

Rice. 5.
Analogy. The pendulum has reached its extreme right point. The speed of the pendulum is zero. The deformation of the spring is maximum and equal to .
third quarter: . The second half of the oscillation period began; processes went in the opposite direction. The capacitor is discharged ( fig. 6).

Rice. 6.
Analogy. The pendulum moves back: from the right extreme point to the equilibrium position.
End of the third quarter: . The capacitor is completely discharged. The current is maximum and is again equal, but this time it has a different direction (Fig. 7).

Rice. 7.
Analogy. The pendulum again passes the equilibrium position with maximum speed, but this time in the opposite direction.
fourth quarter: . The current decreases, the capacitor is charged ( fig. 8).

Rice. eight.
Analogy. The pendulum continues to move to the right - from the equilibrium position to the leftmost point.
End of the fourth quarter and the entire period: . The reverse charge of the capacitor is completed, the current is zero (Fig. 9).

Rice. nine.
This moment is identical to the moment , and this picture is the picture 1 . There was one complete wobble. Now the next oscillation will begin, during which the processes will occur in exactly the same way as described above.
Analogy. The pendulum returned to its original position.
The considered electromagnetic oscillations are undamped- they will continue indefinitely. After all, we assumed that the resistance of the coil is zero!
In the same way, the oscillations of a spring pendulum will be undamped in the absence of friction.
In reality, the coil has some resistance. Therefore, oscillations in a real oscillatory circuit will be damped. So, after one complete oscillation, the charge on the capacitor will be less than the initial value. Over time, the oscillations will completely disappear: all the energy initially stored in the circuit will be released in the form of heat at the resistance of the coil and connecting wires.
In the same way, the vibrations of a real spring pendulum will be damped: all the energy of the pendulum will gradually turn into heat due to the inevitable presence of friction.
Energy transformations in an oscillatory circuit
We continue to consider undamped oscillations in the circuit, assuming the resistance of the coil to be zero. The capacitor has a capacitance, the inductance of the coil is equal to.
Since there is no heat loss, the energy does not leave the circuit: it is constantly redistributed between the capacitor and the coil.
Let's take the moment of time when the charge of the capacitor is maximum and equal to , and there is no current. The energy of the magnetic field of the coil at this moment is zero. All the energy of the circuit is concentrated in the capacitor:
Now, on the contrary, consider the moment when the current is maximum and equal to, and the capacitor is discharged. The energy of the capacitor is zero. All the energy of the circuit is stored in the coil:
At an arbitrary moment in time, when the charge of the capacitor is equal and current flows through the coil, the energy of the circuit is equal to:
Thus,
(1)
Relation (1) is used in solving many problems.
Electromechanical analogies
In the previous leaflet about self-induction, we noted the analogy between inductance and mass. Now we can establish a few more correspondences between electrodynamic and mechanical quantities.
For a spring pendulum we have a relation similar to (1) :
(2)
Here, as you already understood, is the stiffness of the spring, is the mass of the pendulum, and are the current values of the coordinate and velocity of the pendulum, and are their maximum values.
Comparing equalities (1) and (2) with each other, we see the following correspondences:
(3)
(4)
(5)
(6)
Based on these electromechanical analogies, we can foresee a formula for the period of electromagnetic oscillations in an oscillatory circuit.
Indeed, the period of oscillation of a spring pendulum, as we know, is equal to:
In accordance with analogies (5) and (6), we replace here the mass with inductance, and the stiffness with reverse capacitance. We get:
(7)
Electromechanical analogies do not fail: formula (7) gives the correct expression for the oscillation period in the oscillatory circuit. It is called Thomson's formula. We will present its more rigorous derivation shortly.
Harmonic law of oscillations in the circuit
Recall that oscillations are called harmonic, if the fluctuating value changes with time according to the law of sine or cosine. If you managed to forget these things, be sure to repeat the sheet “Mechanical vibrations”.
The oscillations of the charge on the capacitor and the current strength in the circuit turn out to be harmonic. We will prove it now. But first we need to establish the rules for choosing the sign for the charge of the capacitor and for the current strength - after all, during fluctuations, these quantities will take on both positive and negative values.
First we choose positive bypass direction contour. The choice does not play a role; let that be the direction counterclock-wise(Fig. 10).

Rice. 10. Positive bypass direction
The current strength is considered positive class="tex" alt="(!LANG:(I > 0)"> , если ток течёт в положительном направлении. В противном случае сила тока будет отрицательной .!}
The charge of a capacitor is the charge of that plate to which a positive current flows (i.e., the plate indicated by the bypass direction arrow). In this case, charge left capacitor plates.
With such a choice of signs of current and charge, the relation is true: (with a different choice of signs, it could happen). Indeed, the signs of both parts are the same: if class="tex" alt="(!LANG:I > 0"> , то заряд левой пластины возрастает, и потому !} class="tex" alt="(!LANG:\dot(q) > 0"> !}.
The values and change with time, but the energy of the circuit remains unchanged:
(8)
Therefore, the time derivative of energy vanishes: . We take the time derivative of both parts of the relation (8) ; do not forget that complex functions are differentiated on the left (If is a function of , then according to the differentiation rule complex function the derivative of the square of our function will be equal to: ):
Substituting here and , we get:
But the strength of the current is not a function identically equal to zero; That's why
Let's rewrite this as:
(9)
We got differential equation harmonic oscillations of the form , where . This proves that the charge of a capacitor oscillates according to a harmonic law (i.e., according to the law of sine or cosine). The cyclic frequency of these oscillations is equal to:
(10)
This value is also called natural frequency contour; it is with this frequency that free (or, as they say, own fluctuations). The oscillation period is:
We again came to the Thomson formula.
Harmonic dependence of charge on time in general case looks like:
(11)
The cyclic frequency is found by the formula (10) ; amplitude and initial phase are determined from the initial conditions.
We will consider the situation discussed in detail at the beginning of this leaflet. Let the charge of the capacitor be maximum and equal to (as in Fig. 1); there is no current in the loop. Then the initial phase is , so that the charge varies according to the cosine law with amplitude :
(12)
Let's find the law of change of current strength. To do this, we differentiate relation (12) with respect to time, again not forgetting the rule for finding the derivative of a complex function:
We see that the current strength also changes according to the harmonic law, this time according to the sine law:
(13)
The amplitude of the current strength is:
The presence of a "minus" in the law of current change (13) is not difficult to understand. Let's take, for example, the time interval (Fig. 2).
Current flows in the negative direction: . Since , the oscillation phase is in the first quarter: . The sine in the first quarter is positive; therefore, the sine in (13) will be positive in the considered time interval. Therefore, to ensure the negativity of the current, the minus sign in formula (13) is really necessary.
Now look at fig. eight . The current flows in the positive direction. How does our "minus" work in this case? Find out what's going on here!
Let's depict the graphs of charge and current fluctuations, i.e. graphs of functions (12) and (13) . For clarity, we present these graphs in the same coordinate axes (Fig. 11).

Rice. 11. Graphs of fluctuations in charge and current
Note that charge zeros occur at current highs or lows; conversely, current zeros correspond to charge maxima or minima.
Using the cast formula
we write the law of current change (13) in the form:
Comparing this expression with the law of charge change, we see that the phase of the current, equal to , is greater than the phase of the charge by . In this case, the current is said to leading in phase charge on ; or phase shift between current and charge is equal to; or phase difference between current and charge is equal to .
Leading the charge current in phase on graphically manifests itself in the fact that the current graph is shifted to the left on relative to the charge graph. The current strength reaches, for example, its maximum a quarter of the period earlier than the charge reaches its maximum (and a quarter of the period just corresponds to the phase difference).
Forced electromagnetic oscillations
As you remember, forced vibrations occur in the system under the action of a periodic driving force. The frequency of forced oscillations coincides with the frequency of the driving force.
Forced electromagnetic oscillations will be performed in a circuit connected to a sinusoidal voltage source (Fig. 12).

Rice. 12. Forced vibrations
If the source voltage changes according to the law:
then charge and current fluctuate in the circuit with a cyclic frequency (and with a period, respectively, ). The alternating voltage source, as it were, “imposes” its oscillation frequency to the circuit, forcing you to forget about natural frequency.
The amplitude of the forced oscillations of the charge and current depends on the frequency: the amplitude is greater, the closer to the natural frequency of the circuit. resonance- a sharp increase in the amplitude of oscillations. We will talk about resonance in more detail in the next leaflet on AC.