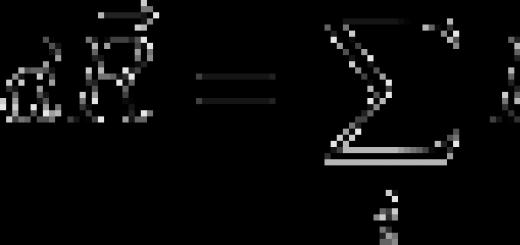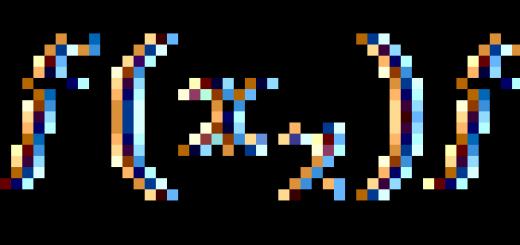Fundamentals of Maxwell's theory for electromagnetic field

Vortex electric field

From Faraday's Law ξ=dФ/dt follows that any a change in the flux of magnetic induction coupled to the circuit leads to the emergence of an electromotive force of induction and, as a result, an induction current appears. Therefore, the occurrence of emf. electromagnetic induction is also possible in a fixed circuit located in an alternating magnetic field. However, emf. in any circuit occurs only when external forces act on current carriers in it - forces of non-electrostatic origin (see § 97). Therefore, the question arises about the nature of extraneous forces in this case.

Experience shows that these extraneous forces are not associated with either thermal or chemical processes in the circuit; their occurrence also cannot be explained by Lorentz forces, since they do not act on immovable charges. Maxwell hypothesized that any alternating magnetic field excites an electric field in the surrounding space, which

and is the cause of the induction current in the circuit. According to Maxwell's ideas, the circuit in which the emf appears plays a secondary role, being a kind of only "device" that detects this field.

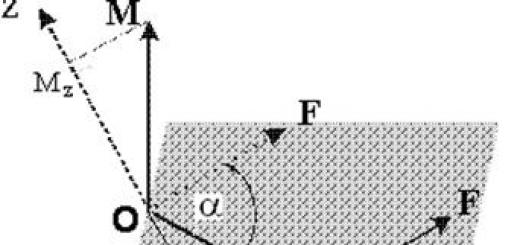
first equation Maxwell argues that changes in the electric field generate a vortex magnetic field.

Second equation Maxwell expresses the law electromagnetic induction Faraday: The EMF in any closed loop is equal to the rate of change (i.e. time derivative) magnetic flux. But the EMF is equal to the tangential component of the electric field strength vector E, multiplied by the length of the circuit. To go to the rotor, as in the first Maxwell equation, it is enough to divide the EMF by the area of ​​\u200b\u200bthe contour, and let the latter go to zero, i.e., take a small contour covering the considered point in space (Fig. 9, c). Then on the right side of the equation there will no longer be a flux, but a magnetic induction, since the flux is equal to the induction multiplied by the area of ​​\u200b\u200bthe circuit.
So, we get: rotE = - dB/dt.
Thus, the vortex electric field is generated by changes in the magnetic field, which is shown in Fig. 9c and is represented by the formula just given.
Third and fourth equations Maxwell deal with charges and the fields generated by them. They are based on the Gauss theorem, which states that the flux of the electric induction vector through any closed surface is equal to the charge inside this surface.

A whole science is based on Maxwell's equations - electrodynamics, which allows solving many useful practical problems using strict mathematical methods. It is possible to calculate, for example, the radiation field of various antennas both in free space and near the surface of the Earth or near the body of some aircraft such as aircraft or rockets. Electrodynamics allows you to calculate the design of waveguides and cavity resonators - devices used at very high frequencies of the centimeter and millimeter wave ranges, where conventional transmission lines and oscillatory circuits are no longer suitable. Without electrodynamics, it would be impossible to develop radar, space radio communications, antenna technology, and many other branches of modern radio engineering.

Bias current

SHIFT CURRENT, a quantity proportional to the rate of change of an alternating electric field in a dielectric or vacuum. The name "current" is due to the fact that the displacement current, like the conduction current, generates a magnetic field.

When constructing the theory of the electromagnetic field, J.K. Maxwell put forward a hypothesis (subsequently confirmed by experiment) that the magnetic field is created not only by the movement of charges (conduction current, or simply current), but also by any change in time of the electric field.

The concept of displacement current was introduced by Maxwell to establish quantitative relationships between the changing electric field and the resulting magnetic field.

According to Maxwell's theory, in an alternating current circuit containing a capacitor, an alternating electric field in the capacitor at each moment of time creates such a magnetic field as the current (called the displacement current) would create if it flowed between the plates of the capacitor. From this definition it follows that J cm = J(i.e., numerical values the conduction current density and the displacement current density are equal), and, therefore, the conduction current density lines inside the conductor continuously change into the displacement current density lines between the capacitor plates. Bias current density j cm characterizes the rate of change of electrical induction D in time:

J cm = + ?D/?t.

The bias current does not generate Joule heat, its main physical property- the ability to create a magnetic field in the surrounding space.

The vortex magnetic field is created by the total current, the density of which is j, is equal to the sum of the conduction current density and the bias current?D/?t. That is why for the value? D /? t the name current was introduced.

Harmonic oscillator called a system that oscillates, described by an expression of the form d 2 s / dt 2 + ω 0 2 s \u003d 0 or

where the two dots above mean twofold differentiation with respect to time. The oscillations of the harmonic oscillator are important example periodic motion and serve as an exact or approximate model in many problems of classical and quantum physics. Examples of a harmonic oscillator are spring, physical, and mathematical pendulum and, an oscillatory circuit (for currents and voltages so small that the elements of the circuit could be considered linear).

Harmonic vibrations

Along with progressive and rotational movements bodies in mechanics are of considerable interest and oscillatory movements. Mechanical vibrations are called movements of bodies that repeat exactly (or approximately) at regular intervals. The law of motion of an oscillating body is given by some periodic function of time x = f (t). Graphic image This function gives a visual representation of the course of the oscillatory process in time.

Examples of simple oscillatory systems are a load on a spring or a mathematical pendulum (Fig. 2.1.1).

Mechanical oscillations, like oscillatory processes of any other physical nature, can be free and forced. Free vibrations are made under the influence internal forces system after the system has been brought out of equilibrium. The oscillations of a weight on a spring or the oscillations of a pendulum are free oscillations. vibrations under the action external periodically changing forces are called forced The simplest type of oscillatory process are simple harmonic vibrations , which are described by the equation

Oscillation frequency f shows how many vibrations are made in 1 s. Frequency unit - hertz(Hz). Oscillation frequency f is related to the cyclic frequency ω and the oscillation period T ratios:

gives the dependence of the fluctuating quantity S from time t; this is the equation of free harmonic oscillations in explicit form. However, the equation of oscillations is usually understood as a different record of this equation, in differential form. For definiteness, we take equation (1) in the form

Differentiate it twice with respect to time:

It can be seen that the following relation holds:

which is called the equation of free harmonic oscillations (in differential form). Equation (1) is a solution to differential equation (2). Since equation (2) - differential equation second order, two initial conditions are needed to obtain complete solution(that is, the definitions of the constants included in equation (1) A and j0); for example, the position and speed of an oscillatory system at t = 0.

Addition of harmonic oscillations of the same direction and the same frequency. beats

Let two harmonic oscillations of the same direction and the same frequency take place

The equation of the resulting oscillation will have the form

We verify this by adding the equations of system (4.1)

Applying the sum cosine theorem and making algebraic transformations:

One can find such quantities A and φ0 that satisfy the equations

Considering (4.3) as two equations with two unknowns A and φ0, we find by squaring and adding them, and then dividing the second by the first:

Substituting (4.3) into (4.2), we get:

Or finally, using the sum cosine theorem, we have:

The body, participating in two harmonic oscillations of the same direction and the same frequency, also performs a harmonic oscillation in the same direction and with the same frequency as the summed oscillations. The amplitude of the resulting oscillation depends on the phase difference (φ2-φ1) of the smoothed oscillations.

Depending on the phase difference (φ2-φ1):

1) (φ2-φ1) = ±2mπ (m=0, 1, 2, ...), then A= A1+A2, i.e. the amplitude of the resulting oscillation A is equal to the sum of the amplitudes of the added oscillations;

2) (φ2-φ1) = ±(2m+1)π (m=0, 1, 2, ...), then A= |A1-A2|, i.e. the amplitude of the resulting oscillation is equal to the difference in the amplitudes of the added oscillations

Periodic changes in the amplitude of oscillations that occur when two harmonic oscillations with close frequencies are added are called beats.

Let two oscillations differ little in frequency. Then the amplitudes of the added oscillations are equal to A, and the frequencies are equal to ω and ω + Δω, and Δω is much less than ω. We choose the reference point so that the initial phases of both oscillations are equal to zero:

Let's solve the system

System solution:

The resulting oscillation can be considered as harmonic with frequency ω, amplitude A, which varies according to the following periodic law:

The frequency of change of A is twice the frequency of change of the cosine. The beat frequency is equal to the difference between the frequencies of the added oscillations: ωb = Δω

Beat period:

Determination of the tone frequency (the sound of a certain beat height by the reference and measured vibrations is the most widely used method for comparing the measured value with the reference. The beat method is used to tune musical instruments, analyze hearing, etc.

Similar information.

Harmonic oscillation is a phenomenon of periodic change of some quantity, in which the dependence on the argument has the character of a sine or cosine function. For example, a quantity that varies in time as follows harmonically fluctuates:

where x is the value of the changing quantity, t is time, the remaining parameters are constant: A is the amplitude of oscillations, ω is the cyclic frequency of oscillations, - complete phase fluctuations, - initial phase fluctuations.

Generalized harmonic oscillation in differential form

(Any non-trivial solution of this differential equation is a harmonic oscillation with a cyclic frequency)

Types of vibrations

Free oscillations are performed under the action of the internal forces of the system after the system has been taken out of equilibrium. For free oscillations to be harmonic, it is necessary that the oscillatory system be linear (described by linear equations of motion), and there should be no energy dissipation in it (the latter would cause damping).

Forced oscillations are performed under the influence of an external periodic force. For them to be harmonic, it is sufficient that the oscillatory system be linear (described by linear equations of motion), and the external force itself changes over time as a harmonic oscillation (that is, that the time dependence of this force is sinusoidal).

Harmonic vibration equation

Equation (1)

gives the dependence of the fluctuating value S on time t; this is the equation of free harmonic oscillations in explicit form. However, the equation of oscillations is usually understood as a different record of this equation, in differential form. For definiteness, we take equation (1) in the form

Differentiate it twice with respect to time:

It can be seen that the following relation holds:

which is called the equation of free harmonic oscillations (in differential form). Equation (1) is a solution to differential equation (2). Since equation (2) is a second-order differential equation, two initial conditions are necessary to obtain a complete solution (that is, to determine the constants A and   included in equation (1); for example, the position and speed of an oscillatory system at t = 0.

A mathematical pendulum is an oscillator, which is a mechanical system consisting of a material point located on a weightless inextensible thread or on a weightless rod in a uniform field of gravitational forces. The period of small eigenoscillations of a mathematical pendulum of length l, motionlessly suspended in a uniform gravitational field with free fall acceleration g, is equal to

and does not depend on the amplitude and mass of the pendulum.

A physical pendulum is an oscillator, which is a rigid body that oscillates in the field of any forces about a point that is not the center of mass of this body, or a fixed axis perpendicular to the direction of the forces and not passing through the center of mass of this body.

Have mathematical expression. Their properties are characterized by a set of trigonometric equations, the complexity of which is determined by the complexity of the oscillatory process itself, the properties of the system and the environment in which they occur, i.e., external factors affecting the oscillatory process.

For example, in mechanics, a harmonic oscillation is a movement that is characterized by:

Rectilinear character;

unevenness;

The movement of a physical body that occurs along a sinusoidal or cosine trajectory, and depends on time.

Based on these properties, we can bring the equation of harmonic oscillations, which has the form:

x \u003d A cos ωt or the form x \u003d A sin ωt, where x is the value of the coordinate, A is the value of the oscillation amplitude, ω is the coefficient.

Such an equation of harmonic oscillations is the main one for all harmonic oscillations that are considered in kinematics and mechanics.

The indicator ωt, which in this formula is under the sign trigonometric function, is called the phase, and it determines the location of the oscillating material point at a given moment in time at a given amplitude. When considering cyclical fluctuations, this indicator is equal to 2l, it shows the amount within the time cycle and is denoted by w. In this case, the equation of harmonic oscillations contains it as an indicator of the magnitude of the cyclic (circular) frequency.

The equation of harmonic oscillations we are considering, as already noted, can take various forms, depending on a number of factors. For example, here is an option. To consider free harmonic oscillations, one should take into account the fact that they are all characterized by damping. This phenomenon manifests itself in different ways: the stopping of a moving body, the cessation of radiation in electrical systems. The simplest example showing a decrease in the vibrational potential is its conversion into thermal energy.

The equation under consideration is: d²s / dt² + 2β x ds / dt + ω²s \u003d 0. In this formula: s is the value of the oscillating quantity that characterizes the properties of a particular system, β is a constant showing the attenuation coefficient, ω is the cyclic frequency.

The use of such a formula allows one to approach the description oscillatory processes in linear systems from a unified point of view, as well as to design and simulate oscillatory processes at the scientific and experimental level.

For example, it is known that final stage their manifestations already cease to be harmonic, that is, the categories of frequency and period for them become simply meaningless and are not reflected in the formula.

The classic way to study harmonic oscillations is In its simplest form, it represents a system that is described by such a differential equation of harmonic oscillations: ds / dt + ω²s = 0. But the variety of oscillatory processes naturally leads to the fact that there is a large number of oscillators. We list their main types:

A spring oscillator is an ordinary load with a certain mass m, which is suspended on an elastic spring. It performs harmonic type, which are described by the formula f = - kx.

Physical oscillator (pendulum) - a rigid body that oscillates around a static axis under the influence of a certain force;

- (almost never occurs in nature). It is an ideal model of a system that includes an oscillating physical body with a certain mass, which is suspended on a rigid weightless thread.

fluctuations called such processes in which the system repeatedly passes through the equilibrium position with a greater or lesser frequency.

Oscillation classification:

a) by nature (mechanical, electromagnetic, fluctuations in concentration, temperature, etc.);

b) in form (simple = harmonic; complex, which are the sum of simple harmonic vibrations);

in) according to the degree of periodicity = periodic (characteristics of the system are repeated after a strictly defined period of time (period)) and aperiodic;

G) in relation to time (undamped = constant amplitude; damped = decreasing amplitude);

G) energy – free (single input of energy into the system from outside = single external impact); forced (multiple (periodic) supply of energy to the system from the outside = periodic external influence); self-oscillations (undamped oscillations arising due to the system's ability to regulate the flow of energy from a constant source).

Conditions for the occurrence of oscillations.

a) The presence of an oscillatory system (a pendulum on a suspension, a spring pendulum, an oscillatory circuit, etc.);

b) The presence of an external source of energy that is able to bring the system out of equilibrium at least once;

c) The emergence of a quasi-elastic restoring force in the system (i.e., a force proportional to the displacement);

d) Presence of inertia (inertial element) in the system.

As an illustrative example, consider the movement of a mathematical pendulum. Mathematical pendulum called a body of small size, suspended on a thin inextensible thread, the mass of which is negligible compared to the mass of the body. In the equilibrium position, when the pendulum hangs on a plumb line, the force of gravity is balanced by the force of the thread tension
. When the pendulum deviates from the equilibrium position by a certain angle α there is a tangential component of gravity F=- mg sinα. The minus sign in this formula means that the tangential component is directed in the direction opposite to the pendulum deflection. She is a restoring force. At small angles α (of the order of 15-20 o), this force is proportional to the displacement of the pendulum, i.e. is quasi-elastic, and the oscillations of the pendulum are harmonic.

When the pendulum is deflected, it rises to a certain height, i.e. he is given a certain amount of potential energy ( E sweat = mgh). When the pendulum moves to the equilibrium position, the transition of potential energy into kinetic energy occurs. At the moment when the pendulum passes the equilibrium position, the potential energy is equal to zero, and the kinetic energy is maximum. Due to the presence of mass m(weight - physical quantity, which determines the inertial and gravitational properties of matter), the pendulum passes the equilibrium position and deviates in the opposite direction. In the absence of friction in the system, the pendulum will continue to oscillate indefinitely.

The harmonic oscillation equation has the form:

x(t) = x m cos(ω 0 t +φ 0 ),

where X- displacement of the body from the equilibrium position;

x m (BUT) is the oscillation amplitude, that is, the maximum displacement modulus,

ω 0 - cyclic (or circular) frequency of oscillations,

t- time.

The value under the cosine sign φ = ω 0 t + φ 0 called phase harmonic vibration. Phase determines the offset at a given time t. The phase is expressed in angular units (radians).

At t= 0 φ = φ 0 , That's why φ 0 called initial phase.

The period of time after which certain states of the oscillatory system are repeated is called period of oscillation T.

The physical quantity reciprocal to the period of oscillation is called oscillation frequency:
. Oscillation frequency ν shows how many oscillations are made per unit of time. Frequency unit - hertz (Hz) - unicycle per second.

Oscillation frequency ν related to cyclic frequency ω and oscillation period T ratios:
.

That is, the circular frequency is the number of complete oscillations that occur in 2π units of time.

Graphically, harmonic oscillations can be represented as a dependence X from t and the method of vector diagrams.

The method of vector diagrams allows you to visualize all the parameters included in the equation of harmonic oscillations. Indeed, if the amplitude vector BUT placed at an angle φ to the axis X, then its projection onto the axis X will be equal to: x = Acos(φ ) . Injection φ and there is an initial phase. If the vector BUT put into rotation with angular velocityω 0 , equal to the circular frequency of oscillations, then the projection of the end of the vector will move along the axis X and take values ​​ranging from -A before +A, and the coordinate of this projection will change over time according to the law: x(t) = BUTcos(ω 0 t+ φ) . The time it takes for the amplitude vector to make one complete revolution is equal to the period T harmonic vibrations. The number of revolutions of the vector per second is equal to the oscillation frequency ν .

Changes in time according to a sinusoidal law:

where X- the value of the fluctuating quantity at the moment of time t, BUT- amplitude , ω - circular frequency, φ is the initial phase of oscillations, ( φt + φ ) is the total phase of oscillations . At the same time, the values BUT, ω and φ - permanent.

For mechanical vibrations fluctuating value X are, in particular, displacement and speed, for electrical oscillations - voltage and current strength.

Harmonic vibrations occupy a special place among all types of vibrations, since this is the only type of vibration whose shape is not distorted when passing through any homogeneous medium, i.e., waves propagating from a source of harmonic vibrations will also be harmonic. Any non-harmonic vibration can be represented as a sum (integral) of various harmonic vibrations (in the form of a spectrum of harmonic vibrations).

Energy transformations during harmonic vibrations.

In the process of oscillations, there is a transition of potential energy Wp into kinetic Wk and vice versa. In the position of maximum deviation from the equilibrium position, the potential energy is maximum, the kinetic energy is zero. As we return to the equilibrium position, the speed of the oscillating body increases, and with it the kinetic energy also increases, reaching a maximum in the equilibrium position. The potential energy then drops to zero. Further-neck movement occurs with a decrease in speed, which drops to zero when the deflection reaches its second maximum. Potential energy here increases to its initial (maximum) value (in the absence of friction). Thus, the oscillations of the kinetic and potential energies occur with a double (compared to the oscillations of the pendulum itself) frequency and are in antiphase (i.e., there is a phase shift between them equal to π ). Total vibration energy W remains unchanged. For a body oscillating under the action of an elastic force, it is equal to:

where v m — maximum speed body (in equilibrium position), x m = BUT- amplitude.

Due to the presence of friction and resistance of the medium, free oscillations damp out: their energy and amplitude decrease with time. Therefore, in practice, not free, but forced oscillations are used more often.