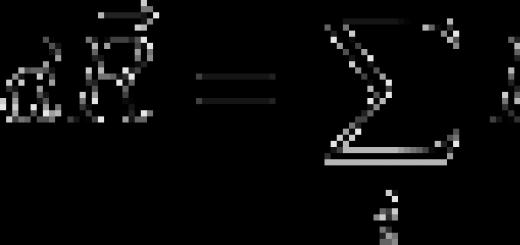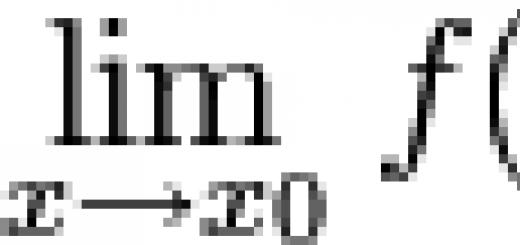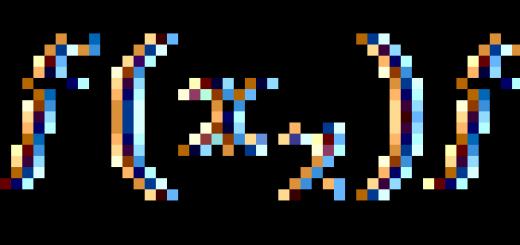Antiderivative function and indefinite integral

Fact 1. Integration is the opposite of differentiation, namely, the restoration of a function from the known derivative of this function. The function restored in this way F(x) is called primitive for function f(x).

Definition 1. Function F(x f(x) on some interval X, if for all values x from this interval the equality F "(x)=f(x), that is, this function f(x) is the derivative of antiderivative function F(x). .

For example, the function F(x) = sin x is the antiderivative for the function f(x) = cos x on the entire number line, since for any value of x (sin x)" = (cos x) .

Definition 2. Indefinite integral of a function f(x) is the collection of all its antiderivatives. This uses the notation

∫

f(x)dx

,

where is the sign ∫ is called the integral sign, the function f(x) is an integrand, and f(x)dx is the integrand.

Thus, if F(x) is some antiderivative for f(x) , then

∫

f(x)dx = F(x) +C

where C - arbitrary constant (constant).

To understand the meaning of the set of antiderivatives of a function as an indefinite integral, the following analogy is appropriate. Let there be a door (a traditional wooden door). Its function is "to be a door". What is the door made of? From a tree. This means that the set of antiderivatives of the integrand "to be a door", that is, its indefinite integral, is the function "to be a tree + C", where C is a constant, which in this context can denote, for example, a tree species. Just as a door is made of wood with some tools, the derivative of a function is "made" of the antiderivative function with formula that we learned by studying the derivative .

Then the table of functions of common objects and their corresponding primitives ("to be a door" - "to be a tree", "to be a spoon" - "to be a metal", etc.) is similar to the table of basic indefinite integrals, which will be given below. The table of indefinite integrals lists common functions, indicating the antiderivatives from which these functions are "made". As part of the tasks for finding the indefinite integral, such integrands are given that can be integrated directly without special efforts, that is, according to the table of indefinite integrals. In more complex problems, the integrand must first be transformed so that tabular integrals can be used.

Fact 2. Restoring a function as an antiderivative, we must take into account an arbitrary constant (constant) C, and in order not to write a list of antiderivatives with various constants from 1 to infinity, you need to write down a set of antiderivatives with an arbitrary constant C, like this: 5 x³+C. So, an arbitrary constant (constant) is included in the expression of the antiderivative, since the antiderivative can be a function, for example, 5 x³+4 or 5 x³+3 and when differentiating 4 or 3 or any other constant vanishes.

We set the integration problem: for a given function f(x) find such a function F(x), whose derivative is equal to f(x).

Example 1 Find the set of antiderivatives of a function

Decision. For this function, the antiderivative is the function

Function F(x) is called antiderivative for the function f(x) if the derivative F(x) is equal to f(x), or, which is the same thing, the differential F(x) is equal to f(x) dx, i.e.

(2)

Therefore, the function is antiderivative for the function . However, it is not the only antiderivative for . They are also functions

where With is an arbitrary constant. This can be verified by differentiation.

Thus, if there is one antiderivative for a function, then for it there is infinite set antiderivatives that differ by a constant term. All antiderivatives for a function are written in the above form. This follows from the following theorem.

Theorem (formal statement of fact 2). If a F(x) is the antiderivative for the function f(x) on some interval X, then any other antiderivative for f(x) on the same interval can be represented as F(x) + C, where With is an arbitrary constant.

In the following example, we already turn to the table of integrals, which will be given in paragraph 3, after the properties of the indefinite integral. We do this before familiarizing ourselves with the entire table, so that the essence of the above is clear. And after the table and properties, we will use them in their entirety when integrating.

Example 2 Find sets of antiderivatives:

Decision. We find sets of antiderivative functions from which these functions are "made". When mentioning formulas from the table of integrals, for now, just accept that there are such formulas, and we will study the table of indefinite integrals in full a little further.

1) Applying formula (7) from the table of integrals for n= 3, we get

2) Using formula (10) from the table of integrals for n= 1/3, we have

3) Since

then according to formula (7) at n= -1/4 find

Under the integral sign, they do not write the function itself f, and its product by the differential dx. This is done primarily to indicate which variable the antiderivative is being searched for. For example,

, ;

here in both cases the integrand is equal to , but its indefinite integrals in the considered cases turn out to be different. In the first case, this function is considered as a function of a variable x, and in the second - as a function of z .

The process of finding the indefinite integral of a function is called integrating that function.

The geometric meaning of the indefinite integral

Let it be required to find a curve y=F(x) and we already know that the tangent of the slope of the tangent at each of its points is a given function f(x) abscissa of this point.

According to geometric sense derivative, tangent of the slope of the tangent at a given point on the curve y=F(x) equal to the value of the derivative F"(x). So, we need to find such a function F(x), for which F"(x)=f(x). Required function in the task F(x) is derived from f(x). The condition of the problem is satisfied not by one curve, but by a family of curves. y=F(x)- one of these curves, and any other curve can be obtained from it by parallel translation along the axis Oy.

Let's call the graph of the antiderivative function of f(x) integral curve. If a F"(x)=f(x), then the graph of the function y=F(x) is an integral curve.

Fact 3. The indefinite integral is geometrically represented by the family of all integral curves as in the picture below. The distance of each curve from the origin is determined by an arbitrary constant (constant) of integration C.

Properties of the indefinite integral

Fact 4. Theorem 1. The derivative of an indefinite integral is equal to the integrand, and its differential is equal to the integrand.

Fact 5. Theorem 2. The indefinite integral of the differential of a function f(x) is equal to the function f(x) up to a constant term , i.e.

(3)

Theorems 1 and 2 show that differentiation and integration are mutually inverse operations.

Fact 6. Theorem 3. The constant factor in the integrand can be taken out of the sign of the indefinite integral , i.e.

Principal Integrals Every Student Should Know

The listed integrals are the basis, the basis of the foundations. These formulas, of course, should be remembered. When calculating more complex integrals, you will have to use them constantly.

Pay special attention to formulas (5), (7), (9), (12), (13), (17) and (19). Do not forget to add an arbitrary constant C to the answer when integrating!

Integral of a constant

∫ A d x = A x + C (1)

Power function integration

In fact, one could confine oneself to formulas (5) and (7), but the rest of the integrals from this group are so common that it is worth paying a little attention to them.

∫ x d x = x 2 2 + C (2)
∫ x 2 d x = x 3 3 + C (3)
∫ 1 x d x = 2 x + C (4)
∫ 1 x d x = log | x | +C(5)
∫ 1 x 2 d x = − 1 x + C (6)
∫ x n d x = x n + 1 n + 1 + C (n ≠ − 1) (7)

Integrals of the exponential function and of hyperbolic functions

Of course, formula (8) (perhaps the most convenient to remember) can be considered as special case formulas (9). Formulas (10) and (11) for the integrals of the hyperbolic sine and hyperbolic cosine are easily derived from formula (8), but it is better to just remember these relationships.

∫ e x d x = e x + C (8)
∫ a x d x = a x log a + C (a > 0, a ≠ 1) (9)
∫ s h x d x = c h x + C (10)
∫ c h x d x = s h x + C (11)

Basic integrals of trigonometric functions

A mistake that students often make: they confuse the signs in formulas (12) and (13). Remembering that the derivative of the sine is equal to the cosine, for some reason many people believe that the integral of sinx functions equal to cosx. This is not true! The integral of sine is "minus cosine", but the integral of cosx is "just sine":

∫ sin x d x = − cos x + C (12)
∫ cos x d x = sin x + C (13)
∫ 1 cos 2 x d x = t g x + C (14)
∫ 1 sin 2 x d x = − c t g x + C (15)

Integrals Reducing to Inverse Trigonometric Functions

Formula (16), which leads to the arc tangent, is naturally a special case of formula (17) for a=1. Similarly, (18) is a special case of (19).

∫ 1 1 + x 2 d x = a r c t g x + C = − a r c c t g x + C (16)
∫ 1 x 2 + a 2 = 1 a a r c t g x a + C (a ≠ 0) (17)
∫ 1 1 − x 2 d x = arcsin x + C = − arccos x + C (18)
∫ 1 a 2 − x 2 d x = arcsin x a + C = − arccos x a + C (a > 0) (19)

More complex integrals

These formulas are also desirable to remember. They are also used quite often, and their output is rather tedious.

∫ 1 x 2 + a 2 d x = ln | x + x2 + a2 | +C(20)
∫ 1 x 2 − a 2 d x = ln | x + x 2 − a 2 | +C(21)
∫ a 2 − x 2 d x = x 2 a 2 − x 2 + a 2 2 arcsin x a + C (a > 0) (22)
∫ x 2 + a 2 d x = x 2 x 2 + a 2 + a 2 2 ln | x + x2 + a2 | + C (a > 0) (23)
∫ x 2 − a 2 d x = x 2 x 2 − a 2 − a 2 2 ln | x + x 2 − a 2 | + C (a > 0) (24)

General integration rules

1) Integral of the sum of two functions is equal to the sum corresponding integrals: ∫ (f (x) + g (x)) d x = ∫ f (x) d x + ∫ g (x) d x (25)

2) The integral of the difference of two functions is equal to the difference of the corresponding integrals: ∫ (f (x) − g (x)) d x = ∫ f (x) d x − ∫ g (x) d x (26)

3) The constant can be taken out of the integral sign: ∫ C f (x) d x = C ∫ f (x) d x (27)

It is easy to see that property (26) is simply a combination of properties (25) and (27).

4) Integral of complex function, if the inner function is linear: ∫ f (A x + B) d x = 1 A F (A x + B) + C (A ≠ 0) (28)

Here F(x) is the antiderivative for the function f(x). Note that this formula only works when the inner function is Ax + B.

Important: there is no universal formula for the integral of the product of two functions, as well as for the integral of a fraction:

∫ f (x) g (x) d x = ? ∫ f (x) g (x) d x = ? (thirty)

This does not mean, of course, that a fraction or a product cannot be integrated. It's just that every time you see an integral like (30), you have to invent a way to "fight" with it. In some cases, integration by parts will help you, somewhere you will have to make a change of variable, and sometimes even "school" formulas of algebra or trigonometry can help.

A simple example for calculating the indefinite integral

Example 1. Find the integral: ∫ (3 x 2 + 2 sin x − 7 e x + 12) d x

We use formulas (25) and (26) (the integral of the sum or difference of functions is equal to the sum or difference of the corresponding integrals. We get: ∫ 3 x 2 d x + ∫ 2 sin x d x − ∫ 7 e x d x + ∫ 12 d x

Recall that the constant can be taken out of the integral sign (formula (27)). The expression is converted to the form

3 ∫ x 2 d x + 2 ∫ sin x d x − 7 ∫ e ​​x d x + 12 ∫ 1 d x

Now let's just use the table of basic integrals. We will need to apply formulas (3), (12), (8) and (1). Let's integrate the power function, sine, exponent and constant 1. Don't forget to add an arbitrary constant C at the end:

3 x 3 3 - 2 cos x - 7 e x + 12 x + C

After elementary transformations, we get the final answer:

X 3 − 2 cos x − 7 e x + 12 x + C

Test yourself with differentiation: take the derivative of the resulting function and make sure that it is equal to the original integrand.

Summary table of integrals

∫ A d x = A x + C

∫ x d x = x 2 2 + C

∫ x 2 d x = x 3 3 + C

∫ 1 x d x = 2 x + C

∫ 1 x d x = log | x | + C

∫ 1 x 2 d x = − 1 x + C

∫ x n d x = x n + 1 n + 1 + C (n ≠ − 1)

∫ e x d x = e x + C

∫ a x d x = a x ln a + C (a > 0, a ≠ 1)

∫ s h x d x = c h x + C

∫ c h x d x = s h x + C

∫ sin x d x = − cos x + C

∫ cos x d x = sin x + C

∫ 1 cos 2 x d x = t g x + C

∫ 1 sin 2 x d x = − c t g x + C

∫ 1 1 + x 2 d x = a r c t g x + C = − a r c c t g x + C

∫ 1 x 2 + a 2 = 1 a a r c t g x a + C (a ≠ 0)

∫ 1 1 − x 2 d x = arcsin x + C = − arccos x + C

∫ 1 a 2 − x 2 d x = arcsin x a + C = − arccos x a + C (a > 0)

∫ 1 x 2 + a 2 d x = ln | x + x2 + a2 | + C

∫ 1 x 2 − a 2 d x = ln | x + x 2 − a 2 | + C

∫ a 2 − x 2 d x = x 2 a 2 − x 2 + a 2 2 arcsin x a + C (a > 0)

∫ x 2 + a 2 d x = x 2 x 2 + a 2 + a 2 2 ln | x + x2 + a2 | + C (a > 0)

∫ x 2 − a 2 d x = x 2 x 2 − a 2 − a 2 2 ln | x + x 2 − a 2 | + C (a > 0)

Download the table of integrals (part II) from this link

If you study at a university, if you have any difficulties with higher mathematics (mathematical analysis, linear algebra, probability theory, statistics), if you need the services of a qualified teacher, go to the page of a tutor in higher mathematics. Let's solve your problems together!

You might also be interested

Table of antiderivatives ("integrals"). Table of integrals. Tabular indefinite integrals. (Simple integrals and integrals with a parameter). Formulas for integration by parts. Newton-Leibniz formula.

Table of antiderivatives ("integrals"). Tabular indefinite integrals. (Simple integrals and integrals with a parameter).
Integral power function.	Power function integral.
An integral that reduces to an integral of a power function if x is driven under the sign of the differential.
	The exponential integral, where a is a constant number.
Integral of a compound exponential function.	The integral of the exponential function.
An integral equal to the natural logarithm.	Integral: "Long logarithm".
	Integral: "Long logarithm".
Integral: "High logarithm".	The integral, where x in the numerator is brought under the sign of the differential (the constant under the sign can be both added and subtracted), as a result, is similar to the integral equal to the natural logarithm.
Integral: "High logarithm".
Cosine integral.	Sine integral.
An integral equal to the tangent.	An integral equal to the cotangent.
Integral equal to both arcsine and arcsine
An integral equal to both the inverse sine and the inverse cosine.	An integral equal to both the arc tangent and the arc cotangent.
The integral is equal to the cosecant.	Integral equal to secant.
An integral equal to the arcsecant.	An integral equal to the arc cosecant.
An integral equal to the arcsecant.	An integral equal to the arcsecant.
An integral equal to the hyperbolic sine.	An integral equal to the hyperbolic cosine.
An integral equal to the hyperbolic sine, where sinhx is the hyperbolic sine in English.	An integral equal to the hyperbolic cosine, where sinhx is the hyperbolic sine in the English version.
An integral equal to the hyperbolic tangent.	An integral equal to the hyperbolic cotangent.
An integral equal to the hyperbolic secant.	An integral equal to the hyperbolic cosecant.

Formulas for integration by parts. Integration rules.

Formulas for integration by parts. Newton-Leibniz formula. Integration rules.
Integration of a product (function) by a constant:
Integration of the sum of functions:
indefinite integrals:
Integration by parts formula definite integrals:
Newton-Leibniz formula definite integrals:	Where F(a),F(b) are the values ​​of the antiderivatives at the points b and a, respectively.

Derivative table. Table derivatives. Derivative of the product. Derivative of private. Derivative of a complex function.

If x is an independent variable, then:

Derivative table. Table derivatives. "table derivative" - ​​yes, unfortunately, that's how they are searched on the Internet
	Power function derivative
	Derivative of the exponent
Derivative of a compound exponential function	Derivative of exponential function
Derivative of a logarithmic function	Derivative natural logarithm
	Derivative of the natural logarithm of a function
Sine derivative	cosine derivative
Cosecant derivative	Secant derivative
Derivative of arcsine	Arc cosine derivative
Derivative of arcsine	Arc cosine derivative
Tangent derivative	Cotangent derivative
Arc tangent derivative	Derivative of inverse tangent
Arc tangent derivative	Derivative of inverse tangent
Arcsecant derivative	Derivative of arc cosecant
Arcsecant derivative	Derivative of arc cosecant
Derivative of the hyperbolic sine Derivative of the hyperbolic sine in the English version	Hyperbolic cosine derivative The derivative of the hyperbolic cosine in the English version
Derivative of the hyperbolic tangent	Derivative of the hyperbolic cotangent
Derivative of hyperbolic secant	Derivative of the hyperbolic cosecant

Differentiation rules. Derivative of the product. Derivative of private. Derivative of a complex function.
Derivative of a product (function) by a constant:
Derivative of the sum (functions):
Derivative of the product (of functions):
The derivative of the quotient (of functions):
Derivative of a complex function:

Properties of logarithms. Basic formulas of logarithms. Decimal (lg) and natural logarithms (ln).

Basic logarithmic identity
Let us show how any function of the form a b can be made exponential. Since a function of the form e x is called exponential, then
Any function of the form a b can be represented as a power of ten

Natural logarithm ln (logarithm base e = 2.718281828459045…) ln(e)=1; log(1)=0

Taylor series. Expansion of a function in a Taylor series.

It turns out that most practically encountered mathematical functions can be represented with any accuracy in the vicinity of a certain point in the form of power series containing the powers of the variable in ascending order. For example, in the vicinity of the point x=1:

When using rows called taylor rows, mixed functions containing, say, algebraic, trigonometric, and exponential functions can be expressed as purely algebraic functions. With the help of series, differentiation and integration can often be quickly carried out.

The Taylor series in the vicinity of the point a has the following forms:

1) , where f(x) is a function that has derivatives of all orders at x=a. R n - the remainder term in the Taylor series is determined by the expression

2)

k-th coefficient (at x k) of the series is determined by the formula

3) A special case of the Taylor series is the Maclaurin series (=McLaren) (the decomposition takes place around the point a=0)

for a=0

the members of the series are determined by the formula

Conditions for the application of Taylor series.

1. In order for the function f(x) to be expanded in a Taylor series on the interval (-R;R), it is necessary and sufficient that the remainder term in the Taylor formula (Maclaurin (=McLaren)) for this function tends to zero at k →∞ on the specified interval (-R;R).

2. It is necessary that there are derivatives for this function at the point in the vicinity of which we are going to build a Taylor series.

Properties of Taylor series.

If f is an analytic function, then its Taylor series at any point a of the domain of f converges to f in some neighborhood of a.

There are infinitely differentiable functions whose Taylor series converges but differs from the function in any neighborhood of a. For example:

Taylor series are used for approximation (an approximation is a scientific method that consists in replacing some objects with others, in one sense or another close to the original, but simpler) functions by polynomials. In particular, linearization ((from linearis - linear), one of the methods of approximate representation of closed nonlinear systems, in which the study of a nonlinear system is replaced by an analysis of a linear system, in a sense equivalent to the original one.) of equations occurs by expanding into a Taylor series and cutting off all the terms above first order.

Thus, almost any function can be represented as a polynomial with a given accuracy.

Examples of some common expansions of power functions in Maclaurin series (=McLaren,Taylor in the vicinity of point 0) and Taylor in the vicinity of point 1. The first terms of expansions of the main functions in Taylor and MacLaren series.

Examples of some common expansions of power functions in Maclaurin series (= MacLaren, Taylor in the vicinity of the point 0)

Examples of some common Taylor series expansions around point 1

Definition 1

The antiderivative $F(x)$ for the function $y=f(x)$ on the segment $$ is a function that is differentiable at each point of this segment and the following equality holds for its derivative:

Definition 2

The collection of all primitives given function$y=f(x)$ defined on some interval is called the indefinite integral of the given function $y=f(x)$. The indefinite integral is denoted by the symbol $\int f(x)dx $.

From the table of derivatives and Definition 2, we obtain a table of basic integrals.

Example 1

Check the validity of formula 7 from the table of integrals:

\[\int tgxdx =-\ln |\cos x|+C,\, \, C=const.\]

Let's differentiate the right side: $-\ln |\cos x|+C$.

\[\left(-\ln |\cos x|+C\right)"=-\frac(1)(\cos x) \cdot (-\sin x)=\frac(\sin x)(\cos x)=tgx\]

Example 2

Check the validity of formula 8 from the table of integrals:

\[\int ctgxdx =\ln |\sin x|+C,\, \, C=const.\]

Differentiate the right side: $\ln |\sin x|+C$.

\[\left(\ln |\sin x|\right)"=\frac(1)(\sin x) \cdot \cos x=ctgx\]

The derivative turned out to be equal to the integrand. Therefore, the formula is correct.

Example 3

Check the validity of formula 11" from the table of integrals:

\[\int \frac(dx)(a^(2) +x^(2) ) =\frac(1)(a) arctg\frac(x)(a) +C,\, \, C=const .\]

Differentiate the right side: $\frac(1)(a) arctg\frac(x)(a) +C$.

\[\left(\frac(1)(a) arctg\frac(x)(a) +C\right)"=\frac(1)(a) \cdot \frac(1)(1+\left( \frac(x)(a) \right)^(2) ) \cdot \frac(1)(a) =\frac(1)(a^(2) ) \cdot \frac(a^(2) ) (a^(2) +x^(2) ) \]

The derivative turned out to be equal to the integrand. Therefore, the formula is correct.

Example 4

Check the validity of formula 12 from the table of integrals:

\[\int \frac(dx)(a^(2) -x^(2) ) =\frac(1)(2a) \ln \left|\frac(a+x)(a-x) \right|+ C,\, \, C=const.\]

Differentiate the right side: $\frac(1)(2a) \ln \left|\frac(a+x)(a-x) \right|+C$.

$\left(\frac(1)(2a) \ln \left|\frac(a+x)(a-x) \right|+C\right)"=\frac(1)(2a) \cdot \frac( 1)(\frac(a+x)(a-x) ) \cdot \left(\frac(a+x)(a-x) \right)"=\frac(1)(2a) \cdot \frac(a-x)( a+x) \cdot \frac(a-x+a+x)((a-x)^(2) ) =\frac(1)(2a) \cdot \frac(a-x)(a+x) \cdot \ frac(2a)((a-x)^(2) ) =\frac(1)(a^(2) -x^(2) ) $The derivative is equal to the integrand. Therefore, the formula is correct.

Example 5

Check the validity of formula 13 "from the table of integrals:

\[\int \frac(dx)(\sqrt(a^(2) -x^(2) ) ) =\arcsin \frac(x)(a) +C,\, \, C=const.\]

Differentiate the right side: $\arcsin \frac(x)(a) +C$.

\[\left(\arcsin \frac(x)(a) +C\right)"=\frac(1)(\sqrt(1-\left(\frac(x)(a) \right)^(2 ) ) ) \cdot \frac(1)(a) =\frac(a)(\sqrt(a^(2) -x^(2) ) ) \cdot \frac(1)(a) =\frac( 1)(\sqrt(a^(2) -x^(2) ) ) \]

The derivative turned out to be equal to the integrand. Therefore, the formula is correct.

Example 6

Check the validity of formula 14 from the table of integrals:

\[\int \frac(dx)(\sqrt(x^(2) \pm a^(2) ) ) =\ln |x+\sqrt(x^(2) \pm a^(2) ) |+ C,\, \, C=const.\]

Differentiate the right side: $\ln |x+\sqrt(x^(2) \pm a^(2) ) |+C$.

\[\left(\ln |x+\sqrt(x^(2) \pm a^(2) ) |+C\right)"=\frac(1)(x+\sqrt(x^(2) \pm a^(2) ) ) \cdot \left(x+\sqrt(x^(2) \pm a^(2) ) \right)"=\frac(1)(x+\sqrt(x^(2) \ pm a^(2) ) ) \cdot \left(1+\frac(1)(2\sqrt(x^(2) \pm a^(2) ) ) \cdot 2x\right)=\] \[ =\frac(1)(x+\sqrt(x^(2) \pm a^(2) ) ) \cdot \frac(\sqrt(x^(2) \pm a^(2) ) +x)( \sqrt(x^(2) \pm a^(2) ) ) =\frac(1)(\sqrt(x^(2) \pm a^(2) ) ) \]

The derivative turned out to be equal to the integrand. Therefore, the formula is correct.

Example 7

Find the integral:

\[\int \left(\cos (3x+2)+5x\right) dx.\]

Let's use the sum integral theorem:

\[\int \left(\cos (3x+2)+5x\right) dx=\int \cos (3x+2)dx +\int 5xdx .\]

Let's use the theorem on taking the constant factor out of the integral sign:

\[\int \cos (3x+2)dx +\int 5xdx =\int \cos (3x+2)dx +5\int xdx .\]

According to the table of integrals:

\[\int \cos x dx=\sin x+C;\] \[\int xdx =\frac(x^(2) )(2) +C.\]

When calculating the first integral, we use rule 3:

\[\int \cos (3x+2) dx=\frac(1)(3) \sin (3x+2)+C_(1) .\]

Hence,

\[\int \left(\cos (3x+2)+5x\right) dx=\frac(1)(3) \sin (3x+2)+C_(1) +\frac(5x^(2) )(2) +C_(2) =\frac(1)(3) \sin (3x+2)+\frac(5x^(2) )(2) +C,\, \, C=C_(1 ) +C_(2) \]