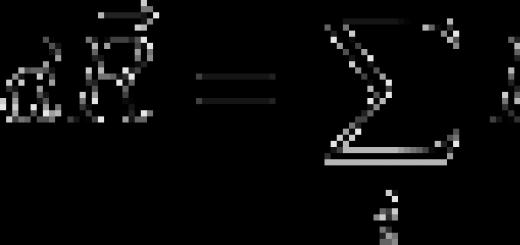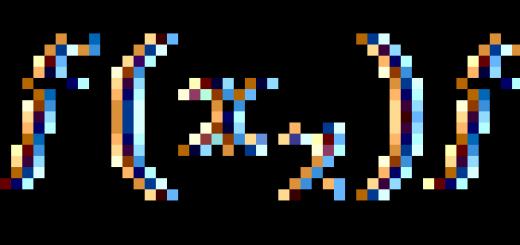Definition. The function F (x) is called antiderivative for the function f (x) on a given interval, if for any x from the given interval F "(x) \u003d f (x).

The main property of primitives.

If F (x) is the antiderivative of the function f (x), then the function F (x) + C , where C is an arbitrary constant, is also the antiderivative of the function f (x) (i.e., all antiderivatives of f(x) are written in the form F(x) + C).

Geometric interpretation.

Graphs of all antiderivatives of a given function f (x) are obtained from the graph of any one antiderivative by parallel transfers along the Oy axis.

Table of primitives.

Rules for finding antiderivatives .

Let F(x) and G(x) be the antiderivatives of the functions f(x) and g(x), respectively. Then:

1.F( x)±G( x) is antiderivative for f(x) ± g(x);

2. a F( x) is antiderivative for af(x);

3. - antiderivative for af(kx +b).

Tasks and tests on the topic "Antiprimitive"

• antiderivative

  Lessons: 1 Assignments: 11 Tests: 1

• Derivative and antiderivative - Preparation for the exam in Mathematics Unified State Examination mathematics

  Jobs: 3

• Integral - Antiderivative and integral Grade 11

  Lessons: 4 Assignments: 13 Tests: 1

• Calculating areas using integrals - Antiderivative and integral Grade 11

  Lessons: 1 Assignments: 10 Quizzes: 1

Having studied this topic, You must know what is called an antiderivative, its main property, geometric interpretation, the rules for finding antiderivatives; be able to find all antiderivatives of functions using a table and rules for finding antiderivatives, as well as an antiderivative passing through given point. Consider solving problems on this topic using examples. Pay attention to the design of the decisions.

Examples.

1. Find out if the function F ( x) = X 3 – 3X+ 1 antiderivative for the function f(x) = 3(X 2 – 1).

Decision: F"( x) = (X 3 – 3X+ 1)′ = 3 X 2 – 3 = 3(X 2 – 1) = f(x), i.e. F"( x) = f(x), therefore, F(x) is an antiderivative for the function f(x).

2. Find all antiderivative functions f(x) :

a) f(x) = X 4 + 3X 2 + 5

Decision: Using the table and the rules for finding antiderivatives, we get:

Answer:

b) f(x) = sin(3 x – 2)

Decision:

Solving integrals is an easy task, but only for the elite. This article is for those who want to learn to understand integrals, but know little or nothing about them. Integral... Why is it needed? How to calculate it? What are definite and indefinite integrals? If the only use of the integral you know is to get something useful from hard-to-reach places with a hook in the shape of an integral icon, then welcome! Learn how to solve integrals and why you can't do without it.

We study the concept of "integral"

Integration was already known in Ancient Egypt. Of course not in modern form, but still. Since then, mathematicians have written a great many books on the subject. Particularly distinguished Newton and Leibniz but the essence of things has not changed. How to understand integrals from scratch? No way! To understand this topic, you will still need a basic knowledge of the basics of mathematical analysis. Information about , which is also necessary for understanding integrals, is already in our blog.

Indefinite integral

Let's have some function f(x) .

The indefinite integral of the function f(x) such a function is called F(x) , whose derivative is equal to the function f(x) .

In other words, an integral is a reverse derivative or antiderivative. By the way, about how to read in our article.

An antiderivative exists for all continuous functions. Also, a constant sign is often added to the antiderivative, since the derivatives of functions that differ by a constant coincide. The process of finding an integral is called integration.

Simple example:

In order not to constantly calculate the primitives elementary functions, it is convenient to summarize them in a table and use ready-made values.

Complete table of integrals for students

Definite integral

When dealing with the concept of an integral, we are dealing with infinitesimal quantities. The integral will help calculate the area of ​​\u200b\u200bthe figure, the mass of an inhomogeneous body passed through uneven movement path and more. It should be remembered that the integral is the sum of infinite a large number infinitesimal terms.

As an example, imagine a graph of some function. How to find the area of ​​a figure bounded by a graph of a function?

With the help of an integral! Let's break the curvilinear trapezoid, bounded by the coordinate axes and the graph of the function, into infinitesimal segments. Thus, the figure will be divided into thin columns. The sum of the areas of the columns will be the area of ​​the trapezoid. But remember that such a calculation will give an approximate result. However, the smaller and narrower the segments, the more accurate the calculation will be. If we reduce them to such an extent that the length tends to zero, then the sum of the areas of the segments will tend to the area of ​​the figure. This is the definite integral, which is written as follows:

The points a and b are called the limits of integration.

Bari Alibasov and the group "Integral"

By the way! For our readers there is now a 10% discount on

Rules for Calculating Integrals for Dummies

Properties of the indefinite integral

How to solve indefinite integral? Here we will consider the properties of the indefinite integral, which will be useful in solving examples.

• The derivative of the integral is equal to the integrand:

• The constant can be taken out from under the integral sign:

• The integral of the sum is equal to the sum of the integrals. Also true for the difference:

Properties of the Definite Integral

• Linearity:

• The sign of the integral changes if the limits of integration are reversed:

• At any points a, b and with:

We have already found out that the definite integral is the limit of the sum. But how to get a specific value when solving an example? For this, there is the Newton-Leibniz formula:

Examples of solving integrals

Below we consider several examples of finding indefinite integrals. We offer you to independently understand the intricacies of the solution, and if something is not clear, ask questions in the comments.

To consolidate the material, watch a video on how integrals are solved in practice. Do not despair if the integral is not given immediately. Turn to a professional student service, and any triple or curvilinear integral over a closed surface will be within your power.

antiderivative function f(x) in between (a;b) such a function is called F(x), that equality holds for any X from the given interval.

If we take into account the fact that the derivative of the constant With equals zero, then the equality holds. So the function f(x) has many prototypes F(x)+C, for an arbitrary constant With, and these antiderivatives differ from each other by an arbitrary constant value.

Definition of the indefinite integral.

The whole set of antiderivatives of a function f(x) is called the indefinite integral of this function and is denoted .

The expression is called integrand, a f(x) – integrand. The integrand is the differential of the function f(x).

The action of finding an unknown function by its given differential is called uncertain integration, because the result of integration is more than one function F(x), and the set of its primitives F(x)+C.

The geometric meaning of the indefinite integral. The graph of the antiderivative D(x) is called the integral curve. In the x0y coordinate system, the graphs of all antiderivatives of a given function represent a family of curves that depend on the value of the constant C and are obtained one from the other by a parallel shift along the 0y axis. For the example above, we have:

J 2 x^x = x2 + C.

The family of antiderivatives (x + C) is interpreted geometrically as a set of parabolas.

If you need to find one from the family of antiderivatives, then additional conditions are set that allow you to determine the constant C. Usually, for this purpose, initial conditions are set: for the value of the argument x = x0, the function has the value D(x0) = y0.

Example. It is required to find that of the antiderivatives of the function y \u003d 2 x, which takes the value 3 at x0 \u003d 1.

The desired antiderivative: D(x) = x2 + 2.

Decision. ^2x^x = x2 + C; 12 + C = 3; C = 2.

2. Basic properties of the indefinite integral

1. The derivative of the indefinite integral is equal to the integrand:

2. The differential of the indefinite integral is equal to the integrand:

3. The indefinite integral of the differential of some function is equal to the sum of this function itself and an arbitrary constant:

4. A constant factor can be taken out of the integral sign:

5. The integral of the sum (difference) is equal to the sum (difference) of the integrals:

6. The property is a combination of properties 4 and 5:

7. The invariance property of the indefinite integral:

If a , then

8. Property:

If a , then

In fact, this property is a special case of integration using the change of variable method, which is discussed in more detail in the next section.

Consider an example:

3. integration method, in which the given integral is reduced to one or more table integrals by identical transformations of the integrand (or expression) and applying the properties of the indefinite integral, is called direct integration. When reducing this integral to a tabular one, the following transformations of the differential are often used (the operation " bringing under the sign of the differential»):

Generally, f'(u)du = d(f(u)). this (formula is very often used in the calculation of integrals.

Find the integral

Decision. We use the properties of the integral and reduce this integral to several tabular ones.

4. Integration by the substitution method.

The essence of the method is that we introduce a new variable, express the integrand in terms of this variable, and as a result we arrive at a tabular (or simpler) form of the integral.

Very often, the substitution method helps out when integrating trigonometric functions and functions with radicals.

Example.

Find the indefinite integral .

Decision.

Let's introduce a new variable . Express X through z:

We perform the substitution of the obtained expressions into the original integral:

From the table of antiderivatives we have .

It remains to return to the original variable X:

Answer:

Lesson and presentation on the topic: "Antiderivative function. Graph of a function"

Additional materials
Dear users, do not forget to leave your comments, feedback, suggestions! All materials are checked by an antivirus program.

Teaching aids and simulators in the online store "Integral" for grade 11
Algebraic problems with parameters, grades 9–11
"Interactive tasks for building in space for grades 10 and 11"

primitive function. Introduction

Guys, you can find derivatives of functions using various formulas and rules. Today we will study the operation, inverse to calculation derivative. The concept of a derivative is often used in real life. Let me remind you: the derivative is the rate of change of a function at a particular point. The processes associated with movement and speed are well described in these terms.

Let's consider the following task: "The speed of an object's movement along a straight line is described by the formula $V=gt$. It is required to restore the law of motion.
Decision.
We know the formula well: $S"=v(t)$, where S is the law of motion.
Our problem is reduced to finding a function $S=S(t)$ whose derivative is equal to $gt$. Looking carefully, you can guess that $S(t)=\frac(g*t^2)(2)$.
Let's check the correctness of the solution of this problem: $S"(t)=(\frac(g*t^2)(2))"=\frac(g)(2)*2t=g*t$.
Knowing the derivative of the function, we found the function itself, that is, we performed the inverse operation.
But it is worth paying attention to this moment. The solution of our problem requires clarification, if any number (constant) is added to the found function, then the value of the derivative will not change: $S(t)=\frac(g*t^2)(2)+c,c=const$.
$S"(t)=(\frac(g*t^2)(2))"+c"=g*t+0=g*t$.

Guys, pay attention: our task has infinite set solutions!
If the problem does not have an initial or some other condition, do not forget to add a constant to the solution. For example, in our task, the position of our body at the very beginning of the movement can be set. Then it is not difficult to calculate the constant, substituting zero into the resulting equation, we get the value of the constant.

What is the name of such an operation?
The operation inverse to differentiation is called integration.
Finding a function by a given derivative - integration.
The function itself will be called antiderivative, that is, the image from which the derivative of the function was obtained.
The antiderivative is usually written with a capital letter $y=F"(x)=f(x)$.

Definition. The function $y=F(x)$ is called the antiderivative function $y=f(x)$ on the interval X if $F’(x)=f(x)$ is true for any $xϵX$.

Let's make a table of antiderivatives for various functions. It should be printed as a reminder and memorized.

In our table, no initial conditions were specified. This means that a constant must be added to each expression on the right side of the table. We will refine this rule later.

Rules for finding antiderivatives

Let's write down some rules that will help us in finding antiderivatives. All of them are similar to the rules of differentiation.

Rule 1 The antiderivative of a sum is equal to the sum of antiderivatives. $F(x+y)=F(x)+F(y)$.

Example.
Find the antiderivative for the function $y=4x^3+cos(x)$.
Decision.
The antiderivative of the sum is equal to the sum of the antiderivatives, then you need to find the antiderivative for each of the presented functions.
$f(x)=4x^3$ => $F(x)=x^4$.
$f(x)=cos(x)$ => $F(x)=sin(x)$.
Then the antiderivative of the original function will be: $y=x^4+sin(x)$ or any function of the form $y=x^4+sin(x)+C$.

Rule 2 If $F(x)$ is an antiderivative for $f(x)$, then $k*F(x)$ is an antiderivative for $k*f(x)$.(We can safely take the coefficient out of the function).

Example.
Find antiderivatives of functions:
a) $y=8sin(x)$.
b) $y=-\frac(2)(3)cos(x)$.
c) $y=(3x)^2+4x+5$.
Decision.
a) The antiderivative for $sin(x)$ is minus $cos(x)$. Then the antiderivative of the original function will take the form: $y=-8cos(x)$.

B) The antiderivative for $cos(x)$ is $sin(x)$. Then the antiderivative of the original function will take the form: $y=-\frac(2)(3)sin(x)$.

C) The antiderivative for $x^2$ is $\frac(x^3)(3)$. The antiderivative for x is $\frac(x^2)(2)$. The antiderivative for 1 is x. Then the antiderivative of the original function will take the form: $y=3*\frac(x^3)(3)+4*\frac(x^2)(2)+5*x=x^3+2x^2+5x$ .

Rule 3 If $y=F(x)$ is the antiderivative for the function $y=f(x)$, then the antiderivative for the function $y=f(kx+m)$ is the function $y=\frac(1)(k)* F(kx+m)$.

Example.
Find the antiderivatives of the following functions:
a) $y=cos(7x)$.
b) $y=sin(\frac(x)(2))$.
c) $y=(-2x+3)^3$.
d) $y=e^(\frac(2x+1)(5))$.
Decision.
a) The antiderivative for $cos(x)$ is $sin(x)$. Then the antiderivative for the function $y=cos(7x)$ will be the function $y=\frac(1)(7)*sin(7x)=\frac(sin(7x))(7)$.

B) The antiderivative for $sin(x)$ is minus $cos(x)$. Then the antiderivative for the function $y=sin(\frac(x)(2))$ is the function $y=-\frac(1)(\frac(1)(2))cos(\frac(x)(2) )=-2cos(\frac(x)(2))$.

C) The antiderivative for $x^3$ is $\frac(x^4)(4)$, then the antiderivative of the original function is $y=-\frac(1)(2)*\frac(((-2x+3) )^4)(4)=-\frac(((-2x+3))^4)(8)$.

D) We slightly simplify the expression to the power of $\frac(2x+1)(5)=\frac(2)(5)x+\frac(1)(5)$.
The antiderivative of the exponential function is itself exponential function. The antiderivative of the original function will be $y=\frac(1)(\frac(2)(5))e^(\frac(2)(5)x+\frac(1)(5))=\frac(5)( 2)*e^(\frac(2x+1)(5))$.

Theorem. If $y=F(x)$ is the antiderivative for the function $y=f(x)$ on the interval X, then the function $y=f(x)$ has infinitely many antiderivatives, and they all have the form $y=F( x)+C$.

If in all the examples that were considered above, it would be required to find the set of all antiderivatives, then the constant C should be added everywhere.
For the function $y=cos(7x)$ all antiderivatives have the form: $y=\frac(sin(7x))(7)+C$.
For the function $y=(-2x+3)^3$ all antiderivatives have the form: $y=-\frac(((-2x+3))^4)(8)+C$.

Example.
According to the given law of change of body speed from time $v=-3sin(4t)$ find the law of motion $S=S(t)$ if at the initial moment of time the body had a coordinate equal to 1.75.
Decision.
Since $v=S'(t)$, we need to find the antiderivative for a given speed.
$S=-3*\frac(1)(4)(-cos(4t))+C=\frac(3)(4)cos(4t)+C$.
In this problem, an additional condition is given - the initial moment of time. This means that $t=0$.
$S(0)=\frac(3)(4)cos(4*0)+C=\frac(7)(4)$.
$\frac(3)(4)cos(0)+C=\frac(7)(4)$.
$\frac(3)(4)*1+C=\frac(7)(4)$.
$C=1$.
Then the law of motion is described by the formula: $S=\frac(3)(4)cos(4t)+1$.

Tasks for independent solution

1. Find antiderivatives of functions:
a) $y=-10sin(x)$.
b) $y=\frac(5)(6)cos(x)$.
c) $y=(4x)^5+(3x)^2+5x$.
2. Find antiderivatives of the following functions:
a) $y=cos(\frac(3)(4)x)$.
b) $y=sin(8x)$.
c) $y=((7x+4))^4$.
d) $y=e^(\frac(3x+1)(6))$.
3. According to the given law of change of body velocity from time $v=4cos(6t)$ find the law of motion $S=S(t)$ if at the initial moment of time the body had a coordinate equal to 2.

For every mathematical action there is an inverse action. For the action of differentiation (finding derivatives of functions), there is also an inverse action - integration. By means of integration, a function is found (restored) by its given derivative or differential. The found function is called primitive.

Definition. Differentiable function F(x) is called antiderivative for the function f(x) on a given interval, if for all X from this interval the equality is true: F′(x)=f (x).

Examples. Find antiderivatives for functions: 1) f (x)=2x; 2) f(x)=3cos3x.

1) Since (x²)′=2x, then, by definition, the function F (x)=x² will be the antiderivative for the function f (x)=2x.

2) (sin3x)′=3cos3x. If we denote f (x)=3cos3x and F (x)=sin3x, then, by the definition of antiderivative, we have: F′(x)=f (x), and, therefore, F (x)=sin3x is an antiderivative for f ( x)=3cos3x.

Note that and (sin3x +5 )′= 3cos3x, and (sin3x -8,2 )′= 3cos3x, ... in general form, we can write: (sin3x +C)′= 3cos3x, where With- some constant. These examples speak of the ambiguity of the action of integration, in contrast to the action of differentiation, when any differentiable function has a single derivative.

Definition. If the function F(x) is the antiderivative for the function f(x) on some interval, then the set of all antiderivatives of this function has the form:

F(x)+C where C is any real number.

The set of all antiderivatives F (x) + C of the function f (x) on the interval under consideration is called the indefinite integral and is denoted by the symbol ∫ (integral sign). Write down: ∫f (x) dx=F (x)+C.

Expression ∫f(x)dx read: "the integral ef from x to de x".

f(x)dx is the integrand,

f(x) is the integrand,

X is the integration variable.

F(x) is the antiderivative for the function f(x),

With is some constant value.

Now the considered examples can be written as follows:

1) ∫ 2хdx=x²+C. 2) ∫ 3cos3xdx=sin3x+C.

What does the sign d mean?

d- differential sign - has a dual purpose: firstly, this sign separates the integrand from the integration variable; secondly, everything after this sign is differentiated by default and multiplied by the integrand.

Examples. Find integrals: 3) ∫ 2pxdx; 4) ∫ 2pxdp.

3) After differential icon d costs XX, a R

∫ 2хрdx=px²+С. Compare with example 1).

Let's do a check. F′(x)=(px²+C)′=p (x²)′+C′=p 2x=2px=f (x).

4) After differential icon d costs R. So the integration variable R, and the multiplier X should be considered as a constant value.

∫ 2хрdр=р²х+С. Compare with examples 1) and 3).

Let's do a check. F′(p)=(p²x+C)′=x (p²)′+C′=x 2p=2px=f (p).