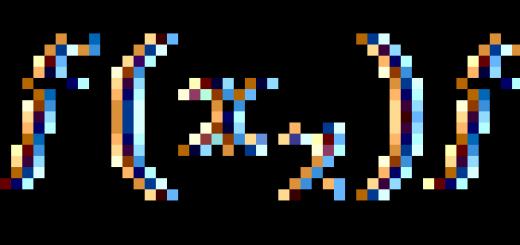Lessons 32-33. Reverse trigonometric functions

09.07.2015 6432 0

Target: consider inverse trigonometric functions, their use for writing solutions trigonometric equations.

I. Communication of the topic and objectives of the lessons

II. Learning new material

1. Inverse trigonometric functions

Let's start this topic with the following example.

Example 1

Let's solve the equation: a) sin x = 1/2; b) sin x \u003d a.

a) On the ordinate axis, set aside the value 1/2 and plot the angles x 1 and x2, for which sin x = 1/2. In this case, x1 + x2 = π, whence x2 = π – x 1 . According to the table of values ​​of trigonometric functions, we find the value x1 = π/6, thenWe take into account the periodicity of the sine function and write down the solutions given equation: where k ∈ Z .

b) It is obvious that the algorithm for solving the equation sin x = a is the same as in the previous paragraph. Of course, now the value of a is plotted along the y-axis. There is a need to somehow designate the angle x1. We agreed to denote such an angle by the symbol arc sin a. Then the solutions of this equation can be written asThese two formulas can be combined into one: wherein

Other inverse trigonometric functions are introduced similarly.

Very often it is necessary to determine the value of an angle from the known value of its trigonometric function. Such a problem is multivalued - there are an infinite number of angles whose trigonometric functions are equal to the same value. Therefore, based on the monotonicity of trigonometric functions, the following inverse trigonometric functions are introduced to uniquely determine the angles.

The arcsine of a (arcsin , whose sine is equal to a, i.e.

Arc cosine of a number a(arccos a) - such an angle a from the interval, the cosine of which is equal to a, i.e.

Arc tangent of a number a(arctg a) - such an angle a from the intervalwhose tangent is a, i.e.tg a = a.

Arc tangent of a number a(arctg a) - such an angle a from the interval (0; π), whose cotangent is equal to a, i.e. ctg a = a.

Example 2

Let's find:

Given the definitions of inverse trigonometric functions, we get:

Example 3

Compute

Let angle a = arcsin 3/5, then by definition sin a = 3/5 and . Therefore, we need to find cos a. Using the basic trigonometric identity, we get:It is taken into account that cos a ≥ 0. So,

Function Properties	Function
	y = arcsin x	y = arccos x	y = arctg x	y = arcctg x
Domain	x ∈ [-1; one]	x ∈ [-1; one]	x ∈ (-∞; +∞)	x ∈ (-∞ +∞)
Range of values	y ∈ [-π/2 ; π/2]	y ∈	y ∈ (-π/2 ; π /2 )	y ∈ (0; π)
Parity	odd	Neither even nor odd	odd	Neither even nor odd
Function zeros (y = 0)	When x = 0	For x = 1	When x = 0	y ≠ 0
Constancy intervals	y > 0 for x ∈ (0; 1], at< 0 при х ∈ [-1; 0)	y > 0 for x ∈ [-1; one)	y > 0 for x ∈ (0; +∞), at< 0 при х ∈ (-∞; 0)	y > 0 for x ∈ (-∞; +∞)
Monotone	Increasing	Decreases	Increasing	Decreases
Relationship with the trigonometric function	sin y \u003d x	cos y = x	tg y = x	ctg y=x
Schedule

Let us give a number of typical examples related to the definitions and basic properties of inverse trigonometric functions.

Example 4

Find the domain of the function

In order for the function y to be defined, it is necessary that the inequalitywhich is equivalent to the system of inequalitiesThe solution to the first inequality is the interval x∈ (-∞; +∞), the second - This interval and is a solution to the system of inequalities, and hence the domain of the function

Example 5

Find the area of ​​change of the function

Consider the behavior of the function z \u003d 2x - x2 (see figure).

It can be seen that z ∈ (-∞; 1]. Given that the argument z function of the inverse tangent varies within the specified limits, from the data in the table we obtain thatThus, the area of ​​change

Example 6

Let us prove that the function y = arctg x odd. Let beThen tg a \u003d -x or x \u003d - tg a \u003d tg (- a), and Therefore, - a \u003d arctg x or a \u003d - arctg X. Thus, we see thati.e., y(x) is an odd function.

Example 7

We express in terms of all inverse trigonometric functions

Let be It's obvious that Then since

Let's introduce an angle As then

Similarly, therefore and

So,

Example 8

Let's build a graph of the function y \u003d cos (arcsin x).

Denote a \u003d arcsin x, then We take into account that x \u003d sin a and y \u003d cos a, i.e. x 2 + y2 = 1, and restrictions on x (x∈ [-one; 1]) and y (y ≥ 0). Then the graph of the function y = cos(arcsin x) is a semicircle.

Example 9

Let's build a graph of the function y \u003d arccos(cosx).

Since the function cos x changes on the segment [-1; 1], then the function y is defined on the entire real axis and changes on the interval . We will keep in mind that y = arccos(cosx) \u003d x on the segment; the function y is even and periodic with a period of 2π. Considering that the function has these properties cos x , Now it's easy to plot.

We note some useful equalities:

Example 10

Find the smallest and greatest value functions Denote then Get a function This function has a minimum at the point z = π/4, and it is equal to The maximum value of the function is reached at the point z = -π/2, and it is equal to Thus, and

Example 11

Let's solve the equation

We take into account that Then the equation looks like:or where By definition of the arc tangent, we get:

2. Solution of the simplest trigonometric equations

Similarly to example 1, you can get solutions to the simplest trigonometric equations.

The equation	Decision
tgx = a
ctg x = a

Example 12

Let's solve the equation

Since the sine function is odd, we write the equation in the formSolutions to this equation:where do we find

Example 13

Let's solve the equation

According to the above formula, we write the solutions of the equation:and find

Note that in particular cases (a = 0; ±1) when solving the equations sin x = a and cos x \u003d but it is easier and more convenient to use not general formulas, but write solutions based on a unit circle:

for the equation sin x = 1 solution

for the equation sin x \u003d 0 solutions x \u003d π k;

for the equation sin x = -1 solution

for the equation cos x = 1 solutions x = 2π k;

for the equation cos x = 0 solution

for the equation cos x = -1 solution

Example 14

Let's solve the equation

Since in this example there is special case equations, then according to the corresponding formula we write the solution:where do we find

III. Control questions (frontal survey)

1. Define and list the main properties of inverse trigonometric functions.

2. Give graphs of inverse trigonometric functions.

3. Solution of the simplest trigonometric equations.

IV. Assignment in the lessons

§ 15, no. 3 (a, b); 4 (c, d); 7(a); 8(a); 12(b); 13(a); 15 (c); 16(a); 18 (a, b); 19 (c); 21;

§ 16, no. 4 (a, b); 7(a); 8 (b); 16 (a, b); 18(a); 19 (c, d);

§ 17, no. 3 (a, b); 4 (c, d); 5 (a, b); 7 (c, d); 9 (b); 10 (a, c).

V. Homework

§ 15, no. 3 (c, d); 4 (a, b); 7 (c); 8 (b); 12(a); 13(b); 15 (d); 16(b); 18 (c, d); 19 (d); 22;

§ 16, no. 4 (c, d); 7(b); 8(a); 16 (c, d); 18(b); 19 (a, b);

§ 17, no. 3 (c, d); 4 (a, b); 5 (c, d); 7 (a, b); 9 (d); 10 (b, d).

VI. Creative tasks

1. Find the scope of the function:

Answers :

2. Find the range of the function:

Answers:

3. Graph the function:

VII. Summing up the lessons

Preparation for the exam in mathematics

Experiment

Lesson 9 Inverse trigonometric functions.

Practice

Lesson summary

Mainly, the ability to work with arc functions will be useful to us when solving trigonometric equations and inequalities.

The tasks that we will now consider are divided into two types: calculating the values ​​of inverse trigonometric functions and converting them using basic properties.

Calculating the values ​​of arc functions

Let's start by calculating the values ​​of the arc functions.

Task #1. Calculate .

As you can see, all the arguments of the arc functions are positive and tabular, which means that we can restore the value of the angles from the first part of the table of values ​​of trigonometric functions for angles from to . This range of angles is included in the range of values ​​of each of the arc functions, so we simply use the table, find the value of the trigonometric function in it and restore which angle it corresponds to.

a)

b)

in)

G)

Answer. .

Task #2. Calculate

.

In this example, we already see negative arguments. Common Mistake in this case, it's just to remove the minus from under the function and simply reduce the task to the previous one. However, this may not be possible in all cases. Let's recall how in the theoretical part of the lesson we stipulated the parity of all arc functions. The odd ones are the arcsine and arctangent, i.e., the minus is taken out of them, and the arccosine and arccotangent are functions general view, to simplify the minus in the argument, they have special formulas. After the calculation, in order to avoid errors, we check that the result is included in the range of values.

When the function arguments are simplified to a positive form, we write out the corresponding angle values ​​from the table.

The question may arise, why not write out the value of the angle corresponding, for example, immediately from the table? Firstly, because the table before is harder to remember than before, and secondly, because there are no negative sine values ​​\u200b\u200bin it, and negative values tangent will give the wrong angle according to the table. It is better to have a one-size-fits-all approach to a solution than to get confused by many different approaches.

Task #3. Calculate .

a) A typical mistake in this case is to start taking out a minus and simplifying something. The first thing to notice is that the arcsine argument is out of scope

Therefore, this entry does not matter, and the arcsine cannot be calculated.

b) The standard error in this case is that they confuse the values ​​\u200b\u200bof the argument and the function and give the answer. This is not true! Of course, the idea arises that in the table the value corresponds to the cosine, but in this case it is confused that the arc functions are calculated not from angles, but from the values ​​of trigonometric functions. That is, not .

In addition, since we have found out what exactly is the argument of the arc cosine, it is necessary to check that it is included in the domain of definition. To do this, remember that , i.e., which means that the arc cosine does not make sense and it cannot be calculated.

By the way, for example, the expression makes sense, because, but since the value of the cosine equal to is not tabular, then it is impossible to calculate the arc cosine using the table.

Answer. The expressions don't make sense.

In this example, we do not consider the arctangent and arccotangent, since they have no limited scope and the values ​​of the functions will be for any arguments.

Task #4. Calculate .

In fact, the task is reduced to the very first one, we just need to separately calculate the values ​​of the two functions, and then substitute them into the original expression.

The arc tangent argument is tabular and the result is in the range.

The arccosine argument is not tabular, but this should not scare us, because whatever the arccosine is equal to, its value when multiplied by zero will result in zero. One important note remains: it is necessary to check whether the argument of the arccosine belongs to the domain of definition, because if it does not, then the whole expression will not make sense, regardless of the fact that it contains multiplication by zero. But , so we can argue that it makes sense and we get zero in the answer.

Let us give another example in which it is necessary to be able to calculate one arc function, knowing the value of another.

Task #5. Calculate if it is known that .

It may seem that it is necessary to first calculate the value of x from the indicated equation, and then substitute it into the desired expression, i.e., into the arc cotangent, but this is not necessary.

Let's recall the formula by which these functions are interconnected:

And we will express from it what we need:

To be sure, you can check that the result lies in the range of the arc cotangent.

Transformations of arc functions using their basic properties

Now let's move on to a series of tasks in which we will have to use transformations of arc functions using their basic properties.

Task #6. Calculate .

For the solution, we will use the main properties of the indicated arc functions, only necessarily checking the restrictions corresponding to them.

a)

b) .

Answer. a) ; b) .

Task #7. Calculate .

A typical mistake in this case is to immediately write 4 in the answer. As we indicated in the previous example, in order to use the main properties of arc functions, it is necessary to check the corresponding restrictions on their argument. We are dealing with a property:

at

But . The main thing at this stage of the decision is not to think that the specified expression does not make sense and cannot be calculated. After all, the quadruple, which is the argument of the tangent, we can reduce by subtracting the period of the tangent, and this will not affect the value of the expression. Having done such actions, we will have a chance to reduce the argument so that it enters the specified range.

Since, therefore, , because .

Task #8. Calculate.

In this example, we are dealing with an expression that is similar to the main property of the arcsine, but only it contains cofunctions. It must be brought to the form of the sine of the arcsine or the cosine of the arccosine. Since it is easier to convert direct trigonometric functions than inverse ones, let's move from sine to cosine using the "trigonometric unit" formula.

As we already know:

In our case, in the role . For convenience, we first calculate .

Before substituting it into the formula, we find out its sign, that is, the sign of the original sine. We must calculate the sine from the value of the arc cosine, whatever this value may be, we know that it lies in the range. This range corresponds to the angles of the first and second quarters, in which the sine is positive (check this for yourself using the trigonometric circle).

Today practical lesson we have considered the calculation and transformation of expressions containing inverse trigonometric functions

Fix the material with the help of simulators

Machine 1 Machine 2 Machine 3 Machine 4 Machine 5

Graduation work on the topic "Inverse trigonometric functions. Problems containing inverse trigonometric functions" was completed at advanced training courses.

Contains brief theoretical material, analyzed examples and tasks for independent solution in each section.

The work is addressed to high school students, teachers.

Download:

Preview:

GRADUATION WORK

TOPIC:

«INVERSE TRIGONOMETRIC FUNCTIONS.

PROBLEMS CONTAINING INVERSE TRIGONOMETRIC FUNCTIONS»

Performed:

mathematic teacher

MOU secondary school №5, Lermontov

GORBACHENKO V.I.

Pyatigorsk 2011

INVERSE TRIGONOMETRIC FUNCTIONS.

PROBLEMS CONTAINING INVERSE TRIGONOMETRIC FUNCTIONS

1. BRIEF THEORETICAL INFORMATION

1.1. Solutions of the simplest equations containing inverse trigonometric functions:

Table 1.

The equation	Decision

1.2. Solution of the simplest inequalities containing inverse trigonometric functions

Table 2.

Inequality	Decision

1.3. Some identities for inverse trigonometric functions

From the definition of inverse trigonometric functions, the identities follow

, (1)

, (2)

, (3)

, (4)

In addition, the identities

, (5)

, (6)

, (7)

, (8)

Identities relating oppositely named inverse trigonometric functions

(9)

(10)

2. EQUATIONS CONTAINING INVERSE TRIGONOMETRIC FUNCTIONS

2.1. Equations of the form etc.

Such equations reduce to rational equations substitution.

Example.

Decision.

Replacement ( ) reduces the equation to a quadratic one, the roots of which are.

Root 3 does not satisfy the condition.

Then we get the reverse substitution

Answer .

Tasks.

2.2. Equations of the form, where - rational function.

To solve equations of this type, it is necessary to set, solve the equation of the simplest formand do the back substitution.

Example.

Decision .

Let be . Then

Answer . .

Tasks .

2.3. Equations containing either different arc functions or arc functions of different arguments.

If the equation includes expressions containing different arc functions, or these arc functions depend on different arguments, then the reduction of such equations to their algebraic consequence is usually carried out by calculating some trigonometric function from both parts of the equation. The resulting extraneous roots are separated by checking. If a tangent or cotangent is chosen as a direct function, then the solutions included in the domain of definition of these functions may be lost. Therefore, before calculating the value of the tangent or cotangent from both parts of the equation, you should make sure that among the points that are not included in the domain of definition of these functions, there are no roots of the original equation.

Example.

Decision .

reschedule to the right side and calculate the value of the sine of both sides of the equation

As a result of the transformations, we get

The roots of this equation

Let's check

When we have

Thus, is the root of the equation.

Substituting , note that the left side of the resulting relation is positive, and the right side is negative. Thus,is an extraneous root of the equation.

Answer. .

Tasks.

2.4. Equations containing inverse trigonometric functions of one argument.

Such equations can be reduced to the simplest ones using the basic identities (1) - (10).

Example.

Decision.

Answer.

Tasks.

3. INEQUALITIES CONTAINING INVERSE TRIGONOMETRIC FUNCTIONS

3.1. The simplest inequalities.

The solution of the simplest inequalities is based on the application of the formulas of Table 2.

Example.

Decision.

Because , then the solution of the inequality is the interval.

Answer .

Tasks.

3.2. Inequalities of the form, is some rational function.

Inequalities of the form, is some rational function, and- one of the inverse trigonometric functions is solved in two stages - first, the inequality with respect to the unknown is solved, and then the simplest inequality containing the inverse trigonometric function.

Example.

Decision.

Let then

Inequality Solutions

Returning to the original unknown, we find that the original inequality reduces to two simple

Combining these solutions, we obtain solutions to the original inequality

Answer .

Tasks.

3.3. Inequalities containing either unlike arc functions or arc functions of different arguments.

Inequalities relating the values ​​of various inverse trigonometric functions or the values ​​of one trigonometric function calculated from different arguments can be conveniently solved by calculating the values ​​of some trigonometric function from both parts of the inequalities. It should be remembered that the resulting inequality will be equivalent to the original one only if the set of values ​​of the right and left parts of the original inequality belongs to the same interval of monotonicity of this trigonometric function.

Example.

Decision.

A bunch of allowed values included in the inequality:. At . Therefore, the valuesare not solutions to the inequality.

At both the right-hand side and the left-hand side of the inequality have values, belonging to the gap . Because in betweenthe sine function is monotonically increasing, then whenthe original inequality is equivalent to

We solve the last inequality

Crossing with a gap, we get the solution

Answer.

Comment. Can be solved using

Tasks.

3.4. Inequality of the form, where is one of the inverse trigonometric functions,is a rational function.

Such inequalities are solved using the substitutionand reduction to the simplest inequality in Table 2.

Example.

Decision.

Let then

Let's do the reverse substitution, we get the system

Answer .

Tasks.

Federal Agency for Education of the Russian Federation

SEI HPE "Mari State University"

Department of Mathematics and MPM

Course work

Inverse trigonometric functions

Performed:

student

33 JNF groups

Yashmetova L. N.

Supervisor:

Ph.D. assistant professor

Borodina M.V.

Yoshkar-Ola

Introduction…………………………………………………………………………...3

Chapter I. Definition of inverse trigonometric functions.

1.1. Function y=arc sin x……………………………………………………........4

1.2. Function y=arccos x…………………………………………………….......5

1.3. Function y=arctg x………………………………………………………….6

1.4. Function y=arcctg x…………………………………………………….......7

Chapter II. Solution of equations with inverse trigonometric functions.

Basic relations for inverse trigonometric functions ... .8

Solving Equations Containing Inverse Trigonometric Functions………………………………………………………………………..11

Calculation of the values ​​of inverse trigonometric functions .................... 21

Conclusion………………………………………………………………………….25

List of used literature…………………………………………...26

Introduction

In many problems, there is a need to find not only the values ​​of trigonometric functions for a given angle, but also, conversely, an angle or an arc for set value some trigonometric function.

Problems with inverse trigonometric functions are contained in the USE tasks (especially a lot in parts B and C). For example, in part B of the Unified State Examination, it was required to find the corresponding value of the tangent by the value of the sine (cosine) or calculate the value of an expression containing tabular values ​​of inverse trigonometric functions. Regarding this type of tasks, we note that such tasks in school textbooks are not enough to form a solid skill in their implementation.

That. purpose term paper is to consider inverse trigonometric functions and their properties, and learn how to solve problems with inverse trigonometric functions.

To achieve the goal, we need to solve the following tasks:

Explore theoretical basis inverse trigonometric functions,

Show the application of theoretical knowledge in practice.

ChapterI. Definition of inverse trigonometric functions

1.1. Function y =arc sinx

Consider the function
. (1)

In this interval, the function is monotonic (increases from -1 to 1), therefore, there is an inverse function

,
. (2)

For every given value at(sine value) from the interval [-1,1] corresponds to one well-defined value X(arc value) from span
. Passing to the generally accepted notation, we get

Where
. (3)

This is the analytical specification of the function inverse to function (1). Function (3) is called arcsine argument . The graph of this function is a curve symmetrical to the graph of the function , where , with respect to the bisector of the I and III coordinate angles.

Let us present the properties of the function, where .

Property 1. Area of ​​change of function values: .

Property 2. The function is odd, i.e.

Property 3. The function, where , has a single root
.

Property 4. If then
; if , then.

Property 5. The function is monotonic: as the argument increases from -1 to 1, the value of the function increases from
before
.

1.2. Functiony = arwithcosx

Consider the function
, . (4)

In this interval, the function is monotonic (decreases from +1 to -1), which means that there is an inverse function for it

, , (5)

those. each value (cosine value) from the interval [-1,1] corresponds to one well-defined value (arc value) from the interval . Passing to the generally accepted notation, we get

, . (6)

This is the analytical specification of the function inverse to function (4). Function (6) is called arc cosine argument X. The graph of this function can be built on the basis of the properties of graphs of mutually inverse functions.

The function , where , has the following properties.

Property 1. Area of ​​change of function values:
.

Property 2. Quantities
and
related by the ratio

Property 3. The function has a single root
.

Property 4. The function does not accept negative values.

Property 5. The function is monotonic: as the argument increases from -1 to +1, the function values ​​decrease from to 0.

1.3. Functiony = arctgx

Consider the function
,
. (7)

Note that this function is defined for all values ​​lying strictly inside the interval from to ; it does not exist at the ends of this interval, since the values

- breakpoints of the tangent.

In the interim
the function is monotonic (increases from -
before
), therefore, for function (1) there is an inverse function:

,
, (8)

those. to each given value (tangent value) from the interval
corresponds to one well-defined value (the magnitude of the arc) from the interval .

Passing to the generally accepted notation, we get

,
. (9)

This is the analytical specification of the function inverse to (7). Function (9) is called arc tangent argument X. Note that when
function value
, and when

, i.e. The function graph has two asymptotes:
and.

The function , , has the following properties.

Property 1. Range of function values
.

Property 2. The function is odd, i.e. .

Property 3. The function has a single root .

Property 4. If a
, then

; if , then
.

Property 5. The function is monotonic: as the argument from to increases, the function values ​​increase from to +.

1.4. Functiony = arcctgx

Consider the function
,
. (10)

This function is defined for all values ​​lying within the interval from 0 to ; it does not exist at the ends of this interval, since the values ​​of and are the discontinuity points of the cotangent. In the interval (0,) the function is monotonic (decreases from to), therefore, for the function (1) there is an inverse function

, (11)

those. to each given value (cotangent value) from the interval (
) corresponds to one well-defined value (the magnitude of the arc) from the interval (0,). Turning to the generally accepted notation, we get connected by the relation. Abstract >> Mathematics by trigonometric functions. To reverse trigonometric functions usually referred to as six functions: arcsine...

The dialectic of the development of the concept functions in school mathematics

Thesis >> Pedagogy

... . Reverse trigonometric functions. The main goal is to study the properties trigonometric functions, teach students to build their graphs. First trigonometric function ...

How did the concept originate and develop? functions

Abstract >> Mathematics

How does this equation include reverse trigonometric function, the cycloid is not algebraic... and also the notation trigonometric) reverse trigonometric, exponential and logarithmic functions. Such functions called elementary. Soon...