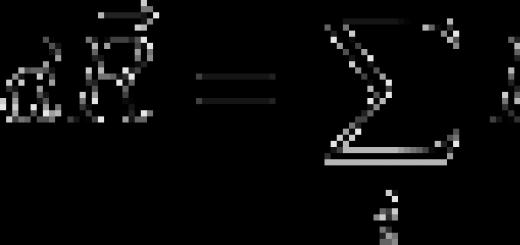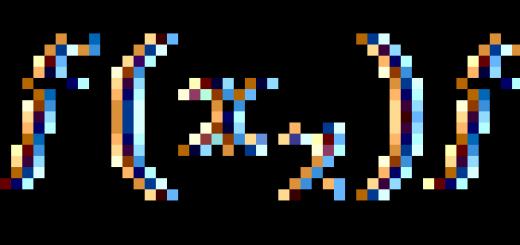Work BUT - scalar physical quantity, measured by the product of the module of the force acting on the body, by the module of its displacement under the action of this force and by the cosine of the angle between the force and displacement vectors:

Modulus of displacement of the body, under the action of force,

The work done by the force

On charts in axes F-S(Fig. 1) the work of the force is numerically equal to the area of ​​the figure bounded by the graph, the axis of displacement and straight lines parallel to the axis of the force.

If several forces act on the body, then in the work formula F- this is not the resultant ma of all these forces, but precisely the force that does the work. If the locomotive pulls the cars, then this force is the traction force of the locomotive, if a body is lifted on the rope, then this force is the rope tension force. It can be both the force of gravity and the force of friction, if the condition of the problem deals with the work of these forces.

Example 1. A body with a mass of 2 kg under the action of a force F moves up the inclined plane by a distance The distance of the body from the Earth's surface increases by .

Force vector F directed parallel to the inclined plane, the modulus of force F is equal to 30 N. What work did the force do during this displacement in the reference frame associated with the inclined plane F? Acceleration of free fall, take equal , coefficient of friction

Solution: The work of a force is defined as the scalar product of the force vector and the displacement vector of the body. Therefore, the strength F when lifting the body up the inclined plane did the work.

If in the condition of the problem in question about the coefficient of performance (COP) of any mechanism, it is necessary to think about what kind of work done by it is useful, and what is spent.

Efficiency of the mechanism (COP) η called the ratio of the useful work done by the mechanism to all the work expended in this case.

Useful work is that which needs to be done, and expended is that which actually has to be done.

Example 2. Let a body of mass m need to be lifted to a height h, while moving it along an inclined plane of length l under the influence of traction F thrust. In this case, the useful work is equal to the product of the force of gravity and the height of the lift:

And the work expended will be equal to the product of the traction force and the length of the inclined plane:

So, the efficiency of the inclined plane is equal to:

Comment: The efficiency of any mechanism cannot be more than 100% - the golden rule of mechanics.

Power N (W) is a quantitative measure of the speed of doing work. Power is equal to the ratio of work to the time for which it is done:

Power is a scalar quantity.

If the body moves uniformly, then we get:

Where is the speed of uniform motion.

in electric or electronic circuit There are two types of elements: passive and active. The active element is able to continuously supply energy to the circuit - battery, generator. Passive elements - resistors, capacitors, inductors, only consume energy.

What is a current source

A power source is a device that continuously supplies electricity to a circuit. It can be a source of direct current and alternating current. Rechargeable batteries are direct current sources, and the electrical outlet is alternating.

One of most interesting characteristics power sources– they are able to convert non-electrical energy into electrical energy, for example:

• chemical in batteries;
• mechanical in generators;
• solar, etc.

Electrical sources are divided into:

1. Independent;
2. Dependent (controlled), the output of which depends on the voltage or current elsewhere in the circuit, which can be either constant or changing over time. Used as equivalent IP for electronic devices.

When talking about circuit laws and analysis, electrical power supplies are often viewed as ideal, that is, theoretically capable of providing an infinite amount of energy without loss, while having the characteristics represented by a straight line. However, in real, or practical, sources, there is always an internal resistance that affects their output.

Important! Power supplies can only be connected in parallel if they have the same voltage value. The series connection will affect the output voltage rating.

The internal resistance of the power supply is represented as being connected in series with the circuit.

Current source power and internal resistance

Consider a simple circuit in which a battery has an EMF E and an internal resistance r and supplies current I to an external resistor of resistance R. The external resistor can be any resistive load. The main purpose of the circuit is to transfer energy from the battery to the load, where it does something useful, like lighting a room.

You can derive the dependence of useful power on resistance:

1. The equivalent resistance of the circuit is R + r (since the load resistance is connected in series with the external load);
2. The current flowing in the circuit will be determined by the expression:

1. EMF output power:

Rout. = E x I = E²/(R + r);

1. Power dissipated as heat, with internal battery resistance:

Pr = I² x r = E² x r/(R + r)²;

1. Power transferred to the load:

P(R) = I² x R = E² x R/(R + r)²;

1. Rout. = Pr + P(R).

Thus, some of the battery's output energy is immediately lost due to heat dissipation on the internal resistance.

Now you can plot P(R) versus R and find out at what load the useful power will take on a maximum value. When analyzing the function for an extremum, it turns out that as R increases, P(R) will also increase monotonically until the point when R does not equal r. At this point, the useful power will be maximum, and then begins to decrease monotonically with a further increase in R.

P(R)max = E²/4r when R = r. In this case, I = E/2r.

Important! This is a very significant result in electrical engineering. Power transfer between the power supply and an external load is most efficient when the load resistance matches the internal resistance of the current source.

If the load resistance is too high, then the current flowing through the circuit is small enough to transfer energy to the load at an appreciable rate. If the load resistance is too low, then most of the output energy is dissipated as heat within the power supply itself.

This condition is called agreement. One example of matching source impedance and external load is an audio amplifier and loudspeaker. The output impedance Zout of the amplifier is set from 4 to 8 ohms, and the nominal input impedance of the speaker Zin is only 8 ohms. Then, if an 8 ohm speaker is connected to the output of the amplifier, it will see the speaker as an 8 ohm load. Connecting two 8 ohm speakers in parallel to each other is equivalent to an amplifier driving a single 4 ohm speaker, and both configurations are within the amplifier's output specifications.

Current source efficiency

When doing work electric shock energy transformations take place. The full work done by the source goes to energy conversion in the entire electrical circuit, and the useful work is only in the circuit connected to the IP.

A quantitative assessment of the efficiency of the current source is carried out according to the most significant indicator that determines the speed of work, – power:

Not all the output power of the IP is used by the energy consumer. The ratio of energy consumed and issued by the source is the formula for the efficiency factor:

η = useful power/output power = Ppol/Pout

Important! Since Ppol. in almost any case, it is less than Pout, η cannot be greater than 1.

This formula can be transformed by substituting expressions for powers:

1. Source output power:

Rout. = I x E = I² x (R + r) x t;

1. Consumed energy:

Rpol. = I x U = I² x R x t;

1. Coefficient:

η = Рpol./Рout. = (I² x R x t)/(I² x (R + r) x t) = R/(R + r).

That is, for a current source, the efficiency is determined by the ratio of resistances: internal and load.

Often, the efficiency indicator is used as a percentage. Then the formula will take the form:

η = R/(R + r) x 100%.

It can be seen from the expression obtained that, subject to the matching condition (R = r), the coefficient η = (R/2 x R) x 100% = 50%. When the transmitted energy is most efficient, the efficiency of the IP itself is only 50%.

Using this coefficient, the efficiency of various IP and electricity consumers is evaluated.

Examples of efficiency values:

• gas turbine - 40%;
• solar battery - 15-20%;
• lithium-ion battery - 89-90%;
• electric heater - close to 100%;
• incandescent lamp - 5-10%;
• LED lamp - 5-50%;
• refrigeration units - 20-50%.

Useful power indicators are calculated for different consumers depending on the type of work performed.

Video

In reality, the work done with the help of any device is always more useful work, since part of the work is done against the friction forces that act inside the mechanism and when moving its individual parts. So, using a movable block, make extra work, lifting the block itself and the rope and, overcoming the friction forces in the block.

We introduce the following notation: we denote useful work by $A_p$, and complete work by $A_(poln)$. In doing so, we have:

Definition

Coefficient of performance (COP) called the ratio of useful work to full. We denote the efficiency by the letter $\eta $, then:

\[\eta =\frac(A_p)(A_(poln))\ \left(2\right).\]

Most often, the efficiency is expressed as a percentage, then its definition is the formula:

\[\eta =\frac(A_p)(A_(poln))\cdot 100\%\ \left(2\right).\]

When creating mechanisms, they try to increase their efficiency, but mechanisms with an efficiency equal to one(and even more so more than one) does not exist.

And so, the efficiency factor is a physical quantity that shows the share that useful work is from all the work done. With the help of efficiency, the efficiency of a device (mechanism, system) that converts or transmits energy that performs work is evaluated.

To increase the efficiency of mechanisms, you can try to reduce the friction in their axes, their mass. If friction can be neglected, the mass of the mechanism is significantly less than the mass, for example, of the load that the mechanism lifts, then the efficiency is slightly less than unity. Then the work done is approximately equal to the useful work:

The golden rule of mechanics

It must be remembered that a gain in work cannot be achieved using a simple mechanism.

Let us express each of the works in formula (3) as the product of the corresponding force by the path traveled under the influence of this force, then we transform formula (3) into the form:

Expression (4) shows that using a simple mechanism, we gain in strength as much as we lose on the way. This law is called the "golden rule" of mechanics. This rule was formulated in ancient greece Hero of Alexandria.

This rule does not take into account the work to overcome friction forces, therefore it is approximate.

Efficiency in power transmission

The efficiency factor can be defined as the ratio of useful work to the energy expended on its implementation ($Q$):

\[\eta =\frac(A_p)(Q)\cdot 100\%\ \left(5\right).\]

To calculate the efficiency of a heat engine, the following formula is used:

\[\eta =\frac(Q_n-Q_(ch))(Q_n)\left(6\right),\]

where $Q_n$ is the amount of heat received from the heater; $Q_(ch)$ - the amount of heat transferred to the refrigerator.

The efficiency of an ideal heat engine that operates according to the Carnot cycle is:

\[\eta =\frac(T_n-T_(ch))(T_n)\left(7\right),\]

where $T_n$ - heater temperature; $T_(ch)$ - refrigerator temperature.

Examples of tasks for efficiency

Example 1

Exercise. The crane engine has a power of $N$. For a time interval equal to $\Delta t$, he lifted a load of mass $m$ to a height $h$. What is the efficiency of the crane?\textit()

Decision. Useful work in the problem under consideration is equal to the work of lifting the body to a height $h$ of a load of mass $m$, this is the work of overcoming the force of gravity. It is equal to:

The total work that is done when lifting a load can be found using the definition of power:

Let's use the definition of the efficiency factor to find it:

\[\eta =\frac(A_p)(A_(poln))\cdot 100\%\left(1.3\right).\]

We transform formula (1.3) using expressions (1.1) and (1.2):

\[\eta =\frac(mgh)(N\Delta t)\cdot 100\%.\]

Answer.$\eta =\frac(mgh)(N\Delta t)\cdot 100\%$

Example 2

Exercise. Ideal gas executes a Carnot cycle, while the efficiency of the cycle is equal to $\eta $. What is the work in a gas compression cycle at constant temperature? The work done by the gas during expansion is $A_0$

Decision. The efficiency of the cycle is defined as:

\[\eta =\frac(A_p)(Q)\left(2.1\right).\]

Consider the Carnot cycle, determine in which processes heat is supplied (it will be $Q$).

Since the Carnot cycle consists of two isotherms and two adiabats, we can immediately say that there is no heat transfer in adiabatic processes (processes 2-3 and 4-1). In isothermal process 1-2 heat is supplied (Fig.1 $Q_1$), in isothermal process 3-4 heat is removed ($Q_2$). It turns out that in expression (2.1) $Q=Q_1$. We know that the amount of heat (the first law of thermodynamics) supplied to the system during an isothermal process goes completely to perform work by the gas, which means:

The gas performs useful work, which is equal to:

The amount of heat that is removed in the isothermal process 3-4 is equal to the work of compression (the work is negative) (since T=const, then $Q_2=-A_(34)$). As a result, we have:

We transform the formula (2.1) taking into account the results (2.2) - (2.4):

\[\eta =\frac(A_(12)+A_(34))(A_(12))\to A_(12)\eta =A_(12)+A_(34)\to A_(34)=( \eta -1)A_(12)\left(2.4\right).\]

Since by condition $A_(12)=A_0,\ $finally we get:

Answer.$A_(34)=\left(\eta -1\right)A_0$

1.15.1. The work of a force on a straight section of the path.

1.15.2. The work of a variable force on a curved path. Graphic image work.

1.15.3. Theorem on the work of the resultant.

1.15.4. Power. Efficiency.

1.15.5. The work and power of the force applied to solid body rotating around a fixed axis.

1.15.1. Let the point M a body to which a force constant in magnitude and direction is applied , moves straight from position M into position M"(Fig. 1.15.1.), Moreover, the angle between the direction of the force and the direction of movement of the point is equal, and the path traveled by the point is equal to S.

Strength can be decomposed into two components: normal not doing work, and tangent , whose modulus .

Since only the second component does the work, the work of the force will be equal to

The work of a constant force during rectilinear movement of its point of application is equal to the product of the modulus of force by the length of the path traveled by its point of application, and by the cosine of uela between the direction of the force and the direction of motion of its point of application.

The work of a force is a scalar quantity, that is, it is completely determined by its numerical value and sign.

It can be seen from formula (1.15.1.) that

1) if , then (forces whose direction is sharp corner with the direction of movement of their point of application, do positive work);

2) if , then (forces whose direction makes an obtuse angle with the direction of motion of their point of application do negative work);

3) if or , then .

The unit of work in the International System of Units (SI) is the work of a force of 1 N when it moves a body a distance of 1 m in the direction of the force. This unit is called the joule (J for short).

The concept of work (sometimes called mechanical work) established in mechanics arose from everyday experience. However, it should be noted that it does not always coincide with what is meant by work from a physiological point of view. So, a person who motionlessly holds a heavy load on outstretched arms, obviously does not commit any mechanical work(S = 0), but from the physiological point of view, it performs, of course, a certain (with a large weight of the load and very significant) work.

1.15.2. Using the concept of the work of a constant force on a straight path established in the previous paragraph, we proceed to the calculation of the work of a force in the most general case.

Let the application point M variable in modulus and direction of force moves from position A to position B , while describing some curvilinear trajectory (Fig. 1.15.2.). Let's break the path , traversed by a point, into a very large number n of such small sections that, without a large error, each such section can be considered rectilinear, and the force acting on this section is constant both in absolute value and in direction. Denote by constant values ​​of the modulus of variable force for given sections of the path , through - the lengths of the corresponding (rectilinear) sections of the track and through -angles between the corresponding directions of force and velocity of the point of its application.

Complete work of the variable force on the final path, AB will obviously be equal to the sum of the work on all its individual sections:

It is clear that than more segments n we will divide the path traveled by the point of application of the variable force , the more precisely the work of this force on a given path is calculated. In the limit, when the number of sections n becomes infinitely large, the length of each of them becomes an infinitely small value.

The work of a force on an infinitesimal displacement of its point of application is called elementary work. Denoting the elementary work of force through and the length of an infinitesimal element of the path through dS,will have

. (1.15.2.)

Then work all the way

The work of a variable force on a finite path is equal to the integral of the elementary work of a given force, calculated within the limits of the change in the path of the point of application of the force.

Now, noticing that the calculation of this integral in many cases presents significant difficulties, let's move on to a simpler and often used in technology graphical method for calculating the work of a variable force.

Let the point M applications of a variable in modulus and in direction of force moves from position to the position , which are determined on its trajectory by the corresponding distances and counted from some beginning O(Fig. 1.15.3.).

Let's take a rectangular coordinate system (Fig. 1.15.3.) And on the selected scales we will postpone: along the abscissa axis, the distance s of the point from the origin, and along the ordinate axis, the corresponding value of the force projection to the direction of the point speed M its applications, i.e., the algebraic value of the tangent component of a given force .

Connecting points with given coordinates s and F t of a continuous curve, we obtain a dependence graph .

The work of the force on its path S will be depicted on the appropriate scale by the area of ​​the figure(Fig. 1.15.3.), bounded by the abscissa axis, a curve and two ordinates corresponding to the initial and final position of the force application point.

When calculating the work of force graphically it is necessary, of course, to take into account the scale in which they were plotted on the chart distances s and the corresponding values ​​of the modulus of force F t.

1.15.3. Theorem. The work of the resultant of several forces on a certain path is equal to algebraic sum the work of the component forces on the same path:

where = is the resultant of forces.

1.15.4. Power force is a value that characterizes the speed with which work is done by this force at a given moment in time.

Average power force for a certain period of time t is equal to the ratio of the work A performed by it during this time to a given period of time:

Power R force at a given time t is equal to the ratio of the elementary work dA of the force for an infinitely small period of time, starting at the moment t, to the value dt of this period of time:

The SI unit of power is the power at which work of 1 joule is done in 1 second. This unit of power is called a watt (Watt for short).

1 W=1 J/s.

The power formula (1.15.4.) at the moment can be given a different form if we substitute into it the previously established [formula (1.15.2.)] expression of elementary work:

The power of the force at a given moment is equal to the product of the module of the given force corresponding to this moment of time, the module of the velocity of the point of its application and the cosine of the angle between the directions of the force and the velocity of the point of its application.

During the operation of any machine, part of the power consumed by it is spent not on doing useful work, but on overcoming the so-called harmful resistances that inevitably arise during the operation of the machine. So, for example, the power consumed by a lathe is spent not only on performing useful work - removing chips, but also on overcoming friction in the moving parts of machines and resistance to their movement from the air.

The ratio of the useful power P P of the machine to the power consumed by it P or the ratio of useful work for some specific period of time to all the work expended A for the same period of time is called mechanical efficiency.

Denoting, as is usually accepted, the coefficient of performance (abbreviated efficiency) with the Greek letter (this one), we will have

Efficiency is one of the most important characteristics of the machine, showing how rationally the power consumed by it is used.

Completely harmful resistances can never be eliminated, and therefore The efficiency is always less than unity.

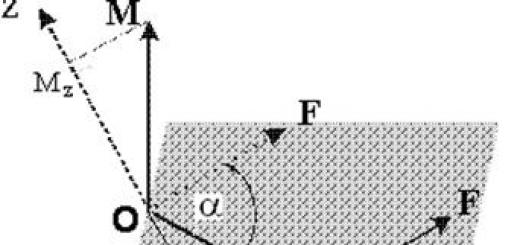
1.15.5. Let at some point M of a rigid body rotating around a fixed axis z (Fig. 1.15.4.), a force is applied . We decompose this force into two mutually perpendicular components: , lying in the plane P, perpendicular to the axis z body rotation, and , perpendicular to this plane, i.e. parallel to the z-axis

The power P of the force applied to a rotating body is equal to the product of the torque of this force times angular velocity body.

Questions for self-examination.

1. What is called the elementary work of force?

2. Give the definition of the work of force on the final segment of the path.

3. Formulate a theorem on the work of the resultant system of forces.

4. How is the work of a constant force vector calculated on a straight section of the path?

5. Define the power of force.

6. What is called efficiency?

7. How is the work and power of the force applied to a body with an axis of rotation calculated?

Power is essentially the rate at which work is done. The greater the power of the work done, the more work is done per unit of time.

Average power is the work done per unit of time.

The amount of power is directly proportional to the amount of work done \( A\) and inversely proportional to time \( t\) for which the work was done.

Power \( N\) is determined by the formula:

The unit of power in the system \ (SI \) is \ (Watt \) (Russian designation - \ (W \), international - \ (W \)).

To determine the engine power of cars and other vehicles, a historically more ancient unit of measurement is used - Horsepower (hp), 1 HP = 736 W.

Example:

The engine power of a car is approximately \(90 hp \u003d 66240 W\).

The power of a car or other vehicle can be calculated if the traction force of the car is known \( F\) and its speed ( v).

This formula is obtained by converting the basic formula for determining power.

Not a single device is capable of using \(100\)% of the energy initially supplied to it to perform useful work. Therefore, an important characteristic of any device is not only power, but also efficiency , which shows how efficiently the energy supplied to the device is used.

Example:

In order for a car to move, the wheels must turn. And in order for the wheels to rotate, the engine must drive the crank mechanism (the mechanism that converts the reciprocating movement of the engine piston into rotational movement of the wheels). In this case, the gears are brought into rotation and most of the energy is released in the form of heat into the surrounding space, resulting in a loss of input energy. The efficiency of the car engine is within \ (40 - 45 \)%. Thus, it turns out that only about \(40\)% of the total gasoline that a car is filled with goes to perform the useful work we need - moving the car.

If we put \(20\) liters of gasoline into the tank of the car, then only \(8\) liters will be spent on moving the car, and \(12\) liters will be burned without doing useful work.

The efficiency is denoted by the letter of the Greek alphabet \(“this”\) η , it is the ratio of useful power \( N\) to full or total power N full.

To determine it, use the formula: η = N N full. Since, by definition, efficiency is a ratio of powers, it does not have a unit of measurement.

It is often expressed as a percentage. If the efficiency is expressed as a percentage, then the formula is used: η = N N full ⋅ 100%.