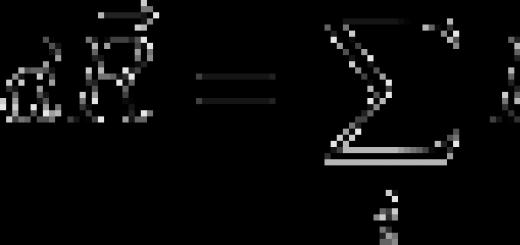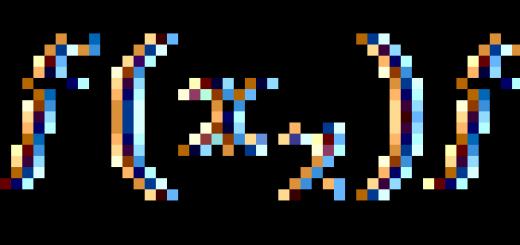Basic Laws of Dynamics. Newton's laws - first, second, third. Galileo's principle of relativity. The law of universal gravitation. Gravity. Forces of elasticity. The weight. Friction forces - rest, sliding, rolling + friction in liquids and gases.

You are here now: Kinematics. Basic concepts. Uniform rectilinear motion. Uniform movement. Uniform circular motion. Reference system. Trajectory, displacement, path, equation of motion, speed, acceleration, relationship between linear and angular velocity.

simple mechanisms. Lever (lever of the first kind and lever of the second kind). Block (fixed block and movable block). Inclined plane. Hydraulic Press. The golden rule of mechanics

Conservation laws in mechanics. Mechanical work, power, energy, law of conservation of momentum, law of conservation of energy, equilibrium of solids

Circular movement. Equation of motion in a circle. Angular velocity. Normal = centripetal acceleration. Period, frequency of circulation (rotation). Relationship between linear and angular velocity

Mechanical vibrations. Free and forced vibrations. Harmonic vibrations. Elastic oscillations. Mathematical pendulum. Energy transformations during harmonic vibrations

mechanical waves. Velocity and wavelength. Traveling wave equation. Wave phenomena (diffraction, interference...)

Hydromechanics and Aeromechanics. Pressure, hydrostatic pressure. Pascal's law. Basic equation of hydrostatics. Communicating vessels. Law of Archimedes. Sailing conditions tel. Fluid flow. Bernoulli's law. Torricelli formula

Molecular physics. Basic provisions of the ICT. Basic concepts and formulas. Properties of an ideal gas. Basic equation of the MKT. Temperature. The equation of state for an ideal gas. Mendeleev-Klaiperon equation. Gas laws - isotherm, isobar, isochore

Wave optics. Corpuscular-wave theory of light. Wave properties of light. dispersion of light. Light interference. Huygens-Fresnel principle. Diffraction of light. Light polarization

Thermodynamics. Internal energy. Job. Quantity of heat. Thermal phenomena. First law of thermodynamics. Application of the first law of thermodynamics to various processes. Heat balance equation. The second law of thermodynamics. Heat engines

Electrostatics. Basic concepts. Electric charge. The law of conservation of electric charge. Coulomb's law. The principle of superposition. The theory of close action. Electric field potential. Capacitor.

Constant electric current. Ohm's law for a circuit section. Operation and DC power. Joule-Lenz law. Ohm's law for a complete circuit. Faraday's law of electrolysis. Electrical circuits - serial and parallel connection. Kirchhoff's rules.

Electromagnetic vibrations. Free and forced electromagnetic oscillations. Oscillatory circuit. Alternating electric current. Capacitor in AC circuit. An inductor ("solenoid") in an alternating current circuit.

Electromagnetic waves. The concept of an electromagnetic wave. Properties of electromagnetic waves. wave phenomena

A magnetic field. Magnetic induction vector. The gimlet rule. Ampere's law and Ampere's force. Lorentz force. Left hand rule. Electromagnetic induction, magnetic flux, Lenz's rule, law of electromagnetic induction, self-induction, magnetic field energy

The quantum physics. Planck's hypothesis. The phenomenon of the photoelectric effect. Einstein's equation. Photons. Bohr's quantum postulates.

Elements of the theory of relativity. Postulates of the theory of relativity. Relativity of simultaneity, distances, time intervals. Relativistic law of addition of velocities. The dependence of mass on speed. The basic law of relativistic dynamics...

Errors of direct and indirect measurements. Absolute, relative error. Systematic and random errors. Standard deviation (error). Table for determining the errors of indirect measurements of various functions.

Uniformly accelerated motion is a motion in which the acceleration vector does not change in magnitude and direction. Examples of such movement: a bicycle that rolls down a hill; a stone thrown at an angle to the horizon. Uniform movement - special case uniformly accelerated motion with an acceleration equal to zero.

Let us consider the case of free fall (a body is thrown at an angle to the horizon) in more detail. Such movement can be represented as the sum of movements about the vertical and horizontal axes.

At any point of the trajectory, the free fall acceleration g → acts on the body, which does not change in magnitude and is always directed in one direction.

Along the X axis the motion is uniform and rectilinear, and along the Y axis it is uniformly accelerated and rectilinear. We will consider the projections of the velocity and acceleration vectors on the axis.

Formula for speed with uniformly accelerated motion:

Here v 0 is the initial speed of the body, a = c o n s t is the acceleration.

Let us show on the graph that with uniformly accelerated motion, the dependence v (t) has the form of a straight line.

​​​​​​​

Acceleration can be determined from the slope of the velocity graph. In the figure above, the acceleration module is equal to the ratio sides of triangle ABC.

a = v - v 0 t = B C A C

The larger the angle β, the greater the slope (steepness) of the graph with respect to the time axis. Accordingly, the greater the acceleration of the body.

For the first graph: v 0 = - 2 m s; a \u003d 0, 5 m s 2.

For the second graph: v 0 = 3 m s; a = - 1 3 m s 2 .

From this graph, you can also calculate the movement of the body in time t. How to do it?

Let's single out a small time interval ∆ t on the graph. We will assume that it is so small that the movement during the time ∆ t can be considered uniform movement with a speed, equal speed body in the middle of the interval ∆ t . Then, the displacement ∆ s during the time ∆ t will be equal to ∆ s = v ∆ t .

Let's divide all time t into infinitely small intervals ∆ t . The displacement s in time t is equal to the area of ​​the trapezoid O D E F .

s = O D + E F 2 O F = v 0 + v 2 t = 2 v 0 + (v - v 0) 2 t .

We know that v - v 0 = a t , so the final formula for moving the body will be:

s = v 0 t + a t 2 2

In order to find the coordinate of the body at a given time, you need to add displacement to the initial coordinate of the body. The change in coordinates depending on time expresses the law of uniformly accelerated motion.

Law of uniformly accelerated motion

Law of uniformly accelerated motion

y = y 0 + v 0 t + a t 2 2 .

Another common task of kinematics that arises in the analysis of uniformly accelerated motion is finding the coordinate at setpoints initial and final speeds and acceleration.

Eliminating t from the above equations and solving them, we obtain:

s \u003d v 2 - v 0 2 2 a.

From the known initial speed, acceleration and displacement, you can find the final speed of the body:

v = v 0 2 + 2 a s .

For v 0 = 0 s = v 2 2 a and v = 2 a s

Important!

The values ​​v , v 0 , a , y 0 , s included in the expressions are algebraic quantities. Depending on the nature of the movement and the direction of the coordinate axes in a particular task, they can take both positive and negative values.

If you notice a mistake in the text, please highlight it and press Ctrl+Enter

1) Analytical method.

We consider the highway to be straight. Let's write down the equation of motion of a cyclist. Since the cyclist was moving uniformly, his equation of motion is:

(the origin of coordinates is placed at the starting point, so the initial coordinate of the cyclist is zero).

The motorcyclist was moving at a uniform speed. He also started moving from the starting point, so his initial coordinate is zero, the initial speed of the motorcyclist is also equal to zero (the motorcyclist began to move from a state of rest).

Considering that the motorcyclist started moving a little later, the motorcyclist's equation of motion is:

In this case, the speed of the motorcyclist changed according to the law:

At the moment when the motorcyclist caught up with the cyclist, their coordinates are equal, i.e. or:

Solving this equation with respect to , we find the meeting time:

This is quadratic equation. We define the discriminant:

Define roots:

Substitute in formulas numerical values and calculate:

We discard the second root as not corresponding to the physical conditions of the problem: the motorcyclist could not catch up with the cyclist 0.37 s after the cyclist began to move, since he himself left the starting point only 2 s after the cyclist started.

Thus, the time when the motorcyclist caught up with the cyclist:

Substitute this value of time into the formula for the law of change in the speed of a motorcyclist and find the value of his speed at this moment:

2) Graphical way.

On one coordinate plane we build graphs of changes over time in the coordinates of the cyclist and motorcyclist (the graph for the coordinates of the cyclist is in red, for the motorcyclist - in green). It can be seen that the dependence of the coordinate on time for a cyclist is linear function, and the graph of this function is a straight line (the case of uniform rectilinear motion). The motorcyclist was moving with uniform acceleration, so the dependence of the motorcyclist’s coordinates on time is quadratic function, whose graph is a parabola.

What is uniformly accelerated motion?

Uniformly accelerated motion in physics is such a motion, the acceleration vector of which does not change in magnitude and direction. talking plain language, uniformly accelerated motion is uneven movement(that is, going at different speeds), whose acceleration is constant over a certain period of time. Imagine that starts moving, for the first 2 seconds its speed is 10 m / s, the next 2 seconds it is already moving at a speed of 20 m / s, and after another 2 seconds already at a speed of 30 m / s. That is, every 2 seconds it accelerates by 10 m / s, such a movement is uniformly accelerated.

From this we can derive an extremely simple definition of uniformly accelerated motion: this is the motion of any physical body, in which its speed changes in the same way for equal periods of time.

Examples of uniformly accelerated motion

A good example of uniformly accelerated motion in Everyday life it could be a bike going downhill (but not a bike driven by a cyclist), or a stone thrown at a certain angle to the horizon.

By the way, the example with a stone can be considered in more detail. At any point of the flight trajectory, the free fall acceleration g acts on the stone. The acceleration g does not change, that is, it remains constant and is always directed in one direction (in fact, this is the main condition for uniformly accelerated motion).

It is convenient to represent the flight of a thrown stone as a sum of movements relative to the vertical and horizontal axes of the coordinate system.

If along the X axis the movement of the stone will be uniform and rectilinear, then along the Y axis it will be uniformly accelerated and rectilinear.

Formula of uniformly accelerated motion

The formula for speed in uniformly accelerated motion will look like this:

Where V 0 is the initial speed of the body, and is the acceleration (as we remember, this value is a constant), t is the total flight time of the stone.

With uniformly accelerated motion, the dependence V(t) will look like a straight line.

Acceleration can be determined from the slope of the velocity graph. In this figure, it is equal to the ratio of the sides of the triangle ABC.

The larger the angle β, the greater the slope and, as a result, the steepness of the graph with respect to the time axis, and the greater will be the acceleration of the body.

• Sivukhin D.V. General course physics. - M.: Fizmatlit, 2005. - T. I. Mechanics. - S. 37. - 560 p. - ISBN 5-9221-0225-7.
• Targ S. M. Short Course theoretical mechanics. - 11th ed. - M .: " graduate School”, 1995. - S. 214. - 416 p. - ISBN 5-06-003117-9.

Uniformly accelerated motion, video

How, knowing the stopping distance, determine the initial speed of the car and how, knowing the characteristics of the movement, such as the initial speed, acceleration, time, determine the movement of the car? We will get answers after we get acquainted with the topic of today's lesson: "Displacement with uniformly accelerated movement, the dependence of coordinates on time with uniformly accelerated movement"

With uniformly accelerated motion, the graph looks like a straight line going up, since its acceleration projection is greater than zero.

With uniform rectilinear motion the area will be numerically equal to the module of the projection of the displacement of the body. It turns out that this fact can be generalized for the case not only of uniform motion, but also for any motion, that is, to show that the area under the graph is numerically equal to the displacement projection modulus. This is done strictly mathematically, but we will use a graphical method.

Rice. 2. Graph of the dependence of speed on time with uniformly accelerated movement ()

Let's divide the graph of the projection of speed from time for uniformly accelerated motion into small time intervals Δt. Let us assume that they are so small that during their length the speed practically did not change, that is, we will conditionally turn the linear dependence graph in the figure into a ladder. At each of its steps, we believe that the speed has not changed much. Imagine that we make the time intervals Δt infinitely small. In mathematics they say: we make a passage to the limit. In this case, the area of ​​such a ladder will indefinitely closely coincide with the area of ​​the trapezoid, which is limited by the graph V x (t). And this means that for the case of uniformly accelerated motion, we can say that the displacement projection module is numerically equal to area, bounded by the graph V x (t): the abscissa and ordinate axes and the perpendicular lowered to the abscissa axis, that is, the area of ​​the trapezoid OABS, which we see in Figure 2.

The task turns from a physical one into a math problem- Finding the area of ​​a trapezoid. This is the standard situation when physicists make up a model that describes a particular phenomenon, and then mathematics comes into play, which enriches this model with equations, laws - that turns the model into a theory.

We find the area of ​​the trapezoid: the trapezoid is rectangular, since the angle between the axes is 90 0, we divide the trapezoid into two shapes - a rectangle and a triangle. Obviously, the total area will be equal to the sum of the areas of these figures (Fig. 3). Let's find their areas: the area of ​​the rectangle is equal to the product of the sides, that is, V 0x t, the area right triangle will be equal to half the product of the legs - 1/2AD BD, substituting the projection values, we get: 1/2t (V x - V 0x), and, remembering the law of change in speed with time during uniformly accelerated motion: V x (t) = V 0x + a x t, it is quite obvious that the difference in the projections of the speeds is equal to the product of the projection of the acceleration a x by the time t, that is, V x - V 0x = a x t.

Rice. 3. Determining the area of ​​a trapezoid ( Source)

Taking into account the fact that the area of ​​the trapezoid is numerically equal to the displacement projection module, we get:

S x (t) \u003d V 0 x t + a x t 2 / 2

We have obtained the law of the dependence of the projection of displacement on time with uniformly accelerated motion in scalar form, in vector form it will look like this:

(t) = t + t 2 / 2

Let's derive one more formula for the displacement projection, which will not include time as a variable. We solve the system of equations, excluding time from it:

S x (t) \u003d V 0 x + a x t 2 / 2

V x (t) \u003d V 0 x + a x t

Imagine that we do not know the time, then we will express the time from the second equation:

t \u003d V x - V 0x / a x

Substitute the resulting value into the first equation:

We get such a cumbersome expression, we square it and give similar ones:

We have obtained a very convenient displacement projection expression for the case when we do not know the time of motion.

Let us have the initial speed of the car, when braking began, is V 0 \u003d 72 km / h, final speed V \u003d 0, acceleration a \u003d 4 m / s 2. Find out the length of the braking distance. Converting kilometers to meters and substituting the values ​​into the formula, we get that the stopping distance will be:

S x \u003d 0 - 400 (m / s) 2 / -2 4 m / s 2 \u003d 50 m

Let's analyze the following formula:

S x \u003d (V 0 x + V x) / 2 t

The projection of movement is half the sum of the projections of the initial and final speeds, multiplied by the time of movement. Recall the displacement formula for average speed

S x \u003d V cf t

In the case of uniformly accelerated motion average speed will:

V cf \u003d (V 0 + V k) / 2

We have come close to solving the main problem of the mechanics of uniformly accelerated motion, that is, obtaining the law according to which the coordinate changes with time:

x(t) \u003d x 0 + V 0 x t + a x t 2 / 2

In order to learn how to use this law, we will analyze a typical problem.

The car, moving from a state of rest, acquires an acceleration of 2 m / s 2. Find the distance traveled by the car in 3 seconds and in the third second.

Given: V 0 x = 0

Let us write down the law according to which the displacement changes with time at

uniformly accelerated motion: S x \u003d V 0 x t + a x t 2 /2. 2 c< Δt 2 < 3.

We can answer the first question of the problem by plugging in the data:

t 1 \u003d 3 c S 1x \u003d a x t 2 / 2 \u003d 2 3 2 / 2 \u003d 9 (m) - this is the path that went

c car in 3 seconds.

Find out how far he traveled in 2 seconds:

S x (2 s) \u003d a x t 2 / 2 \u003d 2 2 2 / 2 \u003d 4 (m)

So, you and I know that in two seconds the car drove 4 meters.

Now, knowing these two distances, we can find the path that he traveled in the third second:

S 2x \u003d S 1x + S x (2 s) \u003d 9 - 4 \u003d 5 (m)