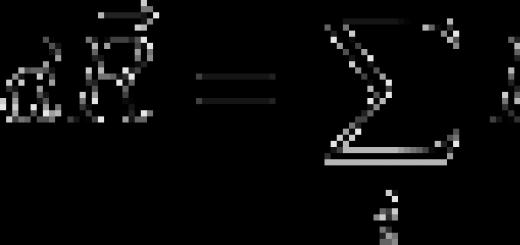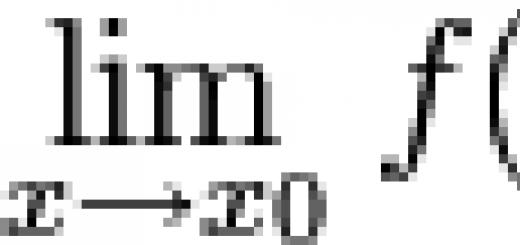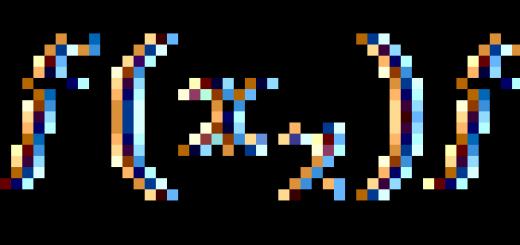Graph of the function y 2 5. Graph of the function. Fractional linear function and its graph

The construction of graphs of functions containing modules usually causes considerable difficulties for schoolchildren. However, everything is not so bad. It is enough to remember several algorithms for solving such problems, and you can easily plot even the most seemingly complex function. Let's see what these algorithms are.

1. Plotting the function y = |f(x)|

Note that the set of function values y = |f(x)| : y ≥ 0. Thus, the graphs of such functions are always located completely in the upper half-plane.

Plotting the function y = |f(x)| consists of the following simple four steps.

1) Construct carefully and carefully the graph of the function y = f(x).

2) Leave unchanged all points of the graph that are above or on the 0x axis.

3) The part of the graph that lies below the 0x axis, display symmetrically about the 0x axis.

Example 1. Draw a graph of the function y = |x 2 - 4x + 3|

1) We build a graph of the function y \u003d x 2 - 4x + 3. It is obvious that the graph of this function is a parabola. Let's find the coordinates of all points of intersection of the parabola with the coordinate axes and the coordinates of the vertex of the parabola.

x 2 - 4x + 3 = 0.

x 1 = 3, x 2 = 1.

Therefore, the parabola intersects the 0x axis at points (3, 0) and (1, 0).

y \u003d 0 2 - 4 0 + 3 \u003d 3.

Therefore, the parabola intersects the 0y axis at the point (0, 3).

Parabola vertex coordinates:

x in \u003d - (-4/2) \u003d 2, y in \u003d 2 2 - 4 2 + 3 \u003d -1.

Therefore, the point (2, -1) is the vertex of this parabola.

Draw a parabola using the received data (Fig. 1)

2) The part of the graph lying below the 0x axis is displayed symmetrically with respect to the 0x axis.

3) We get the graph of the original function ( rice. 2, shown by dotted line).

2. Plotting the function y = f(|x|)

Note that functions of the form y = f(|x|) are even:

y(-x) = f(|-x|) = f(|x|) = y(x). This means that the graphs of such functions are symmetrical about the 0y axis.

Plotting the function y = f(|x|) consists of the following simple chain of actions.

1) Plot the function y = f(x).

2) Leave that part of the graph for which x ≥ 0, that is, the part of the graph located in the right half-plane.

3) Display the part of the graph specified in paragraph (2) symmetrically to the 0y axis.

4) As the final graph, select the union of the curves obtained in paragraphs (2) and (3).

Example 2. Draw a graph of the function y = x 2 – 4 · |x| + 3

Since x 2 = |x| 2 , then the original function can be rewritten as follows: y = |x| 2 – 4 · |x| + 3. And now we can apply the algorithm proposed above.

1) We build carefully and carefully the graph of the function y \u003d x 2 - 4 x + 3 (see also rice. one).

2) We leave that part of the graph for which x ≥ 0, that is, the part of the graph located in the right half-plane.

3) Display the right side of the graph symmetrically to the 0y axis.

(Fig. 3).

Example 3. Draw a graph of the function y = log 2 |x|

We apply the scheme given above.

1) We plot the function y = log 2 x (Fig. 4).

3. Plotting the function y = |f(|x|)|

Note that functions of the form y = |f(|x|)| are also even. Indeed, y(-x) = y = |f(|-x|)| = y = |f(|x|)| = y(x), and therefore, their graphs are symmetrical about the 0y axis. The set of values of such functions: y ≥ 0. Hence, the graphs of such functions are located completely in the upper half-plane.

To plot the function y = |f(|x|)|, you need to:

1) Construct a neat graph of the function y = f(|x|).

2) Leave unchanged the part of the graph that is above or on the 0x axis.

3) The part of the graph located below the 0x axis should be displayed symmetrically with respect to the 0x axis.

4) As the final graph, select the union of the curves obtained in paragraphs (2) and (3).

Example 4. Draw a graph of the function y = |-x 2 + 2|x| – 1|.

1) Note that x 2 = |x| 2. Hence, instead of the original function y = -x 2 + 2|x| - one

you can use the function y = -|x| 2 + 2|x| – 1, since their graphs are the same.

We build a graph y = -|x| 2 + 2|x| – 1. For this, we use algorithm 2.

a) We plot the function y \u003d -x 2 + 2x - 1 (Fig. 6).

b) We leave that part of the graph, which is located in the right half-plane.

c) Display the resulting part of the graph symmetrically to the 0y axis.

d) The resulting graph is shown in the figure with a dotted line (Fig. 7).

2) There are no points above the 0x axis, we leave the points on the 0x axis unchanged.

3) The part of the graph located below the 0x axis is displayed symmetrically with respect to 0x.

4) The resulting graph is shown in the figure by a dotted line (Fig. 8).

Example 5. Plot the function y = |(2|x| – 4) / (|x| + 3)|

1) First you need to plot the function y = (2|x| – 4) / (|x| + 3). To do this, we return to algorithm 2.

a) Carefully plot the function y = (2x – 4) / (x + 3) (Fig. 9).

Note that this function is linear-fractional and its graph is a hyperbola. To build a curve, you first need to find the asymptotes of the graph. Horizontal - y \u003d 2/1 (the ratio of the coefficients at x in the numerator and denominator of a fraction), vertical - x \u003d -3.

2) The part of the chart that is above or on the 0x axis will be left unchanged.

3) The part of the chart located below the 0x axis will be displayed symmetrically with respect to 0x.

4) The final graph is shown in the figure (Fig. 11).

site, with full or partial copying of the material, a link to the source is required.

"Transformation of functions" - Seesaw. Move up the y-axis. Turn on the full volume - you will increase a (amplitude) of air vibrations. Shift along the x-axis to the left. Lesson objectives. 3 points. Music. Plot the function and determine D(f), E(f), and T: The x-compression. Move down the y-axis. Add red color to the palette - you will reduce k (frequency) of electromagnetic oscillations.

"Functions of several variables" - Derivatives of higher orders. A function of two variables can be represented graphically. Differential and integral calculus. Internal and boundary points. Determining the limit of a function of 2 variables. Course of mathematical analysis. Berman. Limit of a function of 2 variables. Function graph. Theorem. Limited area.

"The concept of a function" - Methods for plotting graphs quadratic function. Learning different ways to define a function is an important methodological technique. Features of the study of a quadratic function. Genetic interpretation of the concept of "function". Functions and graphs in the school course of mathematics. The concept of a linear function is highlighted when plotting a graph of some linear function.

"Theme Function" - Analysis. It is necessary to find out not what the student does not know, but what he knows. Laying the groundwork for successful delivery USE and admission to universities. Synthesis. If the students work in different ways, then the teacher should work with them in different ways. Analogy. Generalization. Distribution of USE tasks by main blocks of content school course mathematics.

"Transformation of graphs of functions" - Repeat the types of transformations of graphs. Associate each graph with a function. Symmetry. Lesson Objective: Graphing complex functions. Consider examples of transformations, explain each type of transformation. Transformation of graphs of functions. Stretching. Fix the construction of graphs of functions using transformations of graphs of elementary functions.

"Graphs of functions" - View function. The function range is all values of the dependent variable y. The graph of the function is a parabola. The graph of the function is a cubic parabola. The graph of the function is a hyperbola. The scope and range of the function. Correlate each straight line with its equation: The domain of the function is all values of the independent variable x.

Build a function

We bring to your attention a service for plotting function graphs online, all rights to which belong to the company Desmos. Use the left column to enter functions. You can enter it manually or with virtual keyboard at the bottom of the window. To enlarge the chart window, you can hide both the left column and the virtual keyboard.

Benefits of online charting

Visual display of introduced functions
Building very complex graphs
Plotting implicitly defined graphs (e.g. ellipse x^2/9+y^2/16=1)
The ability to save charts and get a link to them, which becomes available to everyone on the Internet
Scale control, line color
The ability to plot graphs by points, the use of constants
Construction of several graphs of functions at the same time
Plotting in polar coordinates (use r and θ(\theta))

With us it is easy to build graphs of varying complexity online. The construction is done instantly. The service is in demand for finding intersection points of functions, for displaying graphs for their further transfer to a Word document as illustrations for solving problems, for analyzing the behavioral features of function graphs. The best browser for working with charts on this page of the site is Google Chrome. When using other browsers, correct operation is not guaranteed.

"Natural logarithm" - 0.1. natural logarithms. 4. "Logarithmic darts". 0.04. 7.121.

"Power function grade 9" - U. Cubic parabola. Y = x3. Grade 9 teacher Ladoshkina I.A. Y = x2. Hyperbola. 0. Y \u003d xn, y \u003d x-n where n is the given natural number. X. The exponent is an even natural number (2n).

"Quadratic function" - 1 Quadratic function definition 2 Function properties 3 Function graphs 4 Quadratic inequalities 5 Conclusion. Properties: Inequalities: Prepared by Andrey Gerlitz, a student of grade 8A. Plan: Graph: -Intervals of monotonicity at a > 0 at a< 0. Квадратичная функция. Квадратичные функции используются уже много лет.

"Quadratic function and its graph" - Decision. y \u003d 4x A (0.5: 1) 1 \u003d 1 A-belongs. When a=1, the formula y=ax takes the form.

"Class 8 quadratic function" - 1) Construct the top of the parabola. Plotting a quadratic function. x. -7. Plot the function. Algebra Grade 8 Teacher 496 school Bovina TV -1. Construction plan. 2) Construct the axis of symmetry x=-1. y.

1. Linear fractional function and its graph

A function of the form y = P(x) / Q(x), where P(x) and Q(x) are polynomials, is called a fractional rational function.

With a concept rational numbers you are probably already familiar with. Similarly rational functions are functions that can be represented as a quotient of two polynomials.

If a fractional rational function is a quotient of two linear functions - polynomials of the first degree, i.e. view function

y = (ax + b) / (cx + d), then it is called fractional linear.

Note that in the function y = (ax + b) / (cx + d), c ≠ 0 (otherwise the function becomes linear y = ax/d + b/d) and that a/c ≠ b/d (otherwise the function is a constant ). The linear-fractional function is defined for all real numbers, except for x = -d/c. Graphs of linear-fractional functions do not differ in form from the graph you know y = 1/x. The curve that is the graph of the function y = 1/x is called hyperbole. With an unlimited increase in x by absolute value the function y = 1/x decreases in absolute value indefinitely and both branches of the graph approach the abscissa axis: the right one approaches from above, and the left one from below. The lines approached by the branches of a hyperbola are called its asymptotes.

Example 1

y = (2x + 1) / (x - 3).

Solution.

Let's select the integer part: (2x + 1) / (x - 3) = 2 + 7 / (x - 3).

Now it is easy to see that the graph of this function is obtained from the graph of the function y = 1/x by the following transformations: shift by 3 unit segments to the right, stretch along the Oy axis by 7 times and shift by 2 unit segments up.

Any fraction y = (ax + b) / (cx + d) can be written in the same way, highlighting the “whole part”. Consequently, the graphs of all linear-fractional functions are hyperbolas shifted along the coordinate axes in various ways and stretched along the Oy axis.

To plot a graph of some arbitrary linear-fractional function, it is not at all necessary to transform the fraction that defines this function. Since we know that the graph is a hyperbola, it will be enough to find the lines to which its branches approach - the hyperbola asymptotes x = -d/c and y = a/c.

Example 2

Find the asymptotes of the graph of the function y = (3x + 5)/(2x + 2).

Solution.

The function is not defined, for x = -1. Hence, the line x = -1 serves as a vertical asymptote. To find the horizontal asymptote, let's find out what the values of the function y(x) approach when the argument x increases in absolute value.

To do this, we divide the numerator and denominator of the fraction by x:

y = (3 + 5/x) / (2 + 2/x).

As x → ∞ the fraction tends to 3/2. Hence, the horizontal asymptote is the straight line y = 3/2.

Example 3

Plot the function y = (2x + 1)/(x + 1).

Solution.

We select the “whole part” of the fraction:

(2x + 1) / (x + 1) = (2x + 2 - 1) / (x + 1) = 2(x + 1) / (x + 1) - 1/(x + 1) =

2 – 1/(x + 1).

Now it is easy to see that the graph of this function is obtained from the graph of the function y = 1/x by the following transformations: a shift of 1 unit to the left, a symmetric display with respect to Ox, and a shift of 2 unit intervals up along the Oy axis.

Domain of definition D(y) = (-∞; -1)ᴗ(-1; +∞).

Range of values E(y) = (-∞; 2)ᴗ(2; +∞).

Intersection points with axes: c Oy: (0; 1); c Ox: (-1/2; 0). The function increases on each of the intervals of the domain of definition.

Answer: figure 1.

2. Fractional-rational function

Consider a fractional rational function of the form y = P(x) / Q(x), where P(x) and Q(x) are polynomials of degree higher than the first.

Examples of such rational functions:

y \u003d (x 3 - 5x + 6) / (x 7 - 6) or y \u003d (x - 2) 2 (x + 1) / (x 2 + 3).

If the function y = P(x) / Q(x) is a quotient of two polynomials of degree higher than the first, then its graph will, as a rule, be more complicated, and it can sometimes be difficult to build it exactly, with all the details. However, it is often enough to apply techniques similar to those with which we have already met above.

Let the fraction be proper (n< m). Известно, что любую несократимую рациональную дробь можно представить, и притом единственным образом, в виде суммы finite number elementary fractions, the form of which is determined by expanding the denominator of the fraction Q(x) into a product of real factors:

P(x) / Q(x) \u003d A 1 / (x - K 1) m1 + A 2 / (x - K 1) m1-1 + ... + A m1 / (x - K 1) + ... +

L 1 /(x – K s) ms + L 2 /(x – K s) ms-1 + … + L ms /(x – K s) + …+

+ (B 1 x + C 1) / (x 2 +p 1 x + q 1) m1 + … + (B m1 x + C m1) / (x 2 +p 1 x + q 1) + …+

+ (M 1 x + N 1) / (x 2 + p t x + q t) m1 + ... + (M m1 x + N m1) / (x 2 + p t x + q t).

Obviously, the graph of a fractional rational function can be obtained as the sum of graphs of elementary fractions.

Plotting fractional rational functions

Consider several ways to plot a fractional-rational function.

Example 4

Plot the function y = 1/x 2 .

Solution.

We use the graph of the function y \u003d x 2 to plot the graph y \u003d 1 / x 2 and use the method of "dividing" the graphs.

Domain D(y) = (-∞; 0)ᴗ(0; +∞).

Range of values E(y) = (0; +∞).

There are no points of intersection with the axes. The function is even. Increases for all x from the interval (-∞; 0), decreases for x from 0 to +∞.

Answer: figure 2.

Example 5

Plot the function y = (x 2 - 4x + 3) / (9 - 3x).

Solution.

Domain D(y) = (-∞; 3)ᴗ(3; +∞).

y \u003d (x 2 - 4x + 3) / (9 - 3x) \u003d (x - 3) (x - 1) / (-3 (x - 3)) \u003d - (x - 1) / 3 \u003d -x / 3 + 1/3.

Here we used the technique of factoring, reduction and reduction to a linear function.

Answer: figure 3.

Example 6

Plot the function y \u003d (x 2 - 1) / (x 2 + 1).

Solution.

The domain of definition is D(y) = R. Since the function is even, the graph is symmetrical about the y-axis. Before plotting, we again transform the expression by highlighting the integer part:

y \u003d (x 2 - 1) / (x 2 + 1) \u003d 1 - 2 / (x 2 + 1).

Note that the selection of the integer part in the formula of a fractional-rational function is one of the main ones when plotting graphs.

If x → ±∞, then y → 1, i.e., the line y = 1 is a horizontal asymptote.

Answer: figure 4.

Example 7

Consider the function y = x/(x 2 + 1) and try to find exactly its largest value, i.e. most high point right half of the graph. To accurately build this graph, today's knowledge is not enough. It is obvious that our curve cannot "climb" very high, since the denominator quickly begins to “overtake” the numerator. Let's see if the value of the function can be equal to 1. To do this, you need to solve the equation x 2 + 1 \u003d x, x 2 - x + 1 \u003d 0. This equation does not have real roots. So our assumption is wrong. To find the most great importance function, you need to find out for which largest A the equation A \u003d x / (x 2 + 1) will have a solution. Let's replace the original equation with a quadratic one: Ax 2 - x + A = 0. This equation has a solution when 1 - 4A 2 ≥ 0. From here we find highest value A = 1/2.