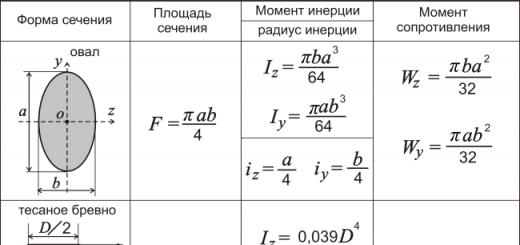
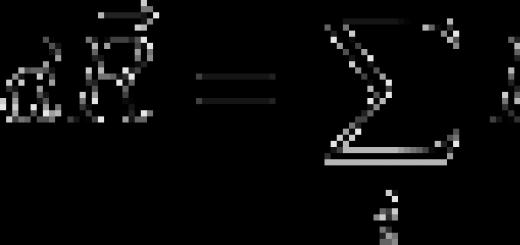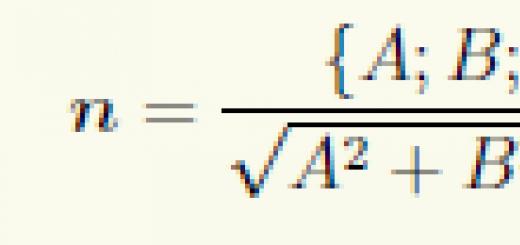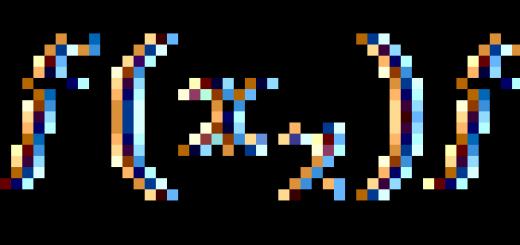The problem is posed as follows: let the desired value z determined in terms of other quantities a, b, c, ... obtained from direct measurements
z = f(a,b,c,...) (1.11)
It is necessary to find the mean value of the function and the error of its measurements, i.e. find confidence interval
with reliability a and relative error.
As for, it is found by substituting into the right side of (11) instead of a, b, c,... their mean values
3. Estimate the half-width of the confidence interval for the result of indirect measurements
,
where derivatives... are calculated at
4. Determine the relative error of the result
5. If the dependence of z on a, b, c,... has the form 
, where k,l,m are any real numbers, you must first find relative mistake
and then absolute .
6. Write the final result as
z = ± Dz , ε = …% for a = … .
Note:
When processing the results of direct measurements, the following rule should be followed: numerical values of all calculated values must contain one digit more than the initial (experimentally determined) values.
For indirect measurements, calculations should be made according to rules of approximation:
Rule 1 When adding and subtracting approximate numbers, you must:
a) highlight the term in which the doubtful figure has the highest digit;
b) round all other terms to the next digit (one spare digit is kept);
c) perform addition (subtraction);
d) as a result, discard the last digit by rounding (the digit of the doubtful digit of the result coincides with the highest of the digits of the doubtful digits of the terms).
Example: 5.4382 10 5 - 2.918 10 3 + 35.8 + 0.064.
In these numbers, the last significant digits are doubtful (wrong ones have already been discarded). We write them in the form 543820 - 2918 + 35.8 + 0.064.
It can be seen that in the first term, the dubious number 2 has the highest digit (tens). Rounding all other numbers up to the next digit and adding, we get
543820 - 2918 + 36 + 0 = 540940 = 5.4094 10 5 .
Rule 2 When multiplying (dividing) approximate numbers, you must:
a) select the number (numbers) with the least number of significant digits ( SIGNIFICANT - numbers other than zero and zeros between them);
b) round the rest of the numbers so that they have one significant digit more (one spare digit is saved) than the one allocated in paragraph a;
c) multiply (divide) the resulting numbers;
d) as a result, leave as many significant digits as there were in the number (numbers) with the least number of significant digits.
Example: .
Rule 3 When raising to a power, when extracting the root, as a result, as many significant digits are saved as there are in the original number.
Example: 
.
Rule 4 When finding the logarithm of a number, the mantissa of the logarithm must have as many significant digits as there are in the original number:
Example: 
.
In the final entry absolute errors should be left only one significant digit. (If this digit turns out to be 1, then another digit is saved after it).
The mean value is rounded to the same digit as the absolute error.
For example: V\u003d (375.21 0.03) cm 3 \u003d (3.7521 0.0003) cm 3.
I\u003d (5.530 0.013) A, A = 
J.
Work order
Determining the cylinder diameter.
1. Using a caliper, measure the cylinder diameter 7 times (in different places and directions). Record the results in a table.
| No. p / p | d i , mm | d i- | (d i- ) 2 | h i , mm And Related information: |
Errors in measured and tabular values cause errors DX avg of an indirectly determined value, and the largest contribution to DX avg is made by the least accurate values with the maximum relative error d. Therefore, to improve the accuracy of indirect measurements, it is necessary to achieve equal accuracy of direct measurements.
(d A, d B, d C, ...).
Rules for finding errors of indirect measurements:
1. Find the natural logarithm of given function
log(X = f(A,B,C,…));
2. Find the total differential (over all variables) from the found natural logarithm given function;
3. Replace the sign of the differential d with the sign absolute error D;
4. Replace all the "minuses" facing absolute errors DA, DB, DC, ... to the "pros".
The result is the formula for the largest relative error d x indirectly measured value X:
d x = = j (A av, B av, C av, …, DA av, DB av, DC av, …).(18)
According to the found relative error d x determine the absolute error of indirect measurement:
DX cf \u003d d x. X cf . (19)
The result of indirect measurements is recorded in standard form and depicted on the numerical axis:
X \u003d (X sr ± DX sr), unit. (twenty)
Example:
Find the values of the relative and average errors of a physical quantity L, determined indirectly by the formula:
, (21)
where π, g, t, k, α, β- quantities whose values are measured or taken from reference tables and entered in a table of measurement results and tabular data (similar to Table 1).
1. Calculate the average value L cf, substituting in (21) the average values from the table - π cf, g cf, t cf, k cf, α cf, β cf.
2. Determine the largest relative error δ L:
a). Formula (21) is logarithmized:
b). The resulting expression (22) is differentiated:
c). Replace the sign of the differential d with Δ, and the "minuses" in front of the absolute errors - with "pluses", and get the expression for the largest relative error δ L:
d). Substituting in the resulting expression the average values of the input quantities and their errors from the table of measurement results, calculate δ L.
3. Then calculate the absolute error ΔLav:
The result is recorded in standard form and is plotted graphically on the axis L:
, units rev.
ELEMENTARY ESTIMATES OF MEASUREMENT ERRORS
Measurement is finding the value of a physical quantity empirically with the help of special technical means - measures, measuring instruments.
A measure is a means of measurement that reproduces a physical quantity of a given size - a unit of measure, its multiple or fractional value. For example, weights 1 kg, 5 kg, 10 kg.
A measuring device is a measuring instrument designed to generate a signal of measuring information in a form accessible to direct perception by an observer. The measuring device allows you to directly or indirectly compare the measured value with the measures. Measurements are also divided into direct and indirect.
With direct measurements, the desired value of the quantity is found directly from the main (experimental) data.
With indirect measurements, the desired value of a quantity is found on the basis of a known relationship between this quantity and the quantities subjected to direct measurements. The measurement principle is the totality of physical phenomena on which measurements are based.
Measurement method - a set of methods for using the principles and means of measurement. Meaning physical quantity, which would ideally reflect in qualitative and quantitative terms the corresponding property of the given object is the true value of the physical quantity. The value of a physical quantity found by measuring it is the result of the measurement.
The deviation of the measurement result from the true value of the measured quantity is the measurement error.
The absolute measurement error is the measurement error, expressed in units of the measured quantity and equal to the difference between the result and the true value of the measured quantity. The ratio of the absolute error to the true value of the measured quantity is the relative measurement error.
The contribution to the measurement error is made by the errors of measuring instruments (instrumental or instrumental error), the imperfection of the measurement method, the error of reading on the scale of the instrument, external influences on the means and objects of measurement, the delay in human response to light and sound signals.
According to the nature of the manifestation of errors, they are divided into systematic and random. A random event is one that, given a set of factors, may or may not occur.
Random error - a component of the measurement error that changes randomly with repeated measurements of the same value. characteristic feature random errors is the change in the magnitude and sign of the error under constant measurement conditions.
Systematic error - a component of the measurement error that remains constant or regularly changes during repeated measurements of the same value. Systematic errors can, in principle, be eliminated by means of corrections, using more accurate instruments and methods (although in practice it is not always easy to detect a systematic error). It is impossible to exclude random errors of individual measurements; the mathematical theory of random phenomena (probability theory) only allows one to establish a reasonable estimate of their magnitude.
Errors of direct measurements
Let us assume that systematic errors are excluded and the errors of measurement results are only random. Let us denote by letters - the results of measurements of a physical quantity, the true value of which is equal to 
. The absolute errors of the results of individual measurements are indicated:
Summing up the obtained left and right sides of equality (1), we obtain:
(2)
The theory of random errors is based on experimentally confirmed assumptions:
errors can take a continuous series of values;
at large numbers measurements random errors of the same magnitude, but different sign meet equally often;
the probability of an error occurring decreases as its magnitude increases. It is also necessary that the errors be small compared to the measured value and independent.
According to assumption (1), with the number of measurements n we get
,
However, the number of dimensions is always finite and remains unknown. But for practical purposes, it is enough to find experimentally the value of a physical quantity so close to the true value that 
can be used instead of the true one. The question is how to estimate the degree of this approximation?
According to probability theory, the arithmetic mean of a series of measurements more reliable than the results of individual measurements, because random deviations from the true value in different directions are equally probable. For the probability of the appearance of the quantity a i in the interval of width 2a i understand the relative frequency of occurrence of values a i falling into the interval 2a i to the number of all appearing values of a i with the number of experiments (measurements) tending to infinity. Obviously, the probability of a certain event is equal to one, the probability of an impossible event is zero, i.e. 0 100%.
The probability that the desired value ( true value its) is contained in the interval (a - a, a + a) we will call the confidence probability (reliability) , and the corresponding interval (a - a, a + a) - the confidence interval; the smaller the error value a, the smaller the probability that the measured value is contained in the interval defined by this error. The converse statement is also true: the lower the reliability of the result, the narrower the confidence interval of the desired value.
For large n (practically for n 100), the half-width of the confidence interval for a given reliability is equal to
,
(3)
where K() = 1 at = 0.68; K() = 2 at = 0.95; K() = 3 with = 0.997.
With a small number of measurements, which is most often found in student laboratory practice, the coefficient K() in (3) depends not only on , but also on the number of measurements n. Therefore, in the presence of only a random error, we will always find the half-width of the confidence interval by the formula
(4)
In (4) coefficient t n is called Student's coefficient. For = 0.95 adopted in student practice, the values of t n are as follows:
The value is called the root-mean-square error of the arithmetic mean from a series of measurements.
The error of the device or measure is usually indicated in his (her) passport or by a symbol on the scale of the device. Usually, the instrument error is understood as the half-width of the interval within which the measured value can be contained with a measurement probability of 0.997, if the measurement error is due only to the instrument error. As a total (total) error of the measurement result, we accept with a probability = 0.95
The absolute error allows you to determine which sign of the result contains an inaccuracy. Relative error gives information about what proportion (percentage) of the measured value is the error (half-width of the confidence interval).
We write the final result of a series of direct measurements of a 0 in the form
.
For example
(6)
Thus, any physical quantity found empirically must be represented by:
No measurement is free from errors, or, more precisely, the probability of measurement without errors approaches zero. The type and causes of errors are very diverse and are influenced by many factors (Fig. 1.2).
The general characteristics of the influencing factors can be systematized from various points of view, for example, by the influence of the listed factors (Fig. 1.2).
According to the measurement results, errors can be divided into three types: systematic, random and misses.
Systematic errors, in turn, they are divided into groups due to their occurrence and the nature of their manifestation. They can be eliminated different ways such as amendments.
rice. 1.2
Random errors caused by a complex set of changing factors, usually unknown and difficult to analyze. Their influence on the measurement result can be reduced, for example, by repeated measurements with further statistical processing obtained results by the method of probability theory.
TO misses include gross errors that occur with sudden changes in the experimental conditions. These errors are also random in nature and should be eliminated once identified.
The accuracy of measurements is estimated by measurement errors, which are divided according to the nature of their occurrence into instrumental and methodical and according to the calculation method into absolute, relative and reduced.
instrumental the error is characterized by the accuracy class measuring instrument, which is given in his passport in the form of standardized basic and additional errors.
methodical the error is due to the imperfection of methods and measuring instruments.
Absolute the error is the difference between the measured G u and the true G values of the quantity, determined by the formula:
Δ=ΔG=G u -G
Note that the quantity has the dimension of the measured quantity.
Relative the error is found from the equality
δ=±ΔG/G u 100%
Given the error is calculated by the formula (accuracy class of the measuring instrument)
δ=±ΔG/G normal 100%
where G norms is the normalizing value of the measured quantity. It is taken equal to:
a) the final value of the scale of the device, if the zero mark is on the edge or outside the scale;
b) the sum of the final values of the scale, excluding signs, if the zero mark is located inside the scale;
c) the length of the scale, if the scale is uneven.
The accuracy class of the device is established during its verification and is a normalized error calculated by the formulas
γ=±ΔG/G normal 100% if∆Gm=const
where ΔG m is the largest possible absolute error of the device;
G k is the final value of the measurement limit of the device; c and d are coefficients that take into account the design parameters and properties of the instrument's measuring mechanism.
For example, for a voltmeter with a constant relative error, the equality takes place
δm =±c
The relative and reduced errors are related by the following dependencies:
a) for any value of the reduced error
δ=±γ G norms /G u
b) for the largest reduced error
δ=±γ m G norms /G u
From these relationships it follows that when measuring, for example, with a voltmeter, in a circuit at the same voltage value, the relative error is the greater, the lower the measured voltage. And if this voltmeter is chosen incorrectly, then the relative error can be commensurate with the value G n , which is invalid. Note that in accordance with the terminology of the tasks being solved, for example, when measuring voltage G \u003d U, when measuring current C \u003d I, the letter designations in the formulas for calculating errors must be replaced with the corresponding symbols.
Example 1.1. Voltmeter with values γ m = 1.0%, U n \u003d G norms, G k \u003d 450 V, measure the voltage U u equal to 10 V. Let us estimate the measurement errors.
Solution.
Answer. The measurement error is 45%. With such an error, the measured voltage cannot be considered reliable.
At limited opportunities choice of instrument (voltmeter), the methodological error can be taken into account by the correction calculated by the formula
Example 1.2. Calculate the absolute error of the V7-26 voltmeter when measuring voltage in a DC circuit. The accuracy class of the voltmeter is given by the maximum reduced error γ m =±2.5%. The limit of the voltmeter scale used in the work is U norms \u003d 30 V.
Solution. The absolute error is calculated according to the known formulas:
(since the reduced error, by definition, is expressed by the formula 
, then from here you can find the absolute error: 
Answer.ΔU = ±0.75 V .
Important steps in the measurement process are the processing of results and rounding rules. The theory of approximate calculations allows, knowing the degree of accuracy of the data, to assess the degree of accuracy of the results even before performing actions: to select data with the appropriate degree of accuracy, sufficient to ensure the required accuracy of the result, but not too high to save the calculator from useless calculations; rationalize the calculation process itself, freeing it from those calculations that will not affect the exact numbers of the results.
When processing the results, rounding rules are applied.
- Rule 1 If the first of the discarded digits is greater than five, then the last of the retained digits is increased by one.
- Rule 2 If the first of the discarded digits is less than five, then no increase is made.
- Rule 3 If the discarded digit is five, and there are no significant figures behind it, then rounding is performed to the nearest even number, i.e. the last digit stored is left unchanged if it is even, and incremented if it is not even.
If there are significant figures after the number five, then rounding is performed according to rule 2.
By applying rule 3 to rounding a single number, we do not increase the rounding accuracy. But with multiple roundings, overnumbers will be about as common as undernumbers. Mutual error compensation will provide the greatest accuracy of the result.
A number that is known to be greater than the absolute error (or equal to it in the worst case) is called limiting absolute error.
The value of the marginal error is not quite certain. For each approximate number, its marginal error (absolute or relative) must be known.
When it is not directly indicated, it is understood that the limiting absolute error is half the unit of the last discharged discharge. So, if an approximate number of 4.78 is given without specifying the marginal error, then it is understood that the marginal absolute error is 0.005. As a result of this agreement, you can always do without indicating the marginal error of a number rounded according to the rules 1-3, i.e., if the approximate number is denoted by the letter α, then
Where Δn is the ultimate absolute error; and δ n is the limiting relative error.
In addition, when processing the results, error rules sum, difference, product and quotient.
- Rule 1 The limiting absolute error of the sum is equal to the sum of the limiting absolute errors of the individual terms, but with a significant number of errors in the terms, mutual compensation of errors usually occurs, therefore the true error of the sum only in exceptional cases coincides with the limiting error or is close to it.
- Rule 2 The limiting absolute error of the difference is equal to the sum of the limiting absolute errors of the minuend or subtrahend.
The limiting relative error is easy to find by calculating the limiting absolute error.
- Rule 3 The limiting relative error of the sum (but not the difference) lies between the smallest and the largest of the relative errors of the terms.
If all terms have the same marginal relative error, then the sum has the same marginal relative error. In other words, in this case, the accuracy of the sum (in percentage terms) is not inferior to the accuracy of the terms.
In contrast to the sum, the difference between approximate numbers may be less accurate than the minuend and the subtracted. The loss of precision is especially great when the minuend and the subtrahend differ little from each other.
- Rule 4 The limiting relative error of the product is approximately equal to the sum of the limiting relative errors of the factors: δ \u003d δ 1 + δ 2, or, more precisely, δ \u003d δ 1 + δ 2 + δ 1 δ 2 where δ is the relative error of the product, δ 1 δ 2 - relative errors factors.
Notes:
1. If approximate numbers with the same number of significant digits are multiplied, then the same number of significant digits should be stored in the product. The last digit stored will not be entirely reliable.
2. If some factors have more significant digits than others, then before multiplication, the first ones should be rounded off, keeping as many digits in them as the least accurate factor has or one more (as a spare), it is useless to save further digits.
3. If it is required that the product of two numbers has in advance given number quite reliable, then in each of the factors the number of exact digits (obtained by measurement or calculation) must be one more. If the number of factors is more than two and less than ten, then in each of the factors the number of exact digits for a full guarantee must be two units more than the required number of exact digits. In practice, it is quite enough to take only one extra digit.
- Rule 5 The limiting relative error of the quotient is approximately equal to the sum of the limiting relative errors of the dividend and divisor. The exact value of the limiting relative error always exceeds the approximate one. The excess percentage is approximately equal to the limiting relative error of the divider.
Example 1.3. Find the limiting absolute error of the quotient 2.81: 0.571.
Solution. The marginal relative error of the dividend is 0.005:2.81=0.2%; divider - 0.005: 0.571 = 0.1%; private - 0.2% + 0.1% = 0.3%. The limiting absolute error of the quotient will approximately be 2.81: 0.571 0.0030=0.015
So, in the quotient 2.81:0.571=4.92 is already the third significant figure not reliable.
Answer. 0,015.
Example 1.4. Calculate the relative error of the readings of the voltmeter connected according to the circuit (Fig. 1.3), which is obtained if we assume that the voltmeter has an infinitely large resistance and does not introduce distortions into the measured circuit. Classify the measurement error for this task.
rice. 1.3
Solution. Let's denote the readings of a real voltmeter as I, and a voltmeter with an infinitely large resistance through I ∞. Required relative error 
notice, that
then we get
Since R AND >>R and R>r, the fraction in the denominator of the last equality is much less than one. Therefore, we can use the approximate formula 
, valid for λ≤1 for any α . Assuming that in this formula α = -1 and λ= rR (r+R) -1 R AND -1 , we get δ ≈ rR/(r+R) R AND .
The greater the resistance of the voltmeter compared to the external resistance of the circuit, the smaller the error. But the condition R< Answer. The error is systematic and methodical. Example 1.5.
The following devices are included in the DC circuit (Fig. 1.4): A - ammeter type M 330 accuracy class K A \u003d 1.5 with a measurement limit of I k \u003d 20 A; A 1 - ammeter type M 366 accuracy class K A1 \u003d 1.0 with a measurement limit I k1 \u003d 7.5 A. Find the largest possible relative error in measuring the current I 2 and possible limits of its actual value if the instruments showed that I \u003d 8 ,0A. and I 1 \u003d 6.0A. Classify the measurement. rice. 1.4 Solution. We determine the current I 2 according to the readings of the device (excluding their errors): I 2 \u003d I-I 1 \u003d 8.0-6.0 \u003d 2.0 A. Find the modules of absolute errors of ammeters A and A 1 For A we have the equality Let's find the sum of modules of absolute errors: Therefore, the largest possible and the same value, expressed in fractions of this value, is equal to 1. 10 3 - for one device; 2 10 3 - for another device. Which of these instruments will be the most accurate? Solution. The accuracy of the device is characterized by a value that is the reciprocal of the error (the more accurate the device, the smaller the error), i.e. for the first device, this will be 1 / (1. 10 3) = 1000, for the second - 1 / (2. 10 3) = 500. Note that 1000 > 500. Therefore, the first device is two times more accurate than the second. A similar conclusion can be reached by checking the correspondence of the errors: 2 . 10 3 / 1 . 10 3 = 2. Answer. The first device is twice as accurate as the second. Example 1.6.
Find the sum of approximate measurements of the device. Find the number of valid characters: 0.0909 + 0.0833 + 0.0769 + 0.0714 + 0.0667 + 0.0625 + 0.0588+ 0.0556 + 0.0526. Solution. Adding all the results of the measurements, we get 0.6187. The maximum maximum error of the sum is 0.00005 9=0.00045. This means that in the last fourth digit of the sum, an error of up to 5 units is possible. Therefore, we round the amount to the third decimal place, i.e. thousandths, we get 0.619 - a result in which all signs are correct. Answer. 0.619. The number of valid characters is three decimal places. MEASUREMENT OF PHYSICAL QUANTITIES. INTRODUCTION The K-402.1 complex is a necessary list of laboratory work provided for by the educational standard and the work program for the section "Dynamics of a rigid body" of the discipline "Physics". It includes a description of laboratory facilities, the order of measurements and an algorithm for calculating certain physical quantities. If a student begins to get acquainted with a particular work in the classroom during the lesson, then two hours allocated for the performance of one laboratory work will not be enough for him and the lag behind the semester work schedule will begin. To exclude this by the educational standard of the second generation, 50% of the hours allocated for the study of the discipline falls on independent work, which is a necessary component of the learning process. The purpose of independent work is to consolidate and deepen knowledge and skills, prepare for lectures, practical and laboratory classes, as well as to form students' independence in acquiring new knowledge and skills. Curricula for various specialties provide for independent study of the discipline "Physics" during the semester from 60 to 120 hours. Of these, laboratory classes account for 20-40 hours, or 2-4 hours for one job. During this time, the student must: read the relevant paragraphs in the textbooks; learn the basic formulas and laws; familiarize yourself with the installation and measurement procedure. To be allowed to perform work on the installation, the student must know the installation device, be able to determine the division value of the measuring device, know the sequence of measurements, be able to process the measurement results, and evaluate the error. After all the calculations and the preparation of the report, the student must draw a conclusion in which to specifically indicate those physical patterns that were verified in the course of the work. There are two types of measurements: direct and indirect. Direct measurements are those measurements in which a comparison of the measure and the object is made. For example, measure the height and diameter of a cylinder using a caliper. With indirect measurements, a physical quantity is determined on the basis of a formula that establishes its connection with the quantities found by direct measurements. The measurement cannot be absolutely accurate. Its result always contains some error. Measurement errors are usually divided into systematic and random. Systematic errors are due to factors that act in the same way when the same measurements are repeated many times. The contribution to systematic errors is made by instrumental or instrument error, which is determined by the sensitivity of the device. In the absence of such data on the instrument, the instrumental error is taken as the price or half the price of the smallest scale division of the instrument. Random errors caused by the simultaneous action of many factors that cannot be taken into account. Most measurements are accompanied by random errors, which differ in that with each repeated measurement they take on a different, unpredictable value. Absolute error will include systematic and random errors: The true value of the measured value will be in the interval: which is called the confidence interval. To determine the random error, first calculate the average of all values obtained during the measurement: where is the result i th dimension, is the number of dimensions. Then, find the errors of individual measurements When processing the measurement results, the Student's distribution is used. Taking into account the Student's coefficient, the random error Table 1.1 Student's coefficient table Student's coefficient shows the deviation of the arithmetic mean from the true value, expressed as a fraction of the root mean square error. Student's coefficient depends on the number of measurements n and on reliability and is indicated in Table. 1.1. The absolute error is calculated by the formula In most cases, not the absolute, but the relative error plays a more significant role. Or All calculation results are entered in table. 1.2. Table 1.2 The result of calculating the measurement error Calculation of errors of indirect measurements Instruction First of all, take several measurements with the instrument of the same value in order to be able to get the actual value. The more measurements you take, the more accurate the result will be. For example, weigh on an electronic scale. Let's say you got results of 0.106, 0.111, 0.098 kg. Now calculate the actual value of the quantity (valid, since the true value cannot be found). To do this, add the results and divide them by the number of measurements, that is, find the arithmetic mean. In the example, the actual value would be (0.106+0.111+0.098)/3=0.105. The second arise from the influence of causes, and random nature. These include incorrect rounding when counting readings and influence. If such errors are much smaller than the divisions of the scale of this measuring instrument, then it is advisable to take half a division as an absolute error. Slip or rough error is the result of observation, which differs sharply from all the others. Absolute error approximate numerical value is the difference between the result, during the measurement, and the true value of the measured quantity. The true or actual value reflects the investigated physical quantity. This error is the simplest quantitative measure of error. It can be calculated using the following formula: ∆X = Hisl - Hist. It can take positive and negative values. For a better understanding, consider. The school has 1205 students, when rounded to 1200 absolute error equals: ∆ = 1200 - 1205 = 5. There are certain calculation of error values. First, absolute error the sum of two independent quantities is equal to the sum of their absolute errors: ∆(Х+Y) = ∆Х+∆Y. A similar approach is applicable for the difference of two errors. You can use the formula: ∆(X-Y) = ∆X+∆Y. Sources: Measurements can be made with varying degrees of accuracy. At the same time, even precision instruments are not absolutely accurate. Absolute and relative errors may be small, but in reality they are almost always present. The difference between the approximate and exact values of a certain quantity is called absolute. error. In this case, the deviation can be both up and down. You will need Instruction Before calculating the absolute error, take several postulates as initial data. Eliminate gross errors. Assume that the necessary corrections have already been calculated and applied to the result. Such an amendment can be a transfer of the initial measurement point. Take as a starting point the fact that random errors are taken into account. This implies that they are less systematic, that is, absolute and relative, characteristic of this particular device. Random errors affect the result of even high-precision measurements. Therefore, any result will be more or less close to the absolute, but there will always be discrepancies. Define this interval. It can be expressed by the formula (Xmeas- ΔX) ≤ Xism ≤ (Xism + ΔX).
Determine the value closest to the value. In measurements, arithmetic is taken, which can be obtained from the formula in the figure. Accept the result as the true value. In many cases, the reading of a reference instrument is taken as accurate. Knowing the true value, you can find the absolute error, which must be taken into account in all subsequent measurements. Find the value of X1 - the data of a particular measurement. Determine the difference ΔX by subtracting the smaller from the larger. When determining the error, only the modulus of this difference is taken into account. note As a rule, it is not possible to carry out an absolutely accurate measurement in practice. Therefore, the marginal error is taken as the reference value. It represents the maximum value of the modulus of absolute error. Useful advice In practical measurements, the value of the absolute error is usually taken as half of the smallest division value. When operating with numbers, the absolute error is taken as half of the value of the digit, which is in the next digit after the exact digits. To determine the accuracy class of the device, the ratio of the absolute error to the measurement result or to the length of the scale is more important. Measurement errors are associated with the imperfection of devices, tools, methods. Accuracy also depends on the attentiveness and condition of the experimenter. Errors are divided into absolute, relative and reduced. Instruction Let a single measurement of the value give the result x. The true value is indicated by x0. Then the absolute errorΔx=|x-x0|. She evaluates the absolute . Absolute error consists of three components: random errors, systematic errors and misses. Usually, when measuring with an instrument, half the division value is taken as an error. For a millimeter ruler, this would be 0.5 mm. The true value of the measured value in the interval (x-Δx; x+Δx). In short, this is written as x0=x±Δx. It is important to measure x and Δx in the same units and write in the same format, such as an integer part and three decimal points. So the absolute error gives the boundaries of the interval in which the true value lies with some probability. Measurements are direct and indirect. In direct measurements, the desired value is immediately measured with the appropriate instrument. For example, bodies with a ruler, voltage with a voltmeter. With indirect measurements, the value is found according to the formula of the relationship between it and the measured values. If the result is a dependence on three directly measured quantities with errors Δx1, Δx2, Δx3, then error indirect measurement ΔF=√[(Δx1 ∂F/∂x1)²+(Δx2 ∂F/∂x2)²+(Δx3 ∂F/∂x3)²]. Here ∂F/∂x(i) are the partial derivatives of the function with respect to each of the directly measured quantities. Useful advice Misses are gross inaccuracies in measurements that occur when the instruments malfunction, the experimenter's inattention, and the experimental methodology is violated. To reduce the likelihood of such misses, be careful when taking measurements and describe the result in detail. Sources: quantitative concept " accuracy does not exist in science. This is a qualitative concept. When defending dissertations, they only talk about errors (for example, measurements). And even if the word " accuracy”, then one should keep in mind a very vague measure of the magnitude, the reciprocal of the error. Instruction A small analysis of the concept of "approximate value". It is possible that the approximate result of the calculation is meant. error ( accuracy) is set here by the performer of the work. This error is indicated, for example, "up to 10 to the minus fourth power." If the error is relative, then in percent or fractions. If the calculations were carried out on the basis of a numerical series (most often Taylor) - on the basis of the modulus of the residual term of the series. About approximate values values are often spoken of as estimated values. Measurement results are random. Therefore, these are the same random variables that have the characteristics of the spread of values, like the same variance or r.s.d. (the average In our age, man has invented and uses a huge variety of various measuring instruments. But no matter how perfect the technology of their manufacture, they all have a greater or lesser error. This parameter, as a rule, is indicated on the instrument itself, and in order to assess the accuracy of the value being determined, one must be able to understand what the numbers indicated on the marking mean. In addition, relative and absolute errors inevitably arise in complex mathematical calculations. It is widely used in statistics, industry (quality control) and in a number of other areas. How this value is calculated and how to interpret its value - this is exactly what will be discussed in this article. Absolute error Let us denote by x the approximate value of a quantity, obtained, for example, by means of a single measurement, and by x 0 its exact value. Now let's calculate the modulus of the difference between these two numbers. The absolute error is exactly the value that we got as a result of this simple operation. Expressed in the language of formulas, this definition can be written as follows: Δ x = | x - x0 |. Relative error The absolute deviation has one important drawback - it does not allow us to assess the degree of importance of the error. For example, we buy 5 kg of potatoes in the market, and an unscrupulous seller, when measuring weight, made a mistake by 50 grams in his favor. That is, the absolute error was 50 grams. For us, such an oversight will be a mere trifle and we will not even pay attention to it. Imagine what would happen if a similar error occurs in the preparation of a medicine? Here everything will be much more serious. And when loading a freight car, deviations are likely to occur much larger than this value. Therefore, the absolute error itself is not very informative. In addition to it, very often, a relative deviation is additionally calculated, equal to the ratio of the absolute error to the exact value of the number. This is written in the following formula: δ = Δ x / x 0 . Error Properties Suppose we have two independent quantities: x and y. We need to calculate the deviation of the approximate value of their sum. In this case, we can calculate the absolute error as the sum of the pre-calculated absolute deviations of each of them. In some measurements, it may happen that errors in determining x and y values cancel each other out. And it may also happen that as a result of addition, the deviations will increase as much as possible. Therefore, when calculating the total absolute error, the worst case should be taken into account. The same is true for the error difference of several values. This property is characteristic only for absolute error, and it cannot be applied to relative deviation, since this will inevitably lead to an incorrect result. Let's consider this situation in the following example. Suppose measurements inside the cylinder showed that the inner radius (R 1) is 97 mm, and the outer one (R 2) is 100 mm. It is required to determine the thickness of its wall. First, find the difference: h \u003d R 2 - R 1 \u003d 3 mm. If the task does not indicate what the absolute error is equal to, then it is taken as half the scale division of the measuring instrument. Thus, Δ (R 2) \u003d Δ (R 1) \u003d 0.5 mm. The total absolute error is: Δ(h) = Δ(R 2) + Δ(R 1) = 1 mm. Now we calculate the relative deviation of all quantities: δ(R 1) \u003d 0.5 / 100 \u003d 0.005, δ(R 1) \u003d 0.5 / 97 ≈ 0.0052, δ(h) = Δ(h)/h = 1/3 ≈ 0.3333>> δ(R 1). As you can see, the error in measuring both radii does not exceed 5.2%, and the error in calculating their difference - the thickness of the cylinder wall - was as much as 33.(3)%! The following property says: the relative deviation of the product of several numbers is approximately equal to the sum of the relative deviations of the individual factors: δ(xy) ≈ δ(x) + δ(y). Moreover, this rule is true regardless of the number of estimated values. The third and final property of relative error is that the relative estimate numbers k-th degree approximately in | k | times greater than the relative error of the original number.
for ammeter 
. (1.1)
, (1.2)
, 
, …, 
.
. (1.3)
.
n
0,6
0,7
0,9
0,95
0,99
1,36
2,0
6,3
12,7
636,6
1,06
1,3
2,9
4,3
31,6
0,98
1,3
2,4
3,2
12,9
0,94
1,2
2,1
2,8
8,7
…
…
…
…
…
…
0,85
1,0
1,7
2,0
3,5
0,84
1,0
1,7
2,0
3,4
.
. (1.4)No. p / p
mm mm mm mm 2 mm 2 mm mm mm mm mm %
