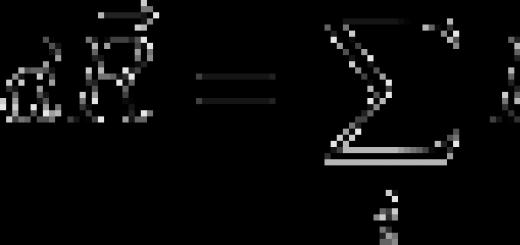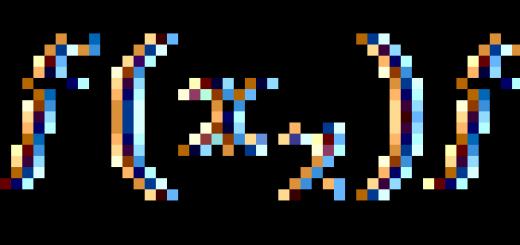Forces act on electric charges in an electrostatic field. Therefore, if the charges move, then these forces do work. Calculate the work of the forces of a homogeneous electrostatic field when moving a positive charge q from a point A exactly B(Fig. 1).
per charge q, placed in a uniform electric field with intensity E, the force \(~\vec F = q \cdot \vec E \) acts. Field work can be calculated by the formula
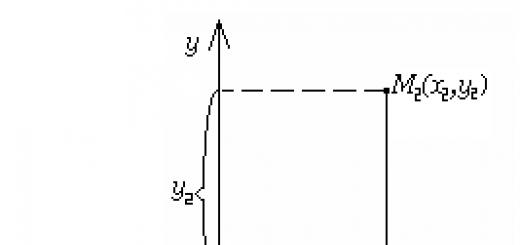
\(~A_(AB) = F \cdot \Delta r \cdot \cos \alpha,\)
where ∆ r⋅cosα = AC = x 2 – x 1 = Δ x- projection of displacement onto the line of force (Fig. 2).

\(~A_(AB) = q \cdot E \cdot \Delta x. \ \ (1)\)
Consider now the movement of the charge along the trajectory ACB(see fig. 1). In this case, the work of a homogeneous field can be represented as the sum of works in the areas AC and CB:
\(~A_(ACB) = A_(AC) + A_(CB) = q \cdot E \cdot \Delta x + 0 = q \cdot E \cdot \Delta x\)
(Location on CB work is zero, because the displacement is perpendicular to the force \(~\vec F \)). As you can see, the field work is the same as when the charge moves along the segment AB.
It is not difficult to prove that the work of the field when moving a charge between points AB along any trajectory everything will be according to the same formula 1.
Thus,
- the work of moving a charge in an electrostatic field does not depend on the shape of the trajectory along which the charge moved q , but depends only on the initial and final positions of the charge.
- This statement is also true for an inhomogeneous electrostatic field.
Let's find work on a closed trajectory ABCA:
\(~A_(ABCA) = A_(AB) + A_(BC) + A_(CA) = q \cdot E \cdot \Delta x + 0 - q \cdot E \cdot \Delta x = 0.\)
The field, the work of forces of which does not depend on the shape of the trajectory and is equal to zero on a closed trajectory, is called potential or conservative.
Potential
It is known from mechanics that the work of conservative forces is associated with a change in potential energy. The system "charge - electrostatic field" has potential energy (energy of electrostatic interaction). Therefore, if we do not take into account the interaction of the charge with gravitational field and environment, then the work done when moving a charge in an electrostatic field is equal to the change in the potential energy of the charge, taken with the opposite sign:
\(~A_(12) = -(W_(2) - W_(1)) = W_(1) - W_(2) . \)
Comparing the resulting expression with Equation 1, we can conclude that
\(~W = -q \cdot E \cdot x, \)
where x is the charge coordinate on the 0X axis directed along the field line (see Fig. 1). Since the charge coordinate depends on the choice of the reference frame, the potential energy of the charge also depends on the choice of the reference frame.
If a W 2 = 0, then at each point of the electrostatic field the potential energy of the charge q 0 is equal to the work that would be done by moving the charge q 0 from a given point to a point with zero energy.
Let an electrostatic field be created in some region of space by a positive charge q. We will place various test charges at some point of this field q 0 . Their potential energy is different, but the ratio \(~\dfrac(W)(q_0) = \operatorname(const)\) for a given point of the field serves as a characteristic of the field, called potential field φ at a given point.
- The potential of the electrostatic field φ at a given point in space is a scalar physical quantity equal to the ratio of potential energy W, which has a point charge q at a given point in space, to the value of this charge:
The SI unit of potential is volt(V): 1 V = 1 J/C.
- Potential is the energy characteristic of the field.
Potential properties.
- The potential, like the potential energy of the charge, depends on the choice of reference system (zero level). AT technique for the zero potential choose the potential of the surface of the Earth or a conductor connected to the ground. Such a conductor is called grounded. AT physics for the reference point (zero level) of the potential (and potential energy) is taken any point infinitely distant from the charges that create the field.
- On distance r from a point charge q, which creates a field, the potential is determined by the formula
- Potential at any point of the field created positive charge q, positive, and the field created by the negative charge is negative: if q> 0, then φ > 0; if q < 0, то φ < 0.
- The potential of the field formed by a uniformly charged conducting sphere with a radius R, at a point at a distance r from the center of the sphere \(~\varphi = k \cdot \dfrac(q)(R)\) for r ≤ R and \(~\varphi = k \cdot \dfrac(q)(r)\) with r > R .
- Superposition principle: the potential φ of the field created by the system of charges at some point in space is equal to algebraic sum potentials created at this point by each charge separately:
Knowing the potential φ of the field at a given point, it is possible to calculate the potential energy of the charge q 0 placed at this point: W 1 = q 0 ⋅φ. If we assume that the second point is at infinity, i.e. W 2 = 0, then
\(~A_(1\infty) = W_(1) = q_0 \cdot \varphi_1 .\)
Potential charge energy q 0 at a given point of the field will be equal to the work of the forces of the electrostatic field to move the charge q 0 from a given point to infinity. From the last formula we have
\(~\varphi_1 = \dfrac(A_(1\infty))(q_0).\)
- The physical meaning of the potential: field potential at a given point numerically equals work by moving a unit positive charge from a given point to infinity.
Potential charge energy q 0 placed in an electrostatic field of a point charge q on distance r From him,
\(~W = k \cdot \dfrac(q \cdot q_0)(r).\)
- If a q and q 0 - like charges, then W> 0 if q and q 0 - charges of different sign, then W < 0.
- Note that this formula can be used to calculate the potential energy of interaction of two point charges, if for zero value W its value is chosen at r = ∞.
Potential difference. Voltage
The work of the forces of the electrostatic field on the movement of the charge q 0 from point 1 exactly 2 fields
\(~A_(12) = W_(1) - W_(2) .\)
We express the potential energy in terms of the field potentials at the corresponding points:
\(~W_(1) = q_0 \cdot \varphi_1 , W_(2) = q_0 \cdot \varphi_2 .\)
\(~A_(12) = q_0 \cdot (\varphi_1 - \varphi_2) .\)
Thus, the work is determined by the product of the charge and the potential difference of the initial and final points.
From this formula, the potential difference
\(~\varphi_1 - \varphi_2 = \dfrac(A_(12))(q_0) .\)
- Potential difference- this is a scalar physical quantity, numerically equal to the ratio of the work of the field forces to move the charge between the given points of the field to this charge.
The SI unit for potential difference is the volt (V).
- 1 V is the potential difference between two such points of the electrostatic field, when moving between which a charge of 1 C is performed by the field forces, work of 1 J is performed.
The potential difference, unlike the potential, does not depend on the choice of the zero point. The potential difference φ 1 - φ 2 is often called electric voltage between given points of the field and denote U:
\(~U = \varphi_1 - \varphi_2 .\)
- Voltage between two points of the field is determined by the work of the forces of this field to move a charge of 1 C from one point to another.
The work of forces electric field sometimes expressed not in joules, but in electronvolts.
- 1 eV is equal to the work done by the field forces when moving an electron ( e\u003d 1.6 10 -19 C) between two points, the voltage between which is 1 V.
Potential difference and tension
Calculate the work done by the forces of the electrostatic field when moving electric charge q 0 from a point with potential φ 1 to a point with potential φ 2 of a uniform electric field.
On the one hand, the work of the field forces \(~A = q_0 \cdot (\varphi_1 - \varphi_2)\).
On the other hand, the work of moving the charge q 0 in a uniform electrostatic field \(~A = q_0 \cdot E \cdot \Delta x\).
Equating the two expressions to work, we get:
\(~q_0 \cdot (\varphi_1 - \varphi_2) = q_0 \cdot E \cdot \Delta x, \;\; E = \dfrac(\varphi_1 - \varphi_2)(\Delta x),\)
where ∆ x- projection of displacement onto the line of force.
This formula expresses the relationship between the intensity and the potential difference of a uniform electrostatic field. Based on this formula, you can set the unit of tension in SI: volt per meter (V/m).
Literature
- Aksenovich L. A. Physics in high school: Theory. Tasks. Tests: Proc. allowance for institutions providing general. environments, education / L. A. Aksenovich, N. N. Rakina, K. S. Farino; Ed. K. S. Farino. - Mn.: Adukatsia i vykhavanne, 2004. - C. 228-233.
- Zhilko, V. V. Physics: textbook. allowance for the 11th grade. general education institutions with Russian. lang. training with a 12-year term of study (basic and advanced levels) /V. V. Zhilko, L. G. Markovich. - 2nd ed., corrected. - Minsk: Nar. asveta, 2008. - S. 86-95.
F - force of interaction of two point charges
q 1 , q 2- the magnitude of the charges
ε α - absolute permittivity of the medium
r - distance between point charges
Conservative electrostatic interaction.
Calculate the work done by the electrostatic field created by the charge q´ by charge movement q from point 1 to point 2.
Work on the way d l is equal to:
![]()
where d r- increment of the radius vector when moving by d l; i.e.
![]()
Then the total work while moving q´ from point 1 to point 2 is equal to the integral:
 |
The work of electrostatic forces does not depend on the shape of the path, but only on the coordinates of the initial and final points of movement . Hence, field strengths are conservative, and the field itself potentially.
The potential of the electrostatic field.
Electrostatic field potential - scalar value equal to the ratio of the potential energy of the charge in the field to this charge:
Energy characteristic of the field at a given point. The potential does not depend on the magnitude of the charge placed in this field.
The potential of the electrostatic field of a point charge.
Consider special case, when the electrostatic field is created by an electric charge Q. To study the potential of such a field, there is no need to introduce a charge q into it. You can calculate the potential of any point of such a field, located at a distance r from the charge Q.


The dielectric constant of the medium has a known value (table), it characterizes the medium in which the field exists. For air, it is equal to one.
The formula for the work of an electrostatic field.
A force acts on the charge q₀ from the side of the field, which can do work and move this charge in the field.


The work of the electrostatic field does not depend on the trajectory. The work of the field when moving the charge along a closed trajectory is equal to zero. For this reason, the forces of the electrostatic field are called conservative, and the field itself is called potential.
Connection of electrostatic field strength with potential.

![]()
The strength at any point of the electric field is equal to the potential gradient at this point, taken with the opposite sign. The minus sign indicates that the intensity E is directed in the direction of decreasing potential.
Capacitance of a conductor and a capacitor.
Electric capacity - a characteristic of a conductor, a measure of its ability to accumulate an electric charge
The formula for the capacitance of a flat capacitor.
Electric field energy.
Energy of a charged capacitor is equal to the work of external forces that must be expended to charge the capacitor. ![]()
Electricity.
Electricity - directed (ordered) motion of charged particles
Conditions for the emergence and existence of electric current.
1. the presence of free charge carriers,
2. the presence of a potential difference. these are the conditions current occurrence,
3. closed circuit,
4. a source of third-party forces that maintains a potential difference.
Third party forces.
Third party forces- forces of a non-electric nature, causing the movement of electric charges inside a direct current source. All forces other than the Coulomb forces are considered external.
emf Voltage.
Electromotive Force (EMF) - a physical quantity that characterizes the work of external (non-potential) forces in sources of direct or alternating current. In a closed conducting circuit, the EMF is equal to the work of these forces in moving a single positive charge along the circuit.
EMF can be expressed in terms of the electric field strength of external forces
Voltage (U)
is equal to the ratio of the work of the electric field on the movement of the charge
to the value of the transferred charge in the circuit section.
Unit of measure for voltage in the SI system:
Current strength.
Current (I)- a scalar value equal to the ratio of the charge q passed through the cross section of the conductor to the time interval t during which the current flowed. The current strength shows how much charge passes through the cross section of the conductor per unit of time.
current density.
Current density j - vector whose modulus is equal to the ratio the strength of the current flowing through a certain area, perpendicular to the direction of the current, to the value of this area.
![]()
The SI unit for current density is the ampere per square meter (A/m2).
When moving a charge in an electrostatic field, acting on
The charge is the Coulomb force, doing work. Let the charge q 0 >0 move in the charge field q>0 from point C to point B along an arbitrary trajectory (Fig. 2.1). Coulomb force acts on q 0With an elementary charge displacement d l, this force does work , where a is the angle between the vectors and . d value l cosa=dr is the projection of the vector onto the direction of the force . Thus, dA=Fdr, . The total work on moving a charge from point C to B is determined by the integral, where r 1 and r 2 are the distances of the charge q to points C and B. From the resulting formula it follows that the work done when moving an electric charge q 0 in the field of a point charge q, does not depend on the shape of the movement path, but only on the start and end points of movement.
A field that satisfies this condition is potential. Therefore, the electrostatic field of a point charge is potential, and the forces acting in it - conservative.
If the charges q and q 0 are of the same sign, then the work of the repulsive forces will be positive when they move away and negative when they approach each other. If the charges q and q 0 are opposite, then the work of the attractive forces will be positive when they approach and negative when they move away from each other.
Let the electrostatic field, in which the charge q 0 moves, be created by a system of charges q 1 , q 2 ,...,q n . Therefore, independent forces act on q 0 , the resultant of which is equal to their vector sum. The work A of the resultant force is equal to the algebraic sum of the work of the component forces, where r i 1 and r i 2 are the initial and final distances between the charges q i and q 0 .
Any charge that is in an electric field is affected by a force. In this regard, when the charge moves in the field, a certain work of the electric field occurs. How to calculate this work?
The work of an electric field is to transfer electric charges along a conductor. It will be equal to the product of the voltage and the time spent on work.
By applying the formula of Ohm's law, we can get several various options formulas for calculating the current work:
A = U˖I˖t = I²R˖t = (U²/R)˖t.
In accordance with the law of conservation of energy, the work of the electric field is equal to the change in the energy of a single section of the circuit, and therefore the energy released by the conductor will be equal to the work of the current.
We express in the SI system:
[A] = V˖A˖s = W˖s = J
1 kWh = 3600000 J.
Let's do an experiment. Consider the movement of a charge in the same field, which is formed by two parallel plates A and B and charged opposite charges. In such a field lines of force are perpendicular to these plates throughout their length, and when plate A is positively charged, then E will be directed from A to B.
Suppose that a positive charge q has moved from point a to point b along an arbitrary path ab = s.
Since the force that acts on the charge that is in the field will be equal to F \u003d qE, the work done when the charge moves in the field according to a given path will be determined by the equality:
A = Fs cos α, or A = qFs cos α.
But s cos α = d, where d is the distance between the plates.
It follows from here: A = qEd.
Let's say now the charge q will move from a and b to essentially acb. The work of the electric field done on this path is equal to the sum of the work done on its individual sections: ac = s₁, cb = s₂, i.e.
A = qEs₁ cos α₁ + qEs₂ cos α₂,
A = qE(s₁ cos α₁ + s₂ cos α₂,).
But s₁ cos α₁ + s₂ cos α₂ = d, and hence in this case A = qEd.
In addition, suppose that the charge q moves from a to b along an arbitrary curved line. To calculate the work done on a given curvilinear path, it is necessary to stratify the field between plates A and B with a certain number of which will be so close to each other that individual sections of the path s between these planes can be considered straight.
In this case, the work of the electric field produced on each of these segments of the path will be equal to A₁ = qEd₁, where d₁ is the distance between two adjacent planes. And the total work on the whole path d will be equal to the product of qE and the sum of distances d₁ equal to d. Thus, as a result of a curvilinear path, the perfect work will be equal to A = qEd.
The examples we have considered show that the work of an electric field in moving a charge from one point to another does not depend on the shape of the path of movement, but depends solely on the position of these points in the field.
In addition, we know that the work done by gravity when moving a body along an inclined plane of length l will be equal to the work done by the body when falling from a height h, and the height of the inclined plane. This means that the work, or, in particular, the work during the movement of the body in the field of gravity, also does not depend on the shape of the path, but depends only on the difference in heights of the first and last points of the path.
Thus, it can be proved that important property can have not only a homogeneous, but any electric field. Gravity has a similar property.
The work of an electrostatic field in moving a point charge from one point to another is determined by the linear integral:
A₁₂ = ∫ L₁₂q (Edl),
where L₁₂ is the trajectory of the charge, dl is the infinitesimal displacement along the trajectory. If the contour is closed, then the symbol ∫ is used for the integral; in this case, it is assumed that the direction of traversal of the contour is selected.
The work of electrostatic forces does not depend on the shape of the path, but only on the coordinates of the first and last points of movement. Therefore, the field strengths are conservative, while the field itself is potential. It is worth noting that the work of any one along a closed path will be equal to zero.