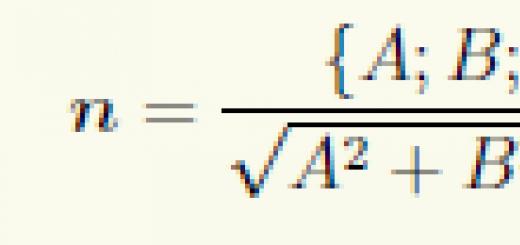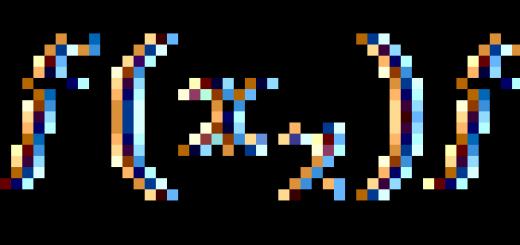Once I witnessed a conversation between two applicants:
– When do you need to add 2πn, and when - πn? I can't remember!
- And I have the same problem.
I wanted to say to them: “It is not necessary to memorize, but to understand!”
This article is addressed primarily to high school students and, I hope, will help them with "understanding" to solve the simplest trigonometric equations:
Number circle
Along with the concept of a number line, there is also the concept number circle. As we know, in a rectangular coordinate system, a circle with a center at the point (0; 0) and a radius of 1 is called a unit circle. Imagine a number line with a thin thread and wind it around this circle: the reference point (point 0), attach it to the “right” point unit circle, we wrap the positive semi-axis counterclockwise, and the negative one - in the direction (Fig. 1). Such a unit circle is called a number circle.
Number circle properties
- Every real number is at one point on the number circle.
- There are infinitely many real numbers on each point of the number circle. Since the length of the unit circle is 2π, the difference between any two numbers at one point on the circle is equal to one of the numbers ±2π; ±4π; ±6π; …
Let's conclude: knowing one of the numbers of point A, we can find all the numbers of point A.

Let's draw the AC diameter (Fig. 2). Since x_0 is one of the numbers of the point A, then the numbers x_0±π ; x_0±3π; x_0±5π; … and only they will be the numbers of the point C. Let's choose one of these numbers, say, x_0+π, and use it to write down all the numbers of the point C: x_C=x_0+π+2πk ,k∈Z. Note that the numbers at points A and C can be combined into one formula: x_(A ; C)=x_0+πk ,k∈Z (for k = 0; ±2; ±4; ... we get the numbers of point A, and for k = ±1, ±3, ±5, … are the numbers of the point C).
Let's conclude: knowing one of the numbers on one of the points A or C of the diameter AC, we can find all the numbers on these points.
- Two opposite numbers are located on points of the circle that are symmetrical about the abscissa axis.
Let's draw a vertical chord AB (Fig. 2). Since points A and B are symmetrical about the Ox axis, the number -x_0 is located at point B and, therefore, all numbers of point B are given by the formula: x_B=-x_0+2πk ,k∈Z. We write the numbers at points A and B with one formula: x_(A ; B)=±x_0+2πk ,k∈Z. Let's conclude: knowing one of the numbers at one of the points A or B of the vertical chord AB, we can find all the numbers at these points. Consider the horizontal chord AD and find the numbers of the point D (Fig. 2). Since BD is the diameter and the number -x_0 belongs to point B, then -x_0 + π is one of the numbers of point D and, therefore, all numbers of this point are given by the formula x_D=-x_0+π+2πk ,k∈Z. Numbers at points A and D can be written using one formula: x_(A ; D)=(-1)^k∙x_0+πk ,k∈Z . (for k= 0; ±2; ±4; ... we get the numbers of point A, and for k = ±1; ±3; ±5; ... - the numbers of point D).
Let's conclude: knowing one of the numbers at one of the points A or D of the horizontal chord AD, we can find all the numbers at these points.
Sixteen main points of the number circle
In practice, the solution of most of the simplest trigonometric equations associated with sixteen points of the circle (Fig. 3). What are these dots? Red, blue and green dots divide the circle into 12 equal parts. Since the length of the semicircle is π, the length of the arc A1A2 is π/2, the length of the arc A1B1 is π/6, and the length of the arc A1C1 is π/3.
Now we can specify one number on the points: 
π/3 on С1 and
The vertices of the orange square are the midpoints of the arcs of each quarter, so the length of the arc A1D1 is equal to π/4, and hence π/4 is one of the numbers of the point D1. Using the properties of the number circle, we can write down all the numbers at all the marked points of our circle using formulas. The figure also shows the coordinates of these points (we omit the description of their acquisition).
Having learned the above, we now have sufficient preparation for solving special cases (for nine values of the number a) the simplest equations.
Solve Equations
1)sinx=1⁄(2).
– What is required of us?
– Find all those numbers x whose sine is 1/2.
Recall the definition of sine: sinx - the ordinate of the point of the number circle, on which the number x is located. On the circle we have two points, the ordinate of which is equal to 1/2. These are the ends of the horizontal chord B1B2. This means that the requirement “solve the equation sinx=1⁄2” is equivalent to the requirement “find all numbers at point B1 and all numbers at point B2”.

2)sinx=-√3⁄2 .
We need to find all the numbers at the points C4 and C3.

3) sinx=1. On the circle we have only one point with ordinate 1 - point A2 and, therefore, we need to find only all the numbers of this point.
Answer: x=π/2+2πk , k∈Z .
4)sinx=-1 .
Only point A_4 has ordinate -1. All numbers of this point will be the horses of the equation.
Answer: x=-π/2+2πk , k∈Z .
5) sinx=0 .
On the circle we have two points with ordinate 0 - points A1 and A3. You can specify the numbers on each of the points separately, but given that these points are diametrically opposed, it is better to combine them into one formula: x=πk ,k∈Z .
Answer: x=πk ,k∈Z .
6)cosx=√2⁄2 .
Recall the definition of cosine: cosx - abscissa of the point of the numerical circle on which the number x is located. On the circle we have two points with the abscissa √2⁄2 - the ends of the horizontal chord D1D4. We need to find all the numbers at these points. We write them down by combining them into one formula.
Answer: x=±π/4+2πk , k∈Z .
7) cosx=-1⁄2 .
We need to find the numbers at the points C_2 and C_3 .
Answer: x=±2π/3+2πk , k∈Z .

10) cosx=0 .
Only points A2 and A4 have abscissa 0, which means that all numbers at each of these points will be solutions to the equation.  .
.

The solutions of the equation of the system are the numbers at the points B_3 and B_4. Inequality cosx<0 удовлетворяют только числа b_3
Answer: x=-5π/6+2πk , k∈Z .
Note that for any admissible value of x, the second factor is positive and, therefore, the equation is equivalent to the system

The solutions of the system equation are the number of points D_2 and D_3 . The numbers of the point D_2 do not satisfy the inequality sinx≤0.5, but the numbers of the point D_3 do.

blog.site, with full or partial copying of the material, a link to the source is required.
The main methods for solving trigonometric equations are: reducing equations to the simplest ones (using trigonometric formulas), introducing new variables, and factoring. Let's consider their application with examples. Pay attention to the registration of the solution of trigonometric equations.
A necessary condition for the successful solution of trigonometric equations is the knowledge of trigonometric formulas (topic 13 of work 6).
Examples.
1. Equations Reducing to the Simplest.
1) Solve the equation
Solution:
Answer:
2) Find the roots of the equation
(sinx + cosx) 2 = 1 – sinxcosx belonging to the segment .
Solution:
Answer:
2. Equations Reducing to Quadratic Equations.
1) Solve the equation 2 sin 2 x - cosx -1 = 0.
Solution: Using the formula sin 2 x \u003d 1 - cos 2 x, we get
Answer:
2) Solve the equation cos 2x = 1 + 4 cosx.
Solution: Using the formula cos 2x = 2 cos 2 x - 1, we get
Answer:
3) Solve the equation tgx - 2ctgx + 1 = 0
Solution:
Answer:
3. Homogeneous equations
1) Solve the equation 2sinx - 3cosx = 0
Solution: Let cosx = 0, then 2sinx = 0 and sinx = 0 - a contradiction with the fact that sin 2 x + cos 2 x = 1. So cosx ≠ 0 and you can divide the equation by cosx. Get
Answer:
2) Solve the equation 1 + 7 cos 2 x = 3 sin 2x
Solution:
Using the formulas 1 = sin 2 x + cos 2 x and sin 2x = 2 sinxcosx, we get
sin2x + cos2x + 7cos2x = 6sinxcosx
sin2x - 6sinxcosx+ 8cos2x = 0
Let cosx = 0, then sin 2 x = 0 and sinx = 0 - a contradiction with the fact that sin 2 x + cos 2 x = 1.
So cosx ≠ 0 and we can divide the equation by cos 2 x .
Get
tg 2x – 6 tgx + 8 = 0
Denote tgx = y
y 2 – 6 y + 8 = 0
y 1 = 4; y2=2
a) tanx = 4, x= arctg4 + 2 k, k
b) tgx = 2, x= arctg2 + 2 k, k .
Answer: arctg4 + 2 k, arctan2 + 2 k, k
4. Equations of the form a sinx + b cosx = with, with≠ 0.
1) Solve the equation.
Solution:
Answer:
5. Equations Solved by Factorization.
1) Solve the equation sin2x - sinx = 0.
The root of the equation f (X) = φ ( X) can only serve as the number 0. Let's check this:
cos 0 = 0 + 1 - the equality is true.
The number 0 is the only root of this equation.
Answer: 0.
You can order a detailed solution to your problem !!!
An equality containing an unknown under the sign of a trigonometric function (`sin x, cos x, tg x` or `ctg x`) is called a trigonometric equation, and we will consider their formulas further.
The simplest equations are `sin x=a, cos x=a, tg x=a, ctg x=a`, where `x` is the angle to be found, `a` is any number. Let's write the root formulas for each of them.
1. Equation `sin x=a`.
For `|a|>1` it has no solutions.
With `|a| \leq 1` has an infinite number of solutions.
Root formula: `x=(-1)^n arcsin a + \pi n, n \in Z`
2. Equation `cos x=a`
For `|a|>1` - as in the case of the sine, there are no solutions among real numbers.
With `|a| \leq 1` has infinite set solutions.
Root formula: `x=\pm arccos a + 2\pi n, n \in Z`

Special cases for sine and cosine in graphs. 
3. Equation `tg x=a`
Has an infinite number of solutions for any values of `a`.
Root formula: `x=arctg a + \pi n, n \in Z`

4. Equation `ctg x=a`
It also has an infinite number of solutions for any values of `a`.
Root formula: `x=arcctg a + \pi n, n \in Z`

Formulas for the roots of trigonometric equations in the table
For sinus:  For cosine:
For cosine:  For tangent and cotangent:
For tangent and cotangent:  Formulas for solving equations containing inverse trigonometric functions:
Formulas for solving equations containing inverse trigonometric functions:

Methods for solving trigonometric equations
The solution of any trigonometric equation consists of two stages:
- using to convert it to the simplest;
- solve the resulting simple equation using the above formulas for the roots and tables.
Let's consider the main methods of solution using examples.
algebraic method.
In this method, the replacement of a variable and its substitution into equality is done.
Example. Solve the equation: `2cos^2(x+\frac \pi 6)-3sin(\frac \pi 3 - x)+1=0`
`2cos^2(x+\frac \pi 6)-3cos(x+\frac \pi 6)+1=0`,
make a replacement: `cos(x+\frac \pi 6)=y`, then `2y^2-3y+1=0`,
we find the roots: `y_1=1, y_2=1/2`, from which two cases follow:
1. `cos(x+\frac \pi 6)=1`, `x+\frac \pi 6=2\pi n`, `x_1=-\frac \pi 6+2\pi n`.
2. `cos(x+\frac \pi 6)=1/2`, `x+\frac \pi 6=\pm arccos 1/2+2\pi n`, `x_2=\pm \frac \pi 3- \frac \pi 6+2\pi n`.
Answer: `x_1=-\frac \pi 6+2\pi n`, `x_2=\pm \frac \pi 3-\frac \pi 6+2\pi n`.
Factorization.
Example. Solve the equation: `sin x+cos x=1`.
Solution. Move to the left all terms of equality: `sin x+cos x-1=0`. Using , we transform and factorize the left side:
`sin x - 2sin^2 x/2=0`,
`2sin x/2 cos x/2-2sin^2 x/2=0`,
`2sin x/2 (cos x/2-sin x/2)=0`,
- `sin x/2 =0`, `x/2 =\pi n`, `x_1=2\pi n`.
- `cos x/2-sin x/2=0`, `tg x/2=1`, `x/2=arctg 1+ \pi n`, `x/2=\pi/4+ \pi n` , `x_2=\pi/2+ 2\pi n`.
Answer: `x_1=2\pi n`, `x_2=\pi/2+ 2\pi n`.
Reduction to a homogeneous equation
First, you need to bring this trigonometric equation to one of two forms:
`a sin x+b cos x=0` (homogeneous equation of the first degree) or `a sin^2 x + b sin x cos x +c cos^2 x=0` (homogeneous equation of the second degree).
Then split both parts by `cos x \ne 0` for the first case, and by `cos^2 x \ne 0` for the second. We get equations for `tg x`: `a tg x+b=0` and `a tg^2 x + b tg x +c =0`, which must be solved using known methods.
Example. Solve the equation: `2 sin^2 x+sin x cos x - cos^2 x=1`.
Solution. Let's write the right side as `1=sin^2 x+cos^2 x`:
`2 sin^2 x+sin x cos x — cos^2 x=` `sin^2 x+cos^2 x`,
`2 sin^2 x+sin x cos x - cos^2 x -` ` sin^2 x - cos^2 x=0`
`sin^2 x+sin x cos x - 2 cos^2 x=0`.
This is a homogeneous trigonometric equation of the second degree, dividing its left and right sides by `cos^2 x \ne 0`, we get:
`\frac (sin^2 x)(cos^2 x)+\frac(sin x cos x)(cos^2 x) - \frac(2 cos^2 x)(cos^2 x)=0`
`tg^2 x+tg x - 2=0`. Let's introduce the replacement `tg x=t`, as a result `t^2 + t - 2=0`. The roots of this equation are `t_1=-2` and `t_2=1`. Then:
- `tg x=-2`, `x_1=arctg (-2)+\pi n`, `n \in Z`
- `tg x=1`, `x=arctg 1+\pi n`, `x_2=\pi/4+\pi n`, ` n \in Z`.
Answer. `x_1=arctg (-2)+\pi n`, `n \in Z`, `x_2=\pi/4+\pi n`, `n \in Z`.
Go to Half Corner
Example. Solve the equation: `11 sin x - 2 cos x = 10`.
Solution. Let's apply the formulas double angle, resulting in: `22 sin (x/2) cos (x/2) -` `2 cos^2 x/2 + 2 sin^2 x/2=` `10 sin^2 x/2+10 cos^ 2x/2`
`4 tg^2 x/2 - 11 tg x/2 +6=0`
Applying the above algebraic method, we get:
- `tg x/2=2`, `x_1=2 arctg 2+2\pi n`, `n \in Z`,
- `tg x/2=3/4`, `x_2=arctg 3/4+2\pi n`, `n \in Z`.
Answer. `x_1=2 arctg 2+2\pi n, n \in Z`, `x_2=arctg 3/4+2\pi n`, `n \in Z`.
Introduction of an auxiliary angle
In the trigonometric equation `a sin x + b cos x =c`, where a,b,c are coefficients and x is a variable, we divide both parts by `sqrt (a^2+b^2)`:
`\frac a(sqrt (a^2+b^2)) sin x +` `\frac b(sqrt (a^2+b^2)) cos x =` `\frac c(sqrt (a^2 +b^2))`.
The coefficients on the left side have the properties of sine and cosine, namely, the sum of their squares is 1 and their modulus is at most 1. Let's denote them as follows: `\frac a(sqrt (a^2+b^2))=cos \varphi` , ` \frac b(sqrt (a^2+b^2)) =sin \varphi`, `\frac c(sqrt (a^2+b^2))=C`, then:
`cos \varphi sin x + sin \varphi cos x =C`.
Let's take a closer look at the following example:
Example. Solve the equation: `3 sin x+4 cos x=2`.
Solution. Dividing both sides of the equation by `sqrt (3^2+4^2)`, we get:
`\frac (3 sin x) (sqrt (3^2+4^2))+` `\frac(4 cos x)(sqrt (3^2+4^2))=` `\frac 2(sqrt (3^2+4^2))`
`3/5 sin x+4/5 cos x=2/5`.
Denote `3/5 = cos \varphi` , `4/5=sin \varphi`. Since `sin \varphi>0`, `cos \varphi>0`, we take `\varphi=arcsin 4/5` as an auxiliary angle. Then we write our equality in the form:
`cos \varphi sin x+sin \varphi cos x=2/5`
Applying the formula for the sum of angles for the sine, we write our equality in the following form:
`sin(x+\varphi)=2/5`,
`x+\varphi=(-1)^n arcsin 2/5+ \pi n`, `n \in Z`,
`x=(-1)^n arcsin 2/5-` `arcsin 4/5+ \pi n`, `n \in Z`.
Answer. `x=(-1)^n arcsin 2/5-` `arcsin 4/5+ \pi n`, `n \in Z`.
Fractional-rational trigonometric equations
These are equalities with fractions, in the numerators and denominators of which there are trigonometric functions.
Example. Solve the equation. `\frac (sin x)(1+cos x)=1-cos x`.
Solution. Multiply and divide the right side of the equation by `(1+cos x)`. As a result, we get:
`\frac (sin x)(1+cos x)=` `\frac ((1-cos x)(1+cos x))(1+cos x)`
`\frac (sin x)(1+cos x)=` `\frac (1-cos^2 x)(1+cos x)`
`\frac (sin x)(1+cos x)=` `\frac (sin^2 x)(1+cos x)`
`\frac (sin x)(1+cos x)-` `\frac (sin^2 x)(1+cos x)=0`
`\frac (sin x-sin^2 x)(1+cos x)=0`
Given that the denominator cannot be zero, we get `1+cos x \ne 0`, `cos x \ne -1`, ` x \ne \pi+2\pi n, n \in Z`.
Equate the numerator of the fraction to zero: `sin x-sin^2 x=0`, `sin x(1-sin x)=0`. Then `sin x=0` or `1-sin x=0`.
- `sin x=0`, `x=\pi n`, `n \in Z`
- `1-sin x=0`, `sin x=-1`, `x=\pi /2+2\pi n, n \in Z`.
Given that ` x \ne \pi+2\pi n, n \in Z`, the solutions are `x=2\pi n, n \in Z` and `x=\pi /2+2\pi n` , `n \in Z`.
Answer. `x=2\pi n`, `n \in Z`, `x=\pi /2+2\pi n`, `n \in Z`.
Trigonometry, and trigonometric equations in particular, are used in almost all areas of geometry, physics, and engineering. The study begins in the 10th grade, there are always tasks for the exam, so try to remember all the formulas of trigonometric equations - they will definitely come in handy for you!
However, you don’t even need to memorize them, the main thing is to understand the essence, and be able to deduce. It's not as difficult as it seems. See for yourself by watching the video.
Your privacy is important to us. For this reason, we have developed a Privacy Policy that describes how we use and store your information. Please read our privacy policy and let us know if you have any questions.
Collection and use of personal information
Personal information refers to data that can be used to identify or contact a specific person.
You may be asked to provide your personal information at any time when you contact us.
The following are some examples of the types of personal information we may collect and how we may use such information.
What personal information we collect:
- When you submit an application on the site, we may collect various information, including your name, phone number, address Email etc.
How we use your personal information:
- Collected by us personal information allows us to contact you and inform you about unique offers, promotions and other events and upcoming events.
- From time to time, we may use your personal information to send you important notices and communications.
- We may also use personal information for internal purposes, such as conducting audits, data analysis and various research in order to improve the services we provide and provide you with recommendations regarding our services.
- If you enter a prize draw, contest or similar incentive, we may use the information you provide to administer such programs.
Disclosure to third parties
We do not disclose information received from you to third parties.
Exceptions:
- In the event that it is necessary - in accordance with the law, judicial order, in legal proceedings, and / or based on public requests or requests from state bodies in the territory of the Russian Federation - disclose your personal information. We may also disclose information about you if we determine that such disclosure is necessary or appropriate for security, law enforcement, or other public interest reasons.
- In the event of a reorganization, merger or sale, we may transfer the personal information we collect to the relevant third party successor.
Protection of personal information
We take precautions - including administrative, technical and physical - to protect your personal information from loss, theft, and misuse, as well as from unauthorized access, disclosure, alteration and destruction.
Maintaining your privacy at the company level
To ensure that your personal information is secure, we communicate privacy and security practices to our employees and strictly enforce privacy practices.
The video course "Get an A" includes all the topics necessary for a successful passing the exam in mathematics for 60-65 points. Completely all tasks 1-13 of the Profile USE in mathematics. Also suitable for passing the Basic USE in mathematics. If you want to pass the exam with 90-100 points, you need to solve part 1 in 30 minutes and without mistakes!
Preparation course for the exam for grades 10-11, as well as for teachers. Everything you need to solve part 1 of the exam in mathematics (the first 12 problems) and problem 13 (trigonometry). And this is more than 70 points on the Unified State Examination, and neither a hundred-point student nor a humanist can do without them.
All the necessary theory. Quick Ways solutions, traps and secrets of the exam. All relevant tasks of part 1 from the Bank of FIPI tasks have been analyzed. The course fully complies with the requirements of the USE-2018.
The course contains 5 big topics, 2.5 hours each. Each topic is given from scratch, simply and clearly.
Hundreds of exam tasks. Text problems and probability theory. Simple and easy to remember problem solving algorithms. Geometry. Theory, reference material, analysis of all types of USE tasks. Stereometry. Cunning tricks for solving, useful cheat sheets, development of spatial imagination. Trigonometry from scratch - to task 13. Understanding instead of cramming. Visual explanation of complex concepts. Algebra. Roots, powers and logarithms, function and derivative. Base for solving complex problems of the 2nd part of the exam.