Chapter 2
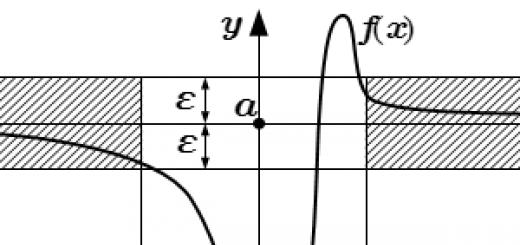
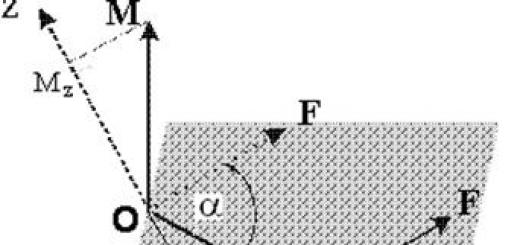
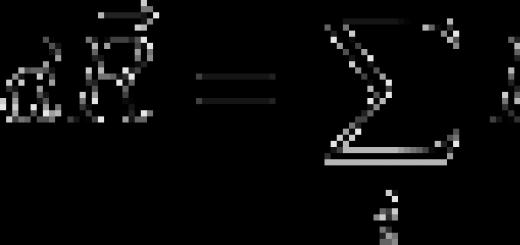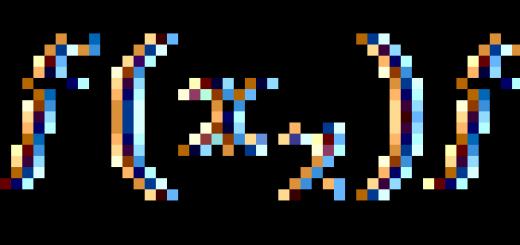3) number

let's match the dot.

The unit circle with the established correspondence will be called

number circle.

This is the second geometric model for the set of real

numbers. The first model - the number line - students already know. There is

analogy: for the number line, the correspondence rule (from number to point)

almost verbatim the same. But there is also a fundamental difference - the source

main difficulties in working with a number circle: on a straight line, each

dot corresponds the only number, on a circle it is not. If a

circle corresponds to a number, then it corresponds to all

numbers of the form

Where is the length of the unit circle, and is an integer

Rice. one

a number indicating the number of complete rounds of the circle in one direction or another

side.

This moment is difficult for students. They should be offered

understanding the essence of the real task:

The stadium running track is 400m long, the runner is 100m away

from the starting point. What path did he take? If he just started running, then

ran 100 m; if you managed to run one lap, then - (

Two circles - () ; if you can run

circles, then the path will be (

) . Now you can compare

the result obtained with the expression

Example 1 What numbers does the dot correspond to

number circle

Decision. Since the length of the whole circle

That is the length of her quarter

Therefore, for all numbers of the form

Similarly, it is established which numbers correspond to the points

called respectively the first, second, third,

fourth quarters of the number circle.

All school trigonometry is based on a numerical model

circles. Experience shows that the shortcomings with this model are too

hasty introduction of trigonometric functions do not allow to create

a solid foundation for the successful assimilation of the material. Therefore, not

you need to hurry, and take some time to consider the following

five different types of problems with a number circle.

The first type of tasks. Finding points on the numerical circle,

corresponding to given numbers, expressed in fractions of a number

Example 2

numbers

Decision. Let's split the arc

in half with a point into three equal parts -

dots

(Fig. 2). Then

So the number

Corresponding point

number
Example

3.
on the

numerical

circles

points,

corresponding numbers:

Decision. We will build

a) Postponing the arc

(its length

) Five times

from the point

in the negative direction

get a point

b) Postponing the arc

(its length

) seven times from

in the positive direction, we get a point separating

third part of the arc

It will correspond to the number

c) Postponing the arc

(its length

) five times from the point

positive

direction, we get a point

Separating the third part of the arc. She and

will match the number

(experience shows that it is better to postpone not

five times over

And 10 times

After this example, it is appropriate to give two main layouts of the numeric

circles: on the first of them (Fig. 3) all quarters are divided in half, on

the second (Fig. 4) - into three equal parts. These layouts are useful to have in the office

mathematics.

Rice. 2

Rice. 3 Rice. 4

Be sure to discuss with students the question: what will happen if

each of the layouts move not in positive, but in negative

direction? On the first layout, the selected points will have to be assigned

other "names": respectively

etc.; on the second layout:

The second type of tasks. Finding points on the numerical circle,

corresponding to given numbers, not expressed in fractions of a number

Example 4 Find points on the number circle corresponding to

numbers 1; 2; 3; -5.

Decision.

Here we have to rely on the fact that

Therefore point 1

located on the arc

closer to the point

Points 2 and 3 are on the arc, the first one is

The second is closer to (Fig. 5).

Let's take a closer look

on finding the point corresponding to the number - 5.

Move from a point

in the negative direction, i.e. clockwise

Rice. 5

arrow. If we go in this direction to the point

Get

This means that the point corresponding to the number - 5 is located

slightly to the right of the dot

(see fig.5).

The third type of tasks. Preparation of analytical records (double

inequalities) for arcs of a numerical circle.

In fact, we are acting on

the same plan that was used in 5-8

classes for studying the number line:

first find a point by number, then by

dot - number, then use double

inequalities for writing gaps on

number line.

Consider, for example, an open

Where is the middle of the first

quarters of a number circle, and

- its middle

second quarter (Fig. 6).

The inequalities characterizing the arc, i.e. representing

An analytical model of the arc is proposed to be compiled in two stages. On the first

stage constitute the core analytical record(this is the main thing to follow

teach students) for a given arc

On the second

stage make up a general record:

If we are talking about arc

Then, when writing the kernel, you need to take into account that

() lies inside the arc, and therefore you have to move to the beginning of the arc

in the negative direction. Hence, the kernel of the analytical notation of the arc

has the form

Rice. 6

The terms "kernel of the analytical

arc records", "analytical record

arcs" are not generally accepted,

considerations.

Fourth

tasks.

Finding

Cartesian

coordinates

number circle points, center

which is combined with the beginning of the system

coordinates.

Let us first consider one rather subtle point, until now

practically not mentioned in current school textbooks.

Starting to study the model "numerical circle on a coordinate

plane", teachers should be clearly aware of what difficulties await

students here. These difficulties are related to the fact that in the study of this

models from schoolchildren are required to have a sufficiently high level

mathematical culture, because they have to work simultaneously in

two coordinate systems - in the "curvilinear", when information about

the position of the point is taken along the circle (number

corresponds to

circle point

(); is the “curvilinear coordinate” of the point), and in

Cartesian rectangular coordinate system (at the point

Like every point

coordinate plane, there is an abscissa and an ordinate). The task of the teacher is to help

schoolchildren in overcoming these natural difficulties. Unfortunately,

usually in school textbooks they do not pay attention to this and from the very

first lessons use notes

Not considering that the letter in

in the mind of a schoolchild is clearly associated with the abscissa in the Cartesian

rectangular coordinate system, and not with the length traveled along the numerical

path circles. Therefore, when working with a number circle, one should not

use symbols

Rice. 7

Let's return to the fourth type of tasks. It's about about the transition from recording

records

(), i.e. from curvilinear to cartesian coordinates.

Compatible number circle with Cartesian rectangular system

coordinates as shown in Fig. 7. Then dots

will have

the following coordinates:

() () () (). Very important

teach students to determine the coordinates of all those points that

marked on two main layouts (see Fig.3,4). For point

It all comes down to

consideration of the isosceles right triangle with hypotenuse

His legs are equal

So the coordinates

). The same is true for points.

But the only difference is that you need to take into account

abscissa and ordinate signs. Specifically:

What should students remember? Only that the modules of the abscissa and

the ordinates at the midpoints of all quarters are equal

And they must know the signs

determine for each point directly from the drawing.

For point

It all comes down to considering a rectangular

triangle with hypotenuse 1 and angle

(Fig. 9). Then the cathet

opposite corner

Will be equal

adjacent

√

Means,

point coordinates

The same is true for the point

only the legs "change places", and therefore

Rice. eight

Rice. nine

we get

). It is the meanings

(up to signs) and will be

“serve” all points of the second layout (see Fig. 4), except for points

as abscissa and ordinate. Suggested way of remembering: "where is shorter,

; where it is longer

Example 5 Find coordinates of a point

(see Fig.4).

Decision. Dot

Closer to the vertical axis than to

horizontal, i.e. the modulus of its abscissa is less than the modulus of its ordinate.

So the modulus of the abscissa is

The module of the ordinate is

signs in both

cases are negative (third quarter). Conclusion: dot

Has coordinates

In the fourth type of problems, one finds Cartesian coordinates all

points presented on the first and second layouts mentioned

In fact, in the course of this type of tasks, we prepare students for

calculation of values ​​of trigonometric functions. If everything is here

worked out quite reliably, then the transition to a new level of abstraction

(ordinate - sine, abscissa - cosine) will be less painful than

The fourth type includes tasks of this type: for a point

find signs of cartesian coordinates

The decision should not cause difficulties for students: the number

match point

Fourth quarter means .

Fifth type of tasks. Finding points on the numerical circle by

given coordinates.

Example 6 Find points with ordinate on a number circle

write down what numbers they correspond to.

Decision. Straight

Crosses the number circle at points
(Fig. 11). With the help of the second layout (see Fig. 4) we set that the point

corresponds to the number

So she

matches all numbers of the form
corresponds to the number

And that means

all numbers of the form

Answer:

Example 7 Find on numeric

circle point with abscissa

write down what numbers they correspond to.

Decision. Straight
√

intersects the number circle at points

- in the middle of the second and third quarters (Fig. 10). With the help of the first

layout set that point

corresponds to the number

And that means everyone

numbers of the form

corresponds to the number

And that means everyone

numbers of the form

Answer:

You must show the second option.

record the answer for example 7. After all, the point

corresponds to the number

Those. all numbers of the form

we get:

Rice. ten

Fig.11

Emphasize the undeniable importance

the fifth type of tasks. In fact, we teach

schoolchildren

decision

protozoa

trigonometric equations: in example 6

it's about the equation

And in the example

- about the equation

understanding of the essence of the matter is important to teach

schoolchildren solve equations of the types

along the number circle

don't rush into formulas

Experience shows that if the first stage (work on

numerical circle) is not worked out reliably enough, then the second stage

(work on formulas) is perceived by schoolchildren formally, that,

Naturally, it must be overcome.

Similar to examples 6 and 7 should be found on the number circle

points with all "major" ordinates and abscissas

As special subjects, it is appropriate to single out the following:

Remark 1. In propaedeutic terms, preparatory

work on the topic "Length of a circle" in the course of geometry of the 9th grade. Important

advice: the system of exercises should include tasks of the type proposed

below. The unit circle is divided into four equal parts by points

the arc is bisected by a point and the arc is bisected by points

into three equal parts (Fig. 12). What are the lengths of the arcs

(it is assumed that the circumnavigation of the circle is carried out in a positive

direction)?

Rice. 12

The fifth type of tasks includes working with conditions like

means
to

decision

protozoa

trigonometric inequalities, we also “fit” gradually.

five lessons and only in the sixth lesson should the definitions of sine and

cosine as the coordinates of a point on a numerical circle. Wherein

it is advisable to solve all types of problems with schoolchildren again, but with

using the introduced notation, offering to perform such

for example, tasks: calculate

solve the equation

inequality

etc. We emphasize that in the first lessons

trigonometry protozoa trigonometric equations and inequalities

are not purpose training, but used as facilities for

mastering the main thing - the definitions of sine and cosine as coordinates of points

number circle.

Let the number

match point

number circle. Then its abscissa

called cosine of a number

and denoted

And its ordinate is called the sine of a number

and is marked. (Fig. 13).

From this definition one can immediately

set the signs of sine and cosine according to

quarters: for sine

For cosine

Dedicate a whole lesson to this (as it is

accepted) is hardly appropriate. Do not do it

force schoolchildren to memorize these signs: any mechanical

memorization, memorization is a violent technique to which students,

When studying trigonometry at school, each student is faced with a very interesting concept of "numerical circle". It depends on the ability of the school teacher to explain what it is and why it is needed, how well the student will go about trigonometry later. Unfortunately, not every teacher can explain this material in an accessible way. As a result, many students get confused even with how to celebrate points on the number circle. If you read this article to the end, you will learn how to do it without problems.

So let's get started. Let's draw a circle, the radius of which is equal to 1. The most "right" point of this circle will be denoted by the letter O:

Congratulations, you have just drawn a unit circle. Since the radius of this circle is 1, then its length is .

Each real number can be associated with the length of the trajectory along the number circle from the point O. The direction of movement is counterclockwise as the positive direction. For negative - clockwise:

Arrangement of points on a number circle

As we have already noted, the length of the numerical circle (unit circle) is equal to. Where then will the number be located on this circle? Obviously from the point O counterclockwise, you need to go half the length of the circle, and we will find ourselves at the desired point. Let's denote it with a letter B:

Note that the same point could be reached by passing the semicircle in the negative direction. Then we would put the number on the unit circle. That is, the numbers and correspond to the same point.

Moreover, the same point also corresponds to the numbers , , , and, in general, infinite set numbers that can be written as , where , that is, it belongs to the set of integers. All this is because from the point B you can make a "round the world" trip in any direction (add or subtract the circumference) and get to the same point. We get an important conclusion that needs to be understood and remembered.

Each number corresponds to a single point on the number circle. But each point on the number circle corresponds to infinitely many numbers.

Let us now divide the upper semicircle of the numerical circle into arcs of equal length with a point C. It is easy to see that the arc length OC is equal to . Let's put aside now from the point C an arc of the same length in a counterclockwise direction. As a result, we get to the point B. The result is quite expected, since . Let's postpone this arc in the same direction again, but now from the point B. As a result, we get to the point D, which will already match the number :

Note again that this point corresponds not only to the number , but also, for example, to the number , because this point can be reached by setting aside from the point O quarter circle in the clockwise direction (in the negative direction).

And, in general, we note again that this point corresponds to an infinite number of numbers that can be written in the form . But they can also be written as . Or, if you like, in the form of . All these records are absolutely equivalent, and they can be obtained from one another.

Let us now break the arc into OC halved dot M. Think now what is the length of the arc OM? That's right, half the arc OC. I.e . What numbers does the dot correspond to M on a number circle? I am sure that now you will realize that these numbers can be written in the form.

But it is possible otherwise. Let's take in the presented formula. Then we get that . That is, these numbers can be written as . The same result could be obtained using a number circle. As I said, both entries are equivalent, and they can be obtained from one another.

Now you can easily give an example of numbers that correspond to points N, P and K on the number circle. For example, numbers , and :

Often it is precisely the minimal positive numbers that are taken to designate the corresponding points on the number circle. Although this is not at all necessary, and the point N, as you already know, corresponds to an infinite number of other numbers. Including, for example, the number .

If you break the arc OC into three equal arcs with dots S and L, so the point S will lie between the points O and L, then the arc length OS will be equal to , and the length of the arc OL will be equal to . Using the knowledge that you received in the previous part of the lesson, you can easily figure out how the rest of the points on the number circle turned out:

Numbers that are not multiples of π on the number circle

Let us now ask ourselves the question, where on the number line to mark the point corresponding to the number 1? To do this, it is necessary from the most "right" point of the unit circle O set aside an arc whose length would be equal to 1. We can only approximately indicate the location of the desired point. Let's proceed as follows.

In this article, we will analyze in great detail the definition of a numerical circle, find out its main property and arrange the numbers 1,2,3, etc. About how to mark other numbers on the circle (for example, \(\frac(π)(2), \frac(π)(3), \frac(7π)(4), 10π, -\frac(29π)( 6)\)) understands .

Number circle call a circle of unit radius, the points of which correspond to arranged according to the following rules:

1) The origin is at the extreme right point of the circle;

2) Counterclockwise - positive direction; clockwise - negative;

3) If we plot the distance \(t\) on the circle in the positive direction, then we will get to the point with the value \(t\);

4) If we plot the distance \(t\) on the circle in the negative direction, then we will get to the point with the value \(–t\).

Why is a circle called a number?
Because it has numbers on it. In this, the circle is similar to the number axis - on the circle, as well as on the axis, for each number there is a certain point.

Why know what a number circle is?
With the help of a numerical circle, the value of sines, cosines, tangents and cotangents is determined. Therefore, for knowledge of trigonometry and passing the exam for 60+ points, you definitely need to understand what a number circle is and how to dot it.

What do the words "... of unit radius ..." mean in the definition?
This means that the radius of this circle is \(1\). And if we construct such a circle centered at the origin, then it will intersect with the axes at the points \(1\) and \(-1\).

It is not necessary to draw it small, you can change the “size” of divisions along the axes, then the picture will be larger (see below).

Why is the radius exactly one? It’s more convenient, because in this case, when calculating the circumference using the formula \(l=2πR\), we get:

The length of the number circle is \(2π\) or approximately \(6,28\).

And what does "... the points of which correspond to real numbers" mean?
As mentioned above, on the number circle for any real number, there will definitely be its “place” - a point that corresponds to this number.

Why determine the origin and direction on the number circle?
The main purpose of the number circle is to uniquely determine its point for each number. But how can you determine where to put an end if you don’t know where to count from and where to move?

Here it is important not to confuse the origin on the coordinate line and on the number circle - these are two different reference systems! Also, don't confuse \(1\) on the \(x\) axis and \(0\) on the circle - these are points on different objects.

What points correspond to the numbers \(1\), \(2\), etc?

Remember, we assumed that the radius of a number circle is \(1\)? This will be our single segment (by analogy with the number axis), which we will put on the circle.

To mark a point on the number circle corresponding to the number 1, you need to travel from 0 a distance equal to the radius in the positive direction.

To mark a point on the circle corresponding to the number \(2\), you need to travel a distance equal to two radii from the origin, so that \(3\) is a distance equal to three radii, etc.

Looking at this picture, you may have 2 questions:
1. What will happen when the circle "ends" (i.e. we make a full circle)?
Answer: let's go to the second round! And when the second is over, we will go to the third and so on. Therefore, an infinite number of numbers can be applied to a circle.

2. Where will they be negative numbers?
Answer: right there! They can also be arranged, counting from zero the required number of radii, but now in a negative direction.

Unfortunately, it is difficult to designate integers on the number circle. This is due to the fact that the length of the numerical circle will not be an integer: \ (2π \). And at the most convenient places (at the points of intersection with the axes) there will also be not integers, but fractions

We present to your attention a video lesson on the topic "Numeric Circle". A definition is given of what sine, cosine, tangent, cotangent and functions are y= sin x, y= cos x, y= tg x, y= ctg x for any numeric argument. We consider standard tasks for the correspondence between numbers and points in a unit number circle to find a single point for each number, and, conversely, to find for each point a set of numbers that correspond to it.

Topic: Elements of the theory of trigonometric functions

Lesson: Number Circle

Our next goal is to determine trigonometric functions: sinus, cosine, tangent, cotangent-

Numeric argument can be plotted on a coordinate line or on a circle.

Such a circle is called a numerical or unit circle, because. for convenience, take a circle with

For example, given a point, mark it on the coordinate line

and on number circle.

When working with a number circle, it was agreed that counterclockwise movement is a positive direction, clockwise movement is negative.

Typical tasks - you need to determine the coordinates given point or, conversely, find a point by its coordinates.

The coordinate line establishes a one-to-one correspondence between points and numbers. For example, a number corresponds to point A with coordinate

Each point B with a coordinate is characterized by only one number - the distance from 0 to taken with a plus or minus sign.

On the number circle, one-to-one correspondence only works in one direction.

For example, there is a point B on coordinate circle(Fig. 2), the arc length is equal to 1, i.e. this point corresponds to 1.

Given a circle, the circumference of a circle. If then is the length of the unit circle.

If we add , we get the same point B, more - we also get to point B, subtract - also point B.

Consider point B: arc length =1, then the numbers characterize point B on the number circle.

Thus, the number 1 corresponds to the only point of the numerical circle - point B, and the point B corresponds to an uncountable set of points of the form .

The following is true for a number circle:

If T. M number circle corresponds to a number then it also corresponds to a number of the form

You can make as many full turns around the number circle in a positive or negative direction as you like - the point is the same. Therefore, trigonometric equations have an infinite number of solutions.

For example, given point D. What are the numbers it corresponds to?

We measure the arc.

the set of all numbers corresponding to the point D.

Consider the main points on the number circle.

The length of the whole circle.

Those. the record of the set of coordinates can be different .

Consider typical tasks on the number circle.

1. Given: . Find: a point on a number circle.

We select the whole part:

It is necessary to find m. on the number circle. , then .

This set also includes the point .

2. Given: . Find: a point on a number circle.

Need to find t.

m. also belongs to this set.

Solving standard problems on the correspondence between numbers and points on a number circle, we found out that it is possible to find a single point for each number, and it is possible to find for each point a set of numbers that are characterized by a given point.

Let's divide the arc into three equal parts and mark the points M and N.

Let's find all the coordinates of these points.

So, our goal is to define trigonometric functions. To do this, we need to learn how to set a function argument. We considered the points of the unit circle and solved two typical problems - to find a point on the number circle and write down all the coordinates of the point of the unit circle.

1. Mordkovich A.G. and others. Algebra 9th grade: Proc. For general education Institutions. - 4th ed. - M.: Mnemosyne, 2002.-192 p.: ill.

2. Mordkovich A.G. and others. Algebra Grade 9: Task book for students educational institutions/ A. G. Mordkovich, T. N. Mishustina and others - 4th ed. — M.: Mnemosyne, 2002.-143 p.: ill.

3. Yu. N. Makarychev, Algebra. Grade 9: textbook for general education students. institutions / Yu. N. Makarychev, N. G. Mindyuk, K. I. Neshkov, I. E. Feoktistov. - 7th ed., Rev. and additional - M .: Mnemosyne, 2008.

4. Alimov Sh.A., Kolyagin Yu.M., Sidorov Yu.V. Algebra. Grade 9 16th ed. - M., 2011. - 287 p.

5. Mordkovich A. G. Algebra. Grade 9 At 2 pm Part 1. Textbook for students of educational institutions / A. G. Mordkovich, P. V. Semenov. - 12th ed., erased. — M.: 2010. — 224 p.: ill.

6. Algebra. Grade 9 At 2 hours. Part 2. Task book for students of educational institutions / A. G. Mordkovich, L. A. Aleksandrova, T. N. Mishustina and others; Ed. A. G. Mordkovich. - 12th ed., Rev. — M.: 2010.-223 p.: ill.

Mordkovich A.G. et al. Algebra Grade 9: Taskbook for students of educational institutions / A. G. Mordkovich, T. N. Mishustina et al. - 4th ed. - M .: Mnemosyne, 2002.-143 p.: ill.

№№ 531; 536; 537; 541; 552.

Video lessons are among the most effective teaching aids, especially in school disciplines such as mathematics. Therefore, the author this material collected in a single whole only useful, important and competent information.

This lesson is designed for 11:52 minutes. Almost the same amount of time is required for a teacher in a lesson to explain new material on a given topic. Although the main advantage of the video lesson will be the fact that students will carefully listen to what the author is talking about, without being distracted by extraneous topics and conversations. After all, if students do not listen carefully, they will miss an important point in the lesson. And if the material is explained by the teacher himself, then his students can easily distract from the main thing with their conversations on abstract topics. And, of course, it becomes clear which way will be more rational.

The author devotes the beginning of the lesson to the repetition of those functions that students got acquainted with earlier in the course of algebra. And the first is proposed to start studying - trigonometric functions. To consider and study them, a new mathematical model. And this model becomes a numerical circle, which, just, is stated in the topic of the lesson. To do this, the concept of a unit circle is introduced, its definition is given. Further in the figure, the author shows all the components of such a circle, and what is useful for students for further learning. Quarters are marked with arcs.

Then the author proposes to consider the number circle. Here he makes the remark that it is more convenient to use the unit circle. This circle shows how the point M is obtained if t>0, t<0 или t=0. После этого вводится понятие самой числовой окружности.

Further, the author reminds students how to find the circumference of a circle. And then it outputs the length of the unit circle. These theoretical data are proposed to be applied in practice. For this, an example is considered where it is required to find a point on a circle corresponding to certain values ​​of numbers. The solution of the example is accompanied by an illustration in the form of a drawing, as well as the necessary mathematical records.

According to the condition of the second example, it is necessary to find points on the number circle. Here, too, the entire solution is accompanied by comments, illustrations, and mathematical notation. This contributes to the development and improvement of mathematical literacy of students. The third example is constructed similarly.

Further, the author notes those numbers on the circle that occur more often than others. Here he proposes to make two layouts of a numerical circle. When both layouts are ready, the next, fourth example is considered, where it is required to find a point on the number circle corresponding to the number 1. After this example, a statement is formulated according to which one can find the point M corresponding to the number t.

Next, a remark is introduced, according to which the trainees learn that the number "pi" corresponds to all the numbers that fall at a given point when it passes the entire circle. This information is reinforced by the fifth example. His solution contains logically correct reasoning and drawings illustrating the situation.

TEXT INTERPRETATION:

NUMERICAL CIRCLE

Previously, we studied functions defined by analytic expressions. And these functions were called algebraic. But in the school course of mathematics, functions of other classes, not algebraic ones, are also studied. Let's start studying trigonometric functions.

In order to introduce trigonometric functions, we need a new mathematical model - a numerical circle. Consider a unit circle. A circle whose radius is equal to the scale segment, without specifying specific units of measurement, will be called a unit. The radius of such a circle is assumed to be 1.

We will use a unit circle in which the horizontal and vertical diameters CA and DВ (ce a and de be) are drawn (see figure 1).

The arc AB will be called the first quarter, the arc BC the second quarter, the arc CD the third quarter, and the arc DA the fourth quarter.

Consider a number circle. In general, any circle can be considered as a numerical circle, but it is more convenient to use a unit circle for this purpose.

DEFINITION A unit circle is given, the starting point A is marked on it - the right end of the horizontal diameter. Let us assign to each real number t (te) a point of the circle according to the following rule:

1) If t>0 (te is greater than zero), then, moving from point A in a counterclockwise direction (the positive direction of going around the circle), we describe the path AM (a em) of length t around the circle. Point M will be the desired point M (t) (em from te).

2) If t<0(тэ меньше нуля), то, двигаясь из точки А в направлении по часовой стрелке (отрицательное направление обхода окружности), опишем по окружности путь АМ (а эм) длины |t| (модуль тэ). Точка М и будет искомой точкой М(t) (эм от тэ).

3) We put the point A in correspondence with the number t = 0.

A unit circle with an established correspondence (between real numbers and points of a circle) will be called a number circle.

It is known that the circumference L (el) is calculated by the formula L =2πR (el is equal to two pi er), where π≈3.14, R is the radius of the circle. For a unit circle R=1cm, then L=2π≈6.28 cm (el equals two pi approximately 6.28).

Consider examples.

EXAMPLE 1. Find a point on the number circle that corresponds to a given number: ,. (pi by two, pi, three pi by two, two pi, eleven pi by two, seven pi, minus five pi by two)

Decision. The first six numbers are positive, so to find the corresponding points of the circle, you need to go around the circle path of a given length, moving from point A in a positive direction. The length of each quarter of the unit circle is equal. Hence, AB =, that is, the point B corresponds to the number (see Fig. 1). AC \u003d, that is, point C corresponds to the number. AD \u003d, that is, point D corresponds to the number. And point A again corresponds to the number, because having passed the path along the circle, we got to the starting point A.

Consider where the point will be located. Since we already know what the circumference is, we will bring it to the form (four pi plus three pi by two). That is, moving from point A in a positive direction, you need to describe twice a whole circle (a path of length 4π) and additionally a path of length that ends at point D.

What? This is 3∙2π + π (three times two pi plus pi). So, moving from point A in a positive direction, you need to describe three times a whole circle and additionally a path of length π, which will end at point C.

To find a point on a numerical circle corresponding to a negative number, you need to go from point A along the circle in a negative direction (clockwise) a path of length, and this corresponds to 2π +. This path will end at point D.

EXAMPLE 2. Find points on the number circle, (pi by six, pi by four, pi by three).

Decision. Dividing the arc AB in half, we get the point E, which corresponds. And dividing the arc AB into three equal parts by points F and O, we get that the point F corresponds, and the point T corresponds

(see figure 2).

EXAMPLE 3. Find points on the numerical circle, (minus thirteen pi by four, nineteen pi by six).

Decision. Postponing the arc AE (a em) with a length (pi by four) from point A thirteen times in the negative direction, we get the point H (ash) - the middle of the arc BC.

Having postponed the arc AF of length (pi by six) from point A nineteen times in the positive direction, we will get to the point N (en), which belongs to the third quarter (arc CD) and CN is equal to the third part of the arc CD (se de).

(See Figure 2 for example).

Most often, you have to look for points on the number circle that correspond to numbers (pi by six, pi by four, pi by three, pi by two), as well as those that are multiples of them, that is, (seven pi by six, five pi by four, four pi by three, eleven pi by two). Therefore, in order to quickly navigate, it is advisable to make two layouts of a numerical circle.

On the first layout, each of the quarters of the numerical circle will be divided into two equal parts, and next to each of the obtained points we will write their “names”:

On the second layout, each of the quarters is divided into three equal parts, and next to each of the twelve points obtained, we also write their “names”:

If we move clockwise, we will get the same “names” for the points on the drawings, only with a minus value. For the first layout:

Similarly, if you move clockwise along the second layout from point O.

EXAMPLE 4. Find on the number circle the points corresponding to the numbers 1 (one).

Decision. Knowing that π≈3.14 (pi is approximately three point fourteen hundredths), ≈ 1.05 (pi times three is approximately one point five hundredths), ≈ 0.79 (pi times four is approximately zero point seventy nine hundredths) . Means,< 1 < (один больше, чем пи на четыре, но меньше, чем пи на три), то есть число 1 находится в первой четверти.

The following statement is true: if the point M of the number circle corresponds to the number t, then it also corresponds to any number of the form t + 2πk(te plus two pi ka), where ka is any integer and kϵ Z(ka belongs to z).

Using this statement, we can conclude that a point corresponds to all points of the form t =+ 2πk (te equals pi times three plus two peaks), where kϵZ ( ka belongs to zet), and to a point (five pi by four) - points of the form t = + 2πk (te equals five pi by four plus two pi ka), where kϵZ ( ka belongs to z) and so on.

EXAMPLE 5. Find a point on the number circle: a) ; b) .

Decision. a) We have: = =(6 +) ∙ π = 6π + = + 3∙ 2π. (twenty pi times three equals twenty times three pi equals six plus two thirds, multiplied by pi equals six pi plus two pi times three equals two pi times three plus three times two pi).

This means that the number corresponds to the same point on the number circle as the number (this is the second quarter) (see the second layout in Figure 4).

b) We have: = - (8 +) ∙ π = + 2π ∙ (- 4) minus four). That is, the number corresponds on the number circle to the same point as the number