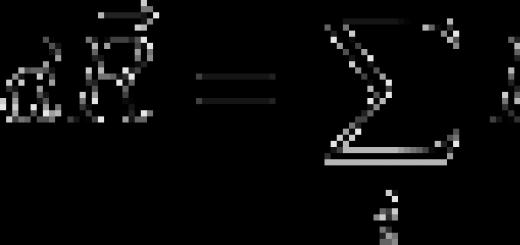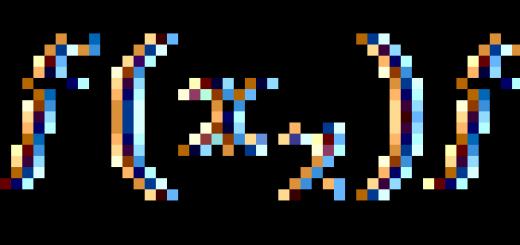In the school course of stereometry, one of the simplest figures that has non-zero dimensions along three spatial axes is a quadrangular prism. Consider in the article what kind of figure it is, what elements it consists of, and also how you can calculate its surface area and volume.

The concept of a prism

In geometry, a prism is a spatial figure, which is formed by two identical bases and side surfaces that connect the sides of these bases. Note that both bases are transformed into each other using the operation of parallel translation by some vector. This task of the prism leads to the fact that all its sides are always parallelograms.

The number of sides of the base can be arbitrary, starting from three. When this number tends to infinity, the prism smoothly turns into a cylinder, since its base becomes a circle, and the side parallelograms, connecting, form a cylindrical surface.

Like any polyhedron, a prism is characterized by sides (planes that bound the figure), edges (segments along which any two sides intersect) and vertices (meeting points of three sides, for a prism two of them are lateral, and the third is the base). The numbers of these three elements of the figure are interconnected by the following expression:

Here P, C and B are the number of edges, sides and vertices, respectively. This expression is a mathematical notation of Euler's theorem.

Above is a picture showing two prisms. At the base of one of them (A) lies a regular hexagon, and the side sides are perpendicular to the bases. Figure B shows another prism. Its sides are no longer perpendicular to the bases, and the base is a regular pentagon.

quadrangular?

As is clear from the description above, the type of prism is primarily determined by the type of polygon that forms the base (both bases are the same, so we can talk about one of them). If this polygon is a parallelogram, then we get a quadrangular prism. So all sides of this are parallelograms. A quadrangular prism has its own name - a parallelepiped.

The number of sides of the parallelepiped is six, with each side having an analogous parallel to it. Since the bases of the parallelepiped are two sides, the remaining four are lateral.

The number of vertices of the parallelepiped is eight, which is easy to see if we remember that the vertices of the prism are formed only at the vertices of the base polygons (4x2=8). Applying Euler's theorem, we obtain the number of edges:

P \u003d C + B - 2 \u003d 6 + 8 - 2 \u003d 12

Of the 12 ribs, only 4 are formed independently by the sides. The remaining 8 lie in the planes of the bases of the figure.

Types of parallelepipeds

The first type of classification lies in the features of the parallelogram that lies at the base. It may look like this:

• ordinary, in which the angles are not equal to 90 o;
• rectangle;
• a square is a regular quadrilateral.

The second type of classification is the angle at which the side crosses the base. Two different cases are possible here:

• this angle is not straight, then the prism is called oblique or oblique;
• the angle is 90 o, then such a prism is rectangular or just straight.

The third type of classification is related to the height of the prism. If the prism is rectangular, and the base is either a square or a rectangle, then it is called a cuboid. If there is a square at the base, the prism is rectangular, and its height is equal to the length of the side of the square, then we get the well-known cube figure.

The surface of the prism and its area

The set of all points that lie on the two bases of the prism (parallelograms) and on its sides (four parallelograms) form the surface of the figure. The area of ​​this surface can be calculated by calculating the area of ​​the base and this value for the side surface. Then their sum will give the desired value. Mathematically, this is written like this:

Here S o and S b are the area of ​​the base and side surface, respectively. The number 2 before S o appears because there are two bases.

Note that the written formula is valid for any prism, and not just for the area of ​​a quadrangular prism.

It is useful to recall that the area of ​​the parallelogram S p is calculated by the formula:

Where the symbols a and h denote the length of one of its sides and the height drawn to this side, respectively.

Area of ​​a rectangular prism with a square base

The base is a square. For definiteness, we denote its side by the letter a. To calculate the area of ​​a regular quadrangular prism, you should know its height. According to the definition for this quantity, it is equal to the length of the perpendicular dropped from one base to another, that is, equal to the distance between them. Let's denote it by the letter h. Since all side faces are perpendicular to the bases for the type of prism under consideration, the height of a regular quadrangular prism will be equal to the length of its side edge.

There are two terms in the general formula for the surface area of ​​a prism. The area of ​​​​the base in this case is easy to calculate, it is equal to:

To calculate the area of ​​the lateral surface, we argue as follows: this surface is formed by 4 identical rectangles. Moreover, the sides of each of them are equal to a and h. This means that the area S b will be equal to:

Note that the product 4*a is the perimeter of the square base. If we generalize this expression to the case of an arbitrary base, then for a rectangular prism the side surface can be calculated as follows:

Where P o is the perimeter of the base.

Returning to the problem of calculating the area of ​​a regular quadrangular prism, we can write the final formula:

S = 2*S o + S b = 2*a 2 + 4*a*h = 2*a*(a+2*h)

Area of ​​an oblique parallelepiped

Calculating it is somewhat more difficult than for a rectangular one. In this case, the area of ​​​​the base of a quadrangular prism is calculated using the same formula as for a parallelogram. The changes relate to the method of determining the area of ​​the lateral surface.

To do this, use the same formula through the perimeter, which is given in the paragraph above. Only now it will have slightly different multipliers. The general formula for S b in the case of an oblique prism is:

Here, c is the length of the side edge of the figure. The value P sr is the perimeter of the rectangular slice. This environment is constructed as follows: it is necessary to intersect all the side faces with a plane so that it is perpendicular to all of them. The resulting rectangle will be the desired slice.

The figure above shows an example of an oblique box. Its cross-hatched section forms right angles with the sides. The perimeter of the section is P sr . It is formed by four heights of lateral parallelograms. For this quadrangular prism, the lateral surface area is calculated using the above formula.

The length of the diagonal of a cuboid

The diagonal of a parallelepiped is a segment that connects two vertices that do not have common sides that form them. There are only four diagonals in any quadrangular prism. For a cuboid with a rectangle at its base, the lengths of all diagonals are equal to each other.

The figure below shows the corresponding figure. The red segment is its diagonal.

D = √(A 2 + B 2 + C 2)

Here D is the length of the diagonal. The remaining symbols are the lengths of the sides of the parallelepiped.

Many people confuse the diagonal of a parallelepiped with the diagonals of its sides. Below is a figure where the diagonals of the sides of the figure are shown with colored segments.

The length of each of them is also determined by the Pythagorean theorem and is equal to the square root of the sum of the squares of the corresponding side lengths.

Prism volume

In addition to the area of ​​\u200b\u200bthe regular quadrangular prism or other types of prisms, in order to solve some geometric problems, their volume should also be known. This value for absolutely any prism is calculated by the following formula:

If the prism is rectangular, then it is enough to calculate the area of ​​its base and multiply it by the length of the edge of the side to get the volume of the figure.

If the prism is a regular quadrilateral, then its volume will be equal to:

It is easy to see that this formula is converted into an expression for the volume of a cube if the length of the side edge h is equal to the side of the base a.

Problem with a cuboid

To consolidate the studied material, we will solve the following problem: there is a rectangular parallelepiped whose sides are 3 cm, 4 cm and 5 cm. It is necessary to calculate its surface area, diagonal length and volume.

S \u003d 2 * S o + S b \u003d 2 * 12 + 5 * 14 \u003d 24 + 70 \u003d 94 cm 2

To determine the length of the diagonal and the volume of the figure, you can directly use the above expressions:

D \u003d √ (3 2 +4 2 +5 2) \u003d 7.071 cm;

V \u003d 3 * 4 * 5 \u003d 60 cm 3.

Problem with an oblique parallelepiped

The figure below shows an oblique prism. Its sides are equal: a=10 cm, b=8 cm, c=12 cm. It is necessary to find the surface area of ​​this figure.

First, let's determine the area of ​​the base. It can be seen from the figure that the acute angle is 50 o. Then its area is:

S o \u003d h * a \u003d sin (50 o) * b * a

To determine the lateral surface area, find the perimeter of the shaded rectangle. The sides of this rectangle are a*sin(45o) and b*sin(60o). Then the perimeter of this rectangle is:

P sr = 2*(a*sin(45o)+b*sin(60o))

The total surface area of ​​this parallelepiped is:

S = 2*S o + S b = 2*(sin(50 o)*b*a + a*c*sin(45 o) + b*c*sin(60 o))

We substitute the data from the condition of the problem for the lengths of the sides of the figure, we get the answer:

From the solution of this problem, it can be seen that trigonometric functions are used to determine the areas of oblique figures.

With the help of this video tutorial, everyone will be able to independently get acquainted with the topic “The concept of a polyhedron. Prism. Prism surface area. During the lesson, the teacher will talk about what geometric shapes such as a polyhedron and prisms are, give the appropriate definitions and explain their essence with specific examples.

With the help of this lesson, everyone will be able to independently get acquainted with the topic “The concept of a polyhedron. Prism. Prism surface area.

Definition. A surface composed of polygons and bounding a certain geometric body will be called a polyhedral surface or a polyhedron.

Consider the following examples of polyhedra:

1. Tetrahedron ABCD is a surface made up of four triangles: ABC, adb, bdc And ADC(Fig. 1).

Rice. one

2. Parallelepiped ABCDA 1 B 1 C 1 D 1 is a surface composed of six parallelograms (Fig. 2).

Rice. 2

The main elements of a polyhedron are faces, edges, vertices.

The faces are the polygons that make up the polyhedron.

Edges are sides of faces.

The vertices are the ends of the edges.

Consider a tetrahedron ABCD(Fig. 1). Let us indicate its main elements.

Facets: triangles ABC, ADB, BDC, ADC.

Ribs: AB, AC, BC, DC, AD, BD.

Peaks: A, B, C, D.

Consider a box ABCDA 1 B 1 C 1 D 1(Fig. 2).

Facets: parallelograms AA 1 D 1 D, D 1 DCC 1, BB 1 C 1 C, AA 1 B 1 B, ABCD, A 1 B 1 C 1 D 1 .

Ribs: AA 1 , BB 1 , SS 1 , DD 1 , AD, A 1 D 1 , B 1 C 1 , BC, AB, A 1 B 1 , D 1 C 1 , DC.

Peaks: A, B, C, D, A 1 ,B 1 ,C 1 ,D 1 .

An important special case of a polyhedron is a prism.

ABSA 1 IN 1 WITH 1(Fig. 3).

Rice. 3

Equal Triangles ABC And A 1 B 1 C 1 are located in parallel planes α and β so that the edges AA 1 , BB 1 , SS 1 are parallel.

I.e ABSA 1 IN 1 WITH 1- triangular prism, if:

1) Triangles ABC And A 1 B 1 C 1 are equal.

2) Triangles ABC And A 1 B 1 C 1 located in parallel planes α and β: ABC║A 1 B 1 C (α ║ β).

3) Ribs AA 1 , BB 1 , SS 1 are parallel.

ABC And A 1 B 1 C 1- the base of the prism.

AA 1 , BB 1 , SS 1- side ribs of the prism.

If from an arbitrary point H 1 one plane (for example, β) drop the perpendicular HH 1 onto the plane α, then this perpendicular is called the height of the prism.

Definition. If the lateral edges are perpendicular to the bases, then the prism is called straight, otherwise it is called oblique.

Consider a triangular prism ABSA 1 IN 1 WITH 1(Fig. 4). This prism is straight. That is, its side edges are perpendicular to the bases.

For example, rib AA 1 perpendicular to the plane ABC. Edge AA 1 is the height of this prism.

Rice. 4

Note that the side face AA 1 V 1 V perpendicular to the bases ABC And A 1 B 1 C 1, since it passes through the perpendicular AA 1 to the foundations.

Now consider an inclined prism ABSA 1 IN 1 WITH 1(Fig. 5). Here the lateral edge is not perpendicular to the plane of the base. If we drop from the point A 1 perpendicular A 1 H on the ABC, then this perpendicular will be the height of the prism. Note that the segment AN is the projection of the segment AA 1 to the plane ABC.

Then the angle between the line AA 1 and plane ABC is the angle between the line AA 1 and her AN projection onto a plane, that is, the angle A 1 AH.

Rice. five

Consider a quadrangular prism ABCDA 1 B 1 C 1 D 1(Fig. 6). Let's see how it turns out.

1) Quadrilateral ABCD equal to a quadrilateral A 1 B 1 C 1 D 1: ABCD = A 1 B 1 C 1 D 1.

2) Quadrangles ABCD And A 1 B 1 C 1 D 1 ABC║A 1 B 1 C (α ║ β).

3) Quadrangles ABCD And A 1 B 1 C 1 D 1 arranged so that the lateral ribs are parallel, that is: AA 1 ║BB 1 ║SS 1 ║DD 1.

Definition. The diagonal of a prism is a segment that connects two vertices of a prism that do not belong to the same face.

For example, AC 1- diagonal of a quadrangular prism ABCDA 1 B 1 C 1 D 1.

Definition. If the side edge AA 1 perpendicular to the plane of the base, then such a prism is called a straight line.

Rice. 6

A special case of a quadrangular prism is the known parallelepiped. Parallelepiped ABCDA 1 B 1 C 1 D 1 shown in fig. 7.

Let's see how it works:

1) Equal figures lie in the bases. In this case - equal parallelograms ABCD And A 1 B 1 C 1 D 1: ABCD = A 1 B 1 C 1 D 1.

2) Parallelograms ABCD And A 1 B 1 C 1 D 1 lie in parallel planes α and β: ABC║A 1 B 1 C 1 (α ║ β).

3) Parallelograms ABCD And A 1 B 1 C 1 D 1 arranged in such a way that the side ribs are parallel to each other: AA 1 ║BB 1 ║SS 1 ║DD 1.

Rice. 7

From a point A 1 drop the perpendicular AN to the plane ABC. Section A 1 H is the height.

Consider how a hexagonal prism is arranged (Fig. 8).

1) Equal hexagons lie at the base ABCDEF And A 1 B 1 C 1 D 1 E 1 F 1: ABCDEF= A 1 B 1 C 1 D 1 E 1 F 1.

2) Planes of hexagons ABCDEF And A 1 B 1 C 1 D 1 E 1 F 1 parallel, that is, the bases lie in parallel planes: ABC║A 1 B 1 C (α ║ β).

3) Hexagons ABCDEF And A 1 B 1 C 1 D 1 E 1 F 1 arranged so that all side edges are parallel to each other: AA 1 ║BB 1 …║FF 1.

Rice. 8

Definition. If any side edge is perpendicular to the plane of the base, then such a hexagonal prism is called a straight line.

Definition. A right prism is called regular if its bases are regular polygons.

Consider a regular triangular prism ABSA 1 IN 1 WITH 1.

Rice. nine

triangular prism ABSA 1 IN 1 WITH 1- correct, this means that regular triangles lie at the bases, that is, all sides of these triangles are equal. Also, this prism is straight. This means that the side edge is perpendicular to the plane of the base. And this means that all side faces are equal rectangles.

So if a triangular prism ABSA 1 IN 1 WITH 1 is correct, then:

1) The side edge is perpendicular to the plane of the base, that is, it is the height: AA 1 ⊥ ABC.

2) The base is a regular triangle: ∆ ABC- right.

Definition. The total surface area of ​​a prism is the sum of the areas of all its faces. Denoted S full.

Definition. The area of ​​the lateral surface is the sum of the areas of all lateral faces. Denoted S side.

The prism has two bases. Then the total surface area of ​​the prism is:

S full \u003d S side + 2S main.

The area of ​​the lateral surface of a straight prism is equal to the product of the perimeter of the base and the height of the prism.

The proof will be carried out on the example of a triangular prism.

Given: ABSA 1 IN 1 WITH 1- direct prism, i.e. AA 1 ⊥ ABC.

AA 1 = h.

Prove: S side \u003d R main ∙ h.

Rice. 10

Proof.

triangular prism ABSA 1 IN 1 WITH 1- straight, so AA 1 B 1 B, AA 1 C 1 C, BB 1 C 1 C - rectangles.

Find the area of ​​the lateral surface as the sum of the areas of the rectangles AA 1 B 1 B, AA 1 C 1 C, BB 1 C 1 C:

S side \u003d AB ∙ h + BC ∙ h + CA ∙ h \u003d (AB + BC + CA) ∙ h \u003d P main ∙ h.

We get S side \u003d R main ∙ h, Q.E.D.

We got acquainted with polyhedrons, a prism, its varieties. We proved the theorem on the lateral surface of a prism. In the next lesson, we will solve problems on a prism.

1. Geometry. Grade 10-11: a textbook for students of educational institutions (basic and profile levels) / I. M. Smirnova, V. A. Smirnov. - 5th edition, corrected and supplemented - M .: Mnemosyne, 2008. - 288 p. : ill.
2. Geometry. Grade 10-11: A textbook for general educational institutions / Sharygin I. F. - M .: Bustard, 1999. - 208 p.: ill.
3. Geometry. Grade 10: Textbook for general educational institutions with in-depth and profile study of mathematics / E. V. Potoskuev, L. I. Zvalich. - 6th edition, stereotype. - M. : Bustard, 008. - 233 p. :ill.

1. Iclass().
2. Shkolo.ru ().
3. Old school ().
4. wikihow().

1. What is the minimum number of faces a prism can have? How many vertices, edges does such a prism have?
2. Is there a prism that has exactly 100 edges?
3. The side rib is inclined to the base plane at an angle of 60°. Find the height of the prism if the side edge is 6 cm.
4. In a right triangular prism, all edges are equal. Its lateral surface area is 27 cm 2 . Find the total surface area of ​​the prism.

Your privacy is important to us. For this reason, we have developed a Privacy Policy that describes how we use and store your information. Please read our privacy policy and let us know if you have any questions.

Collection and use of personal information

Personal information refers to data that can be used to identify or contact a specific person.

You may be asked to provide your personal information at any time when you contact us.

The following are some examples of the types of personal information we may collect and how we may use such information.

What personal information we collect:

• When you submit an application on the site, we may collect various information, including your name, phone number, email address, etc.

How we use your personal information:

• The personal information we collect allows us to contact you and inform you about unique offers, promotions and other events and upcoming events.
• From time to time, we may use your personal information to send you important notices and communications.
• We may also use personal information for internal purposes, such as conducting audits, data analysis and various research in order to improve the services we provide and provide you with recommendations regarding our services.
• If you enter a prize draw, contest or similar incentive, we may use the information you provide to administer such programs.

Disclosure to third parties

We do not disclose information received from you to third parties.

Exceptions:

• In the event that it is necessary - in accordance with the law, judicial order, in legal proceedings, and / or based on public requests or requests from state bodies in the territory of the Russian Federation - disclose your personal information. We may also disclose information about you if we determine that such disclosure is necessary or appropriate for security, law enforcement, or other public interest reasons.
• In the event of a reorganization, merger or sale, we may transfer the personal information we collect to the relevant third party successor.

Protection of personal information

We take precautions - including administrative, technical and physical - to protect your personal information from loss, theft, and misuse, as well as from unauthorized access, disclosure, alteration and destruction.

Maintaining your privacy at the company level

To ensure that your personal information is secure, we communicate privacy and security practices to our employees and strictly enforce privacy practices.

A prism is a geometric three-dimensional figure, the characteristics and properties of which are studied in high school. As a rule, when studying it, such quantities as volume and surface area are considered. In the same article, we will reveal a slightly different question: we will give a method for determining the length of the diagonals of a prism using the example of a quadrangular figure.

What shape is called a prism?

In geometry, the following definition of a prism is given: it is a three-dimensional figure bounded by two polygonal identical sides that are parallel to each other, and a certain number of parallelograms. The figure below shows an example of a prism that fits this definition.

We see that the two red pentagons are equal to each other and are in two parallel planes. Five pink parallelograms connect these pentagons into a single object - a prism. The two pentagons are called the bases of the figure, and its parallelograms are the side faces.

Prisms are straight and inclined, which are also called rectangular and oblique. The difference between them lies in the angles between the base and the side faces. For a rectangular prism, all these angles are 90 o .

By the number of sides or vertices of the polygon at the base, they speak of triangular, pentagonal, quadrangular prisms, and so on. Moreover, if this polygon is regular, and the prism itself is straight, then such a figure is called regular.

The prism shown in the previous figure is a pentagonal oblique. Below is a pentagonal straight prism, which is correct.

All calculations, including the method for determining the diagonals of a prism, are conveniently performed for regular figures.

What elements characterize a prism?

The elements of a figure are the parts that make it up. Specifically for a prism, three main types of elements can be distinguished:

• tops;
• edges or sides;
• ribs.

Faces are bases and side planes, which are parallelograms in the general case. In a prism, each side always belongs to one of two types: either it is a polygon or a parallelogram.

The edges of a prism are those segments that bound each side of the figure. Like faces, edges also come in two types: those belonging to the base and the side surface, or those belonging only to the side surface. The former are always twice as many as the latter, regardless of the type of prism.

The vertices are the intersection points of the three edges of the prism, two of which lie in the plane of the base, and the third belongs to the two side faces. All vertices of the prism are in the planes of the bases of the figure.

The numbers of the described elements are connected in a single equality, which has the following form:

P \u003d B + C - 2.

Here P is the number of edges, B - vertices, C - sides. This equality is called Euler's polyhedron theorem.

The figure shows a triangular regular prism. Everyone can count that it has 6 vertices, 5 sides and 9 edges. These figures are consistent with Euler's theorem.

Prism diagonals

After properties such as volume and surface area, in geometry problems, information about the length of one or another diagonal of the figure under consideration is often found, which is either given or needs to be found from other known parameters. Consider what are the diagonals of a prism.

All diagonals can be divided into two types:

1. Lying in the plane of the faces. They connect non-adjacent vertices of either the polygon at the base of the prism, or the side surface parallelogram. The value of the lengths of such diagonals is determined based on the knowledge of the lengths of the corresponding edges and the angles between them. To determine the diagonals of parallelograms, the properties of triangles are always used.
2. Prisms lying inside the volume. These diagonals connect non-similar vertices of two bases. These diagonals are completely inside the figure. Their lengths are somewhat more difficult to calculate than for the previous type. The calculation method involves taking into account the lengths of the edges and the base, and parallelograms. For straight and regular prisms, the calculation is relatively simple, since it is carried out using the Pythagorean theorem and the properties of trigonometric functions.

Diagonals of the sides of a quadrangular right prism

The figure above shows four identical straight prisms, and the parameters of their edges are given. Diagonal A, Diagonal B, and Diagonal C prisms show the diagonals of three different faces with a dashed red line. Since the prism is a straight line with a height of 5 cm, and its base is a rectangle with sides of 3 cm and 2 cm, it is not difficult to find the marked diagonals. To do this, you need to use the Pythagorean theorem.

The length of the diagonal of the base of the prism (Diagonal A) is:

D A \u003d √ (3 2 +2 2) \u003d √13 ≈ 3.606 cm.

For the side face of a prism, the diagonal is (see Diagonal B):

D B \u003d √ (3 2 +5 2) \u003d √34 ≈ 5.831 cm.

Finally, the length of another side diagonal is (see Diagonal C):

D C \u003d √ (2 2 +5 2) \u003d √29 ≈ 5.385 cm.

Length of the inner diagonal

Now let's calculate the length of the diagonal of the quadrangular prism, which is shown in the previous figure (Diagonal D). This is not so difficult to do if you notice that it is the hypotenuse of a triangle in which the legs will be the height of the prism (5 cm) and the diagonal D A shown in the figure at the top left (Diagonal A). Then we get:

D D \u003d √ (D A 2 +5 2) \u003d √ (2 2 +3 2 +5 2) \u003d √38 ≈ 6.164 cm.

Right quadrangular prism

The diagonal of a regular prism whose base is a square is calculated in the same way as in the example above. The corresponding formula looks like:

D = √(2*a 2 +c 2).

Where a and c are the lengths of the side of the base and the side edge, respectively.

Note that in the calculations we used only the Pythagorean theorem. To determine the lengths of the diagonals of regular prisms with a large number of vertices (pentagonal, hexagonal, and so on), it is already necessary to apply trigonometric functions.