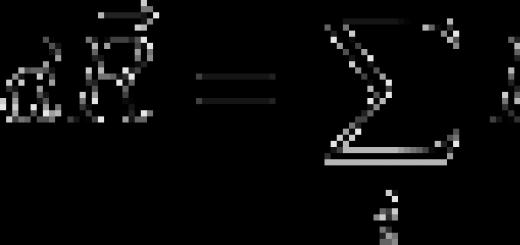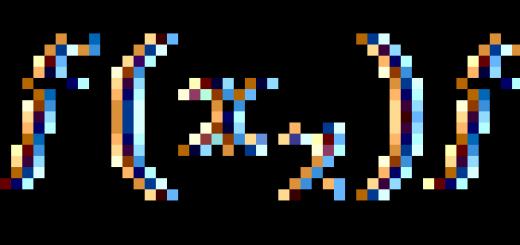Among the whole variety of logarithmic inequalities, inequalities with a variable base are studied separately. They are solved according to a special formula, which for some reason is rarely taught at school:

log k (x ) f (x ) ∨ log k (x ) g (x ) ⇒ (f (x ) − g (x )) (k (x ) − 1) ∨ 0

Instead of a jackdaw "∨", you can put any inequality sign: more or less. The main thing is that in both inequalities the signs are the same.

So we get rid of logarithms and reduce the problem to a rational inequality. The latter is much easier to solve, but when discarding logarithms, extra roots may appear. To cut them off, it is enough to find the area allowed values. If you forgot the ODZ of the logarithm, I strongly recommend repeating it - see "What is a logarithm".

Everything related to the range of acceptable values ​​must be written out and solved separately:

f(x) > 0; g(x) > 0; k(x) > 0; k(x) ≠ 1.

These four inequalities constitute a system and must be fulfilled simultaneously. When the range of acceptable values ​​is found, it remains to cross it with the solution rational inequality- and the answer is ready.

Task. Solve the inequality:

First, let's write the ODZ of the logarithm:

The first two inequalities are performed automatically, and the last one will have to be written. Since the square of a number is zero if and only if the number itself is zero, we have:

x 2 + 1 ≠ 1;
x2 ≠ 0;
x ≠ 0.

It turns out that the ODZ of the logarithm is all numbers except zero: x ∈ (−∞ 0)∪(0; +∞). Now we solve the main inequality:

We perform the transition from the logarithmic inequality to the rational one. In the original inequality there is a “less than” sign, so the resulting inequality should also be with a “less than” sign. We have:

(10 − (x 2 + 1)) (x 2 + 1 − 1)< 0;
(9 − x2) x2< 0;
(3 − x) (3 + x) x 2< 0.

Zeros of this expression: x = 3; x = -3; x = 0. Moreover, x = 0 is the root of the second multiplicity, which means that when passing through it, the sign of the function does not change. We have:

We get x ∈ (−∞ −3)∪(3; +∞). This set is completely contained in the ODZ of the logarithm, which means that this is the answer.

Transformation of logarithmic inequalities

Often the original inequality differs from the one above. This is easy to fix according to the standard rules for working with logarithms - see "Basic properties of logarithms". Namely:

1. Any number can be represented as a logarithm with a given base;
2. The sum and difference of logarithms with the same base can be replaced by a single logarithm.

Separately, I want to remind you about the range of acceptable values. Since there may be several logarithms in the original inequality, it is required to find the DPV of each of them. Thus, the general scheme for solving logarithmic inequalities is as follows:

1. Find the ODZ of each logarithm included in the inequality;
2. Reduce the inequality to the standard one using the formulas for adding and subtracting logarithms;
3. Solve the resulting inequality according to the scheme above.

Task. Solve the inequality:

Find the domain of definition (ODZ) of the first logarithm:

We solve by the interval method. Finding the zeros of the numerator:

3x − 2 = 0;
x = 2/3.

Then - the zeros of the denominator:

x − 1 = 0;
x = 1.

We mark zeros and signs on the coordinate arrow:

We get x ∈ (−∞ 2/3)∪(1; +∞). The second logarithm of the ODZ will be the same. If you don't believe me, you can check. Now we transform the second logarithm so that the base is two:

As you can see, the triples at the base and before the logarithm have shrunk. Get two logarithms with the same base. Let's put them together:

log 2 (x − 1) 2< 2;
log 2 (x − 1) 2< log 2 2 2 .

Got standard logarithmic inequality. We get rid of the logarithms by the formula. Since there is a “less than” sign in the original inequality, the resulting rational expression must also be less than zero. We have:

(f (x) - g (x)) (k (x) - 1)< 0;
((x − 1) 2 − 2 2)(2 − 1)< 0;
x 2 − 2x + 1 − 4< 0;
x 2 - 2x - 3< 0;
(x − 3)(x + 1)< 0;
x ∈ (−1; 3).

We got two sets:

1. ODZ: x ∈ (−∞ 2/3)∪(1; +∞);
2. Answer candidate: x ∈ (−1; 3).

It remains to cross these sets - we get the real answer:

We are interested in the intersection of sets, so we choose the intervals shaded on both arrows. We get x ∈ (−1; 2/3)∪(1; 3) - all points are punctured.

In the article we will consider solution of inequalities. Let's talk plainly about how to build a solution to inequalities with clear examples!

Before considering the solution of inequalities with examples, let's deal with the basic concepts.

Introduction to inequalities

inequality is called an expression in which functions are connected by relation signs >, . Inequalities can be both numerical and alphabetic.
Inequalities with two relation signs are called double, with three - triple, etc. For example:
a(x) > b(x),
a(x) a(x) b(x),
a(x) b(x).
a(x) Inequalities containing the sign > or or are not strict.
Inequality solution is any value of the variable for which this inequality is true.
"Solve the inequality" means that you need to find the set of all its solutions. There are various methods for solving inequalities. For inequality solutions use a number line that is infinite. For example, solving the inequality x > 3 is an interval from 3 to +, and the number 3 is not included in this interval, so the point on the line is denoted by an empty circle, because the inequality is strict.
+
The answer will be: x (3; +).
The value x=3 is not included in the set of solutions, so the parenthesis is round. The infinity sign is always enclosed in a parenthesis. The sign means "belonging".
Consider how to solve inequalities using another example with the sign:
x2
-+
The value x=2 is included in the set of solutions, so the square bracket and the point on the line is denoted by a filled circle.
The answer will be: x . The following example uses such a bracket.

Let's write down the answer: x ≥ -0,5 through intervals:

x ∈ [-0.5; +∞)

Reads: x belongs to the interval from minus 0.5, including, up to plus infinity.

Infinity can never turn on. It's not a number, it's a symbol. Therefore, in such entries, infinity always coexists with a parenthesis.

This form of recording is convenient for complex answers consisting of several gaps. But - just for the final answers. In intermediate results, where a further solution is expected, it is better to use the usual form, in the form simple inequality. We will deal with this in the relevant topics.

Popular tasks with inequalities.

The linear inequalities themselves are simple. Therefore, the tasks often become more difficult. So, to think it was necessary. This, if out of habit, is not very pleasant.) But it is useful. I will show examples of such tasks. Not for you to learn them, it's superfluous. And in order not to be afraid when meeting with similar examples. A little thought - and everything is simple!)

1. Find any two solutions to the 3x - 3 inequality< 0

If it is not very clear what to do, remember the main rule of mathematics:

If you don't know what to do, do what you can!

X < 1

So what? Nothing special. What are we being asked? We are asked to find two specific numbers that are the solution to an inequality. Those. fit the answer. Two any numbers. Actually, this is embarrassing.) A couple of 0 and 0.5 are suitable. A couple -3 and -8. yes these couples infinite set! What is the correct answer?!

I answer: everything! Any pair of numbers, each of which is less than one, would be the correct answer. Write what you want. Let's go further.

2. Solve the inequality:

4x - 3 ≠ 0

Jobs like this are rare. But, as auxiliary inequalities, when finding the ODZ, for example, or when finding the domain of a function, they are encountered all the time. Such a linear inequality can be solved as an ordinary linear equation. Only everywhere, except for the "=" sign ( equals) put the sign " ≠ " (not equal). So you’ll come to the answer, with an inequality sign:

X ≠ 0,75

In more difficult examples better to do it the other way. Make inequality equal. Like this:

4x - 3 = 0

Calmly solve it as taught, and get the answer:

x = 0.75

The main thing, at the very end, when writing down the final answer, is not to forget that we have found x, which gives equality. And we need - inequality. Therefore, we just don’t need this X.) And we need to write it down with the correct icon:

X ≠ 0,75

This approach results in fewer errors. Those who solve equations on the machine. And for those who do not solve equations, inequalities, in fact, are useless ...) Another example of a popular task:

3. Find the smallest integer solution of the inequality:

3(x - 1) < 5x + 9

First, we simply solve the inequality. We open the brackets, transfer, give similar ones ... We get:

X > - 6

Didn't it happen!? Did you follow the signs? And behind the signs of members, and behind the sign of inequality ...

Let's imagine again. We need to find a specific number that matches both the answer and the condition "smallest integer". If it doesn’t immediately dawn on you, you can simply take any number and figure it out. Two is greater than minus six? Certainly! Is there a suitable smaller number? Of course. For example, zero is greater than -6. And even less? We need the smallest possible! Minus three is more than minus six! You can already catch the pattern and stop stupidly sorting out the numbers, right?)

We take a number closer to -6. For example, -5. Response executed, -5 > - 6. Can you find another number less than -5 but greater than -6? You can, for example, -5.5 ... Stop! We've been told whole decision! Does not roll -5.5! What about minus six? Eee! The inequality is strict, minus 6 is no less than minus 6!

So the correct answer is -5.

Hopefully with a choice of value from common solution all clear. Another example:

4. Solve the inequality:

7 < 3x+1 < 13

How! Such an expression is called triple inequality. Strictly speaking, this is an abbreviated notation of the system of inequalities. But you still have to solve such triple inequalities in some tasks ... It is solved without any systems. By the same identical transformations.

It is necessary to simplify, bring this inequality to a pure X. But... What to transfer where!? Here is the time to remember that shifting left-right is shortened form the first identical transformation.

And the full form looks like this: You can add / subtract any number or expression to both parts of the equation (inequality).

There are three parts here. Here we will apply identical transformations to all three parts!

So, let's get rid of the one in the middle part of the inequality. Subtract one from the entire middle part. So that the inequality does not change, we subtract one from the remaining two parts. Like this:

7 -1< 3x+1-1 < 13-1

6 < 3x < 12

Already better, right?) It remains to divide all three parts into three:

2 < X < 4

That's all. This is the answer. X can be any number from two (not including) to four (not including). This answer is also written at intervals, such entries will be in square inequalities. There they are the most common thing.

At the end of the lesson, I will repeat the most important thing. Success in decision linear inequalities depends on the ability to transform and simplify linear equations. If at the same time follow the inequality sign, there will be no problems. What I wish you. no problem.)

If you like this site...

By the way, I have a couple more interesting sites for you.)

You can practice solving examples and find out your level. Testing with instant verification. Learning - with interest!)

you can get acquainted with functions and derivatives.

For example, the expression \(x>5\) is an inequality.

Types of inequalities:

If \(a\) and \(b\) are numbers or , then the inequality is called numerical. In fact, this is just a comparison of two numbers. These inequalities are subdivided into faithful and unfaithful.

For example:
\(-5<2\) - верное числовое неравенство, ведь \(-5\) действительно меньше \(2\);

\(17+3\geq 115\) is an invalid numerical inequality because \(17+3=20\) and \(20\) is less than \(115\) (not greater than or equal to).

If \(a\) and \(b\) are expressions containing a variable, then we have inequality with variable. Such inequalities are divided into types depending on the content:

\(2x+1\geq4(5-x)\)				Variable only to the first power
\(3x^2-x+5>0\)				There is a variable in the second power (square), but no higher powers (third, fourth, etc.)
\(\log_(4)((x+1))<3\)
\(2^(x)\leq8^(5x-2)\)

... etc.

What is a solution to an inequality?

If any number is substituted into the inequality instead of a variable, then it will turn into a numeric one.

If the given value for x makes the original inequality true numerical, then it is called solving the inequality. If not, then this value is not a solution. And to solve inequality- you need to find all its solutions (or show that they do not exist).

For example, if we are in the linear inequality \(x+6>10\), we substitute the number \(7\) instead of x, we get the correct numerical inequality: \(13>10\). And if we substitute \(2\), there will be an incorrect numerical inequality \(8>10\). That is, \(7\) is a solution to the original inequality, but \(2\) is not.

However, the inequality \(x+6>10\) has other solutions. Indeed, we will get the correct numerical inequalities by substituting and \(5\), and \(12\), and \(138\) ... And how can we find all possible solutions? To do this, use For our case, we have:

\(x+6>10\) \(|-6\)
\(x>4\)

That is, we can use any number greater than four. Now we need to write down the answer. Solutions to inequalities, as a rule, are written numerically, additionally marking them on the numerical axis with hatching. For our case we have:

Answer: \(x\in(4;+\infty)\)

When does the sign change in an inequality?

There is one big trap in inequalities, which students really “like” to fall into:

When multiplying (or dividing) inequality by a negative number, it is reversed (“greater than” by “less”, “greater than or equal to” by “less than or equal to”, and so on)

Why is this happening? To understand this, let's look at the transformations numerical inequality\(3>1\). It is true, the trio is really more than one. First, let's try to multiply it by any positive number, for example, two:

\(3>1\) \(|\cdot2\)
\(6>2\)

As you can see, after multiplication, the inequality remains true. And no matter what positive number we multiply, we will always get the correct inequality. Now let's try to multiply by a negative number, for example, minus three:

\(3>1\) \(|\cdot(-3)\)
\(-9>-3\)

It turned out to be an incorrect inequality, because minus nine is less than minus three! That is, in order for the inequality to become true (which means that the transformation of multiplication by a negative was “legal”), you need to flip the comparison sign, like this: \(−9<− 3\).
With division, it will turn out similarly, you can check it yourself.

The rule written above applies to all types of inequalities, and not just to numerical ones.

Example: Solve the inequality \(2(x+1)-1<7+8x\)
Decision:

\(2x+2-1<7+8x\)	Let's move \(8x\) to the left, and \(2\) and \(-1\) to the right, not forgetting to change signs
\(2x-8x<7-2+1\)
\(-6x<6\) \(|:(-6)\)	Divide both sides of the inequality by \(-6\), not forgetting to change from "less" to "greater"
	Let's mark a numerical interval on the axis. Inequality, so the value \(-1\) is “punched out” and we don’t take it in response
	Let's write the answer as an interval

Answer: \(x\in(-1;\infty)\)

Inequalities and DHS

Inequalities, as well as equations, can have restrictions on , that is, on the values ​​of x. Accordingly, those values ​​that are unacceptable according to the ODZ should be excluded from the solution interval.

Example: Solve the inequality \(\sqrt(x+1)<3\)

Decision: It is clear that in order for the left side to be less than \(3\), the root expression must be less than \(9\) (after all, from \(9\) just \(3\)). We get:

\(x+1<9\) \(|-1\)
\(x<8\)

All? Any value of x less than \(8\) will suit us? Not! Because if we take, for example, the value \(-5\) that seems to fit the requirement, it will not be a solution to the original inequality, since it will lead us to calculating the root of a negative number.

\(\sqrt(-5+1)<3\)
\(\sqrt(-4)<3\)

Therefore, we must also take into account the restrictions on the values ​​​​of x - it cannot be such that there is a negative number under the root. Thus, we have the second requirement for x:

\(x+1\geq0\)
\(x\geq-1\)

And for x to be a final solution, it must satisfy both requirements at once: it must be less than \(8\) (to be a solution) and greater than \(-1\) (to be valid in principle). Plotting on the number line, we have the final answer:

Answer: \(\left[-1;8\right)\)