One of the main characteristics in algebra, and indeed in all mathematics, is a degree. Of course, in the 21st century, all calculations can be carried out on an online calculator, but it is better to learn how to do it yourself for the development of brains.

In this article, we will consider the most important issues regarding this definition. Namely, we will understand what it is in general and what are its main functions, what properties exist in mathematics.

Let's look at examples of what the calculation looks like, what are the basic formulas. We will analyze the main types of quantities and how they differ from other functions.

We will understand how to solve various problems using this value. We will show with examples how to raise to a zero degree, irrational, negative, etc.

Online exponentiation calculator

What is the degree of a number

What is meant by the expression "raise a number to a power"?

The degree n of a number a is the product of factors of magnitude a n times in a row.

Mathematically it looks like this:

a n = a * a * a * …a n .

For example:

• 2 3 = 2 in the third step. = 2 * 2 * 2 = 8;
• 4 2 = 4 in step. two = 4 * 4 = 16;
• 5 4 = 5 in step. four = 5 * 5 * 5 * 5 = 625;
• 10 5 \u003d 10 in 5 step. = 10 * 10 * 10 * 10 * 10 = 100000;
• 10 4 \u003d 10 in 4 step. = 10 * 10 * 10 * 10 = 10000.

Below is a table of squares and cubes from 1 to 10.

Table of degrees from 1 to 10

Below are the results of the construction natural numbers to positive powers - "from 1 to 100".

Ch-lo	2nd grade	3rd grade
1	1	1
2	4	8
3	9	27
4	16	64
5	25	125
6	36	216
7	49	343
8	64	512
9	81	279
10	100	1000

Degree properties

What is characteristic of such a mathematical function? Let's look at the basic properties.

Scientists have established the following signs characteristic of all degrees:

• a n * a m = (a) (n+m) ;
• a n: a m = (a) (n-m) ;
• (a b) m =(a) (b*m) .

Let's check with examples:

2 3 * 2 2 = 8 * 4 = 32. On the other hand 2 5 = 2 * 2 * 2 * 2 * 2 = 32.

Similarly: 2 3: 2 2 = 8 / 4 = 2. Otherwise 2 3-2 = 2 1 =2.

(2 3) 2 = 8 2 = 64. What if it's different? 2 6 = 2 * 2 * 2 * 2 * 2 * 2 = 32 * 2 = 64.

As you can see, the rules work.

But how to be with addition and subtraction? Everything is simple. First exponentiation is performed, and only then addition and subtraction.

Let's look at examples:

• 3 3 + 2 4 = 27 + 16 = 43;
• 5 2 - 3 2 = 25 - 9 = 16

But in this case, you must first calculate the addition, since there are actions in brackets: (5 + 3) 3 = 8 3 = 512.

How to produce calculations in more complex cases? The order is the same:

• if there are brackets, you need to start with them;
• then exponentiation;
• then perform operations of multiplication, division;
• after addition, subtraction.

There are specific properties that are not characteristic of all degrees:

1. The root of the nth degree from the number a to the degree m will be written as: a m / n .
2. When raising a fraction to a power: both the numerator and its denominator are subject to this procedure.
3. When raising the product of different numbers to a power, the expression will correspond to the product of these numbers to a given power. That is: (a * b) n = a n * b n .
4. When raising a number to a negative power, you need to divide 1 by a number in the same step, but with a “+” sign.
5. If the denominator of a fraction is in a negative power, then this expression will be equal to the product of the numerator and the denominator in a positive power.
6. Any number to the power of 0 = 1, and to the step. 1 = to himself.

These rules are important in individual cases, we will consider them in more detail below.

Degree with a negative exponent

What to do with a negative degree, that is, when the indicator is negative?

Based on properties 4 and 5(see point above) it turns out:

A (- n) \u003d 1 / A n, 5 (-2) \u003d 1/5 2 \u003d 1/25.

And vice versa:

1 / A (- n) \u003d A n, 1 / 2 (-3) \u003d 2 3 \u003d 8.

What if it's a fraction?

(A / B) (- n) = (B / A) n , (3 / 5) (-2) = (5 / 3) 2 = 25 / 9.

Degree with a natural indicator

It is understood as a degree with exponents equal to integers.

Things to remember:

A 0 = 1, 1 0 = 1; 2 0 = 1; 3.15 0 = 1; (-4) 0 = 1…etc.

A 1 = A, 1 1 = 1; 2 1 = 2; 3 1 = 3…etc.

Also, if (-a) 2 n +2 , n=0, 1, 2…then the result will be with a “+” sign. If a negative number is raised to an odd power, then vice versa.

General properties, and all the specific features described above, are also characteristic of them.

Fractional degree

This view can be written as a scheme: A m / n. It is read as: the root of the nth degree of the number A to the power of m.

With a fractional indicator, you can do anything: reduce, decompose into parts, raise to another degree, etc.

Degree with irrational exponent

Let α be an irrational number and А ˃ 0.

To understand the essence of the degree with such an indicator, Let's look at different possible cases:

• A \u003d 1. The result will be equal to 1. Since there is an axiom - 1 is equal to one in all powers;

А r 1 ˂ А α ˂ А r 2 , r 1 ˂ r 2 are rational numbers;

• 0˂А˂1.

In this case, vice versa: А r 2 ˂ А α ˂ А r 1 under the same conditions as in the second paragraph.

For example, the exponent is the number π. It is rational.

r 1 - in this case it is equal to 3;

r 2 - will be equal to 4.

Then, for A = 1, 1 π = 1.

A = 2, then 2 3 ˂ 2 π ˂ 2 4 , 8 ˂ 2 π ˂ 16.

A = 1/2, then (½) 4 ˂ (½) π ˂ (½) 3 , 1/16 ˂ (½) π ˂ 1/8.

Such degrees are characterized by all the mathematical operations and specific properties described above.

Conclusion

Let's summarize - what are these values ​​for, what are the advantages of such functions? Of course, first of all, they simplify the lives of mathematicians and programmers when solving examples, since they allow minimizing calculations, reducing algorithms, systematizing data, and much more.

Where else can this knowledge be useful? In any working specialty: medicine, pharmacology, dentistry, construction, technology, engineering, design, etc.

Articles on natural sciences and mathematics

Properties of powers with the same base

There are three degree properties with the same bases and natural indicators. This

• Work sum
• Private two powers with the same base is equal to an expression where the base is the same and the exponent is difference indicators of the original multipliers.
• Raising a power of a number to a power is equal to an expression in which the base is the same number and the exponent is work two degrees.

Be careful! Rules regarding addition and subtraction powers with the same base does not exist.

We write these properties-rules in the form of formulas:

• a m? a n = a m+n
• a m? a n = a m–n
• (am) n = a mn

Now consider them on specific examples and try to prove.

5 2 ? 5 3 = 5 5 - here we applied the rule; and now imagine how we would solve this example if we did not know the rules:

5 2 ? 5 3 = 5? five ? five ? five ? 5 \u003d 5 5 - five squared is five times five, and cubed is the product of three fives. The result is a product of five fives, but this is something other than five to the fifth power: 5 5 .

3 9 ? 3 5 = 3 9–5 = 3 4 . Let's write the division as a fraction:

It can be shortened:

As a result, we get:

Thus, we proved that when dividing two powers with the same bases, their indicators must be subtracted.

However, when dividing, it is impossible for the divisor to be equal to zero (since you cannot divide by zero). In addition, since we consider degrees only with natural indicators, we cannot get a number less than 1 as a result of subtracting the indicators. Therefore, the formula a m ? a n = a m–n restrictions are imposed: a ? 0 and m > n.

Let's move on to the third property:
(2 2) 4 = 2 2?4 = 2 8

Let's write in expanded form:
(2 2) 4 = (2 ? 2) 4 = (2 ? 2) ? (2 ? 2) ? (2 ? 2) ? (2 ? 2) = 2 ? 2 ? 2 ? 2 ? 2 ? 2 ? 2 ? 2 = 2 8

You can come to this conclusion and logically reasoning. You need to multiply two squared four times. But there are two deuces in each square, so there will be eight deuces in total.

scienceland.info

Rules for addition and subtraction.

1. From a change in the places of the terms, the sum will not change (commutative property of addition)

13+25=38 can be written as: 25+13=38

2. The result of addition will not change if adjacent terms are replaced by their sum (an associative property of addition).

10+13+3+5=31 can be written as: 23+3+5=31; 26+5=31; 23+8=31 etc.

3. Units add up with ones, tens with tens, and so on.

34+11=45 (3 tens plus 1 tens; 4 ones plus 1 one).

4. Units are subtracted from units, tens from tens, etc.

53-12=41 (3 units minus 2 units; 5 tens minus 1 ten)

note: 10 units make one ten. This must be remembered when subtracting, because if the number of units of the subtracted is greater than that of the reduced, then we can “borrow” one ten from the reduced.

41-12 \u003d 29 (In order to subtract 2 from 1, we first need to “borrow” a unit from tens, we get 11-2 \u003d 9; remember that the reduced one has 1 less less, therefore, there are 3 tens and from it 1 ten is subtracted Answer 29).

5. If one of them is subtracted from the sum of two terms, then the second term will be obtained.

This means that addition can be checked using subtraction.

To check, one of the terms is subtracted from the sum: 49-7=42 or 49-42=7

If, as a result of subtraction, you did not get one of the terms, then an error was made in your addition.

6. If you add the subtrahend to the difference, you get the minuend.

This means that subtraction can be checked by addition.

To check, add the subtrahend to the difference: 19+50=69.

If, as a result of the procedure described above, you did not get a decrease, then an error was made in your subtraction.

Addition and subtraction of rational numbers

This lesson covers addition and subtraction of rational numbers. The topic is classified as complex. Here it is necessary to use the entire arsenal of previously acquired knowledge.

The rules for adding and subtracting integers are also valid for rational numbers. Recall that rational numbers are numbers that can be represented as a fraction, where a - is the numerator of a fraction b is the denominator of the fraction. And b should not be null.

In this lesson, we will increasingly refer to fractions and mixed numbers as one common phrase - rational numbers.

Lesson navigation:

Example 1 Find the value of an expression

We enclose each rational number in brackets along with its signs. We take into account that the plus which is given in the expression is the sign of the operation and does not apply to fractions. This fraction has its own plus sign, which is invisible due to the fact that it is not written down. But we will write it down for clarity:

This is the addition of rational numbers with different signs. To add rational numbers with different signs, you need to subtract the smaller one from the larger module, and put the sign whose module is larger in front of the answer. And in order to understand which module is greater and which is less, you need to be able to compare the modules of these fractions before calculating them:

The modulus of a rational number is greater than the modulus of a rational number. Therefore, we subtracted from . Got an answer. Then, reducing this fraction by 2, we got the final answer.

If desired, some primitive actions, such as enclosing numbers in brackets and putting down modules, can be skipped. This example can be written in a shorter way:

Example 2 Find the value of an expression

We enclose each rational number in brackets along with its signs. We take into account that the minus which is given in the expression is the sign of the operation and does not apply to fractions.

The fraction in this case is a positive rational number that has a plus sign, which is invisible. But we will write it down for clarity:

Let's replace subtraction with addition. Recall that for this you need to add the number opposite to the subtracted to the minuend:

We got the addition of negative rational numbers. To add negative rational numbers, you need to add their modules and put a minus before the answer:

Example 3 Find the value of an expression

In this expression, the fractions have different denominators. To make it easier for ourselves, let's bring these fractions to the same (common) denominator. We will not dwell on this in detail. If you're having trouble, be sure to go back to the fractions lesson and repeat it.

After reducing the fractions to a common denominator, the expression will take the following form:

This is the addition of rational numbers with different signs. We subtract the smaller one from the larger module and put the sign in front of the received answer, the module of which is greater:

Example 4 Find the value of an expression

We got the sum of three terms. First, find the value of the expression, then add to the received answer

First action:

Second action:

Thus, the value of the expression is equal.

The solution for this example can be written shorter

Example 5. Find the value of an expression

Enclose each number in brackets along with its signs. For this mixed number temporarily deploy

Let's calculate the integer parts:

In the main expression instead of write the resulting unit:

Let's convert the resulting expression. To do this, we omit the brackets and write the unit and the fraction together

The solution for this example can be written shorter:

Example 6 Find the value of an expression

Convert the mixed number to an improper fraction. Let's rewrite the rest as is:

We enclose each rational number in brackets along with its signs:

Let's replace subtraction with addition:

We got the addition of negative rational numbers. Let's add the modules of these numbers and put a minus before the received answer:

Thus, the value of the expression is .

The solution for this example can be written shorter:

Example 7 Find value expression

Let's write the mixed number in expanded form. Let's rewrite the rest as is:

Enclose every rational number in brackets together with its signs

Let's replace subtraction with addition where possible:

Let's calculate the integer parts:

In the main expression, instead of writing the resulting number? 7

The expression is an expanded form of writing a mixed number. You can immediately write down the answer by writing together the numbers? 7 and a fraction (hiding the minus of this fraction)

Thus, the value of the expression is

The solution for this example can be written much shorter. If you skip some details, then it can be written as follows:

Example 8 Find the value of an expression

This expression can be calculated in two ways. Let's consider each of them.

First way. The integer and fractional parts of the expression are calculated separately.

First, let's write the mixed numbers in expanded form:

Enclose each number in brackets along with its signs:

Let's replace subtraction with addition where possible:

We got the sum of several terms. According to the associative law of addition, if an expression contains several terms, then the sum will not depend on the order of operations. This will allow us to group the integer and fractional parts separately:

Let's calculate the integer parts:

In the main expression, instead of writing the resulting number? 3

Let's calculate the fractional parts:

In the main expression, instead of writing the resulting mixed number

To calculate the resulting expression, the mixed number needs to be temporarily expanded, then parenthesize each number, and replace subtraction with addition. This must be done very carefully so as not to confuse the signs of the terms.

After transforming the expression, we have a new expression that is easy to calculate. A similar expression was in Example 7. Recall that we added the integer parts separately, and left the fractional part as it is:

So the value of the expression is

The solution for this example can be written shorter

In a short solution, the steps of putting numbers in brackets, replacing subtraction with addition, putting down modules are skipped. If you are studying at a school or other educational institution, then you will be required to skip these primitive steps to save time and space. The above short solution can be written even shorter. It will look like this:

Therefore, while at school or in another educational institution, be prepared for the fact that some actions will have to be performed in the mind.

The second way. Mixed numbers expressions translate into improper fractions and calculated like ordinary fractions.

Enclose in brackets every rational number along with its signs

Let's replace subtraction with addition:

Now the mixed numbers and translate into improper fractions:

We got the addition of negative rational numbers. Let's add their modules and put a minus before the received answer:

Got the same answer as last time.

The detailed solution for the second way is as follows:

Example 9 Find expression expressions

First way. Add the integer and fractional parts separately.

This time, let's try to skip some primitive actions, such as writing an expression in expanded form, putting numbers in brackets, replacing subtraction with addition, putting down modules:

Note that the fractional parts have been reduced to a common denominator.

The second way. Convert mixed numbers to improper fractions and calculate like ordinary fractions.

Example 10 Find the value of an expression

Let's replace subtraction with addition:

The resulting expression does not contain negative numbers, which are the main cause of errors. And since there are no negative numbers, we can remove the plus in front of the subtrahend, and also remove the parentheses. Then we get the simplest expression, which is easy to calculate:

In this example, the integer and fractional parts were calculated separately.

Example 11. Find the value of an expression

This is the addition of rational numbers with different signs. We subtract the smaller one from the larger module and put the sign in front of the resulting number, the module of which is greater:

Example 12. Find the value of an expression

The expression consists of several parameters. According to the order of operations, first of all, you need to perform the actions in brackets.

First, we calculate the expression , then add the expression. The received answers are added.

First action:

Second action:

Third action:

Answer: expression value equals

Example 13 Find the value of an expression

Let's replace subtraction with addition:

Obtained by adding rational numbers with different signs. Subtract the smaller module from the larger one and put the sign in front of the answer, the module of which is greater. But we are dealing with mixed numbers. To understand which module is larger and which is smaller, you need to compare the modules of these mixed numbers. And to compare the modules of mixed numbers, you need to convert them to improper fractions and compare them like ordinary fractions.

The following figure shows all the steps for comparing modules of mixed numbers

Knowing which modulus is larger and which is smaller, we can continue the calculation of our example:

Thus, the value of the expression equals

Consider the addition and subtraction of decimal fractions, which are also rational numbers and which can be both positive and negative.

Example 14 Find the value of the expression?3.2 + 4.3

We enclose each rational number in brackets along with its signs. We take into account that the plus which is given in the expression is the sign of the operation and does not apply to the decimal fraction 4.3. This decimal has its own plus sign, which is invisible due to the fact that it is not written down. But we will write it down for clarity:

This is the addition of rational numbers with different signs. To add rational numbers with different signs, you need to subtract the smaller one from the larger module, and put the sign whose module is larger in front of the answer. And in order to understand which modulus is larger and which is smaller, you need to be able to compare the moduli of these decimal fractions before calculating them:

The modulus of 4.3 is greater than the modulus of 3.2, so we subtracted 3.2 from 4.3. Got the answer 1.1. The answer is yes, because the answer must contain the sign of the larger module, that is, the module |+4,3|.

So the value of the expression?3.2 + (+4.3) is 1.1

Example 15 Find the value of the expression 3.5 + (?8.3)

This is the addition of rational numbers with different signs. As in the previous example, we subtract the smaller one from the larger module and put the sign in front of the answer, the module of which is greater

3,5 + (?8,3) = ?(|?8,3| ? |3,5|) = ?(8,3 ? 3,5) = ?(4,8) = ?4,8

Thus, the value of the expression 3.5 + (?8.3) is equal to?4.8

This example can be written shorter:

Example 16 Find the value of the expression?7.2 + (?3.11)

This is the addition of negative rational numbers. To add negative rational numbers, you need to add their modules and put a minus before the answer. You can skip the entry with modules to avoid cluttering up the expression:

7,2 + (?3,11) = ?7,20 + (?3,11) = ?(7,20 + 3,11) = ?(10,31) = ?10,31

Thus, the value of the expression?7.2 + (?3.11) is?10.31

This example can be written shorter:

Example 17. Find the value of the expression?0.48 + (?2.7)

This is the addition of negative rational numbers. We add their modules and put a minus sign in front of the received answer. You can skip the entry with modules to avoid cluttering up the expression:

0,48 + (?2,7) = (?0,48) + (?2,70) = ?(0,48 + 2,70) = ?(3,18) = ?3,18

Example 18. Find the value of the expression?4,9 ? 5.9

We enclose each rational number in brackets along with its signs. We take into account that the minus which is given in the expression is the sign of the operation and does not apply to the decimal fraction 5.9. This decimal has its own plus sign, which is invisible due to the fact that it is not written down. But we will write it down for clarity:

Let's replace subtraction with addition:

We got the addition of negative rational numbers. Add their modules and put a minus in front of the received answer. You can skip the entry with modules to avoid cluttering up the expression:

(?4,9) + (?5,9) = ?(4,9 + 5,9) = ?(10,8) = ?10,8

Thus, the value of the expression? 4,9 ? 5.9 equals?10.8

= ?(4,9 + 5,9) = ?(10,8) = ?10,8

Example 19. Find the value of the expression 7 ? 9.3

Enclose each number in brackets along with its signs

Let's replace subtraction with addition

We got the addition of rational numbers with different signs. Subtract the smaller module from the larger one and put the sign in front of the answer, the module of which is greater. You can skip the entry with modules to avoid cluttering up the expression:

(+7) + (?9,3) = ?(9,3 ? 7) = ?(2,3) = ?2,3

Thus, the value of the expression 7 ? 9.3 equals?2.3

The detailed solution of this example is written as follows:

7 ? 9,3 = (+7) ? (+9,3) = (+7) + (?9,3) = ?(|?9,3| ? |+7|) =

A short solution would look like this:

Example 20. Find the value of the expression? 0.25 ? (?1,2)

Let's replace subtraction with addition:

We got the addition of rational numbers with different signs. We subtract the smaller one from the larger one and put the sign in front of the answer, the module of which is greater:

0,25 + (+1,2) = |+1,2| ? |?0,25| = 1,2 ? 0,25 = 0,95

The detailed solution of this example is written as follows:

0,25 ? (?1,2) = (?0,25) + (+1,2) = |+1,2| ? |?0,25| = 1,2 ? 0,25 = 0,95

A short solution would look like this:

Example 21. Find the value of the expression?3.5 + (4.1 ? 7.1)

First of all, we will perform the actions in brackets, then add the answer received with the number? 3.5. Let's skip the entry with modules so as not to clutter up the expressions.

First action:

4,1 ? 7,1 = (+4,1) ? (+7,1) = (+4,1) + (?7,1) = ?(7,1 ? 4,1) = ?(3,0) = ?3,0

Second action:

3,5 + (?3,0) = ?(3,5 + 3,0) = ?(6,5) = ?6,5

Answer: the value of the expression ?3.5 + (4.1 ? 7.1) is ?6.5.

3,5 + (4,1 ? 7,1) = ?3,5 + (?3,0) = ?6,5

Example 22. Find the value of the expression (3.5 ? 2.9) ? (3.7 x 9.1)

Let's perform the actions in brackets, then from the number that turned out as a result of the execution of the first brackets, subtract the number that turned out as a result of the execution of the second brackets. Let's skip the entry with modules so as not to clutter up the expressions.

First action:

3,5 ? 2,9 = (+3,5) ? (+2,9) = (+3,5) + (?2,9) = 3,5 ? 2,9 = 0,6

Second action:

3,7 ? 9,1 = (+3,7) ? (+9,1) = (+3,7) + (?9,1) = ?(9,1 ? 3,7) = ?(5,4) = ?5,4

Third act

0,6 ? (?5,4) = (+0,6) + (+5,4) = 0,6 + 5,4 = 6,0 = 6

Answer: the value of the expression (3.5 ? 2.9) ? (3.7 ? 9.1) equals 6.

A short solution to this example can be written as follows:

(3,5 ? 2,9) ? (3,7 ? 9,1) = 0,6 ? (?5,4) = 6,0 = 6

Example 23. Find the value of the expression?3.8 + 17.15 ? 6.2? 6.15

Enclose in brackets every rational number along with its signs

Replace subtraction with addition where possible

The expression consists of several terms. According to the associative law of addition, if the expression consists of several terms, then the sum will not depend on the order of actions. This means that the terms can be added in any order.

We will not reinvent the wheel, but add all the terms from left to right in the order in which they appear:

First action:

(?3,8) + (+17,15) = 17,15 ? 3,80 = 13,35

Second action:

13,35 + (?6,2) = 13,35 ? ?6,20 = 7,15

Third action:

7,15 + (?6,15) = 7,15 ? 6,15 = 1,00 = 1

Answer: expression value? 3.8 + 17.15 ? 6.2? 6.15 is equal to 1.

A short solution to this example can be written as follows:

3,8 + 17,15 ? 6,2 ? 6,15 = 13,35 + (?6,2) ? 6,15 = 7,15 ? 6,15 = 1,00 = 1

Short solutions create fewer problems and confusion, so it's a good idea to get used to them.

Example 24. Find the value of an expression

Let's convert the decimal fraction? 1.8 to a mixed number. We'll rewrite the rest as is. If you have difficulty converting a decimal to a mixed number, be sure to repeat the lesson decimals.

Example 25. Find the value of an expression

Let's replace subtraction with addition. Along the way, we will translate the decimal fraction (? 4.4) into an improper fraction

There are no negative numbers in the resulting expression. And since there are no negative numbers, we can remove the plus in front of the second number, and omit the parentheses. Then we get a simple addition expression, which is easily solved

Example 26. Find the value of an expression

Let's convert the mixed number to an improper fraction, and the decimal fraction? 0.85 to an ordinary fraction. We get the following expression:

We got the addition of negative rational numbers. We add their modules and put a minus sign in front of the received answer. You can skip the entry with modules to avoid cluttering up the expression:

Example 27. Find the value of an expression

Convert both fractions to improper fractions. To convert the decimal 2.05 to an improper fraction, you can convert it first to a mixed number and then to an improper fraction:

After converting both fractions to improper fractions, we get the following expression:

We got the addition of rational numbers with different signs. We subtract the smaller one from the larger module and put the sign whose module is greater in front of the received answer:

Example 28. Find the value of an expression

Let's replace subtraction with addition. Let's convert a decimal to a common fraction

Example 29. Find the value of an expression

Convert decimal fractions? 0.25 and? 1.25 to common fractions, leave the rest as is. We get the following expression:

You can first replace subtraction with addition where possible and add the rational numbers one by one. There is a second option: first add the rational numbers and , and then subtract the rational number from the resulting number. We will use this option.

First action:

Second action:

Answer: expression value equal to?2.

Example 30. Find the value of an expression

Convert decimal fractions to common fractions. Let's leave the rest as is.

We got the sum of several terms. If the sum consists of several terms, then the expression can be evaluated in any order. This follows from the associative law of addition.

Therefore, we can organize the most convenient option for us. First of all, you can add the first and last terms, namely the rational numbers and . These numbers have same denominators, which means it will free us from the need to bring them to it.

First action:

The resulting number can be added to the second term, namely the rational number. Rational numbers have the same denominators in fractional parts, which again is an advantage for us

Second action:

Well, let's add the resulting number? 7 with the last term, namely with a rational number. It is convenient that when calculating this expression, the sevens will disappear, that is, their sum will be equal to zero, since the sum of opposite numbers is equal to zero

Third action:

Answer: the value of the expression is

Did you like the lesson?
Join our new group Vkontakte and start receiving notifications about new lessons

Addition and subtraction of whole numbers

In this lesson we will learn addition and subtraction of whole numbers, as well as rules for their addition and subtraction.

Recall that integers are all positive and negative numbers, as well as the number 0. For example, the following numbers are integers:

Positive numbers can be easily added and subtracted, multiplied and divided. Unfortunately, this cannot be said about negative numbers, which confuse many beginners with their minuses before each digit. As practice shows, mistakes made due to negative numbers upset students the most.

Integer addition and subtraction examples

The first thing to learn is to add and subtract whole numbers using the coordinate line. It is not necessary to draw a coordinate line. It is enough to imagine it in your thoughts and see where the negative numbers are located, and where are the positive ones.

Consider the simplest expression: 1 + 3. The value of this expression is 4:

This example can be understood using the coordinate line. To do this, from the point where the number 1 is located, you need to move three steps to the right. As a result, we will find ourselves at the point where the number 4 is located. In the figure you can see how this happens:

The plus sign in the expression 1 + 3 tells us that we should move to the right in the direction of increasing numbers.

Example 2 Let's find the value of the expression 1 ? 3.

The value of this expression is?2

This example can again be understood using the coordinate line. To do this, from the point where the number 1 is located, you need to move three steps to the left. As a result, we will find ourselves at the point where the negative number? 2 is located. The figure shows how this happens:

Minus sign in expression 1 ? 3 tells us that we should move to the left in the direction of decreasing numbers.

In general, we must remember that if addition is carried out, then we need to move to the right in the direction of increase. If subtraction is carried out, then you need to move to the left in the direction of decrease.

Example 3 Find the value of the expression?2 + 4

The value of this expression is 2

This example can again be understood using the coordinate line. To do this, from the point where the negative number? 2 is located, you need to move four steps to the right. As a result, we will find ourselves at the point where the positive number 2 is located.

It can be seen that we have moved from the point where the negative number? 2 is located to the right by four steps and ended up at the point where the positive number 2 is located.

The plus sign in the expression?2 + 4 tells us that we should move to the right in the direction of increasing numbers.

Example 4 Find the value of the expression?1 ? 3

The value of this expression is?4

This example can again be solved using a coordinate line. To do this, from the point where the negative number? 1 is located, you need to move three steps to the left. As a result, we will find ourselves at the point where the negative number is located? 4

It can be seen that we have moved from the point where the negative number? 1 is located to the left by three steps and ended up at the point where the negative number? 4 is located.

The minus sign in the expression?1 ? 3 tells us that we should move to the left in the direction of decreasing numbers.

Example 5 Find the value of the expression?2 + 2

The value of this expression is 0

This example can be solved using a coordinate line. To do this, from the point where the negative number? 2 is located, you need to move two steps to the right. As a result, we will find ourselves at the point where the number 0 is located

It can be seen that we have moved from the point where the negative number? 2 is located to the right by two steps and ended up at the point where the number 0 is located.

The plus sign in the expression?2 + 2 tells us that we should move to the right in the direction of increasing numbers.

Rules for adding and subtracting integers

To calculate this or that expression, it is not necessary to imagine the coordinate line every time, let alone draw it. It is more convenient to use ready-made rules.

When applying the rules, you need to pay attention to the sign of the operation and the signs of the numbers to be added or subtracted. This will determine which rule to apply.

Example 1 Find the value of the expression?2 + 5

Here a positive number is added to a negative number. In other words, the addition of numbers with different signs is carried out. ?2 is negative and 5 is positive. For such cases, the following rule is provided:

So, let's see which module is larger:

Is the modulus of 5 greater than the modulus of the number?2. The rule requires subtracting the smaller from the larger module. Therefore, we must subtract 2 from 5, and before the received answer put the sign whose modulus is greater.

The number 5 has a larger modulus, so the sign of this number will be in the answer. That is, the answer will be positive:

Is it usually written shorter? 2 + 5 = 3

Example 2 Find the value of the expression 3 + (?2)

Here, as in the previous example, the addition of numbers with different signs is carried out. 3 is a positive number and ?2 is negative. Note that the number?2 is enclosed in brackets to make the expression clearer and prettier. This expression is much easier to understand than the expression 3+?2.

So, we apply the rule of adding numbers with different signs. As in the previous example, we subtract the smaller module from the larger module and put the sign in front of the answer, the module of which is greater:

3 + (?2) = |3| ? |?2| = 3 ? 2 = 1

The modulus of the number 3 is greater than the modulus of the number?2, so we subtracted 2 from 3, and put the sign of the modulus, which is greater, in front of the received answer. The number 3 has a larger module, so the sign of this number is put in the answer. That is, the answer is yes.

Usually written shorter 3 + (? 2) = 1

Example 3 Find the value of the expression 3 ? 7

In this expression, the larger number is subtracted from the smaller number. For such a case, the following rule is provided:

To subtract a larger number from a smaller number, more subtract the smaller and put a minus in front of the answer.

There is a slight snag in this expression. Recall that the equal sign (=) is placed between values ​​and expressions when they are equal to each other.

The value of expression 3 ? 7 how did we know equal?4. This means that any transformations that we will perform in this expression must be equal?4

But we see that the second stage contains the expression 7 ? 3, which is not equal to?4.

To remedy this situation, the expression 7 ? 3 must be taken in brackets and put a minus in front of this bracket:

3 ? 7 = ? (7 ? 3) = ? (4) = ?4

In this case, equality will be observed at each stage:

After the expression has been evaluated, the parentheses can be removed, which we did.

So to be more precise, the solution should look like this:

3 ? 7 = ? (7 ? 3) = ? (4) = ? 4

This rule can be written using variables. It will look like this:

a? b=? (b? a)

A large number of brackets and operation signs can complicate the solution of a seemingly very simple task, so it is more expedient to learn how to write such examples briefly, for example 3 ? 7=? 4.

In fact, the addition and subtraction of integers is reduced to just addition. What does this mean? This means that if you want to subtract numbers, this operation can be replaced by addition.

So let's get acquainted with the new rule:

To subtract one number from another means to add to the minuend a number that will be the opposite of the subtracted one.

For example, consider the simplest expression 5 ? 3. At the initial stages of learning mathematics, we simply put an equal sign and wrote down the answer:

But now we are progressing in learning, so we need to adapt to the new rules. The new rule says that to subtract one number from another means to add to the minuend a number that will be the opposite of the one subtracted.

Using the expression 5?3 as an example, let's try to understand this rule. What is reduced in this expression is 5, and what is subtracted is 3. The rule says that in order to subtract 3 from 5, you need to add to 5 a number that will be opposite to 3. The opposite number for the number 3 is? 3. We write a new expression:

And we already know how to find values ​​for such expressions. This is the addition of numbers with different signs, which we discussed above. To add numbers with different signs, you need to subtract the smaller one from the larger module, and put the sign whose module is greater in front of the received answer:

5 + (?3) = |5| ? |?3| = 5 ? 3 = 2

Is the modulus of 5 greater than the modulus of the number?3. Therefore, we subtracted 3 from 5 and got 2. The number 5 has a larger modulus, so the sign of this number was put in the answer. That is, the answer is positive.

At first, not everyone succeeds in quickly replacing subtraction with addition. This is because positive numbers are written without their plus sign.

For example, in the expression 3 ? The 1 minus sign indicating subtraction is the sign of the operation and does not refer to one. The unit in this case is a positive number and it has its own plus sign, but we don’t see it, because plus is traditionally not written before positive numbers.

And so for the sake of clarity given expression can be written as follows:

For convenience, numbers with their signs are enclosed in brackets. In this case, replacing subtraction with addition is much easier. Subtracted in this case is the number (+1), and the opposite number (?1). Let's replace the operation of subtraction with addition and instead of the subtrahend (+1) we write down the opposite number (? 1)

(+3) ? (+1) = (+3) + (?1) = |+3| ? |?1| = 3 ? 1 = 2

At first glance, it would seem that there is no point in these extra gestures, if you can use the good old method to put an equal sign and immediately write down the answer 2. In fact, this rule will help us out more than once.

Let's solve the previous example 3 ? 7 using the subtraction rule. First, we bring the expression to normal form, placing each number with its signs. Three has a plus sign because it is a positive number. The minus indicating subtraction does not apply to the seven. Seven has a plus sign because it is also a positive number:

Let's replace subtraction with addition:

Further calculation is not difficult:

Example 7 Find the value of the expression?4 ? five

Before us is the operation of subtraction again. This operation must be replaced by addition. To the diminished (?4) we add the number opposite to the subtracted (+5). The opposite number for the subtrahend (+5) is the number (?5).

We have come to a situation where we need to add negative numbers. For such cases, the following rule is provided:

To add negative numbers, you need to add their modules, and put a minus in front of the received answer.

So, let's add the modules of numbers, as the rule requires us to do, and put a minus in front of the received answer:

(?4) ? (+5) = (?4) + (?5) = |?4| + |?5| = 4 + 5 = ?9

The entry with modules must be enclosed in brackets and put a minus before these brackets. So we provide a minus, which should come before the answer:

(?4) ? (+5) = (?4) + (?5) = ?(|?4| + |?5|) = ?(4 + 5) = ?(9) = ?9

The solution for this example can be written shorter:

Example 8 Find the value of the expression?3 ? five ? 7? nine

Let's bring the expression to a clear form. Here, all numbers except the number?3 are positive, so they will have plus signs:

Let us replace the operations of subtraction with the operations of addition. All minuses (except the minus, which is in front of the three) will change to pluses and all positive numbers will change to the opposite:

Now apply the rule for adding negative numbers. To add negative numbers, you need to add their modules and put a minus in front of the received answer:

= ?(|?3| + |?5| + |?7| + |?9|) = ?(3 + 5 + 7 + 9) = ?(24) = ?24

The solution for this example can be written shorter:

3 ? 5 ? 7 ? 9 = ?(3 + 5 + 7 + 9) = ?24

Example 9 Find the value of the expression?10 + 6 ? 15 + 11? 7

Let's bring the expression to a clear form:

There are two operations here: addition and subtraction. We leave addition as it is, and replace subtraction with addition:

(?10) + (+6) ? (+15) + (+11) ? (+7) = (?10) + (+6) + (?15) + (+11) + (?7)

Following the order of actions, we will perform each action in turn, based on the previously studied rules. Entries with modules can be skipped:

First action:

(?10) + (+6) = ? (10 ? 6) = ? (4) = ? 4

Second action:

(?4) + (?15) = ? (4 + 15) = ? (19) = ? 19

Third action:

(?19) + (+11) = ? (19 ? 11) = ? (8) = ?8

Fourth action:

(?8) + (?7) = ? (8 + 7) = ? (15) = ? 15

So the value of the expression ?10 + 6 ? 15 + 11? 7 equals?15

Note. It is not necessary to bring the expression to a clear form by enclosing numbers in brackets. When getting used to negative numbers, this action can be skipped, as it takes time and can be confusing.

So, for adding and subtracting integers, you need to remember the following rules:

To add numbers with different signs, you need to subtract a smaller module from a larger module, and put the sign whose module is larger in front of the answer.

To subtract a larger number from a smaller number, you need to subtract the smaller number from the larger number and put a minus sign in front of the received answer.

To subtract one number from another means to add to the reduced number the opposite of the subtracted one.

To add negative numbers, you need to add their modules, and put a minus sign in front of the received answer.

• Hockey without rules VKontakte The game was released in September 2012, and has already gained almost 700,000 users. There are two game modes and many opportunities for team building. The course of the match in Ultimate Hockey VKontakte is reminiscent of the early games of the NHL series from Electronic Arts. 3 players on […]
• Omaha Holdem Poker Rules Omaha Hi-Lo and Omaha Five Card Omaha Holdem (Omaha Hold "Em) is a slight modification of Texas Hold'em. If you are new to this most popular type of poker, study the rules of Texas Hold'em at the link; their knowledge is necessary to understand the rules of Omaha . Everything […]
• Solving problems in genetics using Mendel's laws 1 and 2 Lecture 8 Julia Kjahrenova 1. - presentation The presentation was published 3 years ago by Alina Artemyeva ." […]
• 5-7 rule algebra A numerical sequence, each member of which, starting from the second, is equal to the previous one, added with the same number d for this sequence, is called an arithmetic progression. The number d is called the difference arithmetic progression. In arithmetic progression, i.e. in […]
• We determine the transport tax rate for vans and other atypical vehicles with category "B" We catch the necessary information from the TCP Let's say right away that the data indicated in line 4 "Vehicle category (A, B, C, D, trailer)" of the passport vehicle(PTS), do not need to be taken into account. After all, the category "B" does not mean at all […]
• Rating of insurance companies OSAGO OSAGO refers to compulsory insurance, it is valid not only in Russia, but also in other countries of the near abroad. These policies are issued by many insurance companies that have received the appropriate license to conduct such activities. But, […]
• Accommodation Ufa hotel Mini-hotel in Ufa 5 Five Rooms We invite guests of the capital to a cozy and comfortable hotel located in the center of Ufa along Komsomolskaya street 159/1. In the immediate vicinity of the hotel there is the Iskra IMAX cinema complex, a circus, a restaurant-club A cafe, a Beer Berry restaurant, a […]
• Rules for using Present Simple Tense in English Present Simple Tense is a grammatical tense that is considered one of the easiest to understand, since the present simple tense exists in all languages. IN Slavic languages Yes sir. If you are reading this article, it means that you are only […]

How to multiply powers? Which powers can be multiplied and which cannot? How do you multiply a number by a power?

In algebra, you can find the product of powers in two cases:

1) if the degrees have the same basis;

2) if the degrees have the same indicators.

When multiplying powers with the same base, the base must remain the same, and the exponents must be added:

When multiplying degrees with the same indicators, the total indicator can be taken out of brackets:

Consider how to multiply powers, with specific examples.

The unit in the exponent is not written, but when multiplying the degrees, they take into account:

When multiplying, the number of degrees can be any. It should be remembered that you can not write the multiplication sign before the letter:

In expressions, exponentiation is performed first.

If you need to multiply a number by a power, you must first perform exponentiation, and only then - multiplication:

www.algebraclass.ru

Addition, subtraction, multiplication, and division of powers

Addition and subtraction of powers

Obviously, numbers with powers can be added like other quantities , by adding them one by one with their signs.

So, the sum of a 3 and b 2 is a 3 + b 2 .
The sum of a 3 - b n and h 5 -d 4 is a 3 - b n + h 5 - d 4.

Odds the same powers of the same variables can be added or subtracted.

So, the sum of 2a 2 and 3a 2 is 5a 2 .

It is also obvious that if we take two squares a, or three squares a, or five squares a.

But degrees various variables And various degrees identical variables, must be added by adding them to their signs.

So, the sum of a 2 and a 3 is the sum of a 2 + a 3 .

It is obvious that the square of a, and the cube of a, is neither twice the square of a, but twice the cube of a.

The sum of a 3 b n and 3a 5 b 6 is a 3 b n + 3a 5 b 6 .

Subtraction powers are carried out in the same way as addition, except that the signs of the subtrahend must be changed accordingly.

Or:
2a 4 - (-6a 4) = 8a 4
3h 2 b 6 - 4h 2 b 6 \u003d -h 2 b 6
5(a - h) 6 - 2(a - h) 6 = 3(a - h) 6

Power multiplication

Numbers with powers can be multiplied like other quantities by writing them one after the other, with or without the multiplication sign between them.

So, the result of multiplying a 3 by b 2 is a 3 b 2 or aaabb.

Or:
x -3 ⋅ a m = a m x -3
3a 6 y 2 ⋅ (-2x) = -6a 6 xy 2
a 2 b 3 y 2 ⋅ a 3 b 2 y = a 2 b 3 y 2 a 3 b 2 y

The result in the last example can be ordered by adding the same variables.
The expression will take the form: a 5 b 5 y 3 .

By comparing several numbers (variables) with powers, we can see that if any two of them are multiplied, then the result is a number (variable) with a power equal to sum degrees of terms.

So, a 2 .a 3 = aa.aaa = aaaaa = a 5 .

Here 5 is the power of the result of the multiplication, equal to 2 + 3, the sum of the powers of the terms.

So, a n .a m = a m+n .

For a n , a is taken as a factor as many times as the power of n is;

And a m , is taken as a factor as many times as the degree m is equal to;

That's why, powers with the same bases can be multiplied by adding the exponents.

So, a 2 .a 6 = a 2+6 = a 8 . And x 3 .x 2 .x = x 3+2+1 = x 6 .

Or:
4a n ⋅ 2a n = 8a 2n
b 2 y 3 ⋅ b 4 y = b 6 y 4
(b + h - y) n ⋅ (b + h - y) = (b + h - y) n+1

Multiply (x 3 + x 2 y + xy 2 + y 3) ⋅ (x - y).
Answer: x 4 - y 4.
Multiply (x 3 + x - 5) ⋅ (2x 3 + x + 1).

This rule is also true for numbers whose exponents are − negative.

1. So, a -2 .a -3 = a -5 . This can be written as (1/aa).(1/aaa) = 1/aaaaa.

2. y-n .y-m = y-n-m .

3. a -n .a m = a m-n .

If a + b are multiplied by a - b, the result will be a 2 - b 2: that is

The result of multiplying the sum or difference of two numbers is equal to the sum or the difference of their squares.

If the sum and difference of two numbers raised to square, the result will be equal to the sum or difference of these numbers in fourth degree.

So, (a - y).(a + y) = a 2 - y 2 .
(a 2 - y 2)⋅(a 2 + y 2) = a 4 - y 4 .
(a 4 - y 4)⋅(a 4 + y 4) = a 8 - y 8 .

Division of powers

Power numbers can be divided like other numbers by subtracting from the divisor, or by placing them in fraction form.

So a 3 b 2 divided by b 2 is a 3 .

Writing a 5 divided by a 3 looks like $\frac $. But this is equal to a 2 . In a series of numbers
a +4 , a +3 , a +2 , a +1 , a 0 , a -1 , a -2 , a -3 , a -4 .
any number can be divided by another, and the exponent will be equal to difference indicators of divisible numbers.

When dividing powers with the same base, their exponents are subtracted..

So, y 3:y 2 = y 3-2 = y 1 . That is, $\frac = y$.

And a n+1:a = a n+1-1 = a n . That is, $\frac = a^n$.

Or:
y2m: ym = ym
8a n+m: 4a m = 2a n
12(b + y) n: 3(b + y) 3 = 4(b + y) n-3

The rule is also valid for numbers with negative degree values.
The result of dividing a -5 by a -3 is a -2 .
Also, $\frac: \frac = \frac .\frac = \frac = \frac $.

h 2:h -1 = h 2+1 = h 3 or $h^2:\frac = h^2.\frac = h^3$

It is necessary to master the multiplication and division of powers very well, since such operations are very widely used in algebra.

Examples of solving examples with fractions containing numbers with powers

1. Reduce exponents in $\frac $ Answer: $\frac $.

2. Reduce the exponents in $\frac$. Answer: $\frac $ or 2x.

3. Reduce the exponents a 2 / a 3 and a -3 / a -4 and bring to a common denominator.
a 2 .a -4 is a -2 first numerator.
a 3 .a -3 is a 0 = 1, the second numerator.
a 3 .a -4 is a -1 , the common numerator.
After simplification: a -2 /a -1 and 1/a -1 .

4. Reduce the exponents 2a 4 /5a 3 and 2 /a 4 and bring to a common denominator.
Answer: 2a 3 / 5a 7 and 5a 5 / 5a 7 or 2a 3 / 5a 2 and 5/5a 2.

5. Multiply (a 3 + b)/b 4 by (a - b)/3.

6. Multiply (a 5 + 1)/x 2 by (b 2 - 1)/(x + a).

7. Multiply b 4 /a -2 by h -3 /x and a n /y -3 .

8. Divide a 4 /y 3 by a 3 /y 2 . Answer: a/y.

degree properties

We remind you that in this lesson we understand degree properties with natural indicators and zero. Degrees with rational indicators and their properties will be discussed in lessons for grade 8.

A degree with a natural indicator has several important properties, which allow you to simplify calculations in examples with powers.

Property #1
Product of powers

When multiplying powers with the same base, the base remains unchanged, and the exponents are added.

a m a n \u003d a m + n, where "a" is any number, and "m", "n" are any natural numbers.

This property of powers also affects the product of three or more powers.

Simplify the expression.
b b 2 b 3 b 4 b 5 = b 1 + 2 + 3 + 4 + 5 = b 15

Present as a degree.
6 15 36 = 6 15 6 2 = 6 15 6 2 = 6 17

Present as a degree.
(0.8) 3 (0.8) 12 = (0.8) 3 + 12 = (0.8) 15

Please note that in the indicated property it was only about multiplying powers with the same bases.. It does not apply to their addition.

You cannot replace the sum (3 3 + 3 2) with 3 5 . This is understandable if
calculate (3 3 + 3 2) = (27 + 9) = 36 and 3 5 = 243

Property #2
Private degrees

When dividing powers with the same base, the base remains unchanged, and the exponent of the divisor is subtracted from the exponent of the dividend.

Write the quotient as a power
(2b) 5: (2b) 3 = (2b) 5 − 3 = (2b) 2

Calculate.

11 3 - 2 4 2 - 1 = 11 4 = 44
Example. Solve the equation. We use the property of partial degrees.
3 8: t = 3 4

Answer: t = 3 4 = 81

Using properties No. 1 and No. 2, you can easily simplify expressions and perform calculations.

Example. Simplify the expression.
4 5m + 6 4 m + 2: 4 4m + 3 = 4 5m + 6 + m + 2: 4 4m + 3 = 4 6m + 8 − 4m − 3 = 4 2m + 5

Example. Find the value of an expression using degree properties.

2 11 − 5 = 2 6 = 64

Please note that property 2 dealt only with the division of powers with the same bases.

You cannot replace the difference (4 3 −4 2) with 4 1 . This is understandable if you calculate (4 3 −4 2) = (64 − 16) = 48, and 4 1 = 4

Property #3
Exponentiation

When raising a power to a power, the base of the power remains unchanged, and the exponents are multiplied.

(a n) m \u003d a n m, where "a" is any number, and "m", "n" are any natural numbers.

Please note that property No. 4, like other properties of degrees, is also applied in reverse order.

(a n b n)= (a b) n

That is, to multiply powers with the same exponents, you can multiply the bases, and leave the exponent unchanged.

Example. Calculate.
2 4 5 4 = (2 5) 4 = 10 4 = 10,000

Example. Calculate.
0.5 16 2 16 = (0.5 2) 16 = 1

In more difficult examples there may be cases when multiplication and division must be performed over powers with different bases and different indicators. In this case, we advise you to do the following.

For example, 4 5 3 2 = 4 3 4 2 3 2 = 4 3 (4 3) 2 = 64 12 2 = 64 144 = 9216

Example of exponentiation of a decimal fraction.

4 21 (−0.25) 20 = 4 4 20 (−0.25) 20 = 4 (4 (−0.25)) 20 = 4 (−1) 20 = 4 1 = 4

Properties 5
Power of the quotient (fractions)

To raise a quotient to a power, you can raise the dividend and the divisor separately to this power, and divide the first result by the second.

(a: b) n \u003d a n: b n, where "a", "b" are any rational numbers, b ≠ 0, n is any natural number.

Example. Express the expression as partial powers.
(5: 3) 12 = 5 12: 3 12

We remind you that a quotient can be represented as a fraction. Therefore, we will dwell on the topic of raising a fraction to a power in more detail on the next page.

Degrees and Roots

Operations with powers and roots. Degree with negative ,

zero and fractional indicator. About expressions that don't make sense.

Operations with degrees.

1. When multiplying powers with the same base, their indicators are added up:

a m · a n = a m + n .

2. When dividing degrees with the same base, their indicators subtracted .

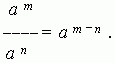
3. The degree of the product of two or more factors is equal to the product of the degrees of these factors.

4. The degree of the ratio (fraction) is equal to the ratio of the degrees of the dividend (numerator) and divisor (denominator):

(a/b) n = a n / b n .

5. When raising a degree to a power, their indicators are multiplied:

All of the above formulas are read and executed in both directions from left to right and vice versa.

EXAMPLE (2 3 5 / 15)² = 2 ² 3 ² 5 ² / 15 ² = 900 / 225 = 4 .

Operations with roots. In all the formulas below, the symbol means arithmetic root(radical expression is positive).

1. The root of the product of several factors is equal to the product of the roots of these factors:

2. The root of the relationship is equal to the ratio roots of the dividend and divisor:

3. When raising a root to a power, it is enough to raise to this power root number:

4. If you increase the degree of the root by m times and simultaneously raise the root number to the m -th degree, then the value of the root will not change:

5. If you reduce the degree of the root by m times and at the same time extract the root of the m-th degree from the radical number, then the value of the root will not change:

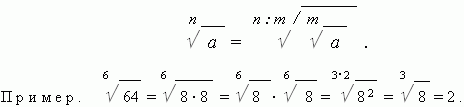
Extension of the concept of degree. So far, we have considered degrees only with a natural indicator; but operations with powers and roots can also lead to negative, zero And fractional indicators. All these exponents require an additional definition.

Degree with a negative exponent. The degree of a certain number with a negative (integer) exponent is defined as one divided by the degree of the same number with an exponent equal to the absolute value of the negative exponent:

Now the formula a m : a n = a m-n can be used not only for m, more than n, but also at m, less than n .

EXAMPLE a 4: a 7 = a 4 — 7 = a — 3 .

If we want the formula a m : a n = a m — n was fair at m = n, we need a definition of the zero degree.

Degree with zero exponent. The degree of any non-zero number with zero exponent is 1.

EXAMPLES. 2 0 = 1, ( – 5) 0 = 1, (– 3 / 5) 0 = 1.

Degree with a fractional exponent. In order to raise a real number a to the power m / n, you need to extract the root of the nth degree from the mth power of this number a:

About expressions that don't make sense. There are several such expressions.

where a ≠ 0 , does not exist.

Indeed, if we assume that x is a certain number, then, in accordance with the definition of the division operation, we have: a = 0· x, i.e. a= 0, which contradicts the condition: a ≠ 0

— any number.

Indeed, if we assume that this expression is equal to some number x, then according to the definition of the division operation we have: 0 = 0 x. But this equality holds for any number x, which was to be proved.

0 0 — any number.

Solution. Consider three main cases:

1) x = 0 – this value does not satisfy this equation

2) when x> 0 we get: x / x= 1, i.e. 1 = 1, whence follows,

what x- any number; but taking into account that

our case x> 0 , the answer is x > 0 ;

Rules for multiplying powers with different bases

DEGREE WITH A RATIONAL INDICATOR,

POWER FUNCTION IV

§ 69. Multiplication and division of powers with the same bases

Theorem 1. To multiply powers with the same bases, it is enough to add the exponents, and leave the base the same, that is

Proof. By definition of degree

2 2 2 3 = 2 5 = 32; (-3) (-3) 3 = (-3) 4 = 81.

We have considered the product of two powers. In fact, the proved property is true for any number of powers with the same bases.

Theorem 2. To divide powers with the same base when the exponent of the dividend more than the indicator divisor, it is enough to subtract the divisor from the indicator of the dividend, and leave the base the same, that is at t > n

(a =/= 0)

Proof. Recall that the quotient of dividing one number by another is the number that, when multiplied by a divisor, gives the dividend. Therefore, prove the formula , where a =/= 0, it's like proving the formula

If t > n , then the number t - p will be natural; therefore, by Theorem 1

Theorem 2 is proved.

Note that the formula

proved by us only under the assumption that t > n . Therefore, from what has been proved, it is not yet possible to draw, for example, the following conclusions:

In addition, we have not yet considered degrees with negative exponents, and we do not yet know what meaning can be given to the expression 3 - 2 .

Theorem 3. To raise a power to a power, it is enough to multiply the exponents, leaving the base of the exponent the same, i.e

Proof. Using the definition of degree and Theorem 1 of this section, we get:

Q.E.D.

For example, (2 3) 2 = 2 6 = 64;

518 (Oral.) Determine X from the equations:

1) 2 2 2 2 3 2 4 2 5 2 6 = 2 x ; 3) 4 2 4 4 4 6 4 8 4 10 = 2 x ;

2) 3 3 3 3 5 3 7 3 9 = 3 x ; 4) 1 / 5 1 / 25 1 / 125 1 / 625 = 1 / 5 x .

519. (Adjusted) Simplify:

520. (Adjusted) Simplify:

521. Present these expressions as degrees with the same bases:

1) 32 and 64; 3) 85 and 163; 5) 4 100 and 32 50;

2) -1000 and 100; 4) -27 and -243; 6) 81 75 8 200 and 3 600 4 150.

We remind you that in this lesson we understand degree properties with natural indicators and zero. Degrees with rational indicators and their properties will be discussed in lessons for grade 8.

An exponent with a natural exponent has several important properties that allow you to simplify calculations in exponent examples.

Property #1
Product of powers

Remember!

When multiplying powers with the same base, the base remains unchanged, and the exponents are added.

a m a n \u003d a m + n, where " a"- any number, and" m", " n"- any natural numbers.

This property of powers also affects the product of three or more powers.

• Simplify the expression.
  b b 2 b 3 b 4 b 5 = b 1 + 2 + 3 + 4 + 5 = b 15
• Present as a degree.
  6 15 36 = 6 15 6 2 = 6 15 6 2 = 6 17
• Present as a degree.
  (0.8) 3 (0.8) 12 = (0.8) 3 + 12 = (0.8) 15

Important!

Please note that in the indicated property it was only about multiplying powers with the same grounds . It does not apply to their addition.

You cannot replace the sum (3 3 + 3 2) with 3 5 . This is understandable if
calculate (3 3 + 3 2) = (27 + 9) = 36 and 3 5 = 243

Property #2
Private degrees

Remember!

When dividing powers with the same base, the base remains unchanged, and the exponent of the divisor is subtracted from the exponent of the dividend.

= 11 3 − 2 4 2 − 1 = 11 4 = 44

Example. Solve the equation. We use the property of partial degrees.
3 8: t = 3 4

T = 3 8 − 4

Answer: t = 3 4 = 81

Using properties No. 1 and No. 2, you can easily simplify expressions and perform calculations.

• Example. Simplify the expression.
  4 5m + 6 4 m + 2: 4 4m + 3 = 4 5m + 6 + m + 2: 4 4m + 3 = 4 6m + 8 − 4m − 3 = 4 2m + 5
• Example. Find the value of an expression using degree properties.
  = = = 2 9 + 2

  2 5

  = 2 11

  2 5

  = 2 11 − 5 = 2 6 = 64

  Important!

  Please note that property 2 dealt only with the division of powers with the same bases.

  You cannot replace the difference (4 3 −4 2) with 4 1 . This is understandable if we consider (4 3 −4 2) = (64 − 16) = 48 , and 4 1 = 4

  Be careful!

  Property #3
  Exponentiation

  Remember!

  When raising a power to a power, the base of the power remains unchanged, and the exponents are multiplied.

  (a n) m \u003d a n m, where "a" is any number, and "m", "n" are any natural numbers.

  
  

  Properties 4
  Product degree

  Remember!

  When raising a product to a power, each of the factors is raised to a power. The results are then multiplied.

  (a b) n \u003d a n b n, where "a", "b" are any rational numbers; "n" - any natural number.

  • Example 1
    (6 a 2 b 3 c) 2 = 6 2 a 2 2 b 3 2 s 1 2 = 36 a 4 b 6 s 2
    
  • Example 2
    (−x 2 y) 6 = ((−1) 6 x 2 6 y 1 6) = x 12 y 6

  Important!

  Please note that property No. 4, like other properties of degrees, is also applied in reverse order.

  (a n b n)= (a b) n

  That is, to multiply degrees with the same exponents, you can multiply the bases, and leave the exponent unchanged.

  • Example. Calculate.
    2 4 5 4 = (2 5) 4 = 10 4 = 10,000
  • Example. Calculate.
    0.5 16 2 16 = (0.5 2) 16 = 1

  In more complex examples, there may be cases when multiplication and division must be performed on powers with different bases and different exponents. In this case, we advise you to do the following.

  For example, 4 5 3 2 = 4 3 4 2 3 2 = 4 3 (4 3) 2 = 64 12 2 = 64 144 = 9216
  

  Example of exponentiation of a decimal fraction.

  4 21 (−0.25) 20 = 4 4 20 (−0.25) 20 = 4 (4 (−0.25)) 20 = 4 (−1) 20 = 4 1 = 4

  Properties 5
  Power of the quotient (fractions)

  Remember!

  To raise a quotient to a power, you can raise the dividend and divisor separately to this power, and divide the first result by the second.

  (a: b) n \u003d a n: b n, where "a", "b" are any rational numbers, b ≠ 0, n is any natural number.

  • Example. Express the expression as partial powers.
    (5: 3) 12 = 5 12: 3 12

  We remind you that a quotient can be represented as a fraction. Therefore, we will dwell on the topic of raising a fraction to a power in more detail on the next page.

In the previous article, we talked about what monomials are. In this material, we will analyze how to solve examples and problems in which they are used. Here we will consider such actions as subtraction, addition, multiplication, division of monomials and raising them to a power with a natural exponent. We will show how such operations are defined, indicate the basic rules for their implementation and what should be the result. All theoretical provisions, as usual, will be illustrated by examples of problems with descriptions of solutions.

It is most convenient to work with the standard notation of monomials, therefore, we present all the expressions that will be used in the article in a standard form. If they are initially set differently, it is recommended to first bring them to a generally accepted form.

Rules for adding and subtracting monomials

The simplest operations that can be performed with monomials are subtraction and addition. IN general case the result of these actions will be a polynomial (a monomial is possible in some special cases).

When we add or subtract monomials, we first write down the corresponding sum and difference in the generally accepted form, after which we simplify the resulting expression. If there are similar terms, they must be given, the brackets must be opened. Let's explain with an example.

Example 1

Condition: add the monomials − 3 · x and 2 , 72 · x 3 · y 5 · z .

Solution

Let's write down the sum of the original expressions. Add parentheses and put a plus sign between them. We will get the following:

(− 3 x) + (2 , 72 x 3 y 5 z)

When we expand the brackets, we get - 3 x + 2 , 72 x 3 y 5 z . This is a polynomial, written in standard form, which will be the result of adding these monomials.

Answer:(− 3 x) + (2 , 72 x 3 y 5 z) = − 3 x + 2 , 72 x 3 y 5 z .

If we have three, four or more terms given, we perform this action in the same way.

Example 2

Condition: swipe in right order specified operations with polynomials

3 a 2 - (- 4 a c) + a 2 - 7 a 2 + 4 9 - 2 2 3 a c

Solution

Let's start by opening parentheses.

3 a 2 + 4 a c + a 2 - 7 a 2 + 4 9 - 2 2 3 a c

We see that the resulting expression can be simplified by reducing like terms:

3 a 2 + 4 a c + a 2 - 7 a 2 + 4 9 - 2 2 3 a c = = (3 a 2 + a 2 - 7 a 2) + 4 a c - 2 2 3 a c + 4 9 = = - 3 a 2 + 1 1 3 a c + 4 9

We have a polynomial, which will be the result of this action.

Answer: 3 a 2 - (- 4 a c) + a 2 - 7 a 2 + 4 9 - 2 2 3 a c = - 3 a 2 + 1 1 3 a c + 4 9

In principle, we can perform the addition and subtraction of two monomials, with some restrictions, so that we end up with a monomial. To do this, it is necessary to observe some conditions regarding the terms and subtracted monomials. We will describe how this is done in a separate article.

Rules for multiplying monomials

The multiplication action does not impose any restrictions on multipliers. The monomials to be multiplied must not meet any additional conditions in order for the result to be a monomial.

To perform multiplication of monomials, you need to perform the following steps:

1. Record the piece correctly.
2. Expand the brackets in the resulting expression.
3. Group, if possible, factors with the same variables and numerical factors separately.
4. Perform the necessary actions with numbers and apply the property of multiplying powers with the same bases to the remaining factors.

Let's see how this is done in practice.

Example 3

Condition: multiply the monomials 2 · x 4 · y · z and - 7 16 · t 2 · x 2 · z 11 .

Solution

Let's start with the composition of the work.

Opening the brackets in it and we get the following:

2 x 4 y z - 7 16 t 2 x 2 z 11

2 - 7 16 t 2 x 4 x 2 y z 3 z 11

All we have to do is multiply the numbers in the first brackets and apply the power property to the second. As a result, we get the following:

2 - 7 16 t 2 x 4 x 2 y z 3 z 11 = - 7 8 t 2 x 4 + 2 y z 3 + 11 = = - 7 8 t 2 x 6 y z 14

Answer: 2 x 4 y z - 7 16 t 2 x 2 z 11 = - 7 8 t 2 x 6 y z 14 .

If we have three or more polynomials in the condition, we multiply them using exactly the same algorithm. We will consider the issue of multiplication of monomials in more detail in a separate material.

Rules for raising a monomial to a power

We know that the product of a certain number of identical factors is called a degree with a natural exponent. Their number is indicated by the number in the index. According to this definition, raising a monomial to a power is equivalent to multiplying the indicated number of identical monomials. Let's see how it's done.

Example 4

Condition: raise the monomial − 2 · a · b 4 to the power of 3 .

Solution

We can replace exponentiation with multiplication of 3 monomials − 2 · a · b 4 . Let's write down and get the desired answer:

(− 2 a b 4) 3 = (− 2 a b 4) (− 2 a b 4) (− 2 a b 4) = = ((− 2) (− 2) (− 2)) (a a a) (b 4 b 4 b 4) = − 8 a 3 b 12

Answer:(− 2 a b 4) 3 = − 8 a 3 b 12 .

But what about when the degree has a large exponent? Write down a large number of multipliers are inconvenient. Then, to solve such a problem, we need to apply the properties of the degree, namely the property of the degree of the product and the property of the degree in the degree.

Let's solve the problem that we cited above in the indicated way.

Example 5

Condition: raise − 2 · a · b 4 to the third power.

Solution

Knowing the property of the degree in the degree, we can proceed to an expression of the following form:

(− 2 a b 4) 3 = (− 2) 3 a 3 (b 4) 3 .

After that, we raise to the power - 2 and apply the exponent property:

(− 2) 3 (a) 3 (b 4) 3 = − 8 a 3 b 4 3 = − 8 a 3 b 12 .

Answer:− 2 · a · b 4 = − 8 · a 3 · b 12 .

We also devoted a separate article to raising a monomial to a power.

Rules for dividing monomials

The last operation with monomials, which we will analyze in this material, is the division of a monomial by a monomial. As a result, we should get a rational (algebraic) fraction (in some cases, it is possible to obtain a monomial). Let us clarify right away that division by zero monomial is not defined, since division by 0 is not defined.

To perform division, we need to write the indicated monomials in the form of a fraction and reduce it, if possible.

Example 6

Condition: divide the monomial − 9 x 4 y 3 z 7 by − 6 p 3 t 5 x 2 y 2 .

Solution

Let's start by writing the monomials in the form of a fraction.

9 x 4 y 3 z 7 - 6 p 3 t 5 x 2 y 2

This fraction can be reduced. After doing this, we get:

3 x 2 y z 7 2 p 3 t 5

Answer:- 9 x 4 y 3 z 7 - 6 p 3 t 5 x 2 y 2 = 3 x 2 y z 7 2 p 3 t 5 .

The conditions under which, as a result of dividing monomials, we get a monomial are given in a separate article.

If you notice a mistake in the text, please highlight it and press Ctrl+Enter