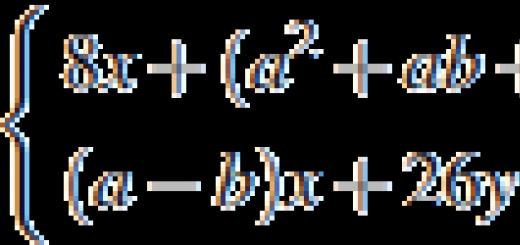In this article we will find answers to questions about how to create a projection of a point onto a plane and how to determine the coordinates of this projection. In the theoretical part we will rely on the concept of projection. We will define the terms and provide information with illustrations. Let's consolidate the acquired knowledge by solving examples.
Projection, types of projection
For the convenience of viewing spatial figures, drawings depicting these figures are used.
Definition 1
Projection of a figure onto a plane– drawing of a spatial figure.
Obviously, there are a number of rules used to construct a projection.
Definition 2
Projection– the process of constructing a drawing of a spatial figure on a plane using construction rules.
Projection plane- this is the plane in which the image is constructed.
The use of certain rules determines the type of projection: central or parallel.
A special case of parallel projection is perpendicular projection or orthogonal: in geometry it is mainly used. For this reason, in speech the adjective “perpendicular” itself is often omitted: in geometry they simply say “projection of a figure” and by this they mean constructing a projection using the method of perpendicular projection. In special cases, of course, something else may be agreed.
Let us note the fact that the projection of a figure onto a plane is essentially a projection of all points of this figure. Therefore, in order to be able to study a spatial figure in a drawing, it is necessary to acquire the basic skill of projecting a point onto a plane. What we will talk about below.
Let us recall that most often in geometry, when speaking about projection onto a plane, they mean the use of a perpendicular projection.
Let us make constructions that will give us the opportunity to obtain a definition of the projection of a point onto a plane.
Let's say a three-dimensional space is given, and in it there is a plane α and a point M 1 that does not belong to the plane α. Draw a straight line through the given point M A perpendicular to a given plane α. We denote the point of intersection of straight line a and plane α as H 1; by construction, it will serve as the base of a perpendicular dropped from point M 1 to plane α.
If a point M 2 is given, belonging to a given plane α, then M 2 will serve as a projection of itself onto the plane α.
Definition 3
- this is either the point itself (if it belongs to a given plane), or the base of a perpendicular dropped from a given point to a given plane.
Finding the coordinates of the projection of a point onto a plane, examples
Let the following be given in three-dimensional space: a rectangular coordinate system O x y z, a plane α, a point M 1 (x 1, y 1, z 1). It is necessary to find the coordinates of the projection of point M 1 onto a given plane.
The solution follows obviously from the definition given above of the projection of a point onto a plane.
Let us denote the projection of the point M 1 onto the plane α as H 1 . According to the definition, H 1 is the intersection point of a given plane α and a straight line a drawn through the point M 1 (perpendicular to the plane). Those. The coordinates of the projection of point M1 that we need are the coordinates of the point of intersection of straight line a and plane α.
Thus, to find the coordinates of the projection of a point onto a plane it is necessary:
Obtain the equation of the plane α (if it is not specified). An article about the types of plane equations will help you here;
Determine the equation of a line a passing through point M 1 and perpendicular to the plane α (study the topic about the equation of a line passing through a given point perpendicular to a given plane);
Find the coordinates of the point of intersection of the straight line a and the plane α (article - finding the coordinates of the point of intersection of the plane and the line). The data obtained will be the coordinates we need for the projection of point M 1 onto the plane α.
Let's look at the theory with practical examples.
Example 1
Determine the coordinates of the projection of point M 1 (- 2, 4, 4) onto the plane 2 x – 3 y + z - 2 = 0.
Solution
As we see, the equation of the plane is given to us, i.e. there is no need to compile it.
Let us write down the canonical equations of a straight line a passing through the point M 1 and perpendicular to the given plane. For these purposes, we determine the coordinates of the directing vector of the straight line a. Since line a is perpendicular to a given plane, the direction vector of line a is the normal vector of the plane 2 x - 3 y + z - 2 = 0. Thus, a → = (2, - 3, 1) – direction vector of straight line a.
Now let’s compose the canonical equations of a line in space passing through the point M 1 (- 2, 4, 4) and having a direction vector a → = (2 , - 3 , 1) :
x + 2 2 = y - 4 - 3 = z - 4 1
To find the required coordinates, the next step is to determine the coordinates of the intersection point of the straight line x + 2 2 = y - 4 - 3 = z - 4 1 and the plane 2 x - 3 y + z - 2 = 0 . For these purposes, we move from the canonical equations to the equations of two intersecting planes:
x + 2 2 = y - 4 - 3 = z - 4 1 ⇔ - 3 · (x + 2) = 2 · (y - 4) 1 · (x + 2) = 2 · (z - 4) 1 · ( y - 4) = - 3 (z + 4) ⇔ 3 x + 2 y - 2 = 0 x - 2 z + 10 = 0
Let's create a system of equations:
3 x + 2 y - 2 = 0 x - 2 z + 10 = 0 2 x - 3 y + z - 2 = 0 ⇔ 3 x + 2 y = 2 x - 2 z = - 10 2 x - 3 y + z = 2
And let's solve it using Cramer's method:
∆ = 3 2 0 1 0 - 2 2 - 3 1 = - 28 ∆ x = 2 2 0 - 10 0 - 2 2 - 3 1 = 0 ⇒ x = ∆ x ∆ = 0 - 28 = 0 ∆ y = 3 2 0 1 - 10 - 2 2 2 1 = - 28 ⇒ y = ∆ y ∆ = - 28 - 28 = 1 ∆ z = 3 2 2 1 0 - 10 2 - 3 2 = - 140 ⇒ z = ∆ z ∆ = - 140 - 28 = 5
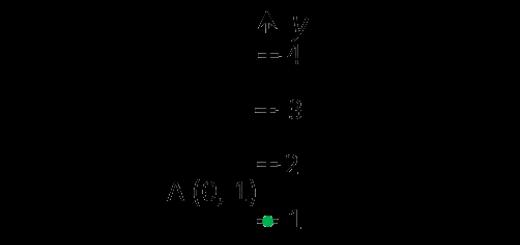
Thus, the required coordinates of a given point M 1 on a given plane α will be: (0, 1, 5).
Answer: (0 , 1 , 5) .
Example 2
In a rectangular coordinate system O x y z of three-dimensional space, points A (0, 0, 2) are given; B (2, - 1, 0); C (4, 1, 1) and M 1 (-1, -2, 5). It is necessary to find the coordinates of the projection M 1 onto the plane A B C
Solution
First of all, we write down the equation of a plane passing through three given points:
x - 0 y - 0 z - 0 2 - 0 - 1 - 0 0 - 2 4 - 0 1 - 0 1 - 2 = 0 ⇔ x y z - 2 2 - 1 - 2 4 1 - 1 = 0 ⇔ ⇔ 3 x - 6 y + 6 z - 12 = 0 ⇔ x - 2 y + 2 z - 4 = 0
Let us write down the parametric equations of the line a, which will pass through the point M 1 perpendicular to the plane A B C. The plane x – 2 y + 2 z – 4 = 0 has a normal vector with coordinates (1, - 2, 2), i.e. vector a → = (1, - 2, 2) – direction vector of straight line a.
Now, having the coordinates of the point of the line M 1 and the coordinates of the direction vector of this line, we write the parametric equations of the line in space:
Then we determine the coordinates of the intersection point of the plane x – 2 y + 2 z – 4 = 0 and the straight line
x = - 1 + λ y = - 2 - 2 λ z = 5 + 2 λ
To do this, we substitute into the equation of the plane:
x = - 1 + λ, y = - 2 - 2 λ, z = 5 + 2 λ
Now, using the parametric equations x = - 1 + λ y = - 2 - 2 · λ z = 5 + 2 · λ, we find the values of the variables x, y and z for λ = - 1: x = - 1 + (- 1) y = - 2 - 2 · (- 1) z = 5 + 2 · (- 1) ⇔ x = - 2 y = 0 z = 3
Thus, the projection of point M 1 onto the plane A B C will have coordinates (- 2, 0, 3).
Answer: (- 2 , 0 , 3) .
Let us separately dwell on the issue of finding the coordinates of the projection of a point onto coordinate planes and planes that are parallel to coordinate planes.
Let points M 1 (x 1, y 1, z 1) and coordinate planes O x y, O x z and O y z be given. The coordinates of the projection of this point onto these planes will be, respectively: (x 1, y 1, 0), (x 1, 0, z 1) and (0, y 1, z 1). Let us also consider planes parallel to the given coordinate planes:
C z + D = 0 ⇔ z = - D C , B y + D = 0 ⇔ y = - D B
And the projections of a given point M 1 onto these planes will be points with coordinates x 1, y 1, - D C, x 1, - D B, z 1 and - D A, y 1, z 1.
Let us demonstrate how this result was obtained.
As an example, let's define the projection of point M 1 (x 1, y 1, z 1) onto the plane A x + D = 0. The remaining cases are similar.
The given plane is parallel to the coordinate plane O y z and i → = (1, 0, 0) is its normal vector. The same vector serves as the direction vector of the line perpendicular to the O y z plane. Then the parametric equations of a straight line drawn through the point M 1 and perpendicular to a given plane will have the form:
x = x 1 + λ y = y 1 z = z 1
Let's find the coordinates of the intersection point of this line and the given plane. Let us first substitute the equalities into the equation A x + D = 0: x = x 1 + λ , y = y 1 , z = z 1 and get: A · (x 1 + λ) + D = 0 ⇒ λ = - D A - x 1
Then we calculate the required coordinates using the parametric equations of the straight line with λ = - D A - x 1:
x = x 1 + - D A - x 1 y = y 1 z = z 1 ⇔ x = - D A y = y 1 z = z 1
That is, the projection of point M 1 (x 1, y 1, z 1) onto the plane will be a point with coordinates - D A, y 1, z 1.
Example 2
It is necessary to determine the coordinates of the projection of point M 1 (- 6, 0, 1 2) onto the coordinate plane O x y and onto the plane 2 y - 3 = 0.
Solution
The coordinate plane O x y will correspond to the incomplete general equation of the plane z = 0. The projection of point M 1 onto the plane z = 0 will have coordinates (- 6, 0, 0).
The plane equation 2 y - 3 = 0 can be written as y = 3 2 2. Now just write down the coordinates of the projection of point M 1 (- 6, 0, 1 2) onto the plane y = 3 2 2:
6 , 3 2 2 , 1 2
Answer:(- 6 , 0 , 0) and - 6 , 3 2 2 , 1 2
If you notice an error in the text, please highlight it and press Ctrl+Enter
The position of a straight line in space is determined by the position of its two points. Therefore, to construct projections of a straight line, it is enough to construct projections of two points belonging to it and connect them to each other.
Depending on the position relative to the projection planes, straight lines of general and particular positions are distinguished.
Straight lines private situation parallel to one or two projection planes.
Straight level lines- straight lines, parallel to one projection plane and inclined to the other two. There are three types of such lines.
sch, called horizontal straight and denoted by the letter To(Fig. 3.1). Its segment is projected onto the plane sch without distortion. The angle between its horizontal projection To " and axis OH equal to the angle of inclination f 2 of the horizontal straight line to the plane n 2, and the angle between its projection To" and the axis OU - angle of inclination f 3 to the plane ts 3. All points on the same horizontal straight line have the same coordinate ъ
Straight line parallel to the plane n 2, called frontal straight and denoted by the letter / (Fig. 3.2). Its segment is projected without distortion onto the plane 7G 2 - The angles of inclination of the frontal line TO THE PLANE are projected onto the same plane in their true magnitude L(angle f[) and plane l 3 (angle f 3). All points on the same frontal straight line have the same coordinate u.
A straight line parallel to plane l 3 is called profile straight R(Fig. 3.3). Its segment is projected onto the plane l 3 without distortion. On this same
the plane is projected into the true value of the angles of inclination of the profile straight line TO THE 7Гі PLANE (angle (pi) and the plane l 2 (angle (p 2). All points of the profile straight line have the same coordinate X.
Projecting straight lines- straight lines, perpendicular to one projection plane and parallel to the other two.
A straight line perpendicular to the plane l is called horizontally projecting(Fig. 3.4). It is projected onto the l i plane in the form of a point, and its frontal and profile projections are parallel to the axis 01. A segment of a horizontally projecting straight line is projected without distortion onto the planes l 2 and lz. Therefore, the horizontally projecting line is both frontal and profile R straight line.
A straight line perpendicular to the plane l 2 is called frontally projecting(Fig. 3.5). It is projected onto the plane l 2 in the form of a point, and its horizontal and profile projections are parallel to the axis OU. A segment of a frontally projecting straight line is projected without distortion on the planes Li and l 3. The frontally projecting line is also horizontal To and profile R straight.
A straight line perpendicular to the plane l 3 is called profile projecting(Fig. 3.6). Its profile projection is a point, and its horizontal and frontal projections are
on i are parallel to the axis OH. A segment of such a straight line is projected into the true value on the plane A] and 712, therefore it is also horizontal AND, and frontal/straight.
A straight line that is not parallel to any of the main projection planes is called a straight line general position(Fig. 3.7). On surface P, P 2 and 7G 3 its segment is projected with distortion, since it is inclined towards them and the angles of inclination in the drawing are also distorted. Thus, from a drawing of a straight line in general position it is impossible to measure the length of its segment or the angles of inclination to the projection planes. To determine these quantities, additional constructions are required.
Direct projections.
Drawing reversibility
Drawing reversibility. By projecting onto one projection plane, an image is obtained that does not allow one to unambiguously determine the shape and dimensions of the depicted object. Projection A 1 (see Fig. 1.4.) does not determine the position of the point itself in space, since it is unknown how far it is removed from the projection plane P 1. In such cases we talk about irreversibility drawing , since it is impossible to reproduce the original using such a drawing. To eliminate uncertainty, images are supplemented with the necessary data. In practice, various methods are used to supplement a single-projection drawing.
CHAPTER 2
A straight line can be considered as the result of the intersection of two planes (Figure 2.1, 2.2.).
The straight line in space is limitless. The limited part of a line is called a segment.
Projecting a line comes down to constructing projections of two arbitrary points of it, since two points completely determine the position of the line in space. By lowering the perpendiculars from points A and B (Fig. 2.2.) to the intersection with the plane P 1, their horizontal projections A 1 and B 1 are determined. Segment A 1 B 1 – horizontal projection of straight line AB. A similar result is obtained by drawing perpendiculars to P 1 from arbitrary points of the line AB. The combination of these perpendiculars (projecting rays) forms a horizontally projecting plane a, which intersects with plane P 1 along straight line A 1 B 1 - the horizontal projection of straight AB. Based on the same considerations, a frontal projection A 2 B 2 of straight AB is obtained (Figure 2.2).
One projection of a straight line does not determine its position in space. Indeed, the segment A 1 B 1 (Fig. 2.1.) can be a projection of an arbitrary segment lying in the projecting plane a. The position of a line in space is uniquely determined by the combination of its two projections. Reconstructing from the horizontal point A 1 B 1 and frontal P 1 and P 2, we obtain two projecting planes a and b, intersecting along a single straight line AB.
The complex drawing (Figure 2.3) shows a straight line segment AB in general position, where A 1 B 1 is horizontal, A 2 B 2 is frontal and A 3 B 3 is a profile projection of the segment. To construct the third projection of the segment. To construct the third projection of a straight line segment using two data, you can use the same methods as for constructing the third projection of a point: projection (Fig. 2.4.), coordinate (Fig. 2.5.) and using a constant straight line of the drawing (Fig. 2.6.).
2.2. The position of the line relative to the projection plane.
In Fig. 1.5. depicts a parallelepiped with a cut off top and an arbitrary triangular pyramid. The edges of a parallelepiped and a pyramid occupy different positions in space relative to the projection planes. To build and read drawings, you need to be able to analyze the positions of a straight line. According to their position in space, straight lines are divided into private straight lines and general straight lines.
Direct private provisions can be projective and direct level.
Projecting lines are those that are perpendicular to one of the projection planes, i.e. parallel to two other planes P 1, is called a horizontally projecting straight line; its horizontal projection A 1 B 1 is a point, and its frontal and profile projections are straight lines parallel to the O z axis. Straight line CD (Fig. 2.7.) perpendicular to the projection plane P 2 is called the frontally projecting straight line; its frontal projection C 2 D 2 is a point, and its horizontal and profile projections are straight lines parallel to the Oy axis. The straight line MN (Fig. 2.8.) perpendicular to the projection plane P 3 is called a profile projecting straight line; its profile projection M 3 N 3 is a point, and its horizontal and frontal projections are straight lines parallel to the Ox axis.
Consequently, on one of the projection planes the projecting straight line is depicted as a point, and on the other two – in the form of segments occupying a horizontal or vertical position, the magnitude of which is horizontal or vertical, the magnitude of which is equal to the natural value of the straight segment itself.
Level lines are lines parallel to one of the projection planes. Straight AB (Fig. 2.9.), parallel to the horizontal plane of projections P 1, is called a horizontal straight line, or, in short, a horizontal. Its frontal projection A 2 B 2 is parallel to the axis of projections Ox, and the horizontal projection A 1 B 1 is equal to the natural value of the straight line segment (A 1 B 1 = AB). The angle b between the horizontal projection A 1 B 1 and the Ox axis is equal to the natural value of the angle of inclination of the straight line AB to the projection plane P 2.
The straight line CD (Fig. 2.10.) parallel to the frontal plane of projections P 2 is called the frontal straight line, or, in short, the frontal. Its horizontal projection C 1 D 1 is parallel to the Ox axis, and its frontal projection C 2 D 2 is equal to the natural value of the straight line segment (C 2 D 2 = CD). The angle a between the frontal projection C 2 D 2 and the Ox axis is equal to the actual angle of inclination of the straight line to the projection plane P 1.
The straight line MN (Fig. 2.11.) parallel to the profile plane of projections P 3 is called the profile straight line. Its frontal M 2 N 2 and horizontal M 1 N 1 projections are perpendicular to the Ox axis, and the profile projection is equal to the natural size of the segment (M 3 N 3 = MN). Angles a and b between the profile projection and the Oy 3 and Oz axes are equal to the actual value of the angles of inclination of the straight line to the plane of the projections P 1 and P 2.
Consequently, the straight lines of the level are projected onto one of the projection planes in natural size, and onto the other two - in the form of segments of reduced size, occupying a vertical or horizontal position in the drawing. From the drawing, you can determine the angles of inclination of these straight lines to the projection planes.
If a straight line lies in the projection plane, then one of its projections (of the same name) coincides with the straight line itself, and the other two coincide with the axes of the projections. For example, straight line AB (Fig. 2.12) lies in the plane P 1. Its horizontal projection A 1 B 1 merges with the straight line AB, and the frontal projection A 2 B 2 merges with the Ox axis. Such a straight line is called the zero horizontal line, since the height of its points (z coordinate) is zero.
Direct line in general position called a straight line inclined to all projection planes. Its projections form acute or obtuse angles with the Ox, Oy and Oz axes, i.e. none of its projections are parallel or perpendicular to the axes. The size of the projections of a line in general position is always less than the natural size of the segment itself. Directly from the drawing, without additional constructions, it is impossible to determine the actual size of the straight line and its angle of inclination to the projection planes.
If a point lies on a line, then the projections of the point are on the same projections of the line and on a common connection line.
In Fig. 2.13. point C lies on straight line AB, since its projections C 1 and C 2 are respectively on the horizontal A 1 B 1 and on the frontal A 2 B 2 projections of the straight line. Points M and N do not belong to the line, since one of the projections of each point is not on the projection of the line of the same name.
The projections of a point divide the projections of a line in the same ratio in which the point itself divides a segment of a line, i.e. Using this rule, divide a given straight line segment in the required ratio. For example, in Fig. 2.14. straight line EF is divided by point K in a ratio of 3:5. The division is made in a manner known from geometric drawing.
The position of a straight line in space is completely determined by any two of its points. In general, the projection of a line is a straight line; in a particular case, it is a point if the line is perpendicular to the projection plane. To construct projections of a line, it is enough to have either the projections of two of its points, or the projection of one point of the line and the direction of the line in space.
According to their location in space relative to the projection planes, straight lines are divided into straight lines of general position, level and projecting .
2.2.1. General lines. These are straight lines, neither parallel nor perpendicular to the projection planes. Projections A 1 B 1, A 2 B 2 And A 3 B 3 segment AB straight AB general position (Fig. 2.18, A) inclined at acute angles to the axes x 12 , y 13 And z 23. The lengths of the projections of segments of this line are always less than the segment itself. Three-picture complex drawing of a line segment in general position, constructed from two points A And IN, shown in Fig. 2.18, b.
2.2.2. Straight levels. These are straight lines parallel to one of the projection planes - P 1,P 2 or P 3. Consequently, we have three types of level lines:
1) horizontal level a (horizontal ), parallel P 1(straight a with a segment AB on it in Fig. 2.19, A, b);
2) front level (frontal ), parallel P 2(straight b with segment CD on it in Fig. 2.20, A);
3) profile level , parallel P 3(straight With with a segment EF on it in Fig. 2.20, b). In Fig. 2.20 visual images of straight lines b And c relative to projection planes are not shown.
Projections of the same name straight line segments of the level are projected in full size, and opposite ones are parallel to the axes separating them from the same ones. Moreover, for the horizontal, the projection of the same name is horizontal, and those of different names are frontal and profile, etc.
Angles of inclination of straight lines a,b And c to projection planes P 1, P 2 And P 3 it is customary to designate accordingly α , β And γ (in Fig. 2.19 the corners α , β And γ not shown).
2.2.3. Projecting straight lines. These are straight lines perpendicular to one of the projection planes and parallel to the other two. Consequently, we have three types of projecting lines:
1) horizontally projecting straight, perpendicular P 1(straight A with a segment AB on it in Fig. 2.21, A);
2) front-projection straight, perpendicular P 2(straight b with a segment CD on it in Fig. 2.21, b);
3)profile-projecting straight, perpendicular P 3(straight c with a segment E.F. on it in Fig. 2.21, V).
In Fig. 2.21 the projections of invisible points are enclosed in brackets. The issue of determining the visibility of points on projections will be discussed in more detail below in the paragraph “Crossing lines”.
For projecting lines, the projections of the same name are points, which follows from the essence of the projecting line along which the projection is carried out.
Each unlike projection of a projecting straight line is perpendicular to the axis separating it from the projection of the same name, and the unlike projection of a segment located on the level line is the natural size of this segment.
2.2.4. Determination of the natural value of a line segment in general position. The actual size of a straight line of a particular position can be immediately determined on a complex drawing of this straight line.
To determine the natural value of a straight line segment in general position, you can apply the previously discussed (see section 2.1.2) method of replacing projection planes
. Figure 2.22 shows the definition of natural size ( N.V..) segment AB a straight line in general position and determining the angles of its inclination to Π 1(corner α
) and to Π 2(corner β
) in this manner.
Additional plane Π 4 carried out in parallel AB (x 14 ||A 1 B 1). Straight AB converted to frontal position, therefore A 4 B 4– natural size AB.
By drawing an additional plane Π 5 ||AB(x 25 ||A 2 B 2), you can also determine the actual size AB. A 5 B 5– natural size AB. Straight AB in system Π 2-Π 5 became horizontal.
Figure 2.23 shows the definition of natural size AB using the triangle method.
The natural value of a segment is equal to the hypotenuse of a right triangle, one leg of which is one of the projections of the segment, and the other is the algebraic difference in the distances of its ends from the plane Π 1(ΔZ).
2.2.5. Mutual position of lines. Straight lines in space can be parallel, intersect and cross.
Parallel lines. From the properties of parallel projections it follows that if lines in space are parallel, then all three pairs of their projections of the same name are parallel. The reverse situation is also obvious: if the projections of lines of the same name are parallel, then the lines in space are parallel.
To determine the parallelism of lines, in the general case, the parallelism of two pairs of projections of the same name is sufficient. If the parallelism of the level lines is determined, then one of the two pairs of parallel projections must be a projection onto the plane of the same name.
In Fig. 2.24 shows projections of parallel lines a And b general position, where a 1 ║ b 1 And a 2 ║ b 2. In Fig. 2.25 shows two horizontal lines c And d. For horizontals, frontal and profile projections are always parallel to the axes that separate them from the horizontal projections of the same name, i.e. c 2║d 2║x 12 And c 3║d 3║y 3. But their horizontal projections are not parallel, i.e. c 1╫d 1. Therefore, straight c And d not parallel.
Intersecting lines. Two intersecting lines lie in the same plane and have one common point. From the properties of parallel projections it is known that if a point lies on a line, then its projections lie on the projections of the line. If a point lies on both lines, that is, at the point of intersection of the lines, then its projection must lie on two projections of the same lines at once, and therefore at the point of intersection of the projections of the lines.
So, if the segments AB And CD two lines intersect at a point K, then the projections of the segments A 1 B 1 And C 1 D 1 intersect at a point K 1, which is the projection of the point K(Fig. 2.26, A). That's why, if projections of lines of the same name intersect at points lying on the same line of projection connection, then the lines intersect in space (Fig. 2.26, b).
To determine whether the lines intersect or not, it is enough that this condition is met for any two projections. The exception is the case when one of the intersecting lines is a profile level. In this case, to check the intersection of lines, it is necessary to construct a profile projection.
Let through the point A it is necessary to draw a horizontal line b, intersecting the line a(Fig. 2.27, A). To do this, through the point A 2 carry out b 2 ║ x 12(stage 1) until the intersection with a 2 at the point K2(Fig.2.27, b). Next, using the projection communication line on a 1 find the point K 1(step 2) and connecting the dots A 1 And K 1(stage 3), we get b 1.
Crossing straight lines.
Crossing lines a And b do not lie in the same plane and, therefore, are not parallel and do not have common points (Fig. 2.28, A). Therefore, if the lines are crossing, then at least one pair of their projections of the same name is not parallel, and the points of intersection of the projections of the same name do not lie on the same line of projection connection (Fig. 2.28, b).
Each such intersection point is a projection of two points belonging to straight lines; these two points lie on the same projecting ray and are called competing .
