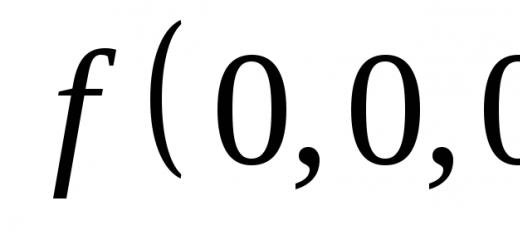Function is one of the most important mathematical concepts. Function - variable dependency at from variable x, if each value X matches a single value at. Variable X called the independent variable or argument. Variable at called the dependent variable. All values of the independent variable (variable x) form the domain of definition of the function. All values that the dependent variable takes (variable y), form the range of values of the function.
Function graph call the set of all points of the coordinate plane, the abscissas of which are equal to the values of the argument, and the ordinates are equal to the corresponding values of the function, that is, the values of the variable are plotted along the abscissa axis x, and the values of the variable are plotted along the ordinate axis y. To graph a function, you need to know the properties of the function. The main properties of the function will be discussed below!
To build a graph of a function, we recommend using our program - Graphing functions online. If you have any questions while studying the material on this page, you can always ask them on our forum. Also on the forum they will help you solve problems in mathematics, chemistry, geometry, probability theory and many other subjects!
Basic properties of functions.
1) Function domain and function range.
The domain of a function is the set of all valid valid argument values x(variable x), for which the function y = f(x) determined.
The range of a function is the set of all real values y, which the function accepts.
In elementary mathematics, functions are studied only on the set of real numbers.
2) Function zeros.
Values X, at which y=0, called function zeros. These are the abscissas of the points of intersection of the function graph with the Ox axis.
3) Intervals of constant sign of a function.
Intervals of constant sign of a function are such intervals of values x, on which the function values y either only positive or only negative are called intervals of constant sign of the function.

4) Monotonicity of the function.
An increasing function (in a certain interval) is a function in which a larger value of the argument from this interval corresponds to a larger value of the function.
A decreasing function (in a certain interval) is a function in which a larger value of the argument from this interval corresponds to a smaller value of the function.
5) Even (odd) function.
An even function is a function whose domain of definition is symmetrical with respect to the origin and for any X f(-x) = f(x). The graph of an even function is symmetrical about the ordinate.
An odd function is a function whose domain of definition is symmetrical with respect to the origin and for any X from the domain of definition the equality is true f(-x) = - f(x). The graph of an odd function is symmetrical about the origin.
Even function
1) The domain of definition is symmetrical with respect to the point (0; 0), that is, if the point a belongs to the domain of definition, then the point -a also belongs to the domain of definition.
2) For any value x f(-x)=f(x)
3) The graph of an even function is symmetrical about the Oy axis.
Odd function has the following properties:
1) The domain of definition is symmetrical about the point (0; 0).
2) for any value x, belonging to the domain of definition, the equality f(-x)=-f(x)
3) The graph of an odd function is symmetrical with respect to the origin (0; 0).
Not every function is even or odd. Functions general view are neither even nor odd.



6) Limited and unlimited functions.
A function is called bounded if there is a positive number M such that |f(x)| ≤ M for all values of x. If such a number does not exist, then the function is unlimited.
7) Periodicity of the function.
A function f(x) is periodic if there is a non-zero number T such that for any x from the domain of definition of the function the following holds: f(x+T) = f(x). This smallest number is called the period of the function. All trigonometric functions are periodic. (Trigonometric formulas).
Function f is called periodic if there is a number such that for any x from the domain of definition the equality f(x)=f(x-T)=f(x+T). T is the period of the function.
Every periodic function has an infinite number of periods. In practice, the smallest positive period is usually considered.
The values of a periodic function are repeated after an interval equal to the period. This is used when constructing graphs.

Back forward
Attention! Slide previews are for informational purposes only and may not represent all the features of the presentation. If you are interested in this work, please download the full version.
Goals:
- formulate the concept of even and odd functions, teach the ability to determine and use these properties when studying functions and constructing graphs;
- develop students’ creative activity, logical thinking, ability to compare and generalize;
- cultivate hard work and mathematical culture; develop communication skills .
Equipment: multimedia installation, interactive whiteboard, handouts.
Forms of work: frontal and group with elements of search and research activities.
Information sources:
1. Algebra 9th class A.G. Mordkovich. Textbook.
2. Algebra 9th grade A.G. Mordkovich. Problem book.
3. Algebra 9th grade. Tasks for student learning and development. Belenkova E.Yu. Lebedintseva E.A.
DURING THE CLASSES
1. Organizational moment
Setting goals and objectives for the lesson.
2. Checking homework
No. 10.17 (9th grade problem book. A.G. Mordkovich).
A) at = f(X), f(X) = 
b) f (–2) = –3; f (0) = –1; f(5) = 69;
c) 1. D( f) = [– 2; + ∞)
2. E( f) = [– 3; + ∞)
3. f(X) = 0 at X ~ 0,4
4. f(X) >0 at X > 0,4 ; f(X)
< 0 при – 2 <
X <
0,4.
5. The function increases with X € [– 2; + ∞)
6. The function is limited from below.
7. at naim = – 3, at naib doesn't exist
8. The function is continuous.
(Have you used a function exploration algorithm?) Slide.
2. Let’s check the table you were asked from the slide.
| Fill the table | |||||
Domain |
Function zeros |
Intervals of sign constancy |
Coordinates of the points of intersection of the graph with Oy | ||
x = –5, |
x € (–5;3) U |
x € (–∞;–5) U |
|||
x ∞ –5, |
x € (–5;3) U |
x € (–∞;–5) U |
|||
x ≠ –5, |
x € (–∞; –5) U |
x € (–5; 2) |
|||
3. Updating knowledge
– Functions are given.
– Specify the scope of definition for each function.
– Compare the value of each function for each pair of argument values: 1 and – 1; 2 and – 2.
– For which of these functions in the domain of definition the equalities hold f(– X)
= f(X), f(– X) = – f(X)? (enter the obtained data into the table) Slide
| f(1) and f(– 1) | f(2) and f(– 2) | graphics | f(– X) = –f(X) | f(– X) = f(X) | ||
| 1. f(X) = | ||||||
| 2. f(X) = X 3 | ||||||
| 3. f(X) = | X | | ||||||
| 4.f(X) = 2X – 3 | ||||||
| 5. f(X) = | X ≠ 0 |
|||||
| 6. f(X)= | X > –1 | and not defined |
4. New material
– While doing this work, guys, we identified another property of the function, unfamiliar to you, but no less important than the others - this is the evenness and oddness of the function. Write down the topic of the lesson: “Even and odd functions”, our task is to learn to determine the evenness and oddness of a function, to find out the significance of this property in the study of functions and plotting graphs.
So, let's find the definitions in the textbook and read (p. 110) . Slide
Def. 1 Function at = f (X), defined on the set X is called even, if for any value XЄ X is executed equality f(–x)= f(x). Give examples.
Def. 2 Function y = f(x), defined on the set X is called odd, if for any value XЄ X the equality f(–х)= –f(х) holds. Give examples.
Where did we meet the terms “even” and “odd”?
Which of these functions will be even, do you think? Why? Which ones are odd? Why?
For any function of the form at= x n, Where n– an integer, it can be argued that the function is odd when n– odd and the function is even when n– even.
– View functions at= and at = 2X– 3 are neither even nor odd, because equalities are not satisfied f(– X) = – f(X), f(–
X) = f(X)
The study of whether a function is even or odd is called the study of a function's parity. Slide
In definitions 1 and 2 we were talking about the values of the function at x and – x, thereby it is assumed that the function is also defined at the value X, and at – X.
Def 3. If a numerical set, together with each of its elements x, also contains the opposite element –x, then the set X called a symmetric set.
Examples:
(–2;2), [–5;5]; (∞;∞) are symmetric sets, and , [–5;4] are asymmetric.
– Do even functions have a domain of definition that is a symmetric set? The odd ones?
– If D( f) is an asymmetric set, then what is the function?
– Thus, if the function at = f(X) – even or odd, then its domain of definition is D( f) is a symmetric set. Is the converse statement true: if the domain of definition of a function is a symmetric set, then is it even or odd?
– This means that the presence of a symmetric set of the domain of definition is a necessary condition, but not sufficient.
– So how do you examine a function for parity? Let's try to create an algorithm.
Slide
Algorithm for studying a function for parity
1. Determine whether the domain of definition of the function is symmetrical. If not, then the function is neither even nor odd. If yes, then go to step 2 of the algorithm.
2. Write an expression for f(–X).
3. Compare f(–X).And f(X):
- If f(–X).= f(X), then the function is even;
- If f(–X).= – f(X), then the function is odd;
- If f(–X) ≠ f(X) And f(–X) ≠ –f(X), then the function is neither even nor odd.
Examples:
Examine function a) for parity at= x 5 +; b) at= ; V) at= .
Solution.
a) h(x) = x 5 +,
1) D(h) = (–∞; 0) U (0; +∞), symmetric set.
2) h (– x) = (–x) 5 + – x5 –= – (x 5 +),
3) h(– x) = – h (x) => function h(x)= x 5 + odd.
b) y =,
at = f(X), D(f) = (–∞; –9)? (–9; +∞), an asymmetric set, which means the function is neither even nor odd.
V) f(X) = , y = f (x),
1) D( f) = (–∞; 3] ≠ ; b) (∞; –2), (–4; 4]?
Option 2
1. Is the given set symmetric: a) [–2;2]; b) (∞; 0], (0; 7) ?
A); b) y = x (5 – x 2).
a) y = x 2 (2x – x 3), b) y =
Graph the Function at = f(X), If at = f(X) is an even function.

Graph the Function at = f(X), If at = f(X) is an odd function.

Mutual check on slide.
6. Homework: №11.11, 11.21,11.22;
Proof of the geometric meaning of the parity property.
***(Assignment of the Unified State Examination option).
1. The odd function y = f(x) is defined on the entire number line. For any non-negative value of the variable x, the value of this function coincides with the value of the function g( X) = X(X + 1)(X + 3)(X– 7). Find the value of the function h( X) = at X = 3.
7. Summing up
Back forward
Attention! Slide previews are for informational purposes only and may not represent all the features of the presentation. If you are interested in this work, please download the full version.
Goals:
- formulate the concept of even and odd functions, teach the ability to determine and use these properties when studying functions and constructing graphs;
- develop students’ creative activity, logical thinking, ability to compare and generalize;
- cultivate hard work and mathematical culture; develop communication skills .
Equipment: multimedia installation, interactive whiteboard, handouts.
Forms of work: frontal and group with elements of search and research activities.
Information sources:
1. Algebra 9th class A.G. Mordkovich. Textbook.
2. Algebra 9th grade A.G. Mordkovich. Problem book.
3. Algebra 9th grade. Tasks for student learning and development. Belenkova E.Yu. Lebedintseva E.A.
DURING THE CLASSES
1. Organizational moment
Setting goals and objectives for the lesson.
2. Checking homework
No. 10.17 (9th grade problem book. A.G. Mordkovich).
A) at = f(X), f(X) = 
b) f (–2) = –3; f (0) = –1; f(5) = 69;
c) 1. D( f) = [– 2; + ∞)
2. E( f) = [– 3; + ∞)
3. f(X) = 0 at X ~ 0,4
4. f(X) >0 at X > 0,4 ; f(X)
< 0 при – 2 <
X <
0,4.
5. The function increases with X € [– 2; + ∞)
6. The function is limited from below.
7. at naim = – 3, at naib doesn't exist
8. The function is continuous.
(Have you used a function exploration algorithm?) Slide.
2. Let’s check the table you were asked from the slide.
| Fill the table | |||||
Domain |
Function zeros |
Intervals of sign constancy |
Coordinates of the points of intersection of the graph with Oy | ||
x = –5, |
x € (–5;3) U |
x € (–∞;–5) U |
|||
x ∞ –5, |
x € (–5;3) U |
x € (–∞;–5) U |
|||
x ≠ –5, |
x € (–∞; –5) U |
x € (–5; 2) |
|||
3. Updating knowledge
– Functions are given.
– Specify the scope of definition for each function.
– Compare the value of each function for each pair of argument values: 1 and – 1; 2 and – 2.
– For which of these functions in the domain of definition the equalities hold f(– X)
= f(X), f(– X) = – f(X)? (enter the obtained data into the table) Slide
| f(1) and f(– 1) | f(2) and f(– 2) | graphics | f(– X) = –f(X) | f(– X) = f(X) | ||
| 1. f(X) = | ||||||
| 2. f(X) = X 3 | ||||||
| 3. f(X) = | X | | ||||||
| 4.f(X) = 2X – 3 | ||||||
| 5. f(X) = | X ≠ 0 |
|||||
| 6. f(X)= | X > –1 | and not defined |
4. New material
– While doing this work, guys, we identified another property of the function, unfamiliar to you, but no less important than the others - this is the evenness and oddness of the function. Write down the topic of the lesson: “Even and odd functions”, our task is to learn to determine the evenness and oddness of a function, to find out the significance of this property in the study of functions and plotting graphs.
So, let's find the definitions in the textbook and read (p. 110) . Slide
Def. 1 Function at = f (X), defined on the set X is called even, if for any value XЄ X is executed equality f(–x)= f(x). Give examples.
Def. 2 Function y = f(x), defined on the set X is called odd, if for any value XЄ X the equality f(–х)= –f(х) holds. Give examples.
Where did we meet the terms “even” and “odd”?
Which of these functions will be even, do you think? Why? Which ones are odd? Why?
For any function of the form at= x n, Where n– an integer, it can be argued that the function is odd when n– odd and the function is even when n– even.
– View functions at= and at = 2X– 3 are neither even nor odd, because equalities are not satisfied f(– X) = – f(X), f(–
X) = f(X)
The study of whether a function is even or odd is called the study of a function's parity. Slide
In definitions 1 and 2 we were talking about the values of the function at x and – x, thereby it is assumed that the function is also defined at the value X, and at – X.
Def 3. If a numerical set, together with each of its elements x, also contains the opposite element –x, then the set X called a symmetric set.
Examples:
(–2;2), [–5;5]; (∞;∞) are symmetric sets, and , [–5;4] are asymmetric.
– Do even functions have a domain of definition that is a symmetric set? The odd ones?
– If D( f) is an asymmetric set, then what is the function?
– Thus, if the function at = f(X) – even or odd, then its domain of definition is D( f) is a symmetric set. Is the converse statement true: if the domain of definition of a function is a symmetric set, then is it even or odd?
– This means that the presence of a symmetric set of the domain of definition is a necessary condition, but not sufficient.
– So how do you examine a function for parity? Let's try to create an algorithm.
Slide
Algorithm for studying a function for parity
1. Determine whether the domain of definition of the function is symmetrical. If not, then the function is neither even nor odd. If yes, then go to step 2 of the algorithm.
2. Write an expression for f(–X).
3. Compare f(–X).And f(X):
- If f(–X).= f(X), then the function is even;
- If f(–X).= – f(X), then the function is odd;
- If f(–X) ≠ f(X) And f(–X) ≠ –f(X), then the function is neither even nor odd.
Examples:
Examine function a) for parity at= x 5 +; b) at= ; V) at= .
Solution.
a) h(x) = x 5 +,
1) D(h) = (–∞; 0) U (0; +∞), symmetric set.
2) h (– x) = (–x) 5 + – x5 –= – (x 5 +),
3) h(– x) = – h (x) => function h(x)= x 5 + odd.
b) y =,
at = f(X), D(f) = (–∞; –9)? (–9; +∞), an asymmetric set, which means the function is neither even nor odd.
V) f(X) = , y = f (x),
1) D( f) = (–∞; 3] ≠ ; b) (∞; –2), (–4; 4]?
Option 2
1. Is the given set symmetric: a) [–2;2]; b) (∞; 0], (0; 7) ?
A); b) y = x (5 – x 2).
a) y = x 2 (2x – x 3), b) y =
Graph the Function at = f(X), If at = f(X) is an even function.

Graph the Function at = f(X), If at = f(X) is an odd function.

Mutual check on slide.
6. Homework: №11.11, 11.21,11.22;
Proof of the geometric meaning of the parity property.
***(Assignment of the Unified State Examination option).
1. The odd function y = f(x) is defined on the entire number line. For any non-negative value of the variable x, the value of this function coincides with the value of the function g( X) = X(X + 1)(X + 3)(X– 7). Find the value of the function h( X) = at X = 3.
7. Summing up
Even function.
Even is a function whose sign does not change when the sign changes x.
x equality holds f(–x) = f(x). Sign x does not affect the sign y.
The graph of an even function is symmetrical about the coordinate axis (Fig. 1).
Examples of an even function:
y=cos x
y = x 2
y = –x 2
y = x 4
y = x 6
y = x 2 + x
Explanation:
Let's take the function y = x 2 or y = –x 2 .
For any value x the function is positive. Sign x does not affect the sign y. The graph is symmetrical about the coordinate axis. This is an even function.
Odd function.
Odd is a function whose sign changes when the sign changes x.
In other words, for any value x equality holds f(–x) = –f(x).
The graph of an odd function is symmetrical with respect to the origin (Fig. 2).
Examples of odd function:
y= sin x
y = x 3
y = –x 3
Explanation:
Let's take the function y = – x 3 .
All meanings at it will have a minus sign. That is a sign x influences the sign y. If the independent variable is a positive number, then the function is positive, if the independent variable is a negative number, then the function is negative: f(–x) = –f(x).
The graph of the function is symmetrical about the origin. This is an odd function.
Properties of even and odd functions:
NOTE:
Not all functions are even or odd. There are functions that do not obey such gradation. For example, the root function at = √X does not apply to either even or odd functions (Fig. 3). When listing the properties of such functions, an appropriate description should be given: neither even nor odd.
Periodic functions.
As you know, periodicity is the repetition of certain processes at a certain interval. The functions that describe these processes are called periodic functions. That is, these are functions in whose graphs there are elements that repeat at certain numerical intervals.
Function study.
1) D(y) – Definition domain: the set of all those values of the variable x. for which the algebraic expressions f(x) and g(x) make sense.
If a function is given by a formula, then the domain of definition consists of all values of the independent variable for which the formula makes sense.
2) Properties of the function: even/odd, periodicity:
Odd And even functions are called whose graphs are symmetric with respect to changes in the sign of the argument.
Odd function- a function that changes the value to the opposite when the sign of the independent variable changes (symmetrical relative to the center of coordinates).
Even function- a function that does not change its value when the sign of the independent variable changes (symmetrical about the ordinate).
Neither even nor odd function (general function)- a function that does not have symmetry. This category includes functions that do not fall under the previous 2 categories.
Functions that do not belong to any of the categories above are called neither even nor odd(or general functions).
Odd functions
Odd power where is an arbitrary integer.
Even functions
Even power where is an arbitrary integer.
Periodic function- a function that repeats its values at some regular argument interval, that is, it does not change its value when adding some fixed non-zero number to the argument ( period functions) over the entire domain of definition.
3) Zeros (roots) of a function are the points where it becomes zero.
Finding the intersection point of the graph with the axis Oy. To do this you need to calculate the value f(0). Find also the points of intersection of the graph with the axis Ox, why find the roots of the equation f(x) = 0 (or make sure there are no roots).
The points at which the graph intersects the axis are called function zeros. To find the zeros of a function you need to solve the equation, that is, find those meanings of "x", at which the function becomes zero.
4) Intervals of constancy of signs, signs in them.
Intervals where the function f(x) maintains sign.
The interval of constancy of sign is the interval at every point of which the function is positive or negative.
ABOVE the x-axis.
BELOW the axle.
5) Continuity (points of discontinuity, nature of the discontinuity, asymptotes).
Continuous function- a function without “jumps”, that is, one in which small changes in the argument lead to small changes in the value of the function.
Removable Break Points
If the limit of the function exists, but the function is not defined at this point, or the limit does not coincide with the value of the function at this point:
![]() ,
,
then the point is called removable break point functions (in complex analysis, a removable singular point).
If we “correct” the function at the point of removable discontinuity and put ![]() , then we get a function that is continuous at a given point. This operation on a function is called extending the function to continuous or redefinition of the function by continuity, which justifies the name of the point as a point removable rupture.
, then we get a function that is continuous at a given point. This operation on a function is called extending the function to continuous or redefinition of the function by continuity, which justifies the name of the point as a point removable rupture.
Discontinuity points of the first and second kind
If a function has a discontinuity at a given point (that is, the limit of the function at a given point is absent or does not coincide with the value of the function at a given point), then for numerical functions there are two possible options associated with the existence of numerical functions unilateral limits:
if both one-sided limits exist and are finite, then such a point is called discontinuity point of the first kind. Removable discontinuity points are discontinuity points of the first kind;
if at least one of the one-sided limits does not exist or is not a finite value, then such a point is called point of discontinuity of the second kind.
Asymptote - straight, which has the property that the distance from a point on the curve to this straight tends to zero as the point moves away along the branch to infinity.
Vertical
Vertical asymptote - limit line ![]() .
.
As a rule, when determining the vertical asymptote, they look for not one limit, but two one-sided ones (left and right). This is done in order to determine how the function behaves as it approaches the vertical asymptote from different directions. For example:
Horizontal
Horizontal asymptote - straight species, subject to the existence limit
![]() .
.
Inclined
Oblique asymptote - straight species, subject to the existence limits
Note: a function can have no more than two oblique (horizontal) asymptotes.
Note: if at least one of the two limits mentioned above does not exist (or is equal to ), then the oblique asymptote at (or ) does not exist.
if in item 2.), then , and the limit is found using the horizontal asymptote formula, ![]() .
.
6) Finding intervals of monotonicity. Find intervals of monotonicity of a function f(x)(that is, intervals of increasing and decreasing). This is done by examining the sign of the derivative f(x). To do this, find the derivative f(x) and solve the inequality f(x)0. On intervals where this inequality holds, the function f(x)increases. Where the reverse inequality holds f(x)0, function f(x) is decreasing.
Finding a local extremum. Having found the intervals of monotonicity, we can immediately determine the local extremum points where an increase is replaced by a decrease, local maxima are located, and where a decrease is replaced by an increase, local minima are located. Calculate the value of the function at these points. If a function has critical points that are not local extremum points, then it is useful to calculate the value of the function at these points as well.
Finding the largest and smallest values of the function y = f(x) on a segment(continuation)
|
1. Find the derivative of the function: f(x). 2. Find the points at which the derivative is zero: f(x)=0x 1, x 2 ,... 3. Determine the affiliation of points X 1 ,X 2 , … segment [ a; b]: let x 1a;b, A x 2a;b . 4. Find the values of the function at selected points and at the ends of the segment: f(x 1), f(x 2),..., f(x a),f(x b), 5. Selecting the largest and smallest function values from those found. Comment. If on the segment [ a; b] there are discontinuity points, then it is necessary to calculate one-sided limits at them, and then take their values into account in choosing the largest and smallest values of the function. |
7) Finding intervals of convexity and concavity. This is done by examining the sign of the second derivative f(x). Find inflection points at the junctions of the convex and concave intervals. Calculate the value of the function at the inflection points. If a function has other points of continuity (except for inflection points) at which the second derivative is 0 or does not exist, then it is also useful to calculate the value of the function at these points. Having found f(x), we solve the inequality f(x)0. On each of the solution intervals the function will be convex downward. Solving the inverse inequality f(x)0, we find the intervals at which the function is convex upward (that is, concave). We define inflection points as those points at which the function changes direction of convexity (and is continuous).
Inflection point of a function- this is the point at which the function is continuous and when passing through which the function changes the direction of convexity.
Conditions of existence
A necessary condition for the existence of an inflection point: if the function is twice differentiable in some punctured neighborhood of the point , then or ![]() .
.