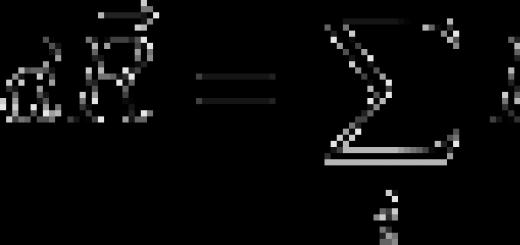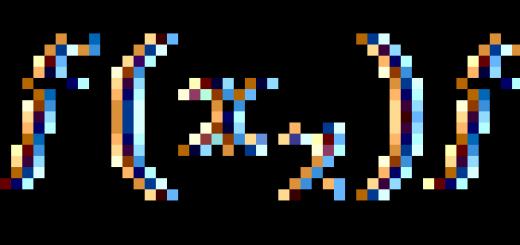Task 10 (OGE - 2015)
Points A and B are marked on a circle centered at O so that ∠ AOB = 18°. The length of the smaller arc AB is 5. Find the length of the larger arc of the circle.
Decision
∠AOB = 18°. The whole circle is 360°. So ∠AOB is 18/360 = 1/20 of the circle.
This means that the smaller arc AB is 1/20 of the entire circle, so the larger arc is the rest, i.e. 19/20 circumference.
1/20 of the circle corresponds to an arc length of 5. Then the length of the larger arc is 5*19 = 95.
Task 10 (OGE - 2015)
Points A and B are marked on a circle centered at O so that ∠ AOB = 40°. The length of the smaller arc AB is 50. Find the length of the larger arc of the circle.
Decision
∠AOB = 40°. The whole circle is 360°. So ∠AOB is 40/360 = 1/9 of the circle.
This means that the smaller arc AB is 1/9 of the entire circle, so the larger arc is the rest, i.e. 8/9 circle.
1/9 of the circle corresponds to an arc length of 50. Then the length of the larger arc is 50 * 8 = 400.
Answer: 400.
Task 10 (GIA - 2014)
The chord length of the circle is 72, and the distance from the center of the circle to this chord is 27. Find the diameter of the circle.

Decision

By the Pythagorean theorem, from a right triangle AOB we get:
AO 2 \u003d OB 2 + AB 2,
AO 2 \u003d 27 2 +36 2 \u003d 729 + 1296 \u003d 2025,
Then the diameter is 2R = 2*45 = 90.
Task 10 (GIA - 2014)
Point O is the center of the circle on which points A, B and C lie. It is known that ∠ABC = 134° and ∠OAB = 75°. Find the angle BCO. Give your answer in degrees.
The circle, its parts, their sizes and ratios are things that the jeweler constantly encounters. Rings, bracelets, castes, tubes, balls, spirals - a lot of round things have to be done. How can you calculate all this, especially if you were lucky enough to skip geometry lessons at school? ..
Let's first look at what the circle has parts and what they are called.
- A circle is a line that encloses a circle.
- An arc is part of a circle.
- A radius is a line segment that connects the center of a circle to a point on the circle.
- A chord is a line segment that connects two points on a circle.
- A segment is a part of a circle bounded by a chord and an arc.
- A sector is a part of a circle bounded by two radii and an arc.
The quantities of interest to us and their designations:

Now let's see what tasks related to the parts of the circle have to be solved.
- Find the length of the development of any part of the ring (bracelet). Given the diameter and chord (option: diameter and central angle), find the length of the arc.
- There is a drawing on the plane, you need to find out its size in projection after bending into an arc. Given the length of the arc and the diameter, find the length of the chord.
- Find out the height of a part obtained by bending a flat workpiece into an arc. Initial data options: arc length and diameter, arc length and chord; find the height of the segment.
Life will prompt other examples, and I gave these only to show the need to set any two parameters to find all the others. That's what we're going to do. Namely, we take five segment parameters: D, L, X, φ and H. Then, choosing all possible pairs from them, we will consider them as initial data and find all the rest by brainstorming.
In order not to burden the reader in vain, I will not give detailed solutions, but will only give results in the form of formulas (those cases where there is no formal solution, I will specify along the way).
And one more remark: about units of measure. All quantities, except for the central angle, are measured in the same abstract units. This means that if, for example, you specify one value in millimeters, then the other does not need to be specified in centimeters, and the resulting values \u200b\u200bwill be measured in the same millimeters (and areas in square millimeters). The same can be said for inches, feet and nautical miles.
And only the central angle in all cases is measured in degrees and nothing else. Because, as practice shows, people who design something round are not inclined to measure angles in radians. The phrase "the angle of pi by four" confuses many, while the "angle of forty-five degrees" is understandable to everyone, since it is only five degrees above the norm. However, in all formulas there will be one more angle - α - as an intermediate value. In terms of meaning, this is half the central angle, measured in radians, but you can safely not delve into this meaning.
1. Diameter D and arc length L are given
; chord length  ;
;
segment height ![]() ; central corner
; central corner ![]() .
.
2. Diameter D and chord length X are given
![]() ; arc length;
; arc length;
segment height ![]() ; central corner
; central corner ![]() .
.
Since the chord divides the circle into two segments, this problem has not one, but two solutions. To get the second, you need to replace the angle α with the angle in the above formulas.
3. Diameter D and central angle φ are given
![]() ; arc length;
; arc length;
chord length  ; segment height
; segment height ![]() .
.
4. Given the diameter D and the height of the segment H
 ; arc length;
; arc length;
chord length  ; central corner
; central corner ![]() .
.
6. Given the length of the arc L and the central angle φ
![]() ; diameter ;
; diameter ;
chord length  ; segment height
; segment height ![]() .
.
8. Given the length of the chord X and the central angle φ
![]() ; arc length
; arc length ![]() ;
;
diameter ; segment height ![]() .
.
9. Given the length of the chord X and the height of the segment H
![]() ; arc length
; arc length ![]() ;
;
diameter ; central corner ![]() .
.
10. Given the central angle φ and the height of the segment H
![]() ; diameter
; diameter ![]() ;
;
arc length; chord length  .
.
The attentive reader could not help but notice that I missed two options:
5. Given the length of the arc L and the length of the chord X
7. Given the length of the arc L and the height of the segment H
These are just those two unpleasant cases when the problem does not have a solution that could be written in the form of a formula. And the task is not so rare. For example, you have a flat piece of length L, and you want to bend it so that its length becomes X (or its height becomes H). What diameter to take a mandrel (crossbar)?
This task is reduced to solving the equations: ![]() ; - in option 5
; - in option 5
; - in option 7
and although they are not solved analytically, they are easily solved programmatically. And I even know where to get such a program: on this very site, under the name . Everything that I tell here at length, she does in microseconds.
To complete the picture, let's add to the results of our calculations the circumference and three values of areas - a circle, a sector and a segment. (The areas will help us a lot when calculating the mass of any round and semicircular parts, but more on that in a separate article.) All these quantities are calculated using the same formulas:
circumference ;
area of a circle  ;
;
sector area ![]() ;
;
segment area ![]() ;
;
And in conclusion, let me remind you once again about the existence of an absolutely free program that performs all of the above calculations, freeing you from the need to remember what arc tangent is and where to look for it.
The part of a figure that forms a circle whose points are equidistant is called an arc. If, from the point of the center of the circle, the rays are drawn to points coinciding with the ends of the arc, its central angle will be formed.
Determining the length of an arc
Produced according to the following formula:
where L is the desired length of the arc, π = 3.14, r is the radius of the circle, α is the central angle.
| L | 3.14×10×85 |
14,82 |
The length of the arc of a circle is 14.82 centimeters.
In elementary geometry, an arc is understood as a subset of a circle located between two points located on it. In practice, solve problems definition her length engineers and architects often have to, since this geometric element is widespread in a wide variety of designs.
Perhaps the first to face this task were the ancient architects, who one way or another had to determine this parameter for the construction of vaults, widely used to bridge the gaps between supports in round, polygonal or elliptical buildings. If you look closely at the masterpieces of ancient Greek, Roman and especially Arabic architecture that have survived to this day, you will notice that arcs and vaults are extremely common in their designs. The creations of modern architects are not so rich in them, but these geometric elements are present, of course, in them.
Length various arcs it is necessary to calculate during the construction of roads and railways, as well as autodromes, and in many cases, traffic safety largely depends on the correctness and accuracy of calculations. The fact is that many turns of highways from the point of view of geometry are precisely arcs, and various physical forces act on transport along them. The parameters of their resultant are largely determined by the length of the arc, as well as its central angle and radius.
Designers of machines and mechanisms have to calculate the lengths of various arcs for the correct and accurate layout of the components of various units. In this case, errors in calculations are fraught with the fact that important and critical parts will interact incorrectly with each other and the mechanism simply will not be able to function as its creators plan. Examples of designs rich in geometric elements such as arcs include internal combustion engines, gearboxes, wood and metalworking equipment, body parts for cars and trucks, etc.
arcs quite widely found in medicine, in particular, in dentistry. For example, they are used to correct malocclusion. Corrective elements, called braces (or bracket systems) and having the appropriate shape, are made from special alloys and are installed in such a way as to change the position of the teeth. It goes without saying that in order for the treatment to be successful, these arcs must be very precisely calculated. In addition, arcs are widely used in traumatology, and perhaps the most striking example of this is the famous Ilizarov apparatus, invented by a Russian doctor in 1951 and extremely successfully used to this day. Its integral parts are metal arcs, equipped with holes through which special knitting needles are threaded, and which are the main supports of the entire structure.
The formula for finding the length of an arc of a circle is quite simple, and very often in important exams such as the USE there are such problems that cannot be solved without using it. You also need to know it to pass international standardized tests, such as SAT and others.
What is the length of the arc of a circle?
The formula looks like this:
l = prα / 180°
What is each of the elements of the formula:
- π - Pi number (constant value equal to ≈ 3.14);
- r is the radius of the given circle;
- α - the value of the angle on which the arc rests (central, not inscribed).
As you can see, in order to solve the problem, r and α must be present in the condition. Without these two quantities, the arc length cannot be found.
How is this formula derived and why does it look like this?
Everything is extremely easy. It will become much clearer if you put 360 ° in the denominator, and add a deuce in the numerator in front. You can also α do not leave it in a fraction, print it and write it with a multiplication sign. This is quite possible to afford, since this element is in the numerator. Then the general view will be like this:
l = (2πr / 360°) × α
Just for convenience, we reduced 2 and 360 °. And now, if you look closely, you can see a very familiar formula for the length of the entire circle, namely - 2pr. The whole circle consists of 360 °, so we divide the resulting measure into 360 parts. Then we multiply by the number α, that is, for the number of "pieces of the pie" that we need. But everyone knows for sure that a number (that is, the length of the entire circle) cannot be divided by a degree. What to do in this case? Usually, as a rule, the degree is reduced with the degree of the central angle, that is, with α. After that, only numbers remain, and as a result, the final answer is obtained.

This can explain why the length of the arc of a circle is found in this way and has this form.
An example of a problem of medium complexity using this formula
Condition: There is a circle with a radius of 10 centimeters. The degree measure of a central angle is 90°. Find the length of the circular arc formed by this angle.
Solution: l = 10π × 90° / 180° = 10π × 1 / 2=5π
Answer: l = 5π
It is also possible that instead of the degree measure, the radian measure of the angle would be given. In no case should you be scared, because this time the task has become much easier. To convert a radian measure to a degree measure, you need to multiply this number by 180 ° / π. So now we can substitute instead α the following combination: m × 180° / π. Where m is the radian value. And then 180 and the number π are reduced and a completely simplified formula is obtained, which looks like this:
- m is the radian measure of the angle;
- r is the radius of the given circle.
How well do you remember all the names associated with the circle? Just in case, we recall - look at the pictures - refresh your knowledge.
Firstly - The center of a circle is a point from which all points on the circle are the same distance.
Secondly - radius - a line segment connecting the center and a point on the circle.

There are a lot of radii (as many as there are points on a circle), but all radii have the same length.
Sometimes for short radius they call it segment length"the center is a point on the circle", and not the segment itself.
And here's what happens if you connect two points on a circle? Also a cut?
So, this segment is called "chord".


Just as in the case of the radius, the diameter is often called the length of a segment connecting two points on a circle and passing through the center. By the way, how are diameter and radius related? Look closely. Of course, the radius is half the diameter.

In addition to chords, there are also secant.

Do you remember the simplest?
The central angle is the angle between two radii.

And now the inscribed angle
An inscribed angle is the angle between two chords that intersect at a point on a circle.

In this case, they say that the inscribed angle relies on an arc (or on a chord).
Look at the picture:


Measuring arcs and angles.
Circumference. Arcs and angles are measured in degrees and radians. First, about degrees. There are no problems for angles - you need to learn how to measure the arc in degrees.

Degree measure (arc value) is the value (in degrees) of the corresponding central angle
What does the word "corresponding" mean here? Let's look carefully:

See the two arcs and the two central angles? Well, a larger arc corresponds to a larger angle (and it's okay that it is larger), and a smaller arc corresponds to a smaller angle.
So, we agreed: the arc contains the same number of degrees as the corresponding central angle.
And now about the terrible - about radians!
What kind of animal is this "radian"?
Imagine this: radians are a way of measuring an angle... in radii!
A radian angle is a central angle whose arc length is equal to the radius of the circle.
Then the question arises - how many radians are in a straightened angle?

In other words: how many radii "fit" in half a circle? Or in another way: how many times the length of half a circle is greater than the radius?
This question was asked by scientists in ancient Greece.
And so, after a long search, they found that the ratio of the circumference to the radius does not want to be expressed in “human” numbers, like, etc.
And it is not even possible to express this attitude through the roots. That is, it turns out that one cannot say that half of the circle is twice or times the radius! Can you imagine how amazing it was to discover people for the first time?! For the ratio of the length of a half circle to the radius, “normal” numbers were enough. I had to enter a letter.
So, is a number expressing the ratio of the length of a semicircle to the radius.
Now we can answer the question: how many radians are in a straight angle? It has a radian. Precisely because half of the circle is twice the radius.
Ancient (and not so) people through the ages (!) they tried to calculate this mysterious number more precisely, to express it better (at least approximately) through "ordinary" numbers. And now we are impossibly lazy - two signs after busy are enough for us, we are used to
Think about it, this means, for example, that y of a circle with a radius of one is approximately equal in length, and it is simply impossible to write down this length with a “human” number - you need a letter. And then this circumference will be equal. And of course, the circumference of the radius is equal.
Let's get back to radians.
We have already found out that a straight angle contains a radian.

What we have:
So glad, that is glad. In the same way, a plate with the most popular angles is obtained.
The ratio between the values of the inscribed and central angles.
There is an amazing fact:
The value of the inscribed angle is half that of the corresponding central angle.

See how this statement looks in the picture. A "corresponding" central angle is one in which the ends coincide with the ends of the inscribed angle, and the vertex is in the center. And at the same time, the “corresponding” central angle must “look” at the same chord () as the inscribed angle.
Why so? Let's look at a simple case first. Let one of the chords pass through the center. After all, that happens sometimes, right?

What happens here? Consider. It is isosceles - after all, and are radii. So, (denoted them).
Now let's look at. This is the outside corner! We recall that an external angle is equal to the sum of two internal ones that are not adjacent to it, and write:
I.e! An unexpected effect. But there is also a central angle for the inscribed.

So, for this case, we proved that the central angle is twice the inscribed angle. But it is a painfully special case: is it true that the chord does not always go straight through the center? But nothing, now this special case will help us a lot. See: second case: let the center lie inside.

Let's do this: draw a diameter. And then ... we see two pictures that have already been analyzed in the first case. Therefore, we already have
So (on the drawing, a)
Well, the last case remains: the center is outside the corner.

We do the same: draw a diameter through a point. Everything is the same, but instead of the sum - the difference.
That's all!
Let's now form two main and very important consequences of the statement that the inscribed angle is half the central one.
Corollary 1
All inscribed angles intersecting the same arc are equal.
We illustrate:

There are countless inscribed angles based on the same arc (we have this arc), they can look completely different, but they all have the same central angle (), which means that all these inscribed angles are equal between themselves.
Consequence 2
The angle based on the diameter is a right angle.
Look: which corner is central to?

Certainly, . But he is equal! Well, that's why (as well as a lot of inscribed angles based on) and is equal to.
Angle between two chords and secants
But what if the angle we are interested in is NOT inscribed and NOT central, but, for example, like this:

or like this?

Is it possible to somehow express it through some central angles? It turns out you can. Look, we're interested.
a) (as outside corner for). But - inscribed, based on the arc - . - inscribed, based on the arc - .

For beauty they say:

The angle between chords is equal to half the sum of the angular values of the arcs included in this angle.
This is written for brevity, but of course, when using this formula, you need to keep in mind the central angles
b) And now - "outside"! How to be? Yes, almost the same! Only now (again apply the property of the outer corner to). That is now.

And that means . Let's bring beauty and brevity in the records and formulations:

The angle between the secants is equal to half the difference in the angular values of the arcs enclosed in this angle.
Well, now you are armed with all the basic knowledge about the angles associated with a circle. Forward, to the assault of tasks!
CIRCLE AND INCORDED ANGLE. MIDDLE LEVEL
What is a circle, even a five-year-old child knows, right? Mathematicians, as always, have an abstruse definition on this subject, but we will not give it (see), but rather remember what the points, lines and angles associated with a circle are called.
Important Terms
Firstly:
| circle center- a point from which the distances from which to all points of the circle are the same. |
Secondly:
There is another accepted expression here: "the chord contracts the arc." Here, here in the figure, for example, a chord contracts an arc. And if the chord suddenly passes through the center, then it has a special name: "diameter".
By the way, how are diameter and radius related? Look closely. Of course,
And now - the names for the corners.
Naturally, isn't it? The sides of the corner come out from the center, which means that the corner is central.
This is where difficulties sometimes arise. Pay attention - NOT ANY angle inside a circle is an inscribed, but only one whose vertex "sits" on the circle itself.
Let's see the difference in the pictures:
They also say differently:
There is one tricky point here. What is a “corresponding” or “own” central angle? Just an angle with vertex at the center of the circle and ends at the ends of the arc? Not certainly in that way. Look at the picture.
One of them, however, does not even look like a corner - it is larger. But in a triangle there cannot be more angles, but in a circle - it may well! So: a smaller arc AB corresponds to a smaller angle (orange), and a larger one to a larger one. Just like, isn't it?
Relationship between inscribed and central angles
Remember a very important statement:
In textbooks, they like to write the same fact like this:
True, with a central angle, the formulation is simpler?
But still, let's find a correspondence between the two formulations, and at the same time learn to find in the figures the "corresponding" central angle and the arc on which the inscribed angle "leans".
Look, here is a circle and an inscribed angle:
Where is its "corresponding" central angle?
Let's look again:
What is the rule?
But! In this case, it is important that the inscribed and central angles "look" on the same side of the arc. For example:
Oddly enough, blue! Because the arc is long, longer than half the circle! So don't ever get confused!
What consequence can be deduced from the "halfness" of the inscribed angle?
And here, for example:
Angle Based on Diameter
Have you already noticed that mathematicians are very fond of talking about the same thing in different words? Why is it for them? You see, although the language of mathematics is formal, it is alive, and therefore, as in ordinary language, every time you want to say it in a way that is more convenient. Well, we have already seen what “the angle rests on the arc” is. And imagine, the same picture is called "the angle rests on the chord." On what? Yes, of course, on the one that pulls this arc!
When is it more convenient to rely on a chord than on an arc?
Well, in particular, when this chord is a diameter.
There is an amazingly simple, beautiful and useful statement for such a situation!
Look: here is a circle, a diameter, and an angle that rests on it.
CIRCLE AND INCORDED ANGLE. BRIEFLY ABOUT THE MAIN
1. Basic concepts.
3. Measurements of arcs and angles.
A radian angle is a central angle whose arc length is equal to the radius of the circle.
This is a number expressing the ratio of the length of a semicircle to the radius.
The circumference of the radius is equal to.
4. The ratio between the values of the inscribed and central angles.
Well, the topic is over. If you are reading these lines, then you are very cool.
Because only 5% of people are able to master something on their own. And if you have read to the end, then you are in the 5%!
Now the most important thing.
You've figured out the theory on this topic. And, I repeat, it's ... it's just super! You are already better than the vast majority of your peers.
The problem is that this may not be enough ...
For what?
For the successful passing of the exam, for admission to the institute on the budget and, MOST IMPORTANTLY, for life.
I will not convince you of anything, I will just say one thing ...
People who have received a good education earn much more than those who have not received it. This is statistics.
But this is not the main thing.
The main thing is that they are MORE HAPPY (there are such studies). Perhaps because much more opportunities open up before them and life becomes brighter? Don't know...
But think for yourself...
What does it take to be sure to be better than others on the exam and be ultimately ... happier?
FILL YOUR HAND, SOLVING PROBLEMS ON THIS TOPIC.
On the exam, you will not be asked theory.
You will need solve problems on time.
And, if you haven’t solved them (LOTS!), you will definitely make a stupid mistake somewhere or simply won’t make it in time.
It's like in sports - you need to repeat many times to win for sure.
Find a collection anywhere you want necessarily with solutions, detailed analysis and decide, decide, decide!
You can use our tasks (not necessary) and we certainly recommend them.
In order to get a hand with the help of our tasks, you need to help extend the life of the YouClever textbook that you are currently reading.
How? There are two options:
- Unlock access to all hidden tasks in this article -
- Unlock access to all hidden tasks in all 99 articles of the tutorial - Buy a textbook - 499 rubles
Yes, we have 99 such articles in the textbook and access to all tasks and all hidden texts in them can be opened immediately.
Access to all hidden tasks is provided for the entire lifetime of the site.
In conclusion...
If you don't like our tasks, find others. Just don't stop with theory.
“Understood” and “I know how to solve” are completely different skills. You need both.
Find problems and solve!