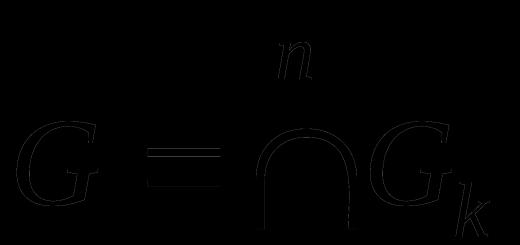
Theorem 3.1. The union of any number of open sets is an open set.

Let G k, where k О N are open sets.

3Select any point X O ÎG. By definition of the union of sets, the point X o belongs to one of the sets G k. Because the G k is an open set, then there exists e- neighborhood of a point x o, which lies entirely in the set Gk: U(x o , e)Ì G k Þ U(xo,e)Ì G.

Got that any point x o ÎG– internal, which means that G– open set. 4

Theorem 3.2 . The intersection of a finite number of open non-empty sets is an open set.

Let G k (k = 1,2, …,n) are open sets.

Let us prove that is an open set.

3Select any point X O ÎG. By definition of intersection of sets X o belongs to each of the sets G k. Since every set G k open, then in any set G k exists e k- neighborhood of a point X O : U(x o , e k)Ì G k. Lots of numbers( e 1 , e 2 ,…, e n) is finite, so there is a number e = min{e 1 ,e 2 ,…,e n). Then e- neighborhood of a point X o is in every e k- neighborhood of a point X O :U(x o , e)M U e(x o , e k) Þ U(x o , e)Ì G.

Got that X o – interior point of the set G, which means that G– open set. 4

Remark 3.1. The intersection of an infinite number of open sets may not be an open set.

Example 3.1. Let in space R G k =(2 – 1/k; 4+ 1/k), Where k= 1,2,…,n,…. G 1 =(1;5), G 2(1.5;4.5), Segment Ì G k and is not an open set, points 2 and 4 are not internal.

Theorem 3.3 . The intersection of any collection of closed non-empty sets is a closed set.

Let Fk- closed sets.

Let us prove that the set is closed, i.e. it contains all its limit points.

3Let X F. From the definition of intersection of sets it follows that in any e- neighborhood of a point X o there are infinitely many points of each set Fk, which means that X o – limit point of each set Fk. Due to the closedness of the sets Fk dot

X O О F k "k Þ x O Î F. Since the point X F, and that means a lot F closed. 4

Theorem 3.4. The union of a finite number of closed sets is a closed set.

Let each set Fk closed.

Let us prove that the set is closed, i.e., if X o – limit point of the set F, That X O О F.

3Let X o – any limit point of the set F, then at any e- neighborhood of a point X o there are infinitely many points of the set. Since the number of sets Fk finite, then X o belongs to at least one of the sets Fk, i.e. X o is the limit point for this set.

Due to isolation Fk dot X o belongs Fk, and therefore many. Since the point X o is chosen arbitrarily, then all limit points belong to the set F, which means a lot F closed. 4

Remark 3.2. The union of an infinite number of closed sets can be an open set.

Example 3.2 . In space R: F k =

F 1 =; F 2 = ; …. The interval (2;5) is an open set.

Let us accept without proof Theorems 3.5 and 3.6 related to the complement of the set E to many X: C x E=CE.

Theorem 3.5 . If the set E closed, then its complement SE open set.

Example 3.3 . E=, C R E =(- ¥, 2)È (5,+¥ ).

Theorem 3.6 . If the set E open, then its complement SE closed set.

Example 3.4 . E=(2,5), C R E =(-¥, 2]È[ 5, +¥ ).

Proof.

1) Indeed, if the point A belongs to the union of open sets, then it belongs to at least one of these sets, which, by the conditions of the theorem, is open. This means that it belongs to a certain neighborhood O(a) of the point A, but then this neighborhood also belongs to the union of all open sets. Therefore, the point A is the internal union point. Because A is an arbitrary union point, then it consists only of internal points, and therefore, by definition, is an open set.

2) Let now X– intersection of a finite number of open sets. If A is a set point X, then it belongs to each of the open sets, and, therefore, is an interior point of each of the open sets. In other words, there are intervals that are entirely contained in the sets, respectively. Let us denote by the smallest of the numbers. Then the interval will be contained simultaneously in all intervals, i.e. will be entirely contained in , and in ,..., and in , i.e. . From here and we conclude that any point is an interior point of the set X, i.e. a bunch of X is open.

From this theorem it follows that the intersection of a finite number of neighborhoods of a point a is again a neighborhood of this point. Note that the intersection of an infinite number of open sets is not always an open set. For example, the intersection of intervals ,... is a set consisting of one point a, which is not an open set (why?).

A point a is called a limit point of a set X if in any punctured neighborhood of this point there is at least one point of the set X.

So, the point is the limit point of the segment , since in any punctured interval of a point there is a point belonging to this segment. For example, a point that satisfies the inequality . And there are obviously many such points.

It is easy to prove that each point of the segment [ 0, 1] is ultimate point of this segment. In other words, the segment consists entirely of its limit points. A similar statement is true for any segment. Note here that all limit points of the set belong to this segment. It is also obvious that all points of the segment will be limit points for the interval (0, 1 ) (prove it!). However, there are already two limiting points 0 and 1 do not belong to the interval (0, 1). In these examples we see that

the limit points of a set may or may not belong to it. It can be proven that in any punctured neighborhood of a limit point a of a set X there are infinitely many points of the set X.

A set X is called a closed set if it contains all its limit points.

So, every segment is a closed set. Interval (0, 1) is not a closed set, since its two limit points do not belong to it 0 and 1. The set of all rational numbers Q is not closed, since it does not contain some of its limit points. In particular, the number is the limit point of the set Q(prove it!), but Q.

Since each point of the set R is the limit point of this set and belongs to it, then R – closed set.

Every finite set is closed, since the set of its limit points is the empty set Æ , which belongs to the set itself.

Closed sets can be bounded, for example, the segment, and unbounded, for example, the set of real numbers R. True

Plan

1. Vector space .
2. Interior point of a set. Open set in space
3. Properties of open sets
4. Limit point of a set. Closed sets in space
5. Properties of closed sets in space

1. Vector space . The concept of metrics. Metric properties

Let be. Elements of space are vectors, where. Two operations have been introduced in space: addition of vectors and multiplication of a vector by a scalar, the properties of which are discussed in the course of algebra and geometry.

Let's define the vector norm as a function:

The vector norm function satisfies the following properties:

Definition 1. Distance in space between vectors is called

Distance properties:

1. i if and only if;

Definition 2. Let be. An open ball of radius centered at a point (denoted) is the set of points such that

Example. - this is the interval (Fig. 1).

Example. (Fig. 2).

Definition 3. Let be. A closed ball of radius with center at a point (denoted) is the set of points such that

Definition 4. A point is called an interior point of this set if there is an open ball that is completely contained in the set.

Definition 5. A set is called an open set if each of its points is an interior point.

Example. The empty set and set are open sets.

Example. Prove that is an open set (Fig. 3).

Let's take it. It means that. Let's denote Consider an open ball. Let's prove that. To do this, let us show what simultaneously belongs to:

Thus, and this means that.

Definition 6. An open parallelepiped in is a set of points for which the following inequalities hold:

Exercise. Show that an open parallelepiped is an open set.

Theorem 1. The intersection of any finite number of open sets is an open set.

Proof. Let be open sets, . Let us show that is an open set. To do this, let’s take and show that this point is internal to:

Since every set is open, there is an open ball for. Let's denote Then

Thus, is internal for this set, and the set itself is open.

Comment. The intersection of an infinite number of open sets may not be an open set.

Example. Let us consider an infinite collection of open sets For them. A set that contains one point is not open.

Theorem 2. The union of any number of open sets is an open set.

Proof. Let be some set of indices. Let the set be open for. Let's consider. Let us show that it is open. To do this, let’s take and show that this point is internal to:

Since is an open set, then, and this means that is an open set.

One of the main tasks of the theory of point sets is the study of the properties of various types of point sets. Let's get acquainted with this theory using two examples and study the properties of the so-called closed and open sets.

The set is called closed , if it contains all its limit points. If a set does not have a single limit point, then it is also considered closed. In addition to its limit points, a closed set can also contain isolated points. The set is called open , if each of its points is internal for it.

Let's give examples of closed and open sets .

Every segment is a closed set, and every interval (a, b) is an open set. Improper half-intervals and closed, and improper intervals and open. The entire line is both a closed and an open set. It is convenient to consider the empty set to be both closed and open at the same time. Any finite set of points on a line is closed, since it has no limit points.

A set consisting of points:

closed; this set has a unique limit point x=0, which belongs to the set.

The main task is to find out how an arbitrary closed or open set is structured. To do this, we will need a number of auxiliary facts, which we will accept without proof.

• 1. The intersection of any number of closed sets is closed.
• 2. The sum of any number of open sets is an open set.
• 3. If a closed set is bounded above, then it contains its supremum. Similarly, if a closed set is bounded below, then it contains its infimum.

Let E be an arbitrary set of points on a line. Let us call the complement of the set E and denote by CE the set of all points on the line that do not belong to the set E. It is clear that if x is an external point for E, then it is an internal point for the set CE and vice versa.

4. If a set F is closed, then its complement CF is open and vice versa.

Proposition 4 shows that there is a very close connection between closed and open sets: some are complements of others. Because of this, it is sufficient to study only closed or only open sets. Knowing the properties of sets of one type allows you to immediately find out the properties of sets of another type. For example, any open set is obtained by removing some closed set from a line.

Let's start studying the properties of closed sets. Let's introduce one definition. Let F be a closed set. An interval (a, b) having the property that none of its points belong to the set F, but the points a and b belong to F, is called an adjacent interval of the set F.

We will also include improper intervals as adjacent intervals, or if point a or point b belongs to the set F, and the intervals themselves do not intersect with F. Let us show that if a point x does not belong to a closed set F, then it belongs to one of its adjacent intervals.

Let us denote by the part of the set F located to the right of the point x. Since the point x itself does not belong to the set F, it can be represented in intersection form:

Each of the sets is F and closed. Therefore, by Proposition 1, the set is closed. If the set is empty, then the entire half-interval does not belong to the set F. Let us now assume that the set is not empty. Since this set is entirely located on a half-interval, it is bounded below. Let us denote its lower bound by b. According to Proposition 3, which means. Further, since b is the infimum of the set, the half-interval (x, b) lying to the left of the point b does not contain points of the set and, therefore, does not contain points of the set F. So, we have constructed a half-interval (x, b) not containing points of the set F, and either or the point b belongs to the set F. Similarly, a half-interval (a, x) is constructed that does not contain points of the set F, and either or. Now it is clear that the interval (a, b) contains the point x and is an adjacent interval of the set F. It is easy to see that if and are two adjacent intervals of the set F, then these intervals either coincide or do not intersect.

From the previous it follows that any closed set on a line is obtained by removing a certain number of intervals from the line, namely adjacent intervals of the set F. Since each interval contains at least one rational point, and there is a countable set of all rational points on the line, it is easy make sure that the number of all adjacent intervals is at most countable. From here we get the final conclusion. Every closed set on a line is obtained by removing from the line at most a countable set of disjoint intervals.

By virtue of Proposition 4, it immediately follows that every open set on a line is nothing more than a countable sum of disjoint intervals. By virtue of Propositions 1 and 2, it is also clear that any set arranged as indicated above is indeed closed (open).

As can be seen from the following example, closed sets can have a very complex structure.

§6. Theorems on open and closed sets

Theorem 1. The union of any number of open sets is an open set.

Let G k – open sets.

Let us prove that is an open set.

Take any point X O  G. By definition of the union of sets, the point X O will belong to at least one of the sets G k . Because G k are open sets, then there exists  - neighborhood of a point X O, which completely belongs to the set G k :

Got that any point X O  G – internal, which means that G– open set. 

Theorem 2 . The intersection of a finite number of open non-empty sets is an open set.

Let G k ( k = 1,2, …,n) are open sets.

Let's prove that
– open set.

Take any point X O  G. By definition of intersection of sets X O belong to each of the sets G k. Because sets G k open, then in any set G k exists  k- neighborhood of a point X O : U( x o ,  k)  G k. Lots of numbers { 1 ,  2 ,…,  n ) is finite, therefore   = min { 1 ,  2 ,…,  n). Then  - neighborhood of a point X O belongs to everyone  k- neighborhood of a point X O :

Got that X O– interior point of the set G, which means that G– open set. 

Note 1. The intersection of an infinite number of open sets may not be an open set.

Example 1 . Let in space R Where k= 1,2,…,n, ….

Theorem 3 . The intersection of an infinite number of closed nonempty sets is a closed set.

Let F k– closed sets.

Let us prove that the sets
closed, i.e. it contains all its limit points.

Theorem 4. The union of a finite number of closed nonempty sets is a closed set.

Let the sets F k- closed.

Let us prove that the set
closed, i.e., if X O F, That X O  F.

Note 2. The union of an infinite number of closed sets can be an open set.

Example 2 . In space R: F k =

Theorem 5 . If the set E closed, then its complement to the set X: C X E=CE – open set.

Example .3 . E=, C R E =

Theorem 6 . If the set E open, then its complement to the set X: C X E=CE – closed set.

Example 4 . E=(2,5), C R E =

§7. Sequences of points in metric space

Definition 1 . A sequence of points in a metric space (X,  ) called mapping f set of natural numbers N into the multitude X: f: NX.

The value of this mapping at a point n  N called n-member of the sequence of points in the metric space and is denoted by x n = f(n). We will denote the sequence (x n) or ( X 1 ,X 2 ,…, X n … ).

Example 1. In space R 2 : X n = (1 n, n+ 1/ n));

Example 2 . In space WITH: (X n = (1/ nx + n 2 x)) where  a,b does not contain 0.

Definition 2 . Let ( x nX, ), (k 1 , k 2 ,…, k n ,… ) is an increasing sequence of natural numbers. Then the sequence (x kn) is called subsequence of the sequence (x n).

Example 3. Sequence (1 / n 2 ) – subsequence of the sequence (1 / n).

Definition 3 . Let ( x n) X, ), Subsequence (x n) is called limited , if there is a closed ball with center A and a final radius R, which contains all members of the sequence, i.e.

.

Note 1 . The concept of a monotone sequence cannot be introduced in all metric spaces.

Definition 4. Let ( x n) – sequence of points of metric space ( X, ). Dot A X called limit of the sequence (x n) If:

  (    N   n   ( , n  N   x n , a  

or, which is the same thing, a number sequence (  x n , a)) - infinitesimal (tends to 0), with n ,those.

and abazanaetstsa

by metric  or
, at n .

If the sequence ( x n) has a finite limit, then it is called convergent, otherwise it is called divergent.

If ( x n) – sequence of points of metric space ( X, ) converges to a point A X, That A– limit point of the sequence ( x n).

The opposite is not always the case.

Note 2 . The same sequence in different metric spaces can both converge and diverge

Example 4. Sequence (1 / n) converges in the space R, but diverges in the space ( X, ), Where
 (x, y)=  X at, because 0
.

The theorems hold for convergent sequences.

Theorem 1. If ( x n) – convergent sequence of metric space ( X, ), then its limit is unique.

  x n ,a 0 And
  x n ,b 0.

According to the axioms of the metric 0   a, b    x n , a +  x n , b. We pass to the limit, at n , We get  a, b = 0  a= b. 

Theorem 2 . If ( x n) – sequence of points of metric space ( X, ) – convergent, then it is bounded.

Let
.

Theorem 3 . If ( x n) – sequence of points of metric space ( X, ) converges to a point A X, then any of its subsequences converges to A.

Let
– any subsequence of the sequence (x n). By condition . It means that:      n    x n ,A   .

Because k n  n, then for everyone n> N right k n > N and therefore 
.

Thus we have proven that     n   , it means that
.

§8. Properties of convergent sequences in some

metric spaces

Theorem 1 (on the coordinate-wise convergence of a sequence in m. pr.R m ). In order for a sequence of points in a metric space R m

(X n = (X 1 ( n ) ,X 2 ( n ) ,…, X m ( n ) ) converged to a point A = (A 1 ,A 2 ,…, A m) this space is necessary and sufficient for numerical sequences ( X 1 ( n ) ), (X 2 ( n ) ),…, (X m ( n ) ) (corresponding coordinates) tended accordingly to the numbers A 1 ,A 2 ,…, A m , i.e.

,
,...,
(1)

If equalities (1) are satisfied, then we say that the sequence ( X n) converges to a point A coordinate by coordinate.

1. Let in m.pr. R m . (2)

Let us prove that equalities (1) are satisfied.

By virtue of equality (2) (by definition of the limit of a sequence) in m.p. R m will have:

  n    x n ,A ,

Where  - metric of metric space R m :

x,y R m .

2. Let equalities (1) be satisfied.

Let us prove that (2) in the metric space R m .

Let  - any positive number is considered a number
. Then

Example 1 . Find the limit a = (a 1 , a 2 ) sequences

in space R 2 .

Thus, = (1/4;3).

Theorem 2 (Bolzana-Weierstrasse in m.pr.R m ). From any limited sequence of space R m a convergent subsequence can be identified.

A particular case of this theorem for space R 1 was proven in the first year.

Theorem 3 . In order for the sequence ( x n) points m.pr. WITH [ a , b] with the Chebyshev metric converged to the element X This, etc., is necessary and sufficient for the functional sequence ( x n) converged uniformly to X on [ a, b].

Let us prove it using the criterion of uniform convergence.

It is known that the functional sequence ( x n) converges uniformly to the limit function X then and only when

Taking into account the definition of the metric in m.pr. WITH[a, b] we get equality

(see definition 4 §7)
by metric  in m.pr. WITH[a, b]. 

Example 2. x n (t) = t n  t ;   n N. it is known that on ;/2 the functional sequence x n (t) = t n converges uniformly to the limit function x (t) = 0. Thus  t ; sequence ( x n) converges to the function x = 0 in m.pr. WITH.

Theorem 4. If A– limit point of the set E metric space ( X,  ), then there is a sequence ( x n), whose members belong E and are not equal A, and ( x n), converges to A in this metric space.

The proof is similar to the proof in space R.

Note 1. Since any norm specifies a metric,

 o ( x, y) =

then in normalized space A it is also possible to define the limit of a sequence of elements of a normed space.

Note 2. Since a pre-Hilbert space is a normed space with norm
, then in a pre-Hilbert space one can also define the limit of the sequence of elements of a pre-Hilbert space.

§9. Complete metric spaces

Definition 1 . Subsequence ( x n) metric space ( X, ) is called fundamental if

An example of a fundamental sequence is any convergent sequence of points in a metric space.

In space R any fundamental sequence is convergent. But for any m.pr. not every fundamental sequence of a metric space ( X,  ) converges in this space.

Example 1 . In m.pr. X = (Q;  =  X at) the sequence is fundamental, but from the 1st year it is known that but e X(e I ).

Definition 2 . The metric space is called complete metric space , if any fundamental sequence of points in this space converges in it.

Example 2 . Metric space R is a complete metric space, because any fundamental sequence converges to a number from space R. This follows from the Cauchy criterion (see 1st course).

Example 3 . Let us prove that space R m- complete metric space.

Let the sequence( x n= x 1 (n) , x 2 (n) ,…, x m( n)) (1)

any fundamental sequence of space R m . Let us show that this sequence is convergent and its limit belongs to the space R m .

On the definition of the fundamental sequence and the definition of the metric in space R m

0 N( ) N  p,n >N  (x p ,x n) 

According to the proof of Theorem 1 §8 Thus, the fundamental nature of number sequences ( x 1 ( n ) ), (x 2 ( n ) ),…, (x m ( n ) ), and hence their convergence (according to the Cauchy criterion).

Let

Consider the point a =(A 1 , A 2 , …, A m). Because A 1 , A 2 , …, A m  R, That A R m. By Theorem 1 §8 we obtain that in m.pr. R m subsequence ( x n) converges to A R m . This means that space R m complete metric space. 

Example 4 . Let us prove that the metric space WITH[a, b] is complete.

Let ( x n) – any fundamental sequence in m.p. WITH[a, b] , its terms are continuous on [ a, b] functions.

Let us prove that the sequence ( x n) converges in metric space WITH [ a , b] . First we show that it converges to the limit function X on the segment [ a, b].

By definition of the fundamental sequence

It means that  t [a, b] (fix t) fundamental is the number sequence ( x n (t) ). This means it has a limit, which we denote by
for each fixed t [a, b].

Let us show that the limit function x(t) continuous on [ a, b]. To do this, in inequality (2) we go to the limit at m . We get

 x (t) x n (t)   n>N t [a,b].

Thus, we have proven that

0 NN  m,n > N x (t) x n (t)   t [a,b].

This means that the sequence ( x n) converges uniformly to the function X on [ a, b]. Because all members of the sequence ( x n) continuous on [ a, b] function, then the limit function is also continuous on this segment, that is, it is an element of the metric space WITH [ a , b]. By Theorem 2 §8 in this space the sequence ( x n) converges to X. This means space WITH [ a , b] – complete metric space. 

Definition 3. A complete normed space is called Banohav th space m .

Banohavian spaces are the spaces:

R P with standards
,
;

l 2 with vector norm x = (x n) = (x 1 , x 2 , … )

C [ a, b] with the norm of functions x(t)
.

And space C 1 [a, b] with norm is not Banoch.

Definition 2 . A complete pre-Hilbert space with respect to norm (2) §3 is called Hilbert space .

Examples of Hilbert spaces are the listed spaces from the examples in §4. The pre-Hilbert space from Example 3 of §4 is not complete with respect to norm (2) and therefore is not Hilbert.

Informatics, 4 year, 1-2 module) Definitionmetricspace(m.p.). Examples. Open and closed sets in m.p. Convergence... linear mappings of normalized spaces. Examples. Normalized space linear mappings. Theorem...

Lecture No. 3 Metric spaces Open and closed sets

Lecture

... spaces. Definition 4. Metricspace is called complete if any fundamental sequence converges in it (to an element of this space!). Examples. 9) B space ...

TO THE STUDY OF METRIC SPACES

Document

What we get is equivalent definitionmetricspace. 4. Prove that for an arbitrary metricspaceáX, rñ are equivalent to the statement... continuous mappings metricspaces continuous. Point to example, What...